Worksheet on Fractions and Decimals on Number Line will let you practice many questions. Practice all the problems available on the Fractions and Decimals on Number Line Worksheet with Answers and gain knowledge of the concept. We have given explanations and answers for every problem in this article. Therefore, cross-check all of your answers after practicing them to check your preparation level. Students of 6th Grade Math can get similar kinds of articles all on our website for free of cost.
Also, find
- Worksheet on Integers and the Number Line
- Addition on a Number Line
- Expressing Numbers on the Number Line
Fractions and Decimals on a Number Line Worksheet PDF
Question 1.
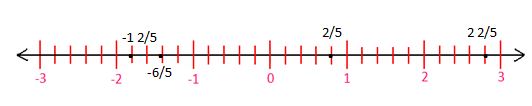
Draw a number line to represent fractions; 2/5, 2 2/5, -1 2/5, and -6/5.
Solution:
Given fractions are 2/5, 2 2/5, -1 2/5, and -6/5.
Firstly, take a number line and divide the number line with 5 equal intervals between integers.

Now, point out the numbers on the above number line.
To represent 2/5 on the number line, move towards the right side of zero as it is a positive number. 2/5 = 0.4
To represent 2 2/5 on the number line, move towards the right side of zero as it is a positive number.
Convert the mixed fraction into decimals to find the exact position of the given number. 2 2/5 = 12/5 = 2.4
To represent -1 2/5 on the number line, move towards the left side of zero as it is a negative number. -1 2/5 = -7/5 = -1.4
To represent -6/5 on the number line, move towards the left side of zero as it is a negative number. -6/5 = -1.2
Question 2.
Represent the following fraction on the same number line
(i) 1/4 (ii) 3/4 (iii) – 7/4 (iv) – 2/4
Solution:
Given fractions are 1/4, 3/4, -7/4, and -2/4.
Firstly, take a number line and divide the number line with 4 equal intervals between integers.
Now, point out the numbers on the above number line.
To represent 1/4 on the number line, move towards the right side of zero as it is a positive number. 1/4 = 0.25
To represent 3/4 on the number line, move towards the right side of zero as it is a positive number. 3/4 = 0.75
To represent -7/4 on the number line, move towards the left side of zero as it is a negative number. -7/4 = -1.75
To represent -2/4 on the number line, move towards the left side of zero as it is a negative number. -2/4 = -0.5
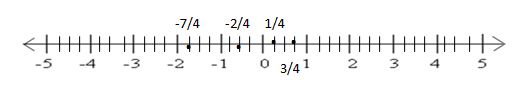
Question 3.
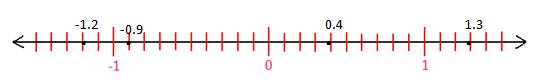
Represent the following decimals on the number line.
(i) 0.4 (ii) 1.3 (iii) -1.2 (iv) -0.9
Solution:
Given decimal numbers are 0.4, 1.3, -1.2, and -0.9
0.4 = 4/10, 1.3 = 13/10, -1.2 = -12/10, -0.9 = 9/10.
Divide the space between the given integers on a number line into 10 equal parts. Each part is equal to 1/10 i.e, 0.1.
To represent 0.4 on the number line, move 4 parts on the right side of zero.
To represent 1.3 on the number line, move 13 parts on the right side of zero.
To represent -1.2 on the number line, move 17 parts on the left side of zero.
To represent -0.9 on the number line, move 9 parts on the left side of zero.
Check the below number line which consists of the given decimal numbers.
Question 4.
Represent the following decimal fraction on the number line.
(i) 1.5 (ii) 0.5 (iii) -1.75 (iv) -0.25
Solution:
Given decimal numbers are 1.5, 0.5, -0.75., and -0.25
1.5 = 15/10, 0.5 = 5/10, -0.75 = -75/10, -0.25 = -25/10.
Divide the space between the given integers on a number line into 4 equal parts. Each part is equal to 1/25 i.e, 0.25.
To represent 1.5 on the number line, move 6 parts on the right side of zero.
To represent 0.5 on the number line, move 2 parts on the right side of zero.
To represent -0.75 on the number line, move 3 parts on the left side of zero.
To represent -0.25 on the number line, move 1 part on the left side of zero.
Check the below number line which consists of the given decimal numbers.
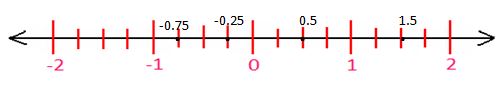
Question 5.
Draw a number line to represent
(i) 1 1/2 (ii) 2.6 (iii) -2½ (iv) -1
Solution:
Given numbers are 1 1/2, 2.6, -2 1/2, and -1.
1 1/2 = 1.5, 2.6, -2 1/2 = -2.5, -1.
Divide the space between the given integers on a number line into 10 equal parts. Each part is equal to 1/10 i.e, 0.1.
To represent 1 1/2 on the number line, move 15 parts on the right side of zero.
To represent 2.6 on the number line, move 2.6 parts on the right side of zero.
To represent -2 1/2 on the number line, move 25 parts on the left side of zero.
To represent -1 on the number line, move 1 part on the left side of zero.
Check the below number line which consists of the given decimal numbers.

Question 6.
If A = -2.6 and B = 1.6 are two points on the same number line. Then, use the number line to find the distance between A and B.
Solution:
Given points are A = -2.6 and B = 1.6.
Represent the two points A and B on a number line.
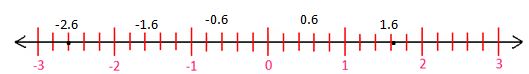
From the number line, there are 4 points differences present between points A and B.
AB = 4 Points
Therefore, the answer is 4 points.
Question 7.
If P = 1.75 and Q = -3/4 are two points on the same number line; use the number line to find the distance between P and Q.
Solution:
Given points are P = 1.75 and Q = -3/4.
Convert the value of Q into a decimal number. Q = -3/4 = -0.75
Represent the two points P and Q on a number line.
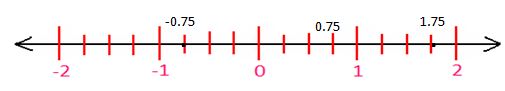
From the number line, there are 2 points differences present between points P and Q.
PQ = 2 Points
Therefore, the answer is 2 points.
See More:
Question 8.
Use a Number line to find the distance between point P and Q, if: (i) A = 1.5 and B = 0.9 (ii) A = -7/4 and B = 1/4
Solution:
(i) Given points are A = 1.5 and B = 0.9.
Represent the two points A and B on a number line.
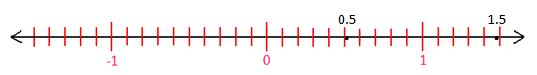
From the number line, there is 0.6 points differences present between points A and B.
AB = 1.5 – 0.9 = 0.6
Therefore, the answer is 0.6 points.
(i) Given points are A = -7/4 and B = 1/4.
Represent the two points A and B on a number line.
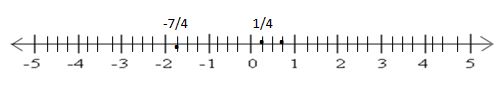
From the number line, there are 1.5 points differences present between points A and B.
AB = -7/4 + 1/4 = 1.5
Therefore, the answer is 1.5 points.
Question 9.
If P = -5/4 and Q = 1.25 are two points on the same number line; use the number line to find the distance between P and Q.
Solution:
Given points are P = -5/4 and Q = 1.25.
Convert the value of P into a decimal number. P = -5/4 = -1.25
Represent the two points P and Q on a number line.
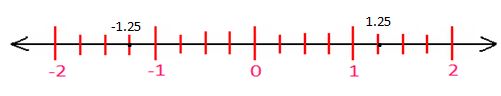
From the number line, there are 3 points differences present between points P and Q.
PQ = 3 Points
Therefore, the answer is 3 points.
Question 10.
If A = 0.5 and B = -15/10 are two points on the same number line; use the number line to find the distance between A and B.
Solution:
Given points are A = 0.5 and B = -15/10.
Convert the value of B into a decimal number. B = -15/10 = -1.5
Represent the two points P and Q on a number line.
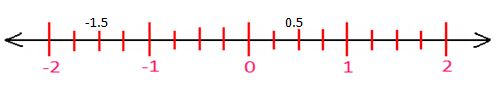
From the number line, there are 2 points differences present between points P and Q.
PQ = 2 Points
Therefore, the answer is 2 points.
