Engage NY Eureka Math Algebra 2 Module 2 Mid Module Assessment Answer Key
Eureka Math Algebra 2 Module 2 Mid Module Assessment Answer Key
Question 1.
a. For each arc indicated below, find the degree measure of its subtended central angle to the nearest degree. Explain your reasoning.
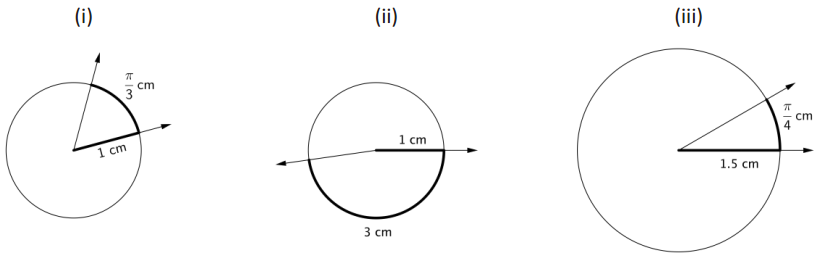
Answer:
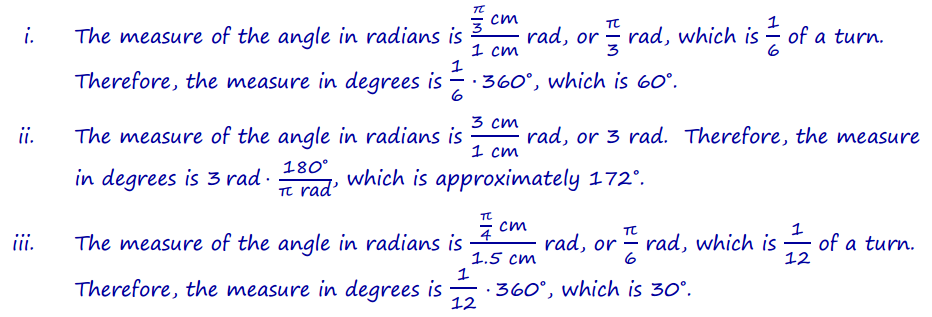
b. Elmo drew a circle with a radius of 1 cm. He drew two radii with an angle of 60° between them and then declared that the radian measure of that angle was \(\frac{\pi}{3}\) cm. Explain why Elmo is not correct in saying this.
Answer:
Angle measurements are not given in length units. He is incorrect in declaring that the angle has units of centimeters.
c. Elmo next drew a circle with a radius of 1 cm. He drew two radii that formed a 60° angle and then declared that the radian measure of that angle is \(\frac{10 \pi}{3}\). Is Elmo correct? Explain your reasoning.
Answer:
This is not correct. The measure of the angle is \(\frac{\pi}{3}\) radians – the measure of an angle is always between 0 and π radians. The amount \(\frac{10 \pi}{3}\) can be thought of as the amount a ray (or a subset of a ray like a radius) has been rotated about the center. In this case, one of the radii can be chosen so that if it is rotated by \(\frac{10 \pi}{3}\) about the center of the circle, its image will be the other radius.
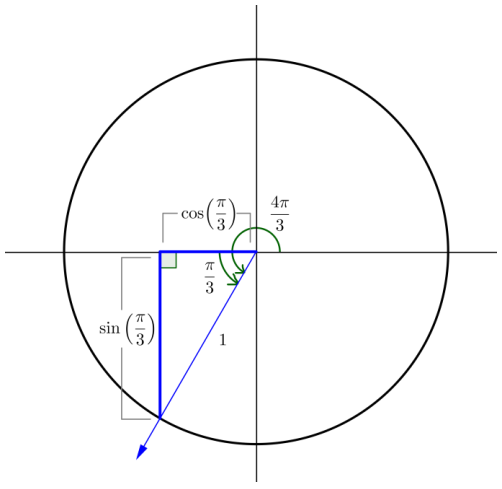
d. Draw a diagram that illustrates why sin\(\left(\frac{4 \pi}{3}\right)=-\frac{\sqrt{3}}{2}\) using the unit circle. Explain how the unit circle helps us to make this calculation.
Answer:

Question 2.
For each part, use your knowledge of the definition of radians and the definitions of sine, cosine, and tangent to place the expressions in order from least to greatest without using a calculator. Explain your reasoning.
a. sin(1°) sin(1) sin(π) sin(60°)
Answer:
Note that one contain is about 57°. Since sin(π) = 0,
sin(π) < sin(1°) < sin(1) < sin(60°)
b. sin(25°) cos(25°) sin\(\left(\frac{3 \pi}{8}\right)\) cos\(\left(\frac{3 \pi}{8}\right)\)
Answer:
There are multiple ways to solve this problem. One way is to convert all expressions to sine because for 0 < θ < \(\frac{\pi}{2}\). We know that cos(25°) = sin(65°) and also cos\(\left(\frac{3 \pi}{8}\right)\) = sin\(\left(\frac{\pi}{8}\right)\). Since \(\frac{3 \pi}{8}\) radians is 67.5° and \(\frac{\pi}{8}\) radians 22.5°, we have 22.5° < 25° < 65° < 67.5°, Thus, sin\(\left(\frac{\pi}{8}\right)\) < sin(25°) < sin(65°) < sin\(\left(\frac{3 \pi}{8}\right)\). Converting back to cosines as needed, we have
cos\(\left(\frac{3 \pi}{8}\right)\) < sin(25°) < cos(25°) < sin\(\left(\frac{3 \pi}{8}\right)\).
c. sin(100°) cos(15°) tan(15°) tan(100°)
Answer:
Note that tan(100°) is negative; all other values are positive. We know that cos(15°) = sin(75°), sin(100°) = sin(80°), and sin(75°) > sin(30°), so
1/2 < cos(15°) < sin(100°).
Looking at the graphs of y = tan(x°) and y = cos(x°), we see that for x < 40, tan(x°) < cos(x°). Thus tan(15°) < cos(15°).
Putting it all together, we see that:
tan(100°) < tan(15°) < cos(15°) < sin (100°)
d. sin(x) sin\(\left(x-\frac{3 \pi}{2}\right)\) sin\(\left(\frac{11 \pi}{4}+x\right)\) sin\(\left(\frac{109 \pi}{107}\right)\)
where x is a very small positive number with x < 0.01
Answer:
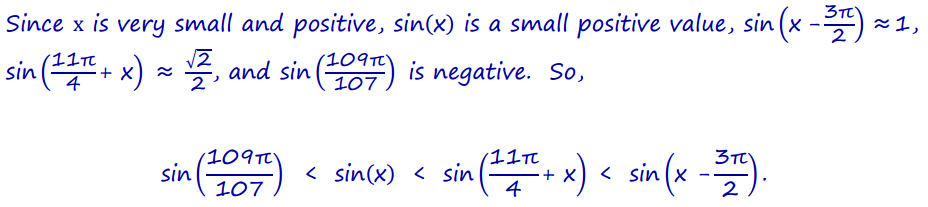
Question 3.
An engineer was asked to design a powered crank to drive an industrial flywheel for a machine in a factory. To analyze the problem, she sketched a simple diagram of the piston motor, connecting rod, and the crank arm attached to the flywheel, as shown below.
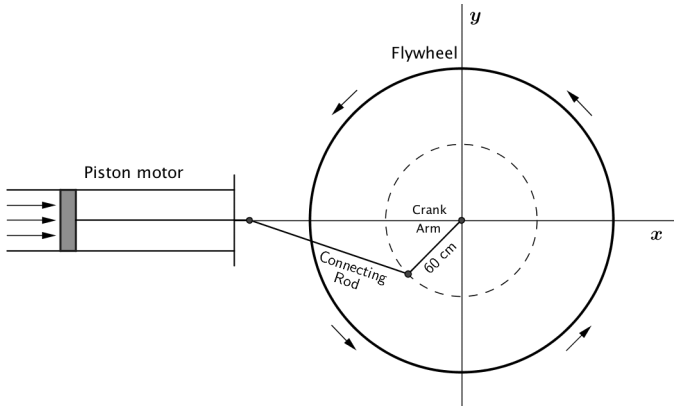
To make her calculations easier, she drew coordinate axes with the origin at the center of the flywheel, and she labeled the joint where the crank arm attaches to the connecting rod by the point P. As part of the design specifications, the crank arm is 60 cm in length, and the motor spins the flywheel at a constant rate of 100 revolutions per minute.
a. With the flywheel spinning, how many radians will the crank arm/connecting rod joint rotate around the origin over a period of 4 seconds? Justify your answer.
Answer:
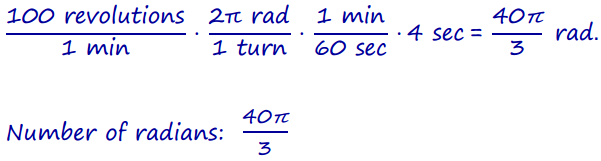
b. With the flywheel spinning, suppose that the joint is located at point P0(O, 60) at time t = 0 seconds; that is, the crank arm and connecting rod are both parallel to the x-axis. Where will the joint be located 4 seconds later?
Answer:
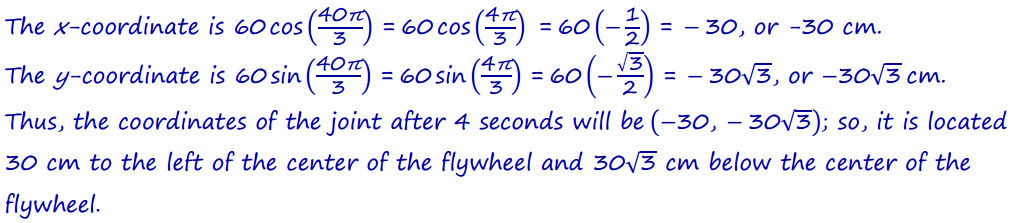
Question 4.
When plotting the graph of y = sin(x), with x measured in radians, Fanuk draws arcs that are semicircles. He argues that semicircles are appropriate because, ¡n his words, “Sine is the height of a point on a circle.”
Here is a picture of a portion of his incorrect graph.
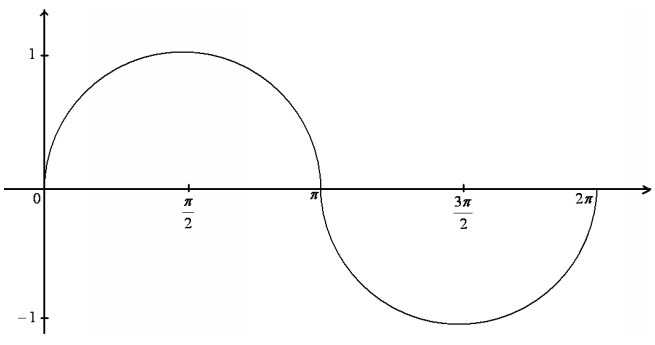
Fanuk claims that the first semicircular arc comes from a circle with center \(\left(\frac{\pi}{2}, 0\right)\).
a. Explain why Fanuk’s claim is incorrect.
Answer:
The first are is NOT a semicircle from a circle with the point \(\left(\frac{\pi}{2}, 0\right)\) as center. There is no consistent “radius” for the first curve of the sine graph. The distance between the purported center \(\left(\frac{\pi}{2}, 0\right)\) and the point (0, 0) on the curve is \(\frac{\pi}{2}\), whereas the distance between \(\left(\frac{\pi}{2}, 0\right)\) and \(\left(\frac{\pi}{2}, 1\right)\) on the curve is 1.
JoJo knows that the arcs in the graph of the sine function are not semicircles, but she suspects each arc might be a section of a parabola.
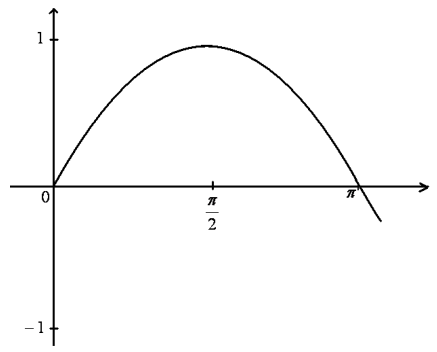
b. Write down the equation of a quadratic function that crosses the x-axis at x = 0 and x = π and has vertex (\(\frac{\pi}{2}\), 1).
Answer:
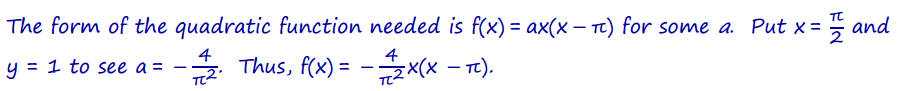
c. Does the arc of a sine curve between x = 0 and x = π match your quadratic function for all values between x = 0 and x = π? Is JoJo correct in her suspicions about the shape of these arcs? Explain.
Answer:

Question 5.
Use the unit circle and the definitions of sine and cosine functions to explain your answer to each question below.
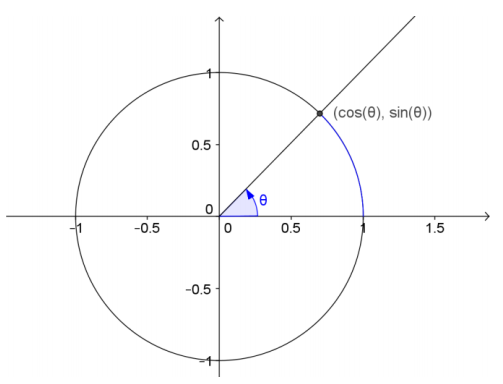
a. Why is sin(3°) so close to 0?
Answer:
When the terminal ray is rotated 3° from the positive x-axis, the y-coordinate of the point on the unity circle where the ray intersects the circle is very small. By definition, the sine function is the y-coordinate. Therefore, sin(3°) will be a small positive number with the rotation is close to 0°.
b. Why is cos(3) so close to -1?
Answer:
When the terminal ray is rotated 3 radians from the positive x-axis, the point on the unit circle where the ray intersects the circle will be very close to the point (-1, 0) since that point corresponds to a rotation of π radians, and 3 is very close to π. By definition, the cosine function is the x-coordinate, so cos(3) will be slightly larger than -1.
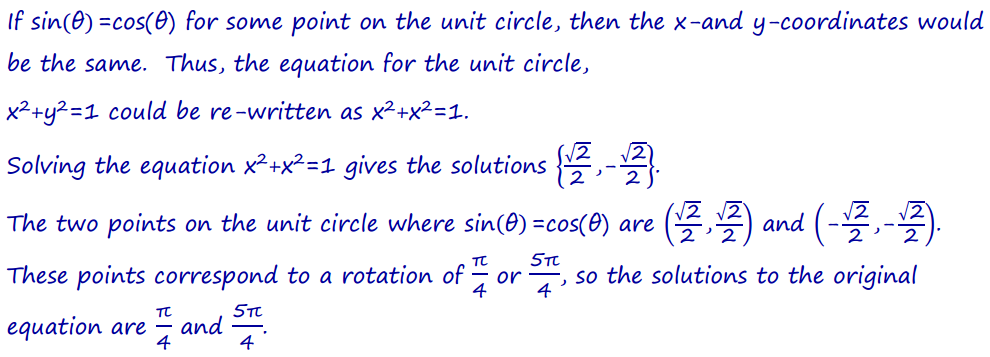
c. Find two solutions to the equation sin(θ) = cos(θ).
Answer: