Angles are formed when two lines intersect each other at a point. The pair of angles are nothing but two angles. The angle pairs can relate to each other in various ways. Those are complementary angles, supplementary angles, vertical angles, adjacent angles, alternate interior angles, alternate exterior angles, and corresponding angles.
Pairs of Angles – Definition
The region between two infinitely long lines pointing a certain direction from a common vertex is called an angle. Which is the amount of turn is measured by an angle. The pairs of angles mean two angles. If there is one common line for two angles, it is known as angle pairs. Get the definitions and examples of all pairs of angles in the following section.
1. Complementary Angles
Two angles whose sum is 90° are called complementary angles and one angle is the complement of another angle.
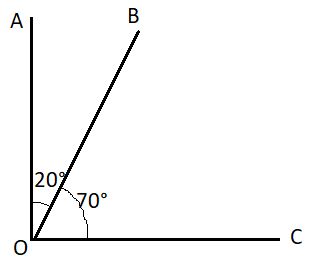
Here, ∠AOB = 20°, ∠BOC = 70°
So, ∠AOB + ∠BOC = 20° + 70° = 90°
Therefore, ∠AOB and ∠BOC are called complementary angles.
∠AOB is a complement of ∠BOC and ∠BOC is the complement of ∠AOB.
Example:
(i) Angles of measure 50° and 40° are complementary angles because 50° + 40° = 90°.
Thus, the complementary angle of 50° is the angle measure 40°. The complementary angle of 40° is the angle measure 50°.
(ii) Complementary of 60° is 90° – 60° = 30°
(iii) Complementary of 45° is 90° – 45°= 45°
(vi) Complementary of 25° is 90 – 25° = 65°
Working rule: To find the complementary angle of a given angle subtracts the measure of an angle from 90°.
So, the complementary angle = 90° – given angle
Also, Read:
2. Supplementary Angles
The pair of angles whose sum is 180° is called the supplementary angles and one angle is called the supplement of the other angle.
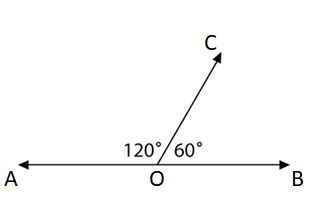
Here, ∠AOC = 120°, ∠COB = 60°
∠AOC + ∠COB = 120° + 60° = 180°
Therefore, ∠AOC, ∠COB are called supplementary angles.
∠AOC is the supplement of ∠COB, ∠COB is a supplement of ∠AOC.
Example:
(i) Angles of measure 90° and 90° are supplementary angles because 90°+ 90° = 180°
Thus, the supplementary angle of 90° is the angle of measure 90°.
(ii) Supplement of 100° is 180° – 100° = 80°
(iii) Supplement of 50° is 180° – 50° = 130°
(iv) Supplement of 95° is 180° – 95°= 85°
(v) Supplement of 140° is 180°- 140° = 40°
Working rule: To find the supplementary angle of the given angle, subtract the measure of angle from 180°.
So, the supplementary angle = 180° – given angle
3. Adjacent Angles
Two non-overlapping angles are said to be adjacent angles if they have a common vertex, common arm, and other two arms lying on the opposite side of this common arm so that their interiors do not overlap.
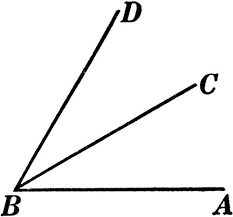
In the above figure, ∠DBC and ∠CBA are non-overlapping, have BC as the common arm and B as the common vertex. The other arms BD, AB of the angles ∠DBC and ∠CBA are opposite sides of the common arm BC.
Hence, the arm ∠DBC and ∠CBA form a pair of adjacent angles.
4. Linear Pair of Angles
The angles are called liner pairs of angles when they are adjacent to each other after the intersection of two lines. Two adjacent angles are said to form a linear pair if their sum is 180°. The types of linear pairs of angles are alternate exterior angles, alternate interior angles, and corresponding angles.
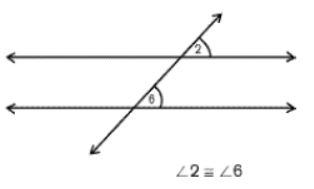
Alternate interior angles
Two angles in the interior of the parallel lines and on opposite sides of the transversal. Alternate interior angles are non-adjacent and congruent.
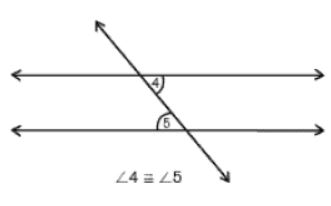
Alternate exterior angles
Two angles in the exterior of the parallel lines, and on the opposite sides of the transversal. Alternate exterior angles are non-adjacent and congruent.
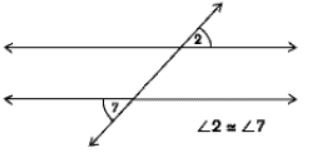
Corresponding angles
The pair of angles, one in the interior and another in the exterior that is on the same side of the transversal. Corresponding angles are non-adjacent and congruent.
5. Vertical Angles
Two angles formed by two intersecting lines having no common arm are called the vertically opposite angles.
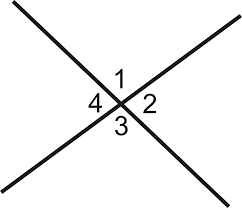
When two lines intersect, then vertically opposite angles are always equal.
∠1 = ∠3
∠2 = ∠4
Pair of Angles Examples
Example 1:
Suppose two angles ∠AOC and ∠ BOC form a linear pair at point O in a line segment AB. If the difference between the two angles is 40°. Then find both the angles.
Solution:
Given that,
∠AOC and ∠BOC form a linear pair
so, ∠AOC + ∠BOC = 180° —- (i)
∠AOC – ∠BOC = 40° —- (ii)
Add both equations
∠AOC + ∠BOC + ∠AOC – ∠BOC = 180° + 40°
2∠AOC = 220°
∠AOC = 220° / 2
∠AOC = 110°
Now, substitute ∠AOC in (i)
110° + ∠ BOC = 180°
∠BOC = 180° – 110°
∠BOC = 70°
Therefore, two angles are 70°, 110°.
Example 2:
Find the values of the angles x, y, and z in the following figure.
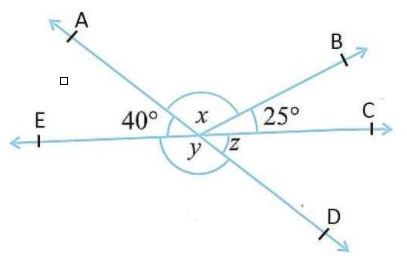
Solution:
From the given figure,
lines AD and EC intersect each other and ∠DOC and ∠AOE are vertically opposite angles
When two lines intersect, then vertically opposite angles are always equal.
So, ∠DOC = ∠AOE
Therefore, z = 40°
AD is a line
∠DOE and ∠AOE are adjacent angles. The sum of adjacent angles are 180°
So, ∠DOE + ∠AOE = 180°
y + 40° = 180°
y = 180°- 40°
y = 140°
And, lines AD and CE intersect
∠DOE, ∠COA are vertically opposite.
When two lines intersect, then vertically opposite angles are always equal.
So, ∠DOE = ∠COA
y = ∠COB + ∠BOAA
140° = x + 25°
140° – 25° = x
x = 115°
Hence, x = 115°, y = 140°, z = 40°
Example 3:
Identify the five pairs of adjacent angles in the following figure.
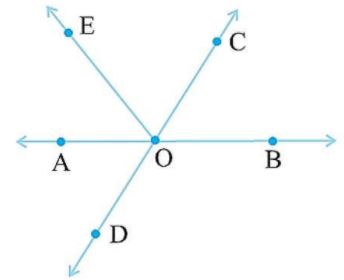
Solution:
Adjacent angles are the angles that have a common side, vertex, and no overlap.
So, (i) ∠AOD, ∠AOE are the adjacent angles
The common side is AO, the common vertex is O and OE, OD is not overlapping.
(ii) ∠AOD, ∠DOB is the adjacent angles
The common vertex is O, the common side is OD and OA, OB is not overlapping.
(iii) ∠DOB, ∠BOC is the adjacent angles
The common side is OB, the common vertex is O, and OD, OC are not overlapping.
(iv) ∠COE, ∠BOC are adjacent angles
The common side is OC, the common vertex is O, and OE, OB are not overlapping.
(v) ∠COE, ∠EOA are adjacent angles
The common vertex is O, the common side is OE, and OC, OA is not overlapping.