Eureka Math Geometry Module 2 Lesson 5 Answer Key

Engage NY Eureka Math Geometry Module 2 Lesson 5 Answer Key

Eureka Math Geometry Module 2 Lesson 5 Opening Exercise Answer Key

Quick Write: Describe how a figure is transformed under a dilation with a scale factor = 1, r > 1, and 0 < r < 1.
Answer:
Sample student responses should include the following points:

A dilation with a scale factor of r = 1 produces an image that is congruent to the original figure.

A dilation with a scale factor of r > 1 produces an ima ge that is larger in size than the original, but the angles of the figure are unchanged, and the lengths of the larger figure are proportional with the original figure.

A dilation with a scale factor of 0 < r < 1 produces an image that is smaller in size than the original, but the angles of the figure are unchanged, and the lengths of the smaller figure are proportional with the original figure.

Discussion
DILATION THEOREM: If a dilation with center 0 and scale factor r sends point P to P’ and Q to Q’, then |P’Q’| = r|PQ|. Furthermore, if r ≠ 1 and O, P, and Q are the vertices of a triangle, then .
Answer:
→ Describe in your own words what the dilation theorem states.

Provide students time to discuss the meaning of the theorem with a partner, and then select students to share their thoughts with the class. Shown below is a sample student response. Consider scripting responses that students might give. Be sure to elicit facts (1) and (2) from students to ensure their understanding of the theorem. Some students may comment on the lengths of segments OP. OP’. QQ, and OQ’. These comments should be acknowledged, but make it clear that the dilation theorem focuses on the parts of a diagram that were not dilated; that is, the points P and Q were dilated, so there is an expectation of what that means and where the images of those points will end up. The focus now with respect to the theorem is on the segments PQ and P’Q’ and what happens to them when the points P and Q are dilated.

The dilation theorem states two things: (1) If two points, P and Q, are dilated from the same center using the same scale factor, then the segment formed when you connect the dilated points P’ and Q’ is exactly the length of \(\overline{P Q}\) multiplied by the scale factor, and (2) the lines containing the segments P’Q’ and PQ are parallel or equal.

For example, if points P and Q are dilated from center O by a scale factor of r = \(\frac{3}{2}\), then the lines containing the segments P’Q’ and PQ are parallel, and P’Q’ = \(\frac{3}{2}\) ∙ PQ, as shown below.

→ The dilation theorem is an important theorem. To prove that the theorem is true, we use the triangle side splitter theorem (from the previous lesson) several times. When we use it, we consider different pairs of parallel lines.

→ Consider the dilation theorem for r = 1. What impact does a scale factor of r = 1 have on a figure and its image? (Encourage students to use what they wrote during the Quick Write activity from the Opening Exercise.)

When the scale factor is r = 1, then the figure remains unchanged. The scale factor being equal to 1 means that each point is taken to itself, that is, a congruence.

→ Consider the dilation theorem with the scenario of O, P, and Q not being the vertices of a triangle but in fact collinear points, as shown below. What impact does that have on the figure and its image?

If the points O, P, and Q are collinear, then the dilated points P’ and Q’ remain on the line, and center O does not move at all.

→ A dilation of collinear points from a center with scale factor r can be used to construct the number line.

To clarify the statement “can be used to construct the number line,” consider showing the series of diagrams below. Begin with the center of dilation at zero and the point x being 1 on the number line, and consider dilations of the point x from the center at zero. The successive lines below show how the point x moves when the scale factor of dilation is increased from r = 2 to r = 4.

→ How is the location of the dilated point x’ related to r and x?
The location of the dilated point was exactly rx.

→ We could continue to dilate the point x by different scale factors to get all of the positive numbers on the number line. We can construct each fraction on the number line by dilating x by that fraction. A rotation of 180° around the center at zero of the whole numbers and fractions is away to construct any rational number. If we considered rational and irrational scale factors, then we get all of the real numbers, that is, the entire real number line.

Note that we do not state that the rational and real number lines can be achieved using a negative scale factor of a dilation. Applying a negative scale factor has the same effect as a 180° rotation (or a reflection across zero) composed with a dilation whose scale factor is the absolute value of the negative scale factor. We do not complicate the issue by introducing a new formalism “negative dilation” into the lesson and, more broadly, the module. Therefore, all dilations have a positive scale factor.

→ Assume that points P and Q are numbers x and y on a number line, and point O, the center of dilation, is the number zero. The points P’ and Q’ correspond to the numbers rx and ry.Explain why the distance between rx and ry is r times the distance between x and y. Use a diagram below if necessary.

Provide students time to discuss with a partner. Then select students to share with the class. A sample student response is shown below.

The distance between points on the number line is the absolute value of their difference. For example, the distance between -2 and 6 is |-2 – 6| = 8 units. Then the distance between rx and ry is |rx – ry|. By the distributive property, |rx – ry| = |r(x – y)|, and since r must be positive, |r(x – y)| = r|x – y|. In terms of the numeric example, if x = -2, y = 6, and r is the scale factor of dilation, then
|r(-2) – r(6)| = |r(-2 – 6)| = r|-2 – 6|.
Therefore, the distance between rx and ry is exactly r times the distance between x and y.

The remaining part of this discussion uses the triangle side splitter theorem to prove the dilation theorem. The goal is to use knowledge about dilation, the triangle side splitter theorem, and theproperties of parallelograms to explain why |P’Q’| = r|PQ| and . Show each step of the proof, and ask students to provide reasoning for the steps independently, with a partner, or in small groups.

Now consider the dilation theorem when O, P, and Q are the vertices of ∆ OPQ. Since P’ and Q’ come from a dilation with scale factor r and center O, we have \(\frac{O P^{\prime}}{O P}=\frac{O Q^{\prime}}{O Q}\) = r.

There are two cases that arise; recall what you wrote in your Quick Write. We must consider the case when r > 1 and when 0 < r < 1. Let’s begin with the latter.

Answer:

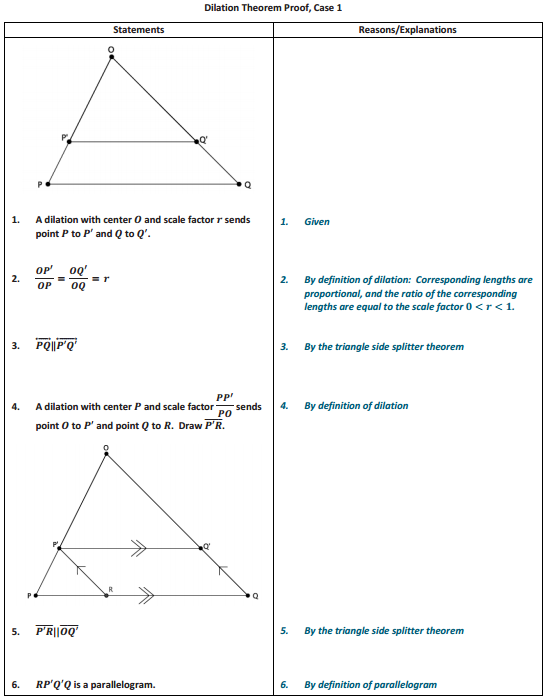
Consider asking students to state what kind of figure is formed by RP’Q’Q and stating what they know about the properties of parallelograms before continuing with the next part of the discussion. Some students may need to see a parallelogram as an isolated figure as opposed to a figure within the triangles.

→ This concludes the proof of Case 1 (when the scale factor of dilation is 0 < r < 1) of the dilation theorem because we have shown that the dilation with center O and scale factor0 < r < 1 sends point P to P’ and Q to Q’, then |P’Q’| = r|PQ|, and since O, P, and Q are the vertices of a triangle, then .

Eureka Math Geometry Module 2 Lesson 5 Exercise Answer Key

Exercise 1.
Prove Case 2: If O, P, and Q are the vertices of a triangle and r > 1, show that (a) and (b) P’Q’ = rPQ. Use the diagram below when writing your proof.

Answer:
Case 2: If r > 1, \(\overline{P Q}\) proportionally splits the sides \(\overline{O P^{\prime}}\) and \(\overline{O Q^{\prime}}\) of ∆OP’Q’. By the triangle side splitter theorem, i – 11. Next, we construct a line segment \(\overline{P R}\) that splits the sides of ∆ OP’Q’ and is parallel to side \(\overline{O Q^{\prime}}\). By the triangle side splitter theorem, \(\overline{P R}\) splits the sides proportionally and so \(\frac{P^{\prime} Q^{\prime}}{R Q^{\prime}}=\frac{O P^{\prime}}{O P}\) = r. Now RQ’ = PQ because the lengths of opposite sides of parallelogram RPQQ’ are equal. So \(\frac{P^{\prime} Q^{\prime}}{P Q}\) = r and |P’Q’|= r|PQ|.

Exercise 2.
a. Produce a scale drawing of ∆ LMN using either the ratio or parallel method with point M as the center and a scale factor of \(\frac{3}{2}\).

Answer:

b. Use the dilation theorem to predict the length of \(\overline{L^{\prime} N^{\prime}}\), and then measure its length directly using a ruler.
Answer:
Lengths of the line segments can vary due to copy production. By the dilation theorem, it should be predicted that L’N’ = \(\frac{3}{2}\) LN. This is confirmed using direct measurement.

c. Does the dilation theorem appear to hold true?
Answer:
The dilation theorem does hold true.

Exercise 3.
Produce a scale drawing of ∆ XYZ with point X as the center and a scale factor of \(\frac{1}{4}\). Use the dilation theorem to predict Y’Z’, and then measure its length directly using a ruler. Does the dilation theorem appear to hold true?

Answer:

Lengths of the line segments can vary due to copy production. By the dilation theorem, it should be predicted that Y’Z’ = \(\frac{1}{4}\)YZ. This is confirmed using direct measurement.

Exercise 4.
Given the diagram below, determine if ∆ DEF is a scale drawing of ∆ DGH. Explain why or why not.

Answer:
No. If ∆ DEF was a scale drawing of ∆ DGH, then \(\frac{D E}{D G}=\frac{E F}{G H}\) by the dilation theorem.
\(\frac{D E}{D G}=\frac{3.2}{6.95}\) ≈ 0.46
\(\frac{E F}{G H}=\frac{5.9}{11.9}\) ≈ 0.50
The ratios of the corresponding sides are not equivalent, so the drawing is not a scale drawing.

Eureka Math Geometry Module 2 Lesson 5 Problem Set Answer Key

Question 1.
∆ AB’C’ is a dilation of ∆ ABC from vertex A, and CC’ = 2. Use the given information in each part and the diagram to find B’C’.

a. AB = 9, AC = 4, and BC = 7
Answer:
B’C’ = 10\(\frac{1}{2}\)

b. AB = 4, AC = 9, and BC = 7
Answer:
B’C’ = 8\(\frac{5}{9}\)

c. AB = 7, AC = 9, and BC = 4
Answer:
B’C’ = 4\(\frac{8}{9}\)

d. AB = 7, AC = 4, and BC = 9
Answer:
B’C’ = 18

e. AB = 4, AC = 7, and BC = 9
Answer:
B’C’ = 11\(\frac{4}{7}\)

f. AB = 9, AC = 7, and BC = 4
Answer:
B’C’ = 5\(\frac{1}{7}\)

Question 2.
Given the diagram, ∠CAB ∠CFE. Find AB.

Answer:
\(\overline{F E}\) || \(\overline{A B}\) because when two lines are cut by a transversal, such that the corresponding angles are congruent, then the lines are parallel.

∆ CFE is a scale drawing of ∆ CAB by the parallel method.

F is the image of A after a dilation from C with a scale factor of \(\frac{8}{4}\).
FE = \(\frac{8}{4}\)(AB) by the dilation theorem.
12 = \(\frac{8}{4}\)(AB)
\(\frac{5}{8}\) = \(\frac{5}{8}\left(\frac{8}{5}\right)\) (AB)
\(\frac{5}{12}\) = 1AB
7.5 = AB

Question 3.
Use the diagram to answer each part below.

a. ∆ OP’Q’ is the dilated image of ∆ OPQ from point 0 with a scale factor of r > 1. Draw a possible \(\overline{P Q}\)?.
Answer:
Placement of the segment will vary; however, by the dilation theorem, \(\overline{P Q}\) must be drawn parallel to \(\overline{P^{\prime} Q^{\prime}}\), and because scale factor r > 1, point P must be between O and P’, and point Q must be between O and Q’.

b. ∆ OP”Q” is the dilated image of ∆ OPQ from point O with a scale factor k > r. Draw a possible \(\overline{\boldsymbol{P}^{\prime \prime} Q^{\prime \prime}}\).
Answer:
Placement of the segment will vary; however, by the dilation theorem, \(\overline{\boldsymbol{P}^{\prime \prime} Q^{\prime \prime}}\) must be drawn parallel to and because scale factor k > r, point P” must be placed such that P’ is between P and P” and Q” placed such that Q’is between Q and Q”.

Possible solutions:

Question 4.
Given the diagram to the right, \(\overline{R S}\) || \(\overline{P Q}\), Area (∆ RST) = 15 units², and Area(∆ OSR) = 21 units², find RS.

Answer:
∆ RST and ∆ OSR have the same altitude, so the lengths of their bases are proportional to their areas.
Area(∆ RST) = \(\frac{1}{2}\)(RT)6 = 15
RT = 5
Since the triangles have the same height 6, the ratio of Area(∆ RST): Area(∆ OSR) is equal to the ratio RT:RO.
\(\frac{15}{21}=\frac{5}{O R}\)
OR = 7
OR = OP + PR
7 = OP + 3
OP = 4
If \(\overline{R S}\) || \(\overline{P Q}\), then ∆ OSR is a scale drawing of ∆ OQP from O with a scale factor of \(\frac{7}{4}\). Therefore, R is the image of P, and S is the image of Q under a dilation from point O. By the dilation theorem:
RS = \(\frac{7}{4}\)(5)
RS = \(\frac{35}{4}\)
RS = 8\(\frac{3}{4}\)
The length of segment RS is 8\(\frac{3}{4}\) units.

Question 5.
Using the information given in the diagram and UX = 9, find Z on \(\overline{X U}\) such that \(\overline{Y Z}\) is an altitude. Then, find YZ and XZ.

Answer:
Altitude \(\overline{Y Z}\) is perpendicular to \(\overline{U X}\); thus, m∠YZU = 90°. Since m∠WVU = 90°, then it follows that \(\overline{Y Z}\) || \(\overline{W V}\) by corresponding ∠‘s converse. So, ∆ UYZ is a scale drawing of ∆ UWV by the parallel method. Therefore, Z is the image of V, and Y is the image of W under a dilation from U with a scale factor of \(\frac{13}{5}\).
By the dilation theorem:
YZ = \(\frac{13}{5}\)WV
YZ = \(\frac{13}{5}\)(4)
YZ = \(\frac{52}{5}\).
YZ = 10\(\frac{2}{5}\)
By the Pythagorean theorem:
UV² + VW² = UW²
UV² + (4²) = (5²)
UV² + 16 = 25
UV² = 9
UV = 3
Since Z is the image of V under the same dilation:
UZ = \(\frac{13}{5}\)UV
UZ = \(\frac{13}{5}\)(3)
UZ = \(\frac{39}{5}\)
UZ = 7\(\frac{4}{5}\)
By addition:
XZ + UZ = UX
XZ + 7\(\frac{4}{5}\) = 9
XZ = 1\(\frac{1}{5}\)
The length of \(\overline{Y Z}\) is 10\(\frac{2}{5}\) units, and the length of \(\overline{X Z}\) is 1\(\frac{1}{5}\) units.

Eureka Math Geometry Module 2 Lesson 5 Exit Ticket Answer Key

Question 1.
Two different points R and Y are dilated from S with a scale factor of \(\frac{3}{4}\), and RY = 15. Use the dilation theorem to describe two facts that are known about \(\overline{R^{\prime} Y^{\prime}}\).
Answer:
By the dilation theorem, R’Y’ = \(\frac{3}{4}\)RY, so R’Y’ = \(\frac{3}{4}\)(15) = 11.25, and .

Question 2.
Which diagram(s) below could represent your conclusions in Problem 1? Explain your answer(s).
a.

Answer:
Diagram (a) can be a dilation with scale factor \(\frac{3}{4}\) since R’S and V’S appear to be \(\frac{3}{4}\) of the distances RS and VS, respectively. However, because both line segments lie on the same line, \(\overline{R^{\prime} Y^{\prime}}\) = \(\overline{R Y}\).

b.

Answer:
Diagram (b) could be a dilation with scale factor \(\frac{3}{4}\) since R‘S and V’S appear to be \(\frac{3}{4}\) of the distances RS and YS, respectively. Because S, Y, and R are vertices of a triangle, \(\overline{R^{\prime} Y^{\prime}}\) = \(\overline{R Y}\).