Eureka Math Algebra 2 Module 1 Lesson 38 Answer Key

Engage NY Eureka Math Algebra 2 Module 1 Lesson 38 Answer Key

Eureka Math Algebra 2 Module 1 Lesson 38 Example Answer Key

Example 1.
Consider the equation 3x + x² = – 7.
What does the value of the discriminant tell us about number of solutIons to this equation?
Solve the equation. Does the number of solutions match the Information provided by the discriminant? Explain.
Answer:
Consider the equation 3x + x² = – 7.
→ What does the value of the discriminant tell us about number of solutions to this equation?
The equation in standard form is x² + 3x + 7 = 0, so we have a = 1, b = 3, c = 7.
The discriminant is 3² – 4(1)(7) = – 19. The negative discriminant indicates that no real solutions exist. There are two complex solutions.

→ Solve the equation. Does the number of solutions match the information provided by the discriminant? Explain.
Using the quadratic formula,
x = \(\frac{-3+\sqrt{-19}}{2}\) or x = \(\frac{-3+\sqrt{-19}}{2}\)
The solutions, in a + bi form, are \(-\frac{3}{2}+\frac{\sqrt{19}}{2} i\) and \(-\frac{3}{2}-\frac{\sqrt{19}}{2} i\).
The two complex solutions are consistent with the rule for a negative discriminant.

Eureka Math Algebra 2 Module 1 Lesson 38 Opening Exercise Answer Key

Exercise 1.
The expression under the radical in the quadratic formula, b² – 4ac, is called the discriminant.
Use the quadratic formula to solve the following quadratic equations. Calculate the discriminant for each equation.
a. x² – 9 = 0
Answer:
The equation x² – 9 = 0 has two real solutions: x = 3 and x = – 3. The discriminant of x² – 9 = 0 is 36.

b. x² – 6x + 9 = 0
Answer:
The equation x² – 6x + 9 = 0 has one real solution: x = 3. The discriminant of x² – 6x + 9 = 0 is 0.

c. x² + 9 = 0
Answer:
The equation x² + 9 = 0 has two complex solutions: x = 3i and x = – 3i. The discriminant of x² + 9 = 0 is – 36.

Exercise 2.
How does the value of the discriminant for each equation relate the number of solutions you found?
Answer:
If the discriminant is negative, the equation has complex solutions. If the discriminant is zero, the equation has one real solution. If the discriminant is positive. the equation has two real solutions.

Eureka Math Algebra 2 Module 1 Lesson 38 Exercise Answer Key

Exercise
Compute the value of the discriminant of the quadratic equation In each part. Use the value of the discriminant to predict the number and type of solutions. Find all real and complex solutions.

a. x² + 2x + 1 = 0
Answer:
We have a = 1, b = 2, and c = 1. Then
b² – 4ac = 2² – 4(1)(1) = 0.
Note that the discriminant Is zero, so this equation has exactly one real solution.
x = \(\frac{-(2) \pm \sqrt{0}}{2(1)}\) = – 1
Thus, the only solution is – 1.

b. x² + 4 = 0
Answer:
We have a = 1, b = 0, and c = 4. Then
b² – 4ac = – 16.
Note that the discriminant is negative, so this equation has two complex solutions.
x = \(\frac{-0 \pm \sqrt{-16}}{2(1)}\)
Thus, the two complex solutions are 2i and – 2i.

c. 9x² – 4x – 14 = 0
Answer:
We have a = 9, b = – 4, and c = – 14. Then
b² – 4ac = (-4)² – 4(9)(- 14)
= 16 + 504
= 520.
Note that the discriminant is positive, so this equation has two distinct real solutions.
Using the quadratic formula,
x = \(\frac{-(-4) \pm 2 \sqrt{130}}{2(9)}\)
So, the two real solutions are \(\frac{2+\sqrt{130}}{9}\) and \(\frac{2-\sqrt{130}}{9}\).

d. 3x² + 4x + 2 = 0
Answer:
We have a = 3, b = 4, and c = 2. Then
b² – 4ac = 4² – 4(3)(2)
= 16 – 24
= – 8.
The discriminant is negative, so there will be two complex solutions. Using the quadratic formula,
x = \(\frac{-4 \pm \sqrt{-8}}{2(3)}\)
So, the two complex solutions are \(-\frac{2}{3}+\frac{\sqrt{2}}{3} i\) and \(-\frac{2}{3}-\frac{\sqrt{2}}{3} i\).

e. x = 2x² + 5
Answer:
We can rewrite this equation in standard form with a = 2, b = – 1, and c = 5:
2x² – x + 5 = 0.
Then
b² – 4ac = (-1)² – 4(2)(5)
= 1 – 40
= – 39.
The discriminant is negative, so there will be two complex solutions. Using the quadratic formula,
x = \(\frac{-(-1) \pm \sqrt{-39}}{2(2)}\)
x = \(\frac{1 \pm i \sqrt{39}}{4}\)
The two solutions are \(\frac{1}{4}+\frac{\sqrt{39}}{4} i\) and \(\frac{1}{4}-\frac{\sqrt{39}}{4} i\).

f. 8x² + 4x + 32 = 0
Answer:
We can factor 4 from the left side of this equation to obtain 4(2x² + x +8) = 0, and we know that a product is zero when one of the factors are zero. Since 4 ≠ 0, we must have 2x² + x + 8 = 0. This is a quadratic equation with a = 2, b = 1, and
c = 8. Then
b² – 4ac = 1² – 4(2)(8) = – 63.
The discriminant is negative, so there will be two complex solutions. Using the quadratic formula,
x = \(\frac{-1 \pm \sqrt{-63}}{2(2)}\)
x = \(\frac{-1 \pm 3 i \sqrt{7}}{4}\)
The complex solutions are \(-\frac{1}{4}+\frac{3 \sqrt{7}}{4} i\) and \(-\frac{1}{4}-\frac{3 \sqrt{7}}{4} i\).

Eureka Math Algebra 2 Module 1 Lesson 38 Problem Set Answer Key

Question 1.
Give an example of a quadratic equation in standard form that has …
a. Exactly two distinct real solutions.
Answer:
Since (x + 1)(x – 1) = – 1, the equation x² – 1 = 0 has two distinct real solutions, 1 and – 1.

b. Exactly one distinct real solution.
Answer:
Since (x + 1)² = x² + 2x + 1, the equation x² + 2x + 1 = 0 has only one real solution, 1.

c. Exactly two complex (non-real) solutions.
Answer:
Since x² + 1 = 0 has no solutions in the real numbers, this equation must have two complex solutions. They are i and -i.

Question 2.
Suppose we have a quadratic equation ax² + bx + c = 0 so that a + c = 0. Does the quadratic equation have one solution or two distinct solutions? Are they real or complex? Explain how you know.
Answer:
If a + c = 0, the neither a = c = 0, a > 0 and c < 0, or a < 0 and c > 0.
The definition of a quadratic polynomial requires that a ≠ 0, so either a > 0 and c < 0 or a < 0 and c > 0.
In either case, 4ac < 0. Because b² is positive and 4ac is negative, we know b² – 4ac > 0.
Therefore, a quadratic equation ax² + bx + c = 0 always has two distinct real solutions when a + c = 0.

Question 3.
Solve the equation 5x² – 4x + 3 = 0.
Answer:
We have a quadratic equation with a = 5, b = – 4, and c = 3.
x = \(\frac{-(-4) \pm 2 \sqrt{-11}}{2(5)}\)
So, the solutions are \(\) and \(\frac{2}{5}-\frac{i \sqrt{11}}{5}\) .

Question 4.
Solve the equation 2x² + 8x = – 9.
Answer:
In standard form, this is the quadratic equation 2x² + 8x + 9 = 0 with a = 2, b = 8, and c = 9.
x = \(\frac{-8 \pm 2 \sqrt{-2}}{2(2)}\) = \(\frac{-4 \pm i \sqrt{2}}{2}\)
Thus, the solutions are 2 + \(\frac{i \sqrt{2}}{2}\) and 2 – \(\frac{i \sqrt{2}}{2}\)

Question 5.
Solve the equation 9x – 9x² = 3 + x + x².
Answer:
In standard form, this is the quadratic equation 10x² – 8x + 3 = 0 with a = 10, b = – 8, and c = 3.
x = \(\frac{-(-8) \pm 2 \sqrt{-14}}{2(10)}\) = \(\frac{8 \pm 2 i \sqrt{14}}{20}\)
Thus, the solutions are \(\frac{2}{5}+\frac{i \sqrt{14}}{10}\) and \(\frac{2}{5}-\frac{i \sqrt{14}}{10}\).

Question 6.
Solve the equation 3x² – x + 1 = 0.
Answer:
This is a quadratic equation with a = 3, b = – 1, and c = 1.
x = \(\frac{-(-1) \pm \sqrt{-11}}{2(3)}\) = \(\frac{1 \pm i \sqrt{11}}{6}\)
Thus, the solutions are \(\frac{1}{6}+\frac{i \sqrt{11}}{6}\) and \(\frac{1}{6}-\frac{i \sqrt{11}}{6}\)

Question 7.
Solve the equation 6x⁴ + 4x² – 3x + 2 = 2x²(3x² – 1).
Answer:
When expanded, this is a quadratic equation with a = 6, b = – 3, and c = 2.
6x⁴ + 4x² – 3x + 2 = 6x⁴ – 2x²
6x² – 3x + 2 = 0
x = \(\frac{-(-3) \pm \sqrt{\{-39\}}}{2(6)}\)
So, the solutions are \(\frac{1}{4}+\frac{i \sqrt{39}}{12}\) and \(\frac{1}{4}-\frac{i \sqrt{39}}{12}\)

Question 8.
Solve the equation 25x² + 100x + 200 = 0.
Answer:
We can factor 25 from the left side of this equation to obtain 25 (x² + 4x + 8) = 0, and we know that a product is zero when one of the factors is zero. Since 25 ≠ 0, we must have x² + 4x + 8 = 0. This is a quadratic equation with a = 1, b = 4, and c = 8. Then
x = \(\frac{-4 \pm 4 \sqrt{-1}}{2}\)
and the solutions are – 2 + 2i and – 2 – 2i.

Question 9.
Write a quadratic equation in standard form such that – 5 is its only solution.
Answer:
(x + 5)² = 0
x² + 10x + 25 = 0

Question 10.
Is it possible that the quadratic equation ax² + bx + c = 0 has a positive real solution if a, b, and c are all positive real numbers?
Answer:
No. The solutions are \(\frac{-b+\sqrt{b^{2}-4 a c}}{2 a}\) and \(\frac{-b-\sqrt{b^{2}-4 a c}}{2 a}\). If b is positive, the second one of these will be negative. So, we need to think about whether or not the first one can be positive. If – b + \(\sqrt{b^{2}-4 a c}\) > 0, then \(\sqrt{b^{2}-4 a c}\) > b; so, b² – 4ac > b², and – 4ac > 0. This means that either a or c must be negative. So, if all three coefficients are positive, then there cannot be a positive solution to ax² + bx + c = 0.

Question 11.
Is it possible that the quadratic equation ax² + bx + c = 0 has a positive real solution if a, b, and c are all negative real numbers?
Answer:
No. If a, b, and c are all negative, then – a, – b, and – c are all positive. The solutions of ax² + bx + c = 0 are the same as the solutions to – ax² – bx – c = 0, and by Problem 10, this equation has no positive real solution since it has all positive coefficients.

Extension:

Question 12.
Show that if k > 3.2, the solutions of 5x² – 8x + k = 0 are not real numbers.
Answer:
We have a = 5, b = – 8, and c = k; then
b² – 4ac = (-8)² – 45k
= 64 – 20k.
When the discriminant is negative, the solutions of the quadratic function are not real numbers.
b² – 4ac = 64 – 20k
k < 3.2
b² -4ac < 64 – 20(3.2)
b² – 4ac < 0 k >3.2
Thus, if k > 3.2, then the discriminant is negative and the solutions of 5x² – 8x + k = 0 are not real numbers.

Question 13.
Let k be a real number, and consider the quadratic equation (k + 1)x² + 4kx + 2 = 0.
a. Show that the discriminant of (k + 1)x² + 4kx + 2 = 0 defines a quadratic function of k.
Answer:
The discriminant of a quadratic equation written in the form ax² + bx + c = 0 is b² – 4ac.
Here, a = k + 1, b = 4k, and c = 2. We get
b²– 4ac = (4k)² – 4 ∙ (k + 1) ∙ 2
= 16k² – 8(k+ 1)
= 16k² – 8k – 8.
With k unknown, we can write f(k) = 16k² – 8k – 8, which is a quadratic function of k.

b. Find the zeros of the function in part (a), and make a sketch of its graph.
Answer:
If f(k) = 0, then we have
0 = 16k² – 8k – 8
= 2k² – k – 1
= 2k² – 2k + k – 1
= 2k(k – 1) + 1 (k – 1)
= (k – 1) (2k + 1).
Then, k – 1 = 0 or 2k+ 1 = 0.
So, k = 1 or k = –\(\frac{1}{2}\).

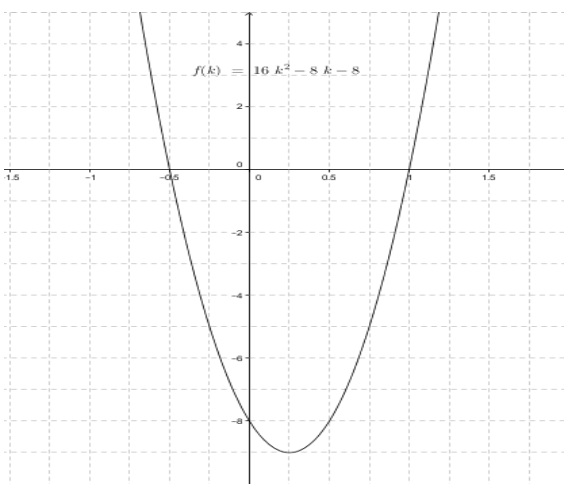
c. For what value of k are there two distinct real solutions to the original quadratic equation?
Answer:
The original quadratic equation has two distinct real solutions when the discriminant given by f(k) is positive. This occurs for all real numbers k such that k < –\(\frac{1}{2}\) or k > 1.

d. For what value of k are there two complex solutions to the given quadratic equation?
Answer:
There are two complex solutions when f(k) < 0. This occurs for all real numbers k such that – \(\frac{1}{2}\) < k < 1.

e. For what value of k is there one solution to the given quadratic equation?
Answer:
There is one solution when f(k) = 0. This occurs at k = –\(\frac{1}{2}\) and k = 1.

Question 14.
We can develop two formulas that can help us find errors in calculated solutions of quadratic equations.
a. Find a formula for the sum S of the solutions of the quadratic equation ax² + bx + c = 0.
Answer:

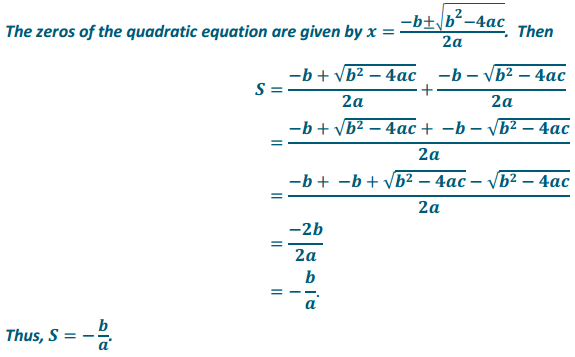
b. Find a formula for the product R of the solutions of the quadratic equation ax² + bx + c = 0.
Answer:

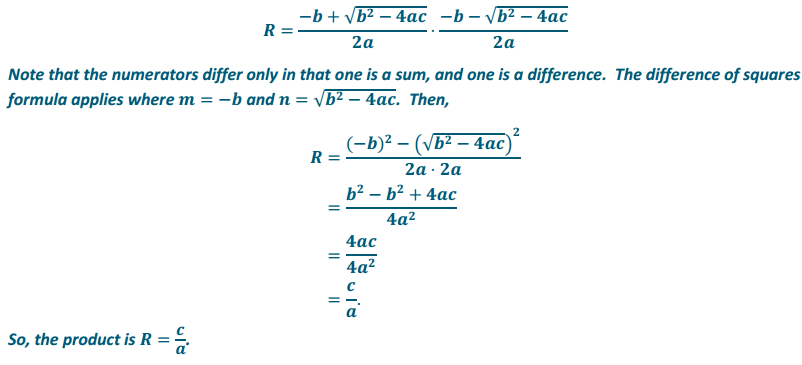
c. June calculated the solutions 7 and – 1 to the quadratic equation x² – 6x + 7 = 0. Do the formulas from parts (a) and (b) detect an error in her solutions? If not, determine if her solution is correct.
Answer:
The sum formula agrees with June’s calculations. From June’s zeros,
7 + – 1 = 6,
and from the formula,
S = \(\frac{6}{1}\) = 6.
However, the product formula does not agree with her calculations. From June’s zeros,
7 ∙ – 1 – 7,
and from the formula,
R = \(\frac{7}{1}\) = 7.
June’s solutions are not correct: (7)² – 6(7) + 7 = 49 – 42 + 7 = 14; so, 7 is not a solution to this quadratic equation. Likewise, 1 – 6 + 7 = 2, so 1 is also not a solution to this equation. Thus, the formulas caught her error.

d. Paul calculated the solutions 3 – i√2 and 3 + i√2 to the quadratic equation x² – 6x + 7 = 0. Do the formulas from parts (a) and (b) detect an error in his solutions? If not, determine if his solutions are correct.
Answer:
In part (c), we calculated that R = 7 and S = 6. From Paul’s zeros,
S = 3 + i√2 + 3 – i√2 = 6,
and for the product,
R = (3 + i√2) ∙ (3 – i√2)
= 3² – (i√2)²
= 9 – 1 ∙ 2
= 11.
This disagrees with the calculated version of R. So, the formulas do find that he made an error.

e. Joy calculated the solutions 3 – √2 and 3 + √2 to the quadratic equation x² – 6x + 7 = 0. Do the formulas from parts (a) and (b) detect an error in her solutions? If not, determine if her solutions are correct.
Answer:
Joy’s zeros will have the same sum as Paul’s, so S = 6, which agrees with the sum from the formula. For the product of her zeros we get
R = (3 – √2) (3 + √2)
= 9 – 2
= 7,
which agrees with the formulas.
Checking her solutions in the original equation, we find
(3 – √2)² – 6(3 – √2) + 7 = (9 – 6√2 + 2) – 18 + 6√2 + 7 = 0,
(3 + √2)² – 6(3 – √2) + 7 = (9 + 6√2 + 2) – 18 + 6√2 + 7 = 0.
Thus, Joy has correctly found the solutions of this quadratic equation.

f. If you find solutions to a quadratic equation that match the results from parts (a) and (b), does that mean your solutions are correct?
Answer:
Not necessarily. We only know that if the sum and product of the solutions do not match S and R, then we have not found a solution. Evidence suggests that if the sum and product of the solutions do match S and R, then we have found the correct solutions, but we do not know for sure until we check.

g. Summarize the results of this exercise.
Answer:
For a quadratic equation of the form ax² + bx + c = 0, the sum of the solutions is given by S = –\(\frac{b}{a}\) and the product of the solutions is given by R = \(\frac{c}{a}\) . So, multiplying and adding the calculated solutions will identify if we have made an error. Passing these checks, however, does not guarantee that the numbers we found are the correct solutions.

Eureka Math Algebra 2 Module 1 Lesson 38 Exit Ticket Answer Key

Use the discriminant to predict the nature of the solutions to the equation 4x – 3x² = 10. Then, solve the equation.
Answer:
3x² – 4x + 10 = 0
We have a = 3, b = -4, and c= 10. Then
b² – 4ac = (-4)² – 4(3)(10)
= 16 – 120
= – 104.
The value of the discriminant is negative, indicating that there are two complex solutions.
x = \(\frac{-(-4) \pm \sqrt{-104}}{2(3)}\)
x = \(\frac{4 \pm 2 i \sqrt{26}}{6}\)
Thus, the two solutions are \(\frac{2}{3}+\frac{\sqrt{26}}{3} i\) and \(\frac{2}{3}-\frac{\sqrt{26}}{3} i\).