Eureka Math Precalculus Module 1 Lesson 6 Answer Key

Engage NY Eureka Math Precalculus Module 1 Lesson 6 Answer Key

Eureka Math Precalculus Module 1 Lesson 6 Exercise Answer Key

Opening Exercise
Perform the indicated arithmetic operations for complex numbers z = -4 + 5i and w = -1-2i.

a. z + w
Answer:
z + w = -5 + 3i

b. z-w
Answer:
z-w = -3 + 7i

c.z + 2w
Answer:
z + 2w = -6 + i

d.z-z
Answer:
z-z = 0 + 0i

e. Explain how you add and subtract complex numbers.
Answer:
Add or subtract the real components and the imaginary components separately.

Exercises

Exercise 1.
The length of the vector that represents z₁ = 6-8i is 10 because \(\sqrt{6^{2} + (-8)^{2}}\) = \(\sqrt{100}\) = 10.
a. Find at least seven other complex numbers that can be represented as vectors that have length 10.
Answer:
There are an infinite number of complex numbers that meet this criteria; the most obvious are 10, 6 + 8i, 8 + 6i, 10i, -6 + 8i, -8 + 6i, -10, -8-6i, -6-8i, -10i, 8-6i, and -8 + 6i. The associated vectors for these numbers are shown in the sample response for part (b).

b. Draw the vectors on the coordinate axes provided below.
Answer:

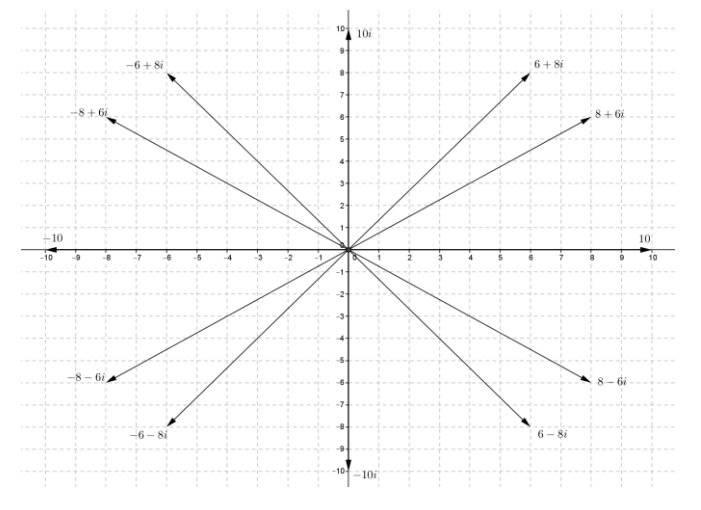
c. What do you observe about all of these vectors?
Answer:
Students should observe that the tips of the vectors lie on the circle of radius 10 centered at the origin.

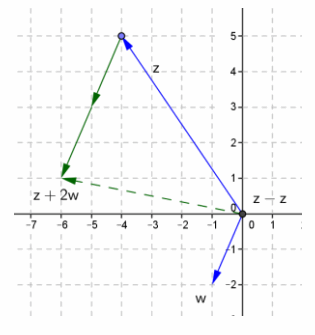
Exercise 2.
In the Opening Exercise, we computed z + 2w. Calculate this sum using vectors.
Answer:

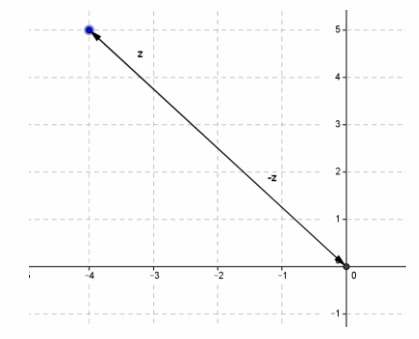
Exercise 3.
In the Opening Exercise, we also computed z-z. Calculate this sum using vectors.
Answer:

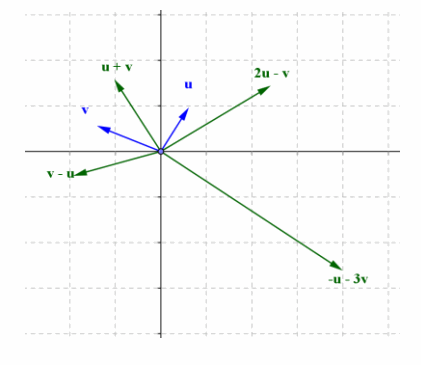
Exercise 4.
For the vectors u and v pictured below, draw the specified sum or difference on the coordinate axes provided.
a. u + v
b. v-u
c. 2u-v
d. -u-3v
Answer:

Exercise 5.
Find the sum of 4 + i and -3 + 2i geometrically.
Answer:
1 + 3i

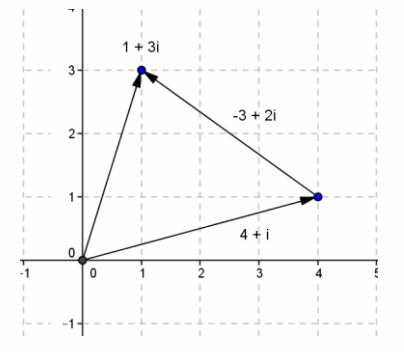
Exercise 6.
Show that (7 + 2i)-(4-i) = 3 + 3i by representing the complex numbers as vectors.
Answer:

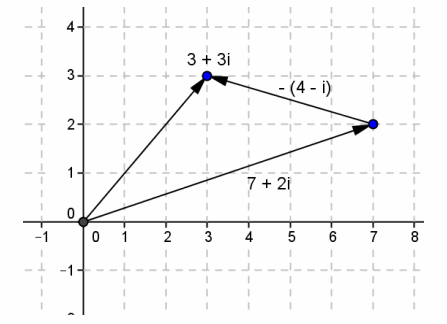
Eureka Math Precalculus Module 1 Lesson 6 Problem Set Answer Key

Question 1.
Let z = 1 + i and w = 1-3i. Find the following. Express your answers in a + bi form.
a. z + w
Answer:
1 + i + 1-3i = 2-2i

b. z-w
Answer:
1 + i-(1-3i) = 1 + i-1 + 3i
= 0 + 4i

c. 4w
Answer:
4(1-3i) = 4-12i

d. 3z + w
Answer:
3(1 + i) + 1-3i = 3 + 3i + 1-3i
= 4 + 0i

e. -w-2z
Answer:
-(1-3i)-2(1 + i) = -1 + 3i-2-2i
= -3 + i

f. What is the length of the vector representing z?
Answer:
The length of the vector representing z is \(\sqrt{1^{2} + 1^{2}}\) = \(\sqrt{2}\).

g. What is the length of the vector representing w?
Answer:
The length of the vector representing w is \(\sqrt{1^{2} + (-3)^{2}}\) = \(\sqrt{10}\).

Question 2.
Let u = 3 + 2i, v = 1 + i, and w = -2-i. Find the following. Express your answer in a + bi form, and represent the result in the plane.

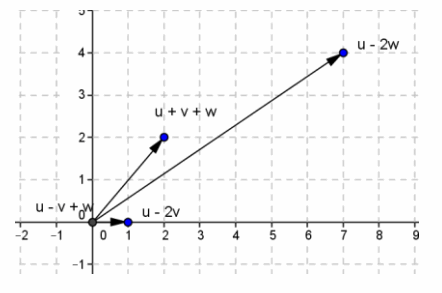
a. u-2v
Answer:
3 + 2i-2(1 + i) = 3 + 2i-2-2i
= 1 + 0i

b. u-2w
Answer:
3 + 2i-2(-2-i) = 3 + 2i + 4 + 2i
= 7 + 4i

c. u + v + w
Answer:
3 + 2i + 1 + i-2-i = 2 + 2i

d. u-v + w
Answer:
3 + 2i-(1 + i)-2-i = 3 + 2i-1-i-2-i
= 0 + 0i

e. What is the length of the vector representing u?
Answer:
The length of the vector representing u is \(\sqrt{3^{2} + 2^{2}}\) = \(\sqrt{13}\).

f. What is the length of the vector representing u-v + w?
Answer:
The length of the vector representing u-v + w = \(\sqrt{0^{2} + 0^{2}}\) = \(\sqrt{0}\) = 0.

Question 3.
Find the sum of -2-4i and 5 + 3i geometrically.
Answer:
3-i

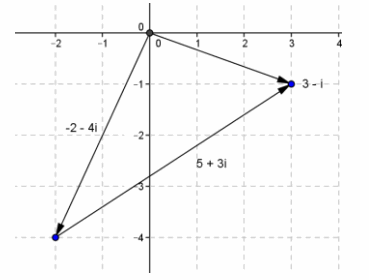
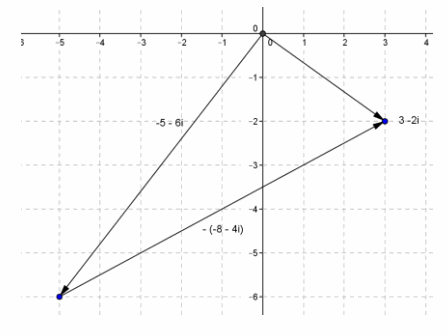
Question 4.
Show that (-5-6i)-(-8-4i) = 3-2i by representing the complex numbers as vectors.
Answer:

Question 5.
Let z₁ = a₁ + b₁ i, z₂ = a₂ + b₂ i, and z₃ = a₃ + b₃ i. Prove the following using algebra or by showing with vectors.
Answer:
z₁ + z₂ = z₂ + z₁
z₁ + z₂ = (a₁ + b₁ i) + (a₂ + b₂ i)
= (a₂ + b₂ i) + (a₁ + b₁ i)
= z₂ + z₁

b. z₁ + (z₂ + z₃ ) = (z₁ + z₂ ) + z₃
Answer:
z₁ + (z₂ + z₃ ) = (a₁ + b₁ i) + ((a₂ + b₂ i) + (a₃ + b₃ i))
= ((a₁ + b₁ i) + (a₂ + b₂ i)) + (a₃ + b₃ i)
= (z₁ + z₂ ) + z₃

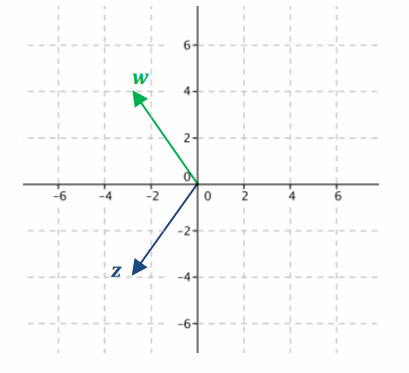
Question 6.
Let z = -3-4i and w = -3 + 4i.
a. Draw vectors representing z and w on the same set of axes.
Answer:

b. What are the lengths of the vectors representing z and w?
Answer:
The length of the vector representing z is \(\sqrt{(-3)^{2} + (-4)^{2}}\) = \(\sqrt{25}\) = 5.
The length of the vector representing w is \(\sqrt{(-3)^{2} + 4^{2}}\) = \(\sqrt{25}\) = 5.

c. Find a new vector, u_z, such that u_z is equal to z divided by the length of the vector representing z.
Answer:
u_z = \(\frac{-3-4 i}{5}\) = \(\frac{-3}{5}\) – \(\frac{4}{5}\)i

d. Find u_w, such that u_w is equal to w divided by the length of the vector representing w.
Answer:
u_w = \(\frac{-3 + 4 i}{5}\) = \(\frac{-3}{5}\) + \(\frac{4}{5}\)i

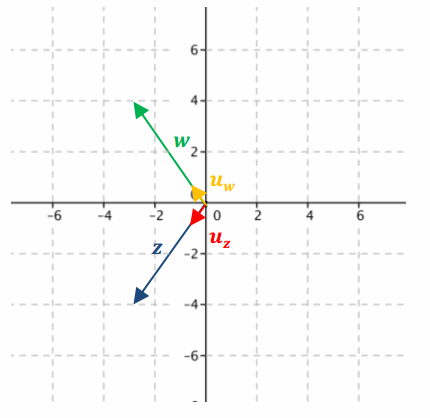
e. Draw vectors representing u_z and u_w on the same set of axes as part (a).
Answer:

f. What are the lengths of the vectors representing u_z and u_w?
Answer:
The length of the vector representing u_z is \(\sqrt{\left(-\frac{3}{5}\right)^{2} + \left(-\frac{4}{5}\right)^{2}}\) = \(\sqrt{\frac{9}{25} + \frac{16}{25}}\) = \(\sqrt{\frac{25}{25}}\) = \(\sqrt{1}\) = 1.
The length of the vector representing u_w is \(\sqrt{\left(-\frac{3}{5}\right)^{2} + \left(\frac{4}{5}\right)^{2}}\) = \(\sqrt{\frac{9}{25} + \frac{16}{25}}\) = \(\sqrt{\frac{25}{25}}\) = \(\sqrt{1}\) = 1.

g. Compare the vectors representing u_z to z and u_w to w. What do you notice?
Answer:
The vectors representing u_z and u_w are in the same direction as z and w, respectively, but their lengths are only 1.

h. What is the value of u_z times u_w?
Answer:
(\(\frac{3}{5}\)–\(\frac{4}{5}\)i)(\(\frac{3}{5}\))²-(\(\frac{4}{5}\)i)² = (\(\frac{9}{25}\)) + (\(\frac{16}{25}\)) = 1

i. What does your answer to part (h) tell you about the relationship between u_z and u_w?
Answer:
Since their product is 1, we know that u_z and u_w are reciprocals of each other.

Question 7.
Let z = a + bi.
a. Let u_z be represented by the vector in the direction of z with length 1. How can you find u_z? What is the value of u_z?
Answer:
Find the length of z, and then divide z by its length.
u_z = \(\frac{a + b i}{\sqrt{a^{2} + b^{2}}}\)

b. Let u_w be the complex number that when multiplied by u_z, the product is 1. What is the value of u_w?
Answer:
From Problem 4, we expect u_w = \(\frac{a-b i}{\sqrt{a^{2} + b^{2}}}\). Multiplying, we get
\(\frac{a + b i}{\sqrt{a^{2} + b^{2}}}\) ∙ \(\frac{a-b i}{\sqrt{a^{2} + b^{2}}}\) = \(\frac{a^{2}-(b i)^{2}}{a^{2} + b^{2}}\)
= \(\frac{a^{2} + b^{2}}{a^{2} + b^{2}}\)
= 1

c. What number could we multiply z by to get a product of 1?
Answer:
Since we know that u_z is equal to z divided by the length of z and that u_z ∙ u_w = 1, we get
z ∙ \(\frac{1}{\sqrt{a^{2} + b^{2}}}\)∙\(\frac{a-b i}{\sqrt{a^{2} + b^{2}}}\) = z∙\(\frac{a-b i}{a^{2} + b^{2}}\) = 1
So, multiplying z by \(\frac{a-b i}{a^{2} + b^{2}}\) will result in a product of 1.

Question 8.
Let z = -3 + 5i.
a. Draw a picture representing z + w = 8 + 2i.
Answer:

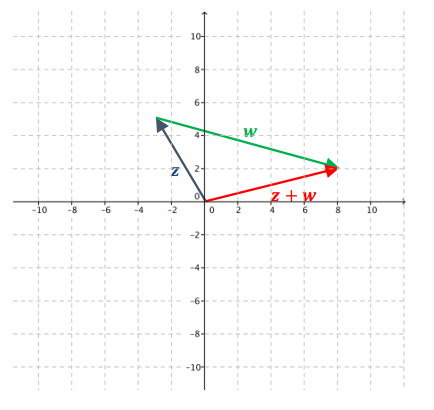
b. What is the value of w?
Answer:
w = 11 – 3i

Eureka Math Precalculus Module 1 Lesson 6 Exit Ticket Answer Key

Answer:

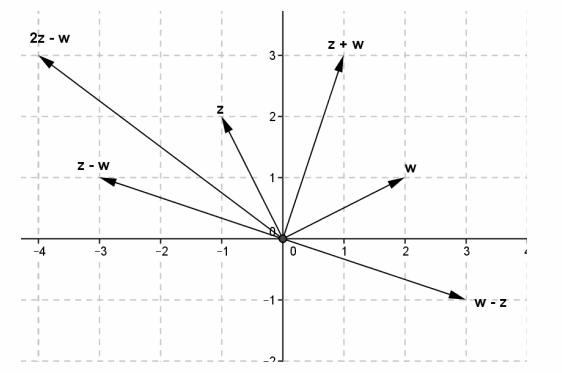
Let z = -1 + 2i and w = 2 + i. Find the following, and verify each geometrically by graphing z, w, and each result.

a. z + w
Answer:
1 + 3i

b. z-w
Answer:
-3 + i

c. 2z-w
Answer:
-4 + 3i

d. w-z
Answer:
3-i