Eureka Math Grade 8 Module 4 Lesson 14 Answer Key

Engage NY Eureka Math 8th Grade Module 4 Lesson 14 Answer Key

Eureka Math Grade 8 Module 4 Lesson 14 Exercise Answer Key

Exercise 1.
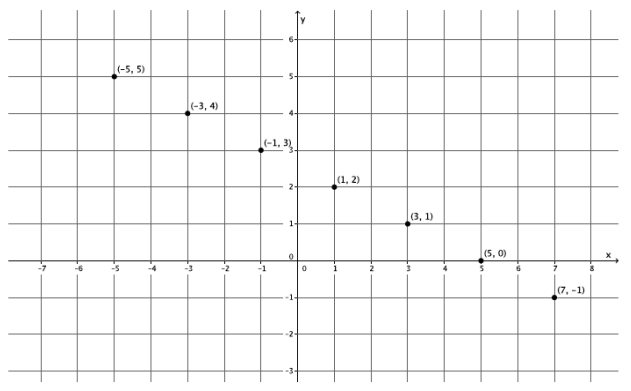
Find at least four solutions to graph the linear equation 1x+2y=5.
Answer:

Exercise 2.
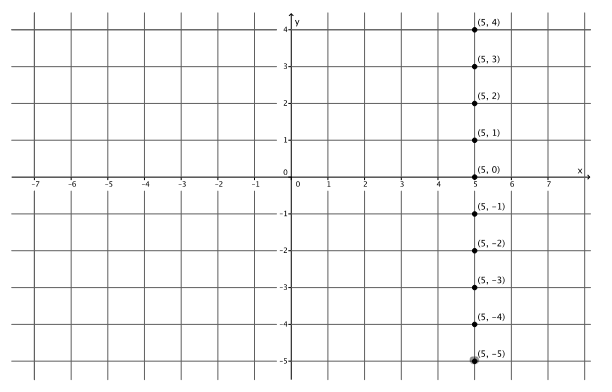
Find at least four solutions to graph the linear equation 1x+0y=5.
Answer:

Exercise 3.
What was different about the equations in Exercises 1 and 2? What effect did this change have on the graph?
Answer:
In the first equation, the coefficient of y was 2. In the second equation, the coefficient of y was 0. The graph changed from being slanted to a vertical line.

Exercises 4–6
Students complete Exercises 4–6 independently. Students need graph paper to complete the exercises.

Exercise 4.
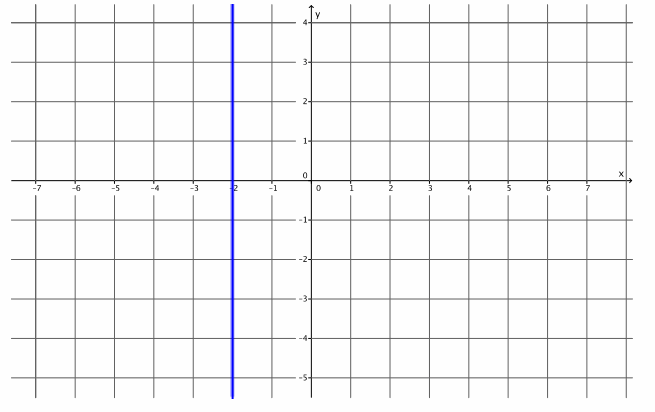
Graph the linear equation x=-2.
Answer:

Exercise 5.
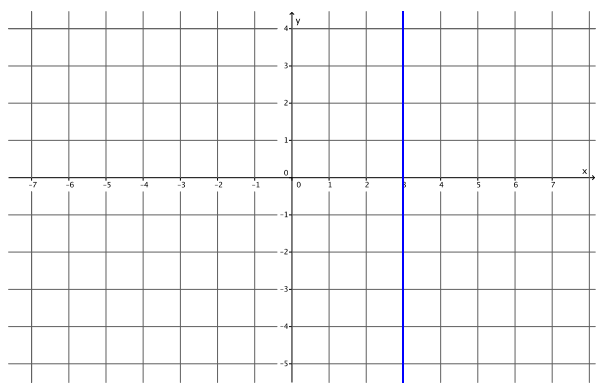
Graph the linear equation x=3.
Answer:

Exercise 6.
What will the graph of x=0 look like?
Answer:
The graph of x=0 will look like a vertical line that goes through the point (0,0). It will be the same as the y-axis.

Exercises 7–9
Students complete Exercises 7–9 independently or in pairs in preparation for the discussion that follows. Students need graph paper to complete the exercises.

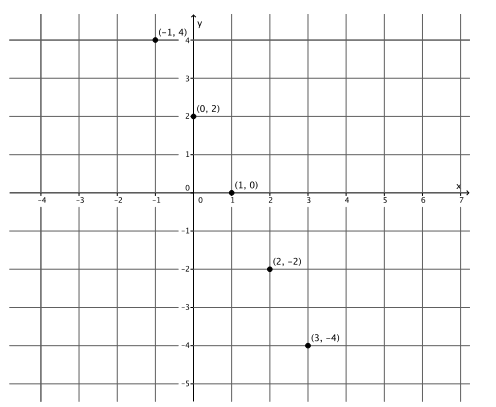
Exercise 7.
Find at least four solutions to graph the linear equation 2x+1y=2.
Answer:

Exercise 8.
Find at least four solutions to graph the linear equation 0x+1y=2.
Answer:

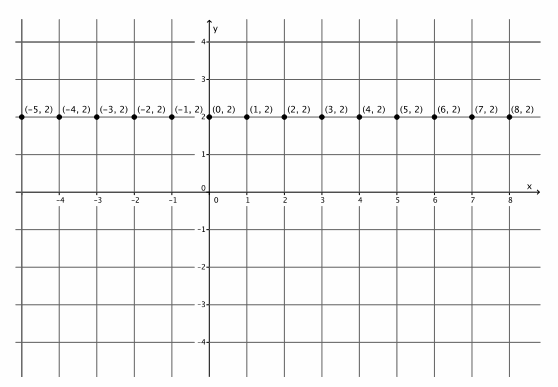
Exercise 9.
What was different about the equations in Exercises 7 and 8? What effect did this change have on the graph?
Answer:
In the first equation, the coefficient of x was 2. In the second equation, the coefficient of x was 0. The graph changed from being a slanted line to a horizontal line.

Exercises 10–12
Students complete Exercises 10–12 independently. Students need graph paper to complete the exercises.

Exercise 10.
Graph the linear equation y=-2.
Answer:

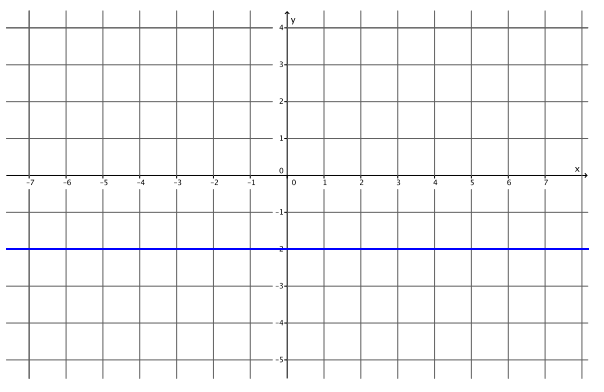
Exercise 11.
Graph the linear equation y=3.
Answer:

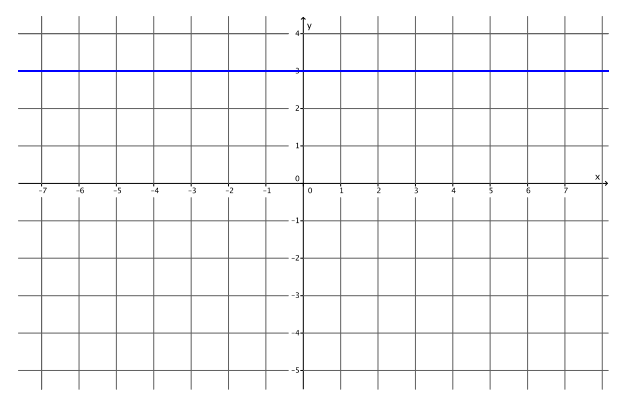
Exercise 12.
What will the graph of y=0 look like?
Answer;
The graph of y=0 will look like a horizontal line that goes through the point (0,0). It will be the same as the x-axis.

Eureka Math Grade 8 Module 4 Lesson 14 Exit Ticket Answer Key

Question 1.
Graph the linear equation ax+by=c, where a=0, b=1, and c=1.5.

Answer:

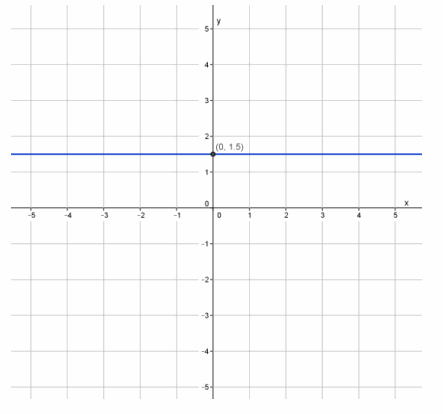
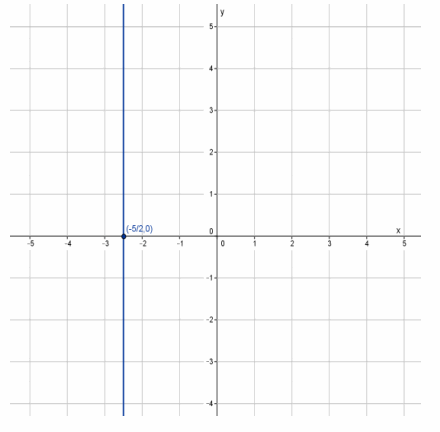
Question 2.
Graph the linear equation ax+by=c, where a=1, b=0, and c=-\(\frac{5}{2}\).

Answer:

Question 3.
What linear equation represents the graph of the line that coincides with the x-axis?
Answer:
y=0

Question 4.
What linear equation represents the graph of the line that coincides with the y-axis?
Answer:
x=0

Eureka Math Grade 8 Module 4 Lesson 14 Problem Set Answer Key

Students need graph paper to complete the Problem Set.

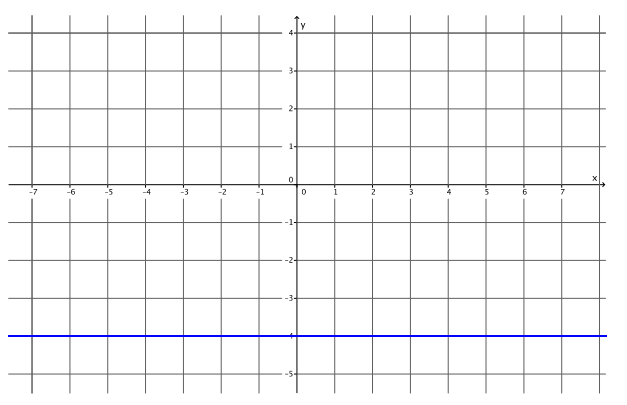
Question 1.
Graph the two-variable linear equation ax+by=c, where a=0, b=1, and c=-4.
Answer:

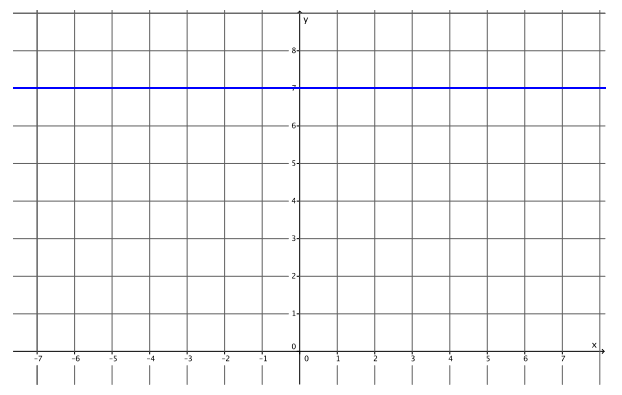
Question 2.
Graph the two-variable linear equation ax+by=c, where a=1, b=0, and c=9.
Answer:

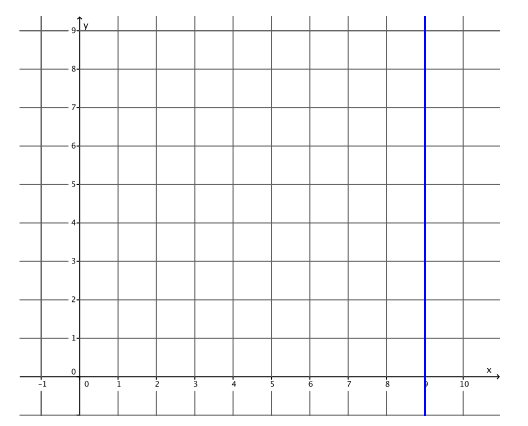
Question 3.
Graph the linear equation y=7.
Answer:

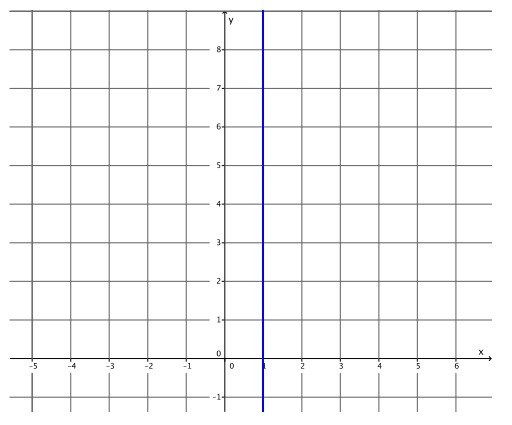
Question 4.
Graph the linear equation x=1.
Answer:

Question 5.
Explain why the graph of a linear equation in the form of y=c is the horizontal line, parallel to the x-axis passing through the point (0,c).
Answer:
The graph of y=c passes through the point (0,c), which means the graph of y=c cannot be parallel to the y-axis because the graph intersects it. For that reason, the graph of y=c must be the horizontal line parallel to the
x-axis.

Question 6.
Explain why there is only one line with the equation y=c that passes through the point (0,c).
Answer:
There can only be one line parallel to another that goes through a given point. Since the graph of y=c is parallel to the x-axis and it goes through the point (0,c), then it must be the only line that does. Therefore, there is only one line that is the graph of the equation y=c that passes through (0,c).