Engage NY Eureka Math 7th Grade Module 5 Mid Module Assessment Answer Key
Eureka Math Grade 7 Module 5 Mid Module Assessment Task Answer Key
Round all decimal answers to the nearest hundredth.
Question 1.
Each student in a class of 38 students was asked to report how many siblings (brothers and sisters) he has. The data are summarized in the table below.
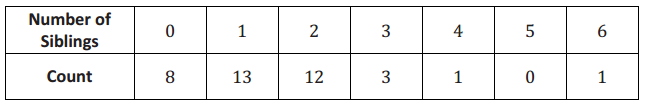
a. Based on the data, estimate the probability that a randomly selected student from this class is an only child.
b. Based on the data, estimate the probability that a randomly selected student from this class has three or more siblings.
c. Consider the following probability distribution for the number of siblings:
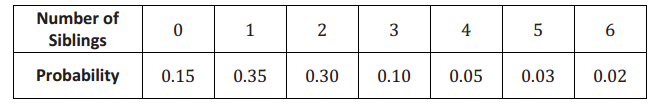
Explain how you could use simulation to estimate the probability that you will need to ask at least five students the question, “Are you an only child?” before you find one that is an only child.
Answer:
a. \(\frac{8}{38}\) ≈ 0.21
b. \(\frac{3+0+1+1}{38}\) = \(\frac{5}{38}\) ≈ 0.13
c. Put 100 counting chips in a bag with 15 red and 85 blue. Red represents the portion of students who don’t have a sibling, and blue represents the portion of students who have one or more siblings. Pull out chips until you get a red one (putting the chips back each time), and record the number of tries it took until a red chip was pulled out. Repeat 1000 times, and see how often you need to try 5 or more times to get a red chip.
Question 2.
A cell phone company wants to predict the probability of a seventh grader in your city, City A, owning a cell phone. Records from another city, City B, indicate that 201 of 1,000 seventh graders own a cell phone.
a. Assuming the probability of a seventh grader owning a cell phone is similar for the two cities, estimate the probability that a randomly selected seventh grader from City A owns a cell phone.
b. The company estimates the probability that a randomly selected seventh-grade male owns a cell phone is 0.25. Does this imply that the probability that a randomly selected seventh-grade female owns a cell phone is 0.75? Explain.
c. According to the data, which of the following is more likely?
- A seventh-grade male owning a cell phone
- A seventh grader owning a cell phone
Explain your choice.
Suppose the cell phone company sells three different plans to its customers:
- Pay-as-you-go: The customer is charged per minute for each call.
- Unlimited minutes: The customer pays a flat fee per month and can make unlimited calls with no additional charges.
- Basic plan: The customer is not charged per minute unless the customer exceeds 500 minutes in the month; then, the customer is charged per minute for the extra minutes.
Consider the chance experiment of selecting a customer at random and recording which plan she purchased.
d. What outcomes are in the sample space for this chance experiment?
e. The company wants to assign probabilities to these three plans. Explain what is wrong with each of the following probability assignments.
Case 1: The probability of pay-as-you-go is 0.40, the probability of unlimited minutes is 0.40, and the probability of the basic plan is 0.30.
Case 2: The probability of pay-as-you-go is 0.40, the probability of unlimited minutes is 0.70, and the probability of the basic plan is -0.10.
Now, consider the chance experiment of randomly selecting a cell phone customer and recording both the cell phone plan for that customer and whether or not the customer exceeded 500 minutes last month.
f. One possible outcome of this chance experiment is (pay-as-you-go, over 500). What are the other possible outcomes in this sample space?
g. Assuming the outcomes of this chance experiment are equally likely, what is the probability that the selected cell phone customer had a basic plan and did not exceed 500 minutes last month?
h. Suppose the company randomly selects 500 of its customers and finds that 140 of these customers purchased the basic plan and did not exceed 500 minutes. Would this cause you to question the claim that the outcomes of the chance experiment described in part (g) are equally likely? Explain why or why not.
Answer:
a. \(\frac{201}{1000}\) = 0.201
b. No. 0.75 would be the probability a male does not own a cell phone. The probabilities for females could be very different.
c. male = 0.25 > overall = 0.201 This implies the probability is a little higher for male seventh-grade students than for all seventh-grade students, so males are more likely to own a cell phone.
d. (1) pay-as-you-go
(2) unlimited minutes
(3) basic plan
e. Case 1: The sum of the three probabilities in the sample space is larger than 1, which cannot happen.
Case 2: There cannot be negative probabilities.
f. (pay-as-you-go, < 500)
(basic plan, ≥ 500)
(basic plan, < 500) (unlimited, > 500)
(unlimited, < 500)
(put exactly 500 in < 500)
g. \(\frac{1}{6}\) ≈ 0.167
h. \(\frac{140}{500}\) = 0.280
Yes. 0.280 is a lot higher than 0.167, which seems to indicate this outcome is more likely than some of the others.
Question 3.
In the game of darts, players throw darts at a circle divided into 20 wedges. In one variation of the game, the score for a throw is equal to the wedge number that the dart hits. So, if the dart hits anywhere in the 20 wedge, you earn 20 points for that throw.

a. If you are equally likely to land in any wedge, what is the probability you will score 20 points?
b. If you are equally likely to land in any wedge, what is the probability you will land in the upper right and score 20, 1, 18, 4, 13, or 6 points?
c. Below are the results of 100 throws for one player. Does this player appear to have a tendency to land in the upper right more often than we would expect if the player were equally likely to land in any wedge?

Answer:
a. \(\frac{1}{20}\) = 0.05
b. \(\frac{6}{20}\) = 0.30
c. \(\frac{7+6+3+4+6+4}{100}\) = \(\frac{30}{100}\) = 0.30
This is exactly how often we would predict this to happen.