Engage NY Eureka Math Geometry Module 1 Lesson 30 Answer Key
Eureka Math Geometry Module 1 Lesson 30 Opening Exercise Answer Key
Opening Exercise:
In ∆ABC to the right, D is the midpoint of \(\overline{A B}\), E is the midpoint of \(\overline{B C}\), and F is the midpoint of \(\overline{A C}\). Complete each statement below.
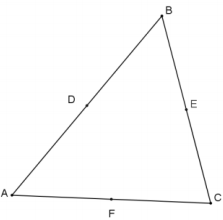
\(\overline{D E}\) is parallel to _____ and measures ______ the length of _______.
Answer:
\(\overline{A C}\), \(\frac{1}{2}\), \(\overline{A C}\)
\(\overline{D F}\) is parallel to _____ and measures ______ the length of _______.
Answer:
\(\overline{B C}\), \(\frac{1}{2}\), \(\overline{B C}\)
\(\overline{E F}\) is parallel to _____ and measures _______ the length of ______.
Answer:
\(\overline{A B}\), \(\frac{1}{2}\), \(\overline{A B}\)
Discussion:
In the previous two lessons, we proved that (a) the midsegment of a triangle is parallel to the third side and half the length of the third side and (b) diagonals of a parallelogram bisect each other. We use both of these facts to prove the following assertion:
All medians of a triangle are ____. That is, the three medians of a triangle (the segments connecting each vertex to the midpoint of the opposite side) meet at a single point. This point of concurrency is called the ____, or the center of gravity, of the triangle. The proof also shows a length relationship for each median: The length from the vertex to the centroid is ______ the length from the centroid to the midpoint of the side.
Answer:
concurrent, centroid, twice
Eureka Math Geometry Module 1 Lesson 30 Example Answer Key
Example 1:
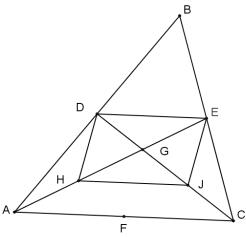
Provide a valid reason for each step in the proof below.
Given: ∆ABC with D, E, and F the midpoints of sides \(\overline{A B}\), \(\overline{B C}\), and \(\overline{A C}\), respectively
Prove: The three medians of ABC meet at a single point.
Question (1)
Draw midsegment \(\overline{D E}\). Draw \(\overline{A E}\) and \(\overline{D C}\) label their intersection as point G.
Answer:
Question (2)
Construct and label the midpoint of \(\overline{A G}\) as point H and the midpoint of \(\overline{G C}\) as point J.
Answer:
Question (3)
\(\overline{D E}\) || \(\overline{A C}\),
Answer:
\(\overline{D E}\) is a midsegment of ∆ABC.
Question (4)
\(\overline{H J}\) || \(\overline{A C}\),
Answer:
\(\overline{H J}\) is a midsegment of ∆AGC.
Question (5)
\(\overline{D E}\) || \(\overline{H J}\),
Answer:
If two segments are parallel to the same segment, then they are parallel to each other.
Question (6)
DE = \(\frac{1}{2}\)AC and HJ = \(\frac{1}{2}\)AC,
Answer:
Definition of a midsegment.
Question (7)
DEJH is a parallelogram.
Answer:
One pair of sides of a quadrilateral are parallel and equal in length.
Question (8)
HG = EG and JG = DG,
Answer:
Diagonals of a parallelogram bisect each other.
Question (9)
AH = HG and CJ = JG,
Answer:
Definition of a midpoint
Question (10)
AH = HG = GE and CJ = JG = GD,
Answer:
Substitution property of equality
Question (11)
AG = 2GE and CG = 2GD,
Answer:
Partition property or segment addition.
Question (12)
We can complete Steps (1) – (11) to include the median from B; the third median, \(\overline{B F}\), passes through point G, which divides it into two segments such that the longer part is twice the shorter.
Answer:
Question (13)
The intersection point of the medians divides each median into two parts with lengths in a ratio of 2 : 1; therefore, all medians are concurrent at that point.
The three medians of a triangle are concurrent at the centroid _______. or the center of gravity. This point of concurrency divides the length of each median in a ratio of _______; the length from the vertex to the centroid is ________ the length from the centroid to the midpoint of the side.
Answer:
centroid, 2 : 1, twice
Example 2:
In ∆ABC, the medians are concurrent at F. DF = 4, BF = 16, AG = 30. Find each of the following measures.
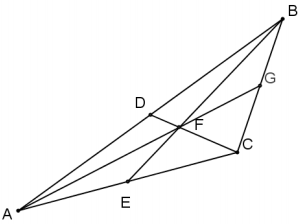
a. FC =
Answer:
8
b. DC =
Answer:
12
c. AF =
Answer:
20
d. BE =
Answer:
24
e. FG =
Answer:
10
f. EF =
Answer:
8
Example 3:
In the figure to the right, ∆ABC is reflected over AB to create ∆ABD. Points P, E, and F are midpoints of AB, BD, and \(\overline{B C}\), respectively. If AH = AG, prove that PH = GP.
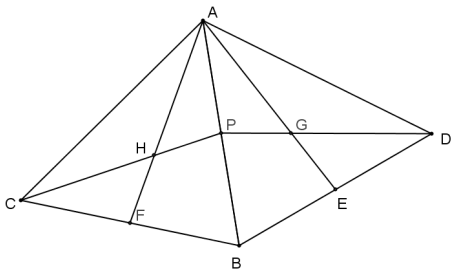
Answer:
ABC is reflected over \(\overline{A B}\) to create ∆ABD.
AF = AE Segments preserved, rigid motion
∠FAB ≅ ∠EAB Angles preserved, rigid motion
AH = AG Given
AP = AP Reflexive property C
∆APH ≅ ∆APG SAS
PH = GP Corresponding sides of congruent triangles are equal in measure.
Eureka Math Geometry Module 1 Lesson 30 Problem Set Answer Key
Ty is building a model of a hang glider using the template below. To place his supports accurately, Ty needs to locate the center of gravity on his model.
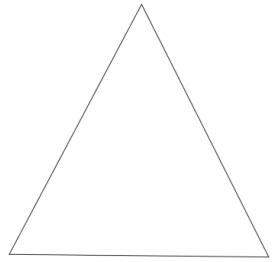
Question 1.
Use your compass and straightedge to locate the center of gravity on Ty’s model.
Answer:
Question 2.
Explain what the center of gravity represents on Ty’s model.
Answer:
The center of gravity is the centroid.
Question 3.
Describe the relationship between the longer and shorter sections of the line segments you drew as you located the center of gravity.
Answer:
The centroid divides the length of each median in a ratio of 2 : 1.
Eureka Math Geometry Module 1 Lesson 30 Exit Ticket Answer Key
Question 1.
DQ, FP, and RE are all medians of DEF, and C is the centroid. DQ = 24, FC = 10, RC = 7. Find DC, CQ, FP, and
CE.
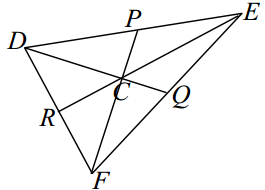
Answer:
Dc = 16, CQ = 8, FP = 15, and CE = 14.