Engage NY Eureka Math Algebra 2 Module 1 Lesson 8 Answer Key
Eureka Math Algebra 2 Module 1 Lesson 8 Opening Exercise Answer Key
Opening Exercise: When is 2n – 1 prime and when is it composite?
Complete the table to investigate which numbers of the form 2 – 1 are prime and which are composite.
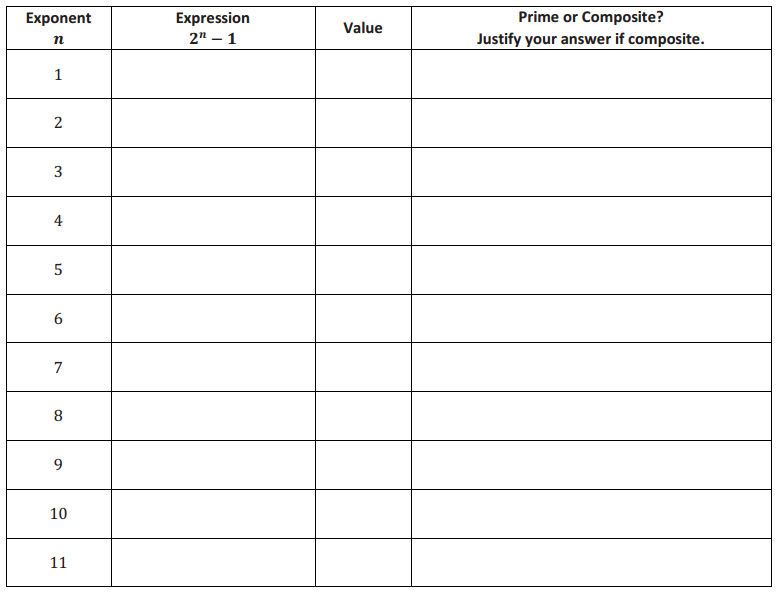
Answer:
What patterns do you notice in this table about which expressions are prime and which are composite?
Answer:
Answers will vary. Suggested responses are in the discussion questions
Eureka Math Algebra 2 Module 1 Lesson 8 Exercise Answer Key
Exercises 1 – 3
For Exercises 1 – 3, find a factor of each expression using the method discussed in Example 1.
Exercise 1.
215 – 1
Answer:
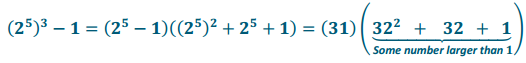
Thus, 31 is a factor of 215 – 1.
Exercise 2.
299 – 1
Answer:
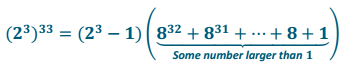
Thus, 7 is a factor of 299 – 1.
Exercise 3.
2537 – 1 (Hint: 537 is the product of two prime numbers that are both less than 50.)
Answer:
Using a calculator we see that 537 = 17 ∙ 31, so
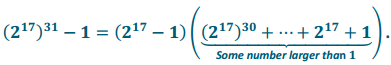
Thus, 217 – 1 is a factor of 2537 – 1.
Exercise 4.
How quickly can a computer factor a very large number?
How long would It take a computer to factor some squares of very large prime numbers?
The time in seconds required to factor an n-digit number of the form p2, where p is a large prime, can roughly be approximated by f(n) = 3.4 × 10(n – 13)/2. Some values of this function are listed in the table below.
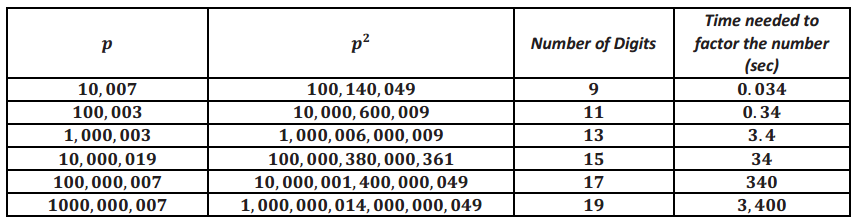
Use the function given above to determine how long it would take this computer to factor a number that contains 32 digits.
Answer:
Using the given function, f(32) = 1.08 × 1010 seconds = 1.80 × 108 minutes = 3 × 106 hours = 125, 000 days, which is about 342.5 years.
Eureka Math Algebra 2 Module 1 Lesson 8 Problem Set Answer Key
Question 1.
Factor 412 – 1 in two different ways using the identity xn – an = (x – a) (xn + axn – 1 + a2xn-2 + ……… + an) and the difference of squares identity.
Answer:
(46 – 1) (46 + 1)
(4 – 1) (411 + 410 + ……. + 4 + 1)
Question 2.
Factor 212 + 1 using the identity xn + an = (x + a) (xn – axn-1 + a2xn-2 + ……… + an) for odd numbers n.
Answer:
(24)3 + 1 = (24 + 1) ((24)2 – 24 + 1)
Question 3.
Is 10, 000, 000, 001 prime? Explain your reasoning.
Answer:
No, because it is of the form 1010 + 1 which could be written as (102)5 + 1 = (102 + 1) ((102)4 – …. + 1).
Question 4.
Explain why 2n – 1 is never prime if n is a composite number.
Answer:
If n is composite, then it can be written in the form n = ab where a and b are integers larger than 1.
Then 2n -1 = 2ab – 1 = (2a)b – 1 = (2a – 1) ((2a)b – 1 + …….. + 2a + 1 For a > 1, this number will be composite because 2a – 1 will be larger than 1.
Question 5.
Fermat numbers are of the form 2n + 1 where n is a positive integer.
a. Create a table of Fermat numbers for odd values of n up to 9.
| n | 2n + 1 |
| 1 | |
| 3 | |
| 5 | |
| 7 | |
| 9 |
Answer:
| n | 2n + 1 |
| 1 | 21 + 1 = 3 |
| 3 | 23 + 1 = 9 |
| 5 | 25 + 1 = 33 |
| 7 | 27 + 1 = 129 |
| 9 | 29 + 1 = 513 |
b. Explain why if n is odd, the Fermat number 2n + 1 will always be divisible by 3.
Answer:
The Fermat number 2n + 1 will factor as (2 + 1)(2n – 1 – 2n – 2 + …….. + 1) using the identity in Exercise 2.
c. Complete the table of values for even values of n up to 12.
| n | 2n + 1 |
| 2 | |
| 4 | |
| 6 | |
| 8 | |
| 10 | |
| 12 |
Answer:
| n | 2n + 1 |
| 2 | 22 + 1 = 5 |
| 4 | 24 + 1 = 17 |
| 6 | 26 + 1 = 65 |
| 8 | 28 + 1 = 257 |
| 10 | 210 + 1 = 1,025 |
| 12 | 212 + 1 = 4,097 |
d. Show that if n can be written in the form 2k where k is odd, then 2n + 1 is divisible by 5.
Answer:
Let n = 2k, where k is odd. Then
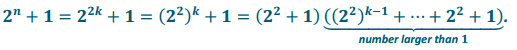
Since 22 + 1 = 5, we know that 5 is a factor of 2n + 1. This only holds when k is an odd number because that is the only case when we can factor expressions of the form xk + 1.
e. Which even numbers are not divisible by an odd number? Make a conjecture about the only Fermat numbers that might be prime.
Answer:
The powers of 2 are the only positive integers that are not divisible by any odd numbers. This implies that when the exponent n in 2n + 1 is a power of 2, the Fermat number 2n + 1 might be prime.
Question 6.
Complete this table to explore which numbers can be expressed as the difference of two perfect squares.
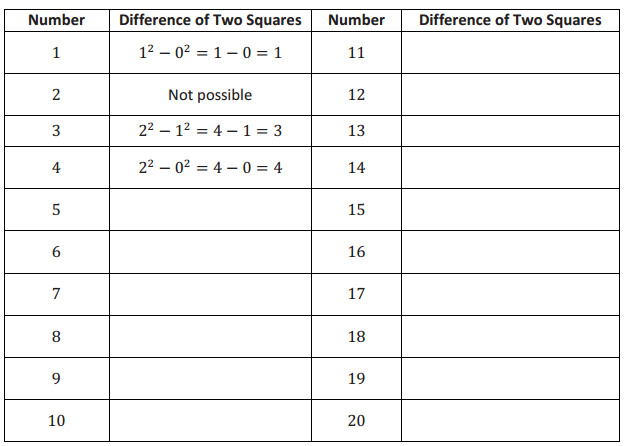
Answer:
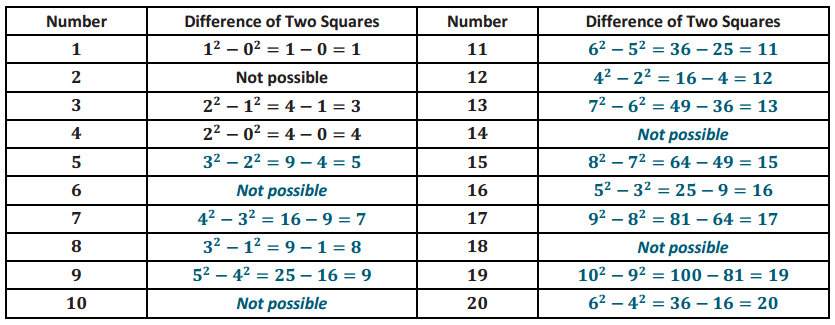
a. For which odd numbers does it appear to be possible to write the number as the difference of two squares?
Answer:
It appears that we can write any positive odd number as the difference of two squares.
b. For which even numbers does it appear to be possible to write the number as the difference of two squares?
Answer:
It appears that we can write any multiple of 4 as the difference of two squares.
c. Suppose that n is an odd number that can be expressed as n = a2 – b2for positive integers a and b. What do you notice about a and b?
Answer:
When n is odd, a and b are consecutive whole numbers and a + b = n.
d. Suppose that n is an even number that can be expressed as n = a2 – b2 for positive integers a and b. What do you notice about a and b?
Answer:
When n is an even number that can be written as a difference of squares, then n is a multiple of 4, and a and b are either consecutive even integers or consecutive odd integers. We also have a + b = \(\frac{n}{2}\).
Question 7.
Express the numbers from 21 to 30 as the difference of two squares, if possible.
Answer:
This is not possible for 22, 26, and 30, Otherwise we have the following.
21 = 112 – 102
23 = 122 – 112
24 = 72 – 52
25 = 132 – 122
27 = 142 – 132
28 = 82 – 62
29 = 152 – 142
Question 8.
Prove this conjecture: Every positive odd number m can be expressed as the difference of the squares of two consecutive numbers that sum to the original number m.
a. Let m be a positive odd number. Then for some integer n, m = 2n + 1. We will look at the consecutive
integers n and n + 1. Show that n + (n + 1) = m.
Answer:
n + (n + 1) = n + n + 1
= 2n + 1
= m
b. What is the difference of squares of n + 1 and n?
Answer:
(n + 1)2 – n2 = n2 + 2n + 1 – n2
= 2n + 1
= m
c. What can you conclude from parts (a) and (b)?
Answer:
We can write any positive odd number m as the difference of squares of two consecutive numbers that sum to m.
Question 9.
Prove this conjecture: Every positive multiple of ??can be expressed as the difference of squares of two numbers that differ by 2. Use the table below to organize your work for parts (a) – (c).
a. Write each multiple of 4 in the table as a difference of squares.
Answer:
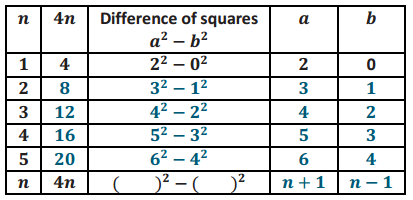
b. What do you notice about the numbers a and b that are squared? How do they relate to the number n?
Answer:
The values of a and b in the differences of two squares differ by 2 every time. They are one larger and one smaller than n; that is, a = n + 1 and b = n – 1.
c. Given a positive integer of the form 4n, prove that there are integers a and b so that 4n = a2 – b2 and that a – b = 2. (Hint: Refer to parts (a) and (b) for the relationship between n and a and b.)
Answer:
Define a = n + 1 and b = n – 1 Then we can calculate a2 – b2 as follows.
a2 – b2 = (n + 1)2 – (n – 1)2
= ((n + 1) + (n – 1)) ((n + 1) – (n – 1))
= (2n) (2)
= 4n
We can also see that
a – b = (n + 1) – (n – 1)
= n + 1 – n + 1
= 2.
Thus every positive multiple of 4 can be written as a difference of squares of two integers that differ by 2.
Question 10.
The steps below prove that the only positive even numbers that can be written as a difference of square integers are the multiples of 4. That is, completing this exercise will prove that it is impossible to write a number of the form 4n – 2 as a difference of square integers.
a. Let m be a positive even integer that we can write as the difference of square integers m = a2 – b2. Then m = (a + b) (a – b) for integers a and b. How do we know that either a and b are both even or a and b are both odd?
Answer:
If one of a and b is even and the other one is odd, then one of a2 and b2 is even and the other one is odd. Since the difference of an odd and an even number is odd, this means that m = a2 – b2 would be odd. Since we know that m is even, it must be that either a and b are both even or a and b are both odd.
b. Is a + b even or odd? What about a – b? How do you know?
Answer:
Since a and b are either both odd or both even, we know that both a + b and a – b are even.
c. Is 2 a factor of a + b? Is 2 a factor of a – b? Is 4 a factor of (a + b) (a – b)? Explain how you know.
Answer:
Because a + b and a – b are both even, 2 is a factor of both a + b and a – b. Thus, 22 = 4 is a factor of (a + b) (a – b).
d. Is 4 a factor of any integer of the form 4n – 2?
Answer:
No. If 4 were a factor of 4n – 2, we could factor it out: 4n – 2 = 4 (n – \(\frac{1}{2}\)). But this means that n – \(\frac{1}{2}\) is an integer, which it clearly is not. This means that 4 is not a factor of any number of the form 4n – 2.
e. What can you conclude from your work in parts (a) – (d)?
Answer:
If m is a positive even integer and m can be written as the difference of two square integers, then m cannot be of the form 4n – 2 for any integer n. Another way to say this is that the positive integers of the form 4n – 2 for some integer n cannot be written as the difference of two square integers.
Question 11.
Explain why the prime number 17 can only be expressed as the difference of two squares in only one way, but the composite number 24 can be expressed as the difference of two squares in more than one way.
Answer:
Since every odd number can be expressed as the difference of two squares, a2 – b2 = (a + b) (a – b), the number
17 must fit this pattern. Because 17 is prime, there is only one way to factor 17, which is 17 = 1 ∙ 17.
Let a + b = 17 and a – b = 1. The two numbers that satisfy this system of equations are 8 and 9. Thus,
17 = 1 ∙ 17
= (9 – 8) (9 + 8)
= 92 – 82.
A composite number has more than one factorization, not all of which will lead to writing the number as the difference of squares of two integers. For the number 24, you could use
24 = 2 ∙ 12
= (7 – 5) (7 + 5)
= 72 – 52.
Or, you could use
24 = 4 ∙ 6
= (5 – 1) (5 + 1)
= 52 – 12.
Question 12.
Explain why you cannot use the factors of 3 and 8 to rewrite 24 as the difference of two square integers.
Answer:
If 24 = 3 ∙ 8, then a – b = 3 and a + b = 8. The solution to this system of equations is (5.5, 2.5). If we are
restricting this problem to the set of whole numbers, then you cannot apply the identity to rewrite ?? as the difference of two perfect squares where a and b are whole numbers. It certainly is true that
24 = (5. 5 – 2. 5) (5. 5 + 2. 5) = 5. 52 – 2.52, but this is not necessarily an easy way to calculate 24.
Eureka Math Algebra 2 Module 1 Lesson 8 Exit Ticket Answer Key
Question 1.
Express the prime number 31 in the form 2p – 1 where p is a prime number and as a difference of two perfect squares using the identity (a + b)(a – b) = a2 – b2.
Answer:
31 = (16 – 15) (16 + 15)
31 = 25 – 1
= 162 – 152