Big Ideas Math Algebra 1 Answers Chapter 10 Radical Functions and Equations

Your practice session without Big Ideas Math Algebra 1 Answers Chapter 10 Radical Functions and Equations is incomplete. As it includes all crucial and important study resources like Questions from Exercises 10.1 to 10.4, along with Chapter Test, Review Tests, Cumulative Practice, Quiz, etc. So, check out the below modules and enhance your math proficiency by referring to the BIM Algebra 1 Solution Key of Ch 10 Radical Functions and Equations.

Big Ideas Math Book Algebra 1 Answer Key Chapter 10 Radical Functions and Equations

Not only improving your subject knowledge even you can also clear all your queries on math complex concepts of Chapter 10 with the help of the BIM Algebra 1 Answer Key. Just make use of this respective Chapter 10 Big Ideas Math Algebra 1 Radical Functions and Equations Answers and prepare for the exams accordingly. All you have to do is tap on the links and utilize the topic-wise Ch 10 BIM Math Textbook Answers Algebra 1 for your reference without paying a single penny.

Radical Functions and Equations

• Radical Functions and Equations Maintaining Mathematical Proficiency – Page 541
• Radical Functions and Equations Mathematical Practices – Page 542

Lesson: 1 Graphing Square Root Functions

• Lesson 10.1 Graphing Square Root Functions – Page(543-550)
• Graphing Square Root Functions 10.1 Exercises – Page(548-550)

Lesson: 2 Graphing Cube Roots Functions

• Lesson 10.2 Graphing Cube Roots Functions – Page(551-556)
• Graphing Cube Roots Functions 10.2 Exercises – Page(555-556)
• Radical Functions and Equations Study Skills: Making Note Cards – Page 557
• Study Skills: Making Note Cards

Quiz

• Radical Functions and Equations 10.1–10.2 Quiz – Page 558

Lesson: 3 Solving Radical Equations

• Lesson 10.3 Solving Radical Equations – Page(559-566)
• Solving Radical Equations 10.3 Exercises – Page(564-566)

Lesson: 4 Inverse of a Function

• Lesson 10.4 Inverse of a Function – Page(567-574)
• Inverse of a Function 10.4 Exercises – Page(572-574)

Performance Task

• Radical Functions and Equations Performance Task: Medication and the Mosteller Formula – Page 575
• Radical Functions and Equations Chapter Review – Page (576-578)
• Radical Functions and Equations Chapter Test – Page 579
• Radical Functions and Equations Cumulative Assessment – Page(580-581)

Radical Functions and Equations Maintaining Mathematical Proficiency

Evaluate the expression.
Question 1.
7\(\sqrt{25}\) + 10
Answer:
Given the expression
7\(\sqrt{25}\) + 10
\(\sqrt{25}\) = 5
7(5) + 10 = 35 +10 = 45
Thus 7\(\sqrt{25}\) + 10 = 45

Question 2.
-8 – \(\sqrt{\frac{64}{16}}\)
Answer:
Given the expression
-8 – \(\sqrt{\frac{64}{16}}\)
\(\sqrt{\frac{64}{16}}\) = \(\sqrt{4}\) = 2
– 8 – 2 = -10
Thus -8 – \(\sqrt{\frac{64}{16}}\) = -10

Question 3.
\(5\left(\frac{\sqrt{81}}{3}-7\right)\)
Answer:
Given the expression
\(5\left(\frac{\sqrt{81}}{3}-7\right)\)
\(\sqrt{81}\) = 9
5(9/3 – 7)
5(3 – 7) = 5(-4) = -20
Thus \(5\left(\frac{\sqrt{81}}{3}-7\right)\) = -20

Question 4.
-2(3\(\sqrt{9}\) + 13)
Answer:
Given the expression
-2(3\(\sqrt{9}\) + 13)
= -2(3 (3) + 13)
= -2(9 + 13)
= -2(22)
= -44
Thus -2(3\(\sqrt{9}\) + 13) = -44

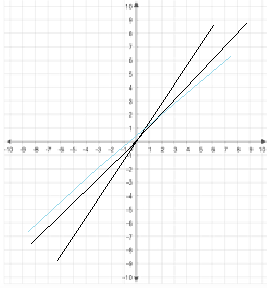
Graph f and g. Describe the transformations from the graph of f to the graph of g.
Question 5.
f(x) = x; g(x) = 2x – 2
Answer:
Given,
f(x) = x,
g(x) = 2x – 2
Substitute the value of x in f(x)
g(x) = 2(f(x)) – 2
h(x) = 2x

Question 6.
f(x) = x; g(x) = \(\frac{1}{3}\)x + 5
Answer:
Given,
f(x) = x
g(x) = \(\frac{1}{3}\)x + 5
Substitute the value of x in f(x)
h(x) = \(\frac{1}{3}\)(f(x)) + 5
h(x) = \(\frac{1}{3}\)x

Question 7.
f(x) = x; g(x) = -x + 3
Answer:
Given,
f(x) = x
g(x) = -x + 3
h(x) = -1(f(x)) + 3
= -x

Question 8.
ABSTRACT REASONING
Let a and b represent constants, where b ≥ 0. Describe the transformations from the graph of m(x) = ax + b to the graph of n(x) = -2ax – 4b.
Answer:

Radical Functions and Equations Mathematical Practices

Mathematically proficient students distinguish correct reasoning from flawed reasoning.

Monitoring Progress

Question 1.
Which of the following square roots are rational numbers? Explain your reasoning.
\(\sqrt{0}, \sqrt{1}, \sqrt{3}, \sqrt{4}, \sqrt{5}, \sqrt{6}, \sqrt{7}, \sqrt{8}, \sqrt{9}\)
Answer:
Zero has one square root which is 0.
\(\sqrt{0}\) is a rational number.
\(\sqrt{1}\) is a rational number.
\(\sqrt{3}\) is a irrational number.
\(\sqrt{4}\) is a rational number.
\(\sqrt{5}\) is a irrational number.
\(\sqrt{6}\) is a irrational number.
\(\sqrt{7}\) is a irrational number.
\(\sqrt{8}\) is a irrational number.
\(\sqrt{9}\) is a rational number.

Question 2.
The sequence of steps shown appears to prove that 1 = 0. What is wrong with this argument?

Answer:
x – 1 = 0
x – 1 + 1 = 1
x = 1

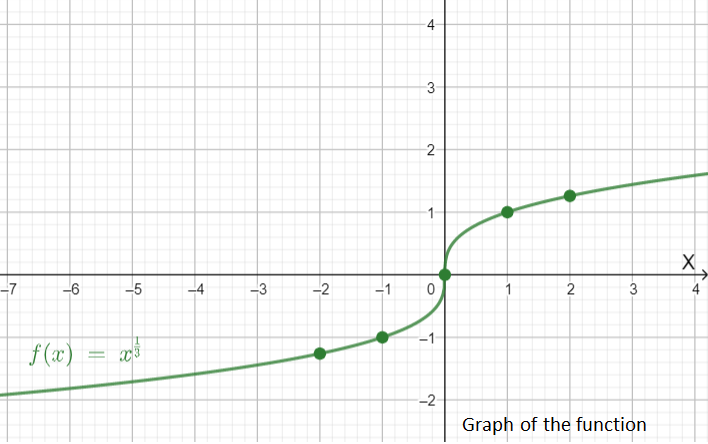
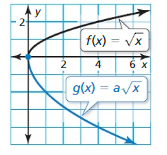
Lesson 10.1 Graphing Square Root Functions

Essential Question What are some of the characteristics of the graph of a square root function?

EXPLORATION 1

Graphing Square Root Functions
Work with a partner.

• Make a table of values for each function.
• Use the table to sketch the graph of each function.
• Describe the domain of each function.
• Describe the range of each function.

Answer:

EXPLORATION 2

Writing Square Root Functions
Work with a partner. Write a square root function, y = f (x), that has the given values. Then use the function to complete the table.

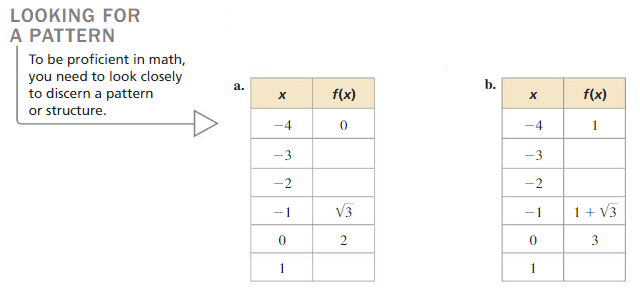
Answer:

Communicate Your Answer

Question 3.
What are some of the characteristics of the graph of a square root function?
Answer:
The domain of the square root function f(x)=√x is given in interval form by: [0,+∞)
The range of the square root function f(x)=√x is given in interval form by: [0,+∞)
The x and y intercepts are both at (0,0)
The square root function is an increasing function.

Question 4.
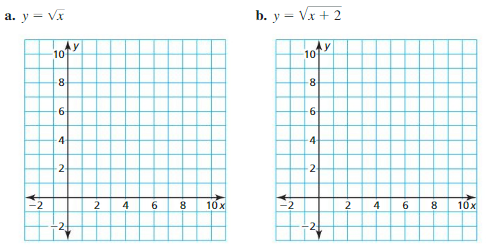
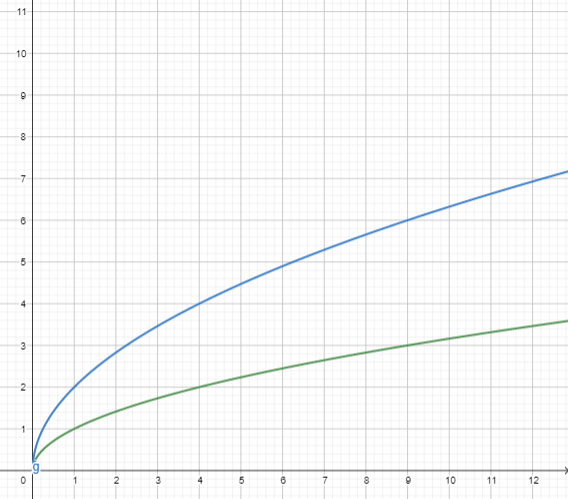
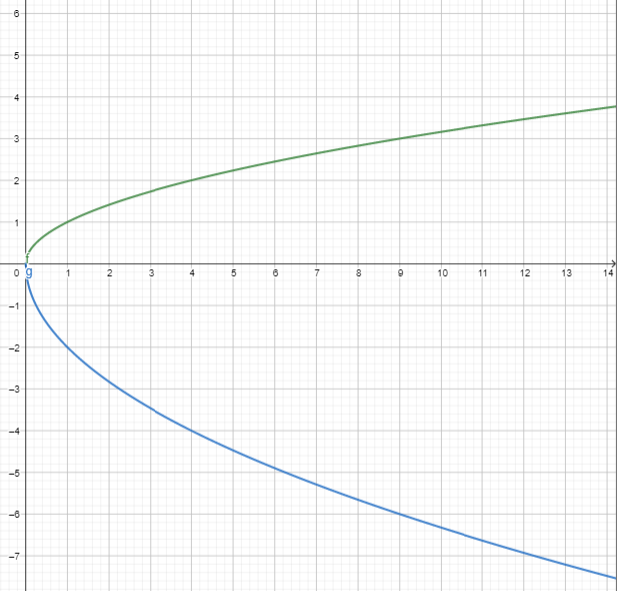
Graph each function. Then compare the graph to the graph of f(x) = \(\sqrt{x}\).
a. g(x) = \(\sqrt{x-1}\)
b. g(x) = \(\sqrt{x-1}\)
c. g(x) = 2\(\sqrt{x}\)
d. g(x) = -2 \(\sqrt{x}\)
Answer:
a. g(x) = \(\sqrt{x-1}\)

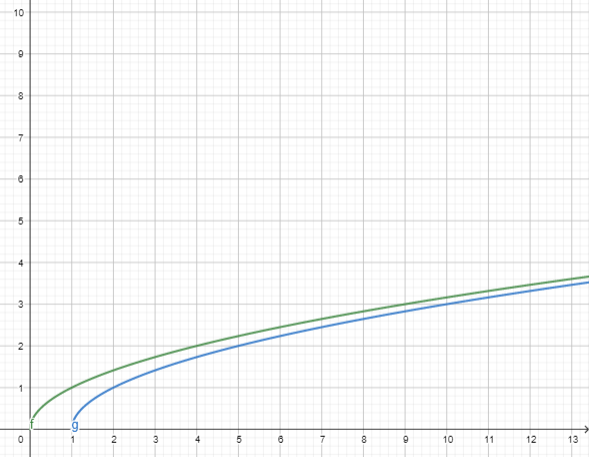
b. g(x) = \(\sqrt{x-1}\)

c. g(x) = 2\(\sqrt{x}\)

d. g(x) = -2 \(\sqrt{x}\)

Monitoring Progress

Describe the domain of the function.
Question 1.
f(x) = 10 \(\sqrt{x}\)
Answer:

Question 2.
y = \(\sqrt{2x}\) + 7
Answer:

Question 3.
h(x) = \(\sqrt{-x+1}\)
Answer:

Graph the function. Describe the range.
Question 4.
g(x) = \(\sqrt{x}\) – 4
Answer:

Question 5.
y = \(\sqrt{2x}\) + 5
Answer:

Question 6.
n(x) = 5\(\sqrt{x}\)
Answer:

Graph the function. Compare the graph to the graph of f(x) = \(\sqrt{x}\) .
Question 7.
h(x) = \(\sqrt{\frac{1}{4} x}\)
Answer:

Question 8.
g(x) = \(\sqrt{x}\) – 6
Answer:

Question 9.
m(x) = -3\(\sqrt{x}\)
Answer:

Question 10.
Let g(x) = \(\frac{1}{2} \sqrt{x+4}+1\). Describe the transformations from the graph of f(x) = \(\sqrt{x}\) to the graph of g. Then graph g.
Answer:

Question 11.
In Example 5, compare the velocities by finding and interpreting their average rates of change over the interval d = 30 to d = 40.
Answer:

Question 12.
WHAT IF?
At what depth does the velocity of the tsunami exceed 100 meters per second?
Answer:
y = \(\sqrt{9.8x}\)

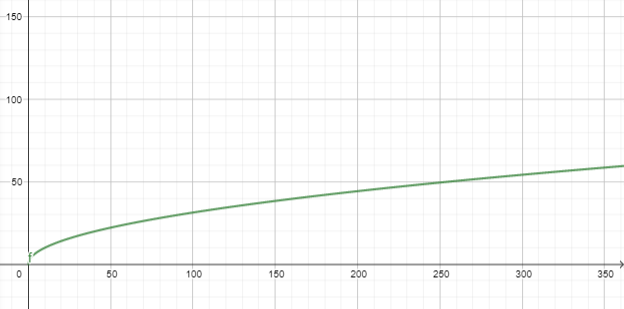
The depth at which the velocity of the tsunami exceeds 100 meters per second is 1021 meters.

Graphing Square Root Functions 10.1 Exercises

Vocabulary and Core Concept Check

Question 1.
COMPLETE THE SENTENCE
A ________ is a function that contains a radical expression with the independent variable in the radicand.
Answer:
A radical is a function that contains a radical expression with the independent variable in the radicand.

Question 2.
VOCABULARY
Is y = 2x\(\sqrt{5}\) a square root function? Explain.
Answer:
No; y = 2x\(\sqrt{5}\) is a linear function.

Question 3.
WRITING
How do you describe the domain of a square root function?
Answer:
The domain of a square root function is the x-values for which the radicand is greater than or equal to 0.

Question 4.
REASONING
Is the graph of g(x) = 1.25\(\sqrt{x}\) a vertical stretch or a vertical shrink of the graph of f(x) = \(\sqrt{x}\)? Explain.
Answer:
g(x) = 1.25\(\sqrt{x}\)
Graph of g(x) is vertical stretch of graph f(x) = \(\sqrt{x}\)
Since corresponding to each x value of g(x) is more than f(x)

Monitoring Progress and Modeling with Mathematics

In Exercises 5–14, describe the domain of the function.
Question 5.
y = 8\(\sqrt{x}\)
Answer:
x ≥ 0
The domain is the set of real numbers greater than or equal to 0.

Question 6.
y = \(\sqrt{4x}\)
Answer:
4x ≥ 0 that implies x ≥ 0
Therefore the domain is [0, ∞)

Question 7.
y = 4 + \(\sqrt{-x}\)
Answer:

Question 8.
y = \(\sqrt{-\frac{1}{2^{x}}}\) + 1
Answer:
y = \(\sqrt{-\frac{1}{2^{x}}}\) + 1 to be defined
–\(\frac{1}{3}\) x ≥ 0
x  ≤ 0
Therefore the domain is (-∞, 0]

Question 9.
h(x) = \(\sqrt{x-4}\)
Answer:

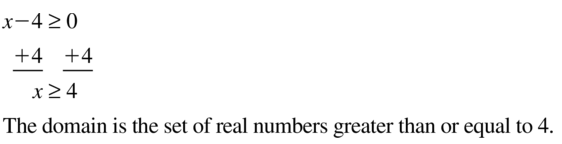
Question 10.
p(x) = \(\sqrt{x+7}\)
Answer:
The square root will be defined only when the function inside the square root must be non negative.
p(x) = \(\sqrt{x+7}\) to be defined
x + 7 ≥ 0
x ≥ -7
Therefore the domain is [-7, ∞]

Question 11.
f(x) = \(\sqrt{-x+8}\)
Answer:

Question 12.
g(x) = \(\sqrt{-x-1}\)
Answer:
g(x) = \(\sqrt{-x-1}\)
-x – 1 ≥ 0
-(x + 1)≥ 0
x + 1 ≤ 0
x ≤ -1
Therefore the domain is (-∞, -1]

Question 13.
m(x) = 2\(\sqrt{x+4}\)
Answer:

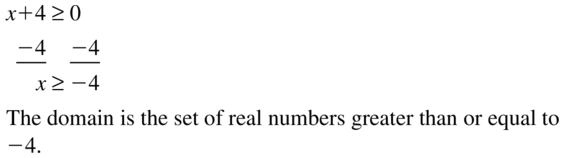
Question 14.
n(x) = \(\frac{1}{2} \sqrt{-x}-2\)
Answer:
n(x) = \(\frac{1}{2} \sqrt{-x}-2\)
-x – 2 ≥ 0
-(x + 2)≥ 0
x + 2 ≤ 0
x ≤ -2
Therefore the domain is (-∞, -2]

In Exercises 15–18, match the function with its graph. Describe the range.
Question 15.
y = \(\sqrt{x-3}\)
Answer:

Question 16.
y = 3\(\sqrt{x}\)
Answer:
y = 3\(\sqrt{x}\) ≥ 0∀ x ∈ R
That is the range is [0, ∞)
Thus the correct answer is option C.

Question 17.
y = \(\sqrt{x}\) – 3
Answer:

Question 18.
y = \(\sqrt{-x+3}\)

Answer:
y = \(\sqrt{-x+3}\)
≥ 0∀ x ∈ R
That is the range is [0, ∞)
Thus option A is eliminated
x-intercept of y is 3.
Also as x will decrease y will increase.
Thus the correct answer is option B.

In Exercises 19–26, graph the function. Describe the range.
Question 19.
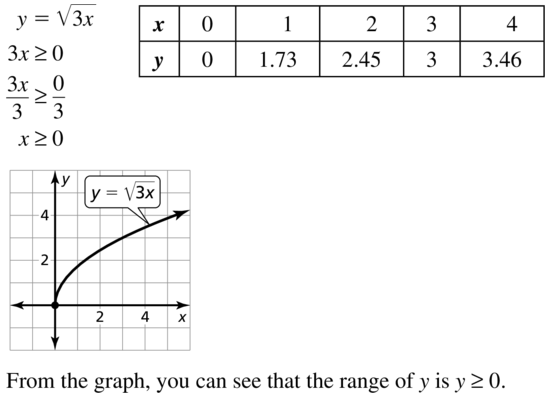
y = \(\sqrt{3x}\)
Answer:

Question 20.
y = 4\(\sqrt{-x}\)
Answer:

Question 21.
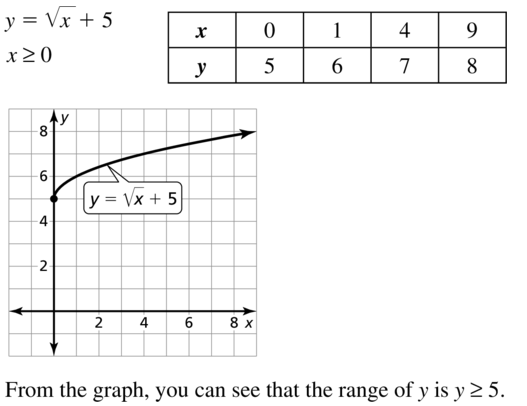
y = \(\sqrt{x}\) + 5
Answer:

Question 22.
y = -2 + \(\sqrt{x}\)
Answer:

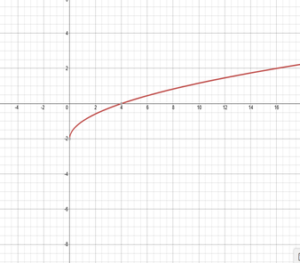
The range is [-2, ∞)

Question 23.
f(x) = – \(\sqrt{x-3}\)
Answer:

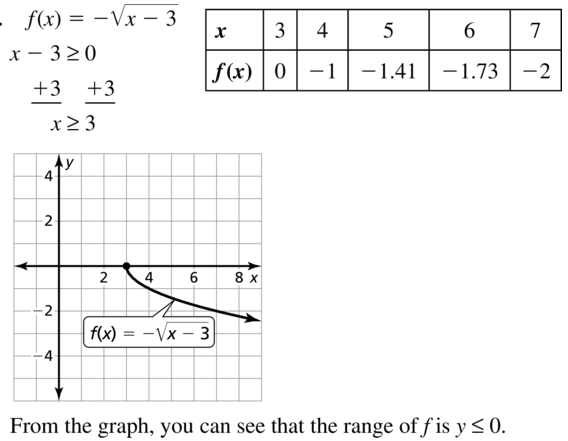
Question 24.
g(x) = \(\sqrt{x+4}\)
Answer:

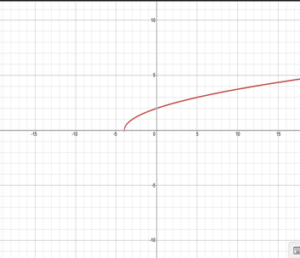
The range is [0, -∞)

Question 25.
h(x) = \(\sqrt{x+2}\) – 2
Answer:

Question 26.
f(x) = –\(\sqrt{x-1}\) + 3
Answer:
Step 1: Use the domain to make a table of values.
The radicand must be greater than or equal to 0
x – 1 ≥ 0
x ≥ 1
Step 2: Plot the ordered pairs
Step 3: Draw a smooth curve through the points starting at (1, 3)

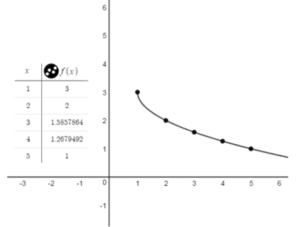
In Exercises 27–34, graph the function. Compare the graph to the graph of f (x) = \(\sqrt{x}\).
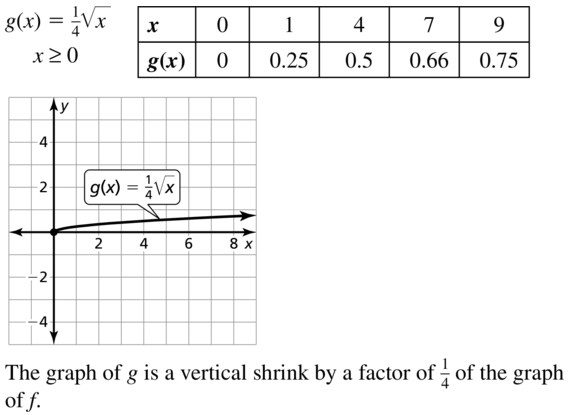
Question 27.
g(x) = \(\frac{1}{4} \sqrt{x}\)
Answer:

Question 28.
r(x) = \(\sqrt{2x}\)
Answer:
x = 0 g(0) = 0
x = 1 g(1) = 1.41
x = 2 g(2) = 2
x = 3 g(3) = 2.45
x = 4 g(4) = 2.83

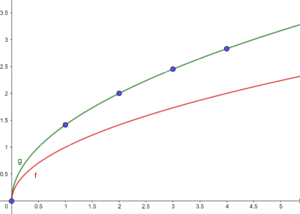
Question 29.
h(x) = \(\sqrt{x+3}\)
Answer:

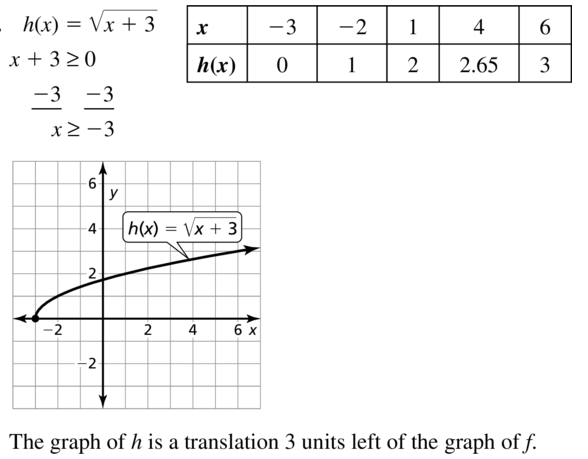
Question 30.
q(x) = \(\sqrt{x}\) + 8
Answer:
Step 1: Use the domain to make a table of values.
The radicand must be greater than or equal to 0
x ≥ 0
Step 2: Plot the ordered pairs
Step 3: Draw a smooth curve through the points starting at (0, 8)

The graph of q(x) is the graph of f(x) that is:
Vertically shifted 8 units upward

Question 31.
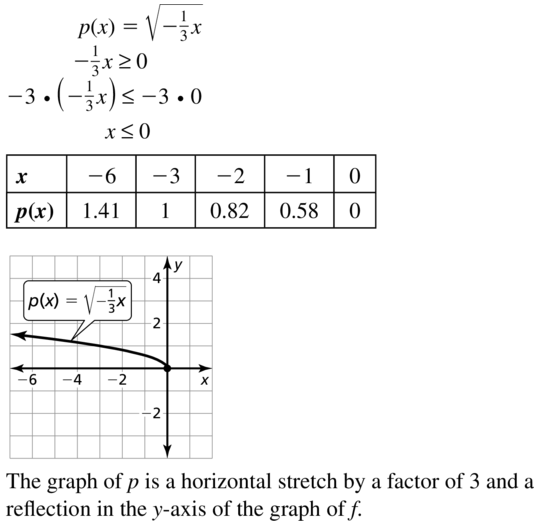
p(x) = \(\sqrt{-\frac{1}{3} x}\)x
Answer:

Question 32.
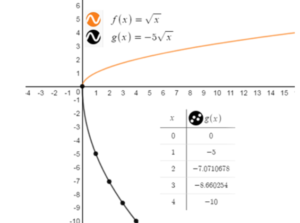
g(x) = -5\(\sqrt{x}\)
Answer:
Step 1: Use the domain to make a table of values.
The radicand must be greater than or equal to 0
x ≥ 0
Step 2: Plot the ordered pairs
Step 3: Draw a smooth curve through the points starting at (0, 0)

The graph of q(x) is the graph of f(x) that is:
Reflected in the x-axis
Vertically stretched by a factor of 5.

Question 33.
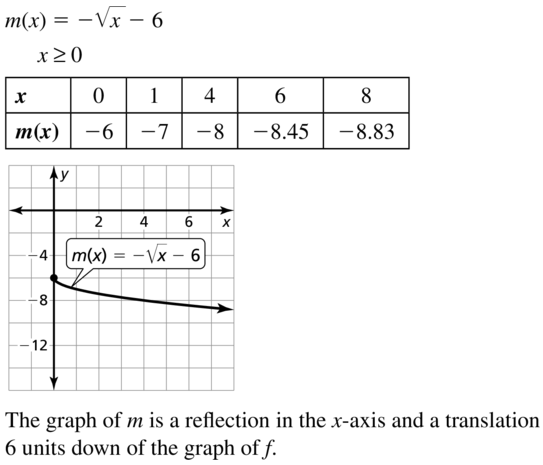
m(x) = –\(\sqrt{x}\) – 6
Answer:

Question 34.
n(x) = –\(\sqrt{x}\) – 4
Answer:
Step 1: Use the domain to make the table of values
The radicand must be greater than or equal to 0
Step 2: Plot the ordered pairs
Step 3: Draw a smooth curve through the points starting at (4, 0)

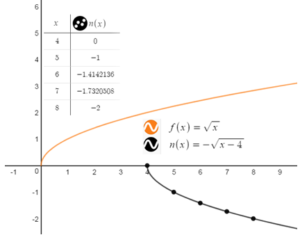
The graph of m(x) is the graph of f(x)
-Reflected in the x-axis
– Horizontally shifted 4 units to the right

Question 35.
ERROR ANALYSIS
Describe and correct the error in graphing the function y = \(\sqrt{x}\) + 1 .

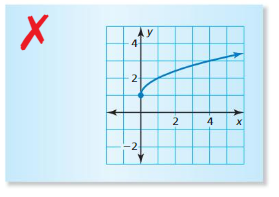
Answer:

Question 36.
ERROR ANALYSIS
Describe and correct the error in comparing the graph of g(x) = \(-\frac{1}{4} \sqrt{x}\) to the graph of f (x) = \(\sqrt{x}\).

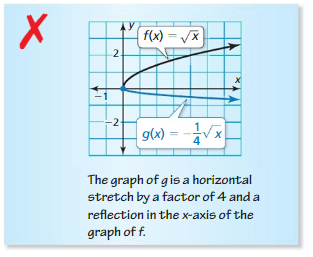
Answer:
The graph of g is a reflection in the x-axis and a vertical shrink by a factor of 1/4 not a horizontal stretch.

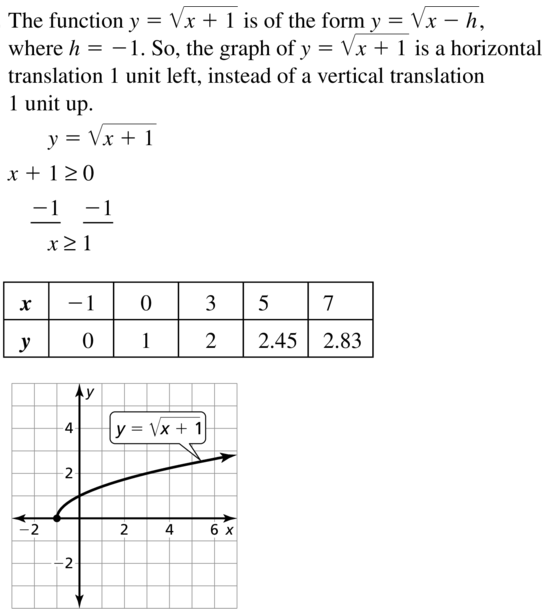
In Exercises 37–44, describe the transformations from the graph of f (x) = \(\sqrt{x}\) to the graph of h. Then graph h.
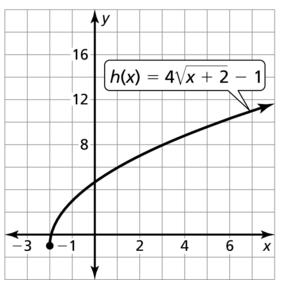
Question 37.
h(x) = 4\(\sqrt{x+2}\) – 1
Answer:

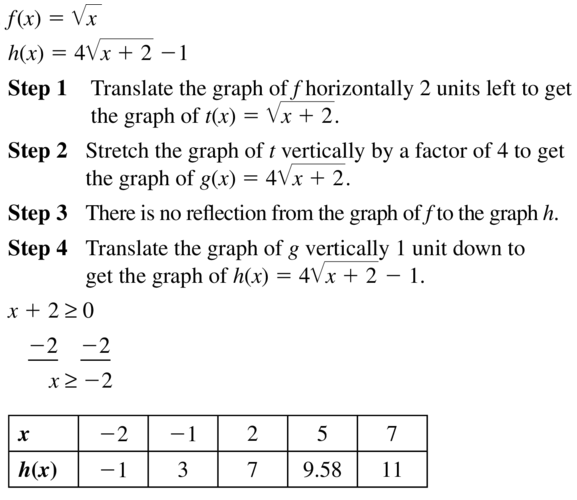
Question 38.
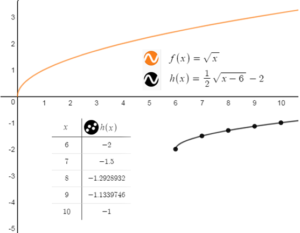
h(x) = \(\frac{1}{2} \sqrt{x-6}\)+ 3
Answer:
The graph of h(x) is the graph of f(x)
-Vertically shrinked by a factor of 1/2 →\(\frac{1}{2} \sqrt{x}\)
– Horizontally shifted 6 units to the right \(\frac{1}{2} \sqrt{x-6}\)
– Vertically shifted 3 units upward → \(\frac{1}{2} \sqrt{x-6}\)+ 3
Step 1: Use the domain to make a table of values.
The radicand must be greater than or equal to 0
x – 6 ≥ 0
x ≥ 6
Step 2: Plot the ordered pairs
Step 3: Draw a smooth curve through the points starting at (6, -2)

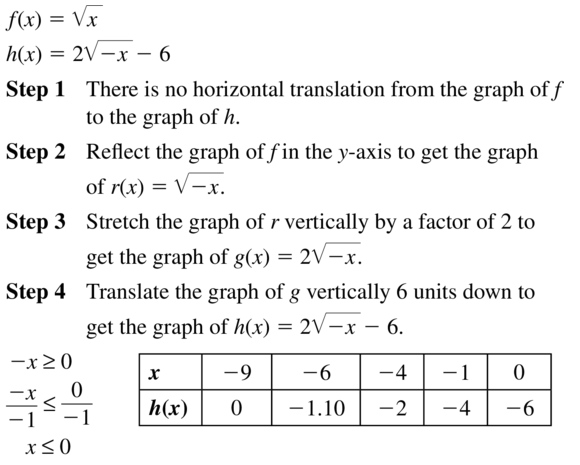
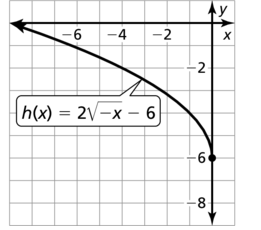
Question 39.
h(x) = 2\(\sqrt{-x}\) – 6
Answer:

Question 40.
h(x) = –\(\sqrt{x-3}\) – 2
Answer:
The graph of h(x) is the graph of f(x)
– Reflected in the x-axis – √x
– Horizontally shifted 3 units to the right → –\(\sqrt{x-3}\)
– Vertically shifted 2 units downward→ –\(\sqrt{x-3}\) – 2
Step 1: Use the domain to make a table of values.
The radicand must be greater than or equal to 0
x – 3 ≥ 0
x ≥ 3
Step 2: Plot the ordered pairs
Step 3: Draw a smooth curve through the points starting at (3, -2)

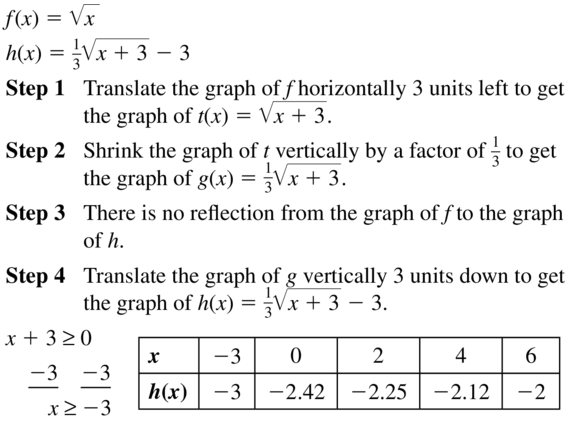
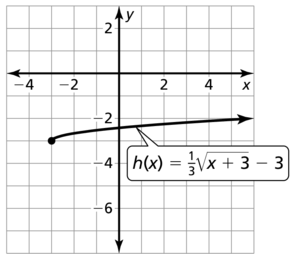
Question 41.
h(x) = \(\frac{1}{3} \sqrt{x+3}\) + 3
Answer:

Question 42.
h(x) = 2\(\sqrt{x-1}\) + 4
Answer:
The graph of h(x) is the graph of f(x)
-Vertically shrinked by a factor of 2 →2\(\sqrt{x}\)
– Horizontally shifted 1 units to the right 2\(\sqrt{x-1}\)
– Vertically shifted 4 units upward → 2\(\sqrt{x-1}\) + 4
Step 1: Use the domain to make a table of values.
The radicand must be greater than or equal to 0
x – 1 ≥ 0
x ≥ 1
Step 2: Plot the ordered pairs
Step 3: Draw a smooth curve through the points starting at (1, 4)

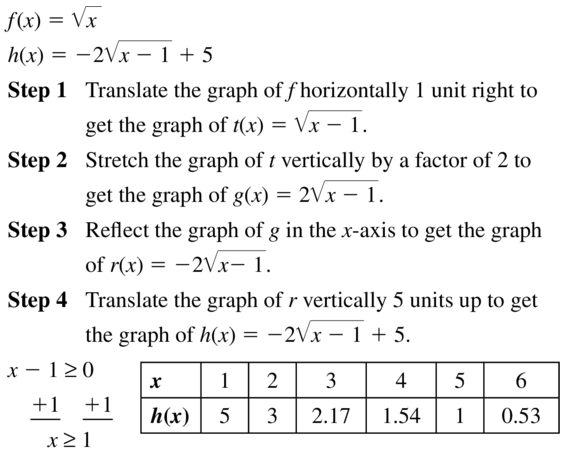
Question 43.
h(x) = -2\(\sqrt{x-1}\) + 5
Answer:

Question 44.
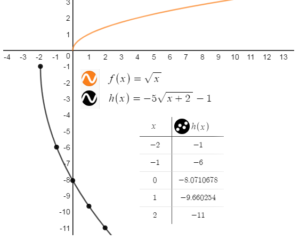
h(x) = -5\(\sqrt{x+2}\) – 1
Answer:
The graph of h(x) is the graph of f(x)
-Reflection in the x-axis → –\(\sqrt{x}\)
-Vertically shrinked by a factor of 5 →-5\(\sqrt{x}\)
– Horizontally shifted 2 units to the left -5\(\sqrt{x+2}\)
– Vertically shifted 1 units downward→ -5\(\sqrt{x+2}\) – 1
Step 1: Use the domain to make a table of values.
The radicand must be greater than or equal to 0
x + 2 ≥ 0
x ≤ -2
Step 2: Plot the ordered pairs
Step 3: Draw a smooth curve through the points starting at (-2, -1)

Question 45.
COMPARING FUNCTIONS
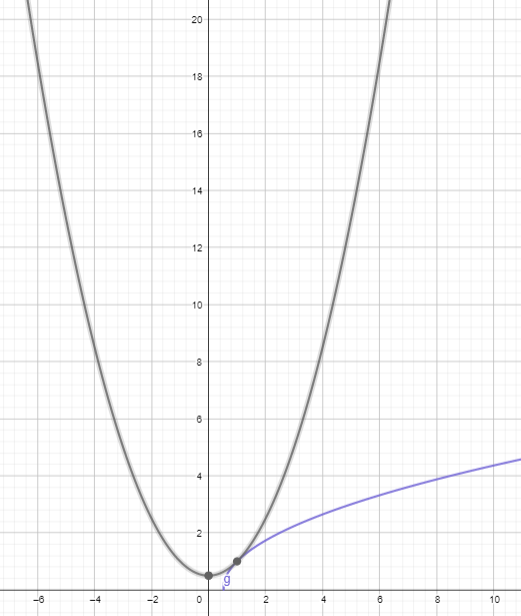
The model S(d ) = \(\sqrt{30df}\) represents the speed S (in miles per hour) of a van before it skids to a stop, where f is the drag factor of the road surface and d is the length (in feet) of the skid marks. The drag factor of Road Surface A is 0.75. The graph shows the speed of the van on Road Surface B. Compare the speeds by finding and interpreting their average rates of change over the interval d = 0 to d = 15.

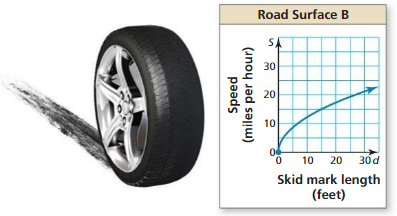
Answer:

Question 46.
COMPARING FUNCTIONS
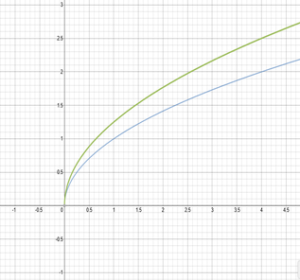
The velocity v (in meters per second) of an object in motion is given by v(E ) = \(\sqrt{\frac{2 E}{m}}\), where E is the kinetic energy of the object (in joules) and m is the mass of the object (in kilograms). The mass of Object A is 4 kilograms. The graph shows the velocity of Object B. Compare the velocities of the objects by finding and interpreting the average rates of change over the interval E = 0 to E = 6.

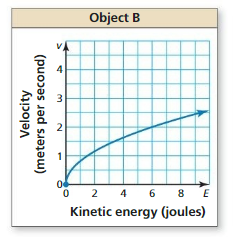
Answer:
To calculate the average rates of change, use points whose E-coordinates are 0 and 6.
Object A: Evaluate S when E = 0 and E = 6
ν(0) = √2(0)/4 = 0
ν(6) = √2(6)/4 = 3
The average rate of change is
s(6) – s(0)/6 – 0 = 0.28
B.
Use the graph to estimate.
(0, 0), (6, 2)
s(6) – s(0)/6 – 0 = (2 – 0)/(6 – 0) = 0.33
From 0 to 6 joules, the velocity of object A increases at an average rate of 0.28 m/s
and the velocity of object B increases at an average rate of 0.33 m/s

Question 47.
OPEN-ENDED
Consider the graph of y = \(\sqrt{x}\).
a. Write a function that is a vertical translation of the graph of y = \(\sqrt{x}\).
b. Write a function that is a reflection of the graph of y = \(\sqrt{x}\).
Answer:

Question 48.
REASONING
Can the domain of a square root function include negative numbers? Can the range include negative numbers? Explain your reasoning.
Answer:
Can the domain of a square root function include negative numbers?
Yes, if the domain includes x < 0
An example would be: y = √-x
where the domain is x ≤ 0
Can the range include negative numbers?
Yes, if the range includes y < 0
An example would be: y = -√x
where the range is y ≤ 0

Question 49.
PROBLEM SOLVING
The nozzle pressure of a fire hose allows firefighters to control the amount of water they spray on a fire. The flow rate f(in gallons per minute) can be modeled by the function f = 12\(\sqrt{p}\), where p is the nozzle pressure (in pounds per square inch).

a. Use a graphing calculator to graph the function. At what pressure does the flow rate exceed 300 gallons per minute?
b. What happens to the average rate of change of the flow rate as the pressure increases?
Answer:

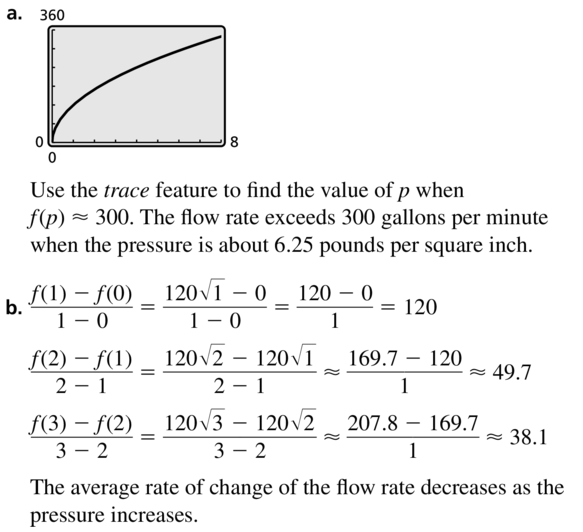
Question 50
PROBLEM SOLVING
The speed s (in meters per second) of a long jumper before jumping can be modeled by the function s = 10.9\(\sqrt{h}\), where h is the maximum height (in meters from the ground) of the jumper.

a. Use a graphing calculator to graph the function. A jumper is running 9.2 meters per second. Estimate the maximum height of the jumper.

Answer:
A jumper is running 9.2 meters per second.

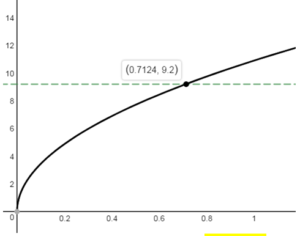
The maximum height of the jumper is approximately 0.71 meters from the ground.

b. Suppose the runway and pit are raised on a platform slightly higher than the ground. How would the graph of the function be transformed?
Answer: The graph of the function will be vertically translated.

Question 51.
MATHEMATICAL CONNECTIONS
The radius r of a circle is given by r = \(\sqrt{\frac{A}{\pi}}\), where A is the area of the circle.

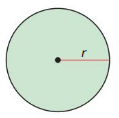
a. Describe the domain of the function. Use a graphing calculator to graph the function.
b. Use the trace feature to approximate the area of a circle with a radius of 5.4 inches.
Answer:

Question 52.
REASONING
Consider the function f(x) = 8a\(\sqrt{x}\).
a. For what value of a will the graph of f be identical to the graph of the parent square root function?
Answer:
The parent function is y = \(\sqrt{x}\)
8a = 1
a = 1/8

b. For what values of a will the graph of f be a vertical stretch of the graph of the parent square root function?
Answer:
A vertical stretch y = k \(\sqrt{x}\)
|k| > 1
8a > 1
a > 1/8

c. For what values of a will the graph of f be a vertical shrink and a reflection of the graph of the parent square root function?
Answer:
A reflection will be y = -k \(\sqrt{x}\) a vertocal shrink y = -k \(\sqrt{x}\)
will occur if 0 < k < 1
0 < -8a < 1
-1/8 < a < 0

Question 53.
REASONING
The graph represents the function f(x) = \(\sqrt{x}\).

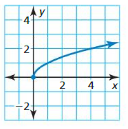
a. What is the minimum value of the function?
b. Does the function have a maximum value? Explain.
c. Write a square root function that has a maximum value. Does the function have a minimum value? Explain.
d. Write a square root function that has a minimum value of -4.
Answer:

Question 54.
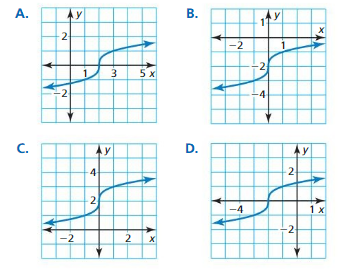
HOW DO YOU SEE IT?
Match each function with its graph. Explain your reasoning.

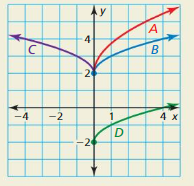
Answer:
A. A function is a vertical translation of the parent function 2 units upward so the corresponding graph is graph B
B. The function is a vertical translation of 4 units downwards of the function f(x)so the corresponding graph is graph D
C. The function is a reflection about the y-axis of the function f(x) so the corresponding graph is graph C.
D. The function is a horizontal shrink of the function f(x) so the corresponding graph is graph A.

Question 55.
REASONING
Without graphing, determine which function’s graph rises more steeply, f(x) = 5\(\sqrt{x}\) or g(x) = \(\sqrt{5x}\). Explain your reasoning.
Answer:

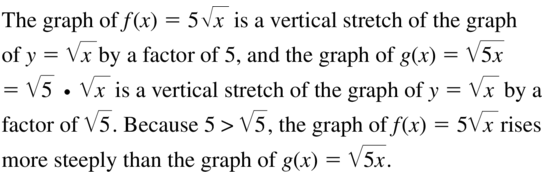
Question 56.
THOUGHT PROVOKING
Use a graphical approach to find the solutions of x – 1 = \(\sqrt{5x-9}\). Show your work. Verify your solutions algebraically.
Answer:
Graph both sides as the related functions:
y1 = x – 1
y2 = \(\sqrt{5x-9}\)
y1 has a slope of 1 and y-intercept of -1
y2 has a domain of x ≥ 1.8 so use point plotting

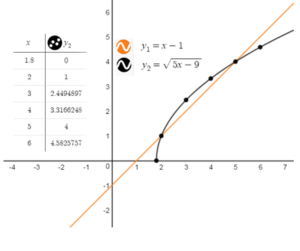
Their point of intersection will be the solution
(2, 1) and (5, 4)
Squaring on both sides
x² – 2x + 1 = 5x – 9
x² – 7x + 1 = – 9
x² – 7x + 10 = 0
(x – 5) (x – 2) = 0
x = 2, 5
Solve for corresponding y-coordinates
x = 2 → y = 2 – 1 = 1
x = 5 → y = 5 – 1 = 4
The solutions are
(2, 1) and (5, 4)

Question 57.
OPEN-ENDED
Write a radical function that has a domain of all real numbers greater than or equal to -5 and a range of all real numbers less than or equal to 3.
Answer:

Maintaining Mathematical Proficiency

Evaluate the expression.(Section 6.2)
Question 58.
\(\sqrt [ 3]{ 343 }\)
Answer:
\(\sqrt [ 3]{ 343 }\) = 7

Question 59.
\(\sqrt [ 3]{ -64 }\)
Answer:

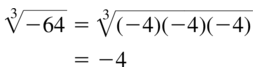
Question 60.
\(-\sqrt[3]{-\frac{1}{27}}\)
Answer:
\(-\sqrt[3]{-\frac{1}{27}}\)
= \(-\sqrt[3]{-\frac{1}{3³}}\)
= -1/3

Factor the polynomial.(Section 7.5)
Question 61.
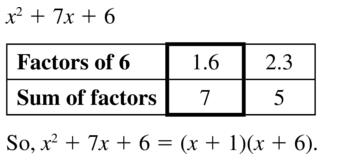
x² + 7x + 6
Answer:

Question 62.
d² – 11d + 28
Answer:
d² – 11d + 28
d² – 7d – 4d + 28
(d – 7) (d – 4) are the factors
d = 7, 4

Question 63.
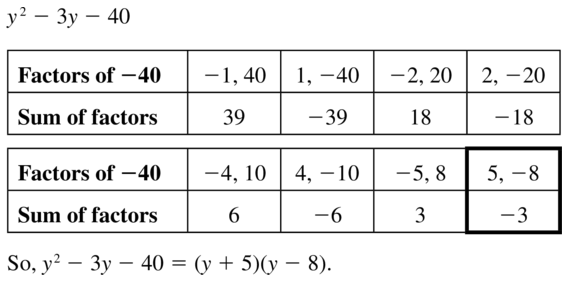
y² – 3y – 40
Answer:

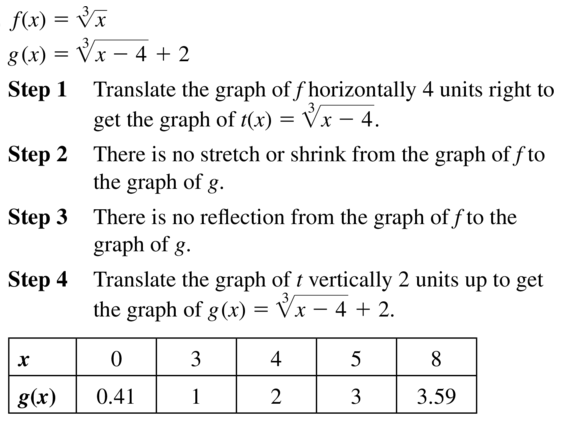
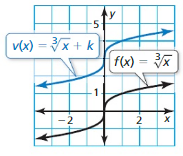
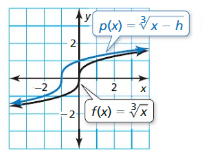
Lesson 10.2 Graphing Cube Roots Functions

Essential Question What are some of the characteristics of the graph of a cube root function?

EXPLORATION 1

Graphing Cube Root Functions
Work with a partner.

•  Make a table of values for each function. Use positive and negative values of x.
• Use the table to sketch the graph of each function.• Describe the domain of each function.
• Describe the range of each function.

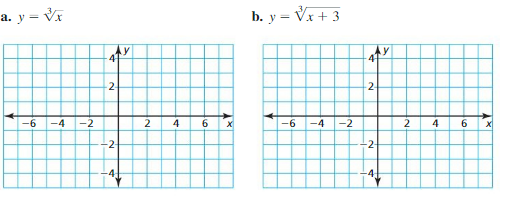
Answer:
a.

The domain and range from the graph
The domain of y is (-∞, ∞)
The range of y is (-∞, ∞)
b.

The domain and range from the graph
The domain of y is (-∞, ∞)
The range of y is (-∞, ∞)

EXPLORATION 2

Writing Cube Root Functions
Work with a partner. Write a cube root function, y = f(x), that has the given values. Then use the function to complete the table.

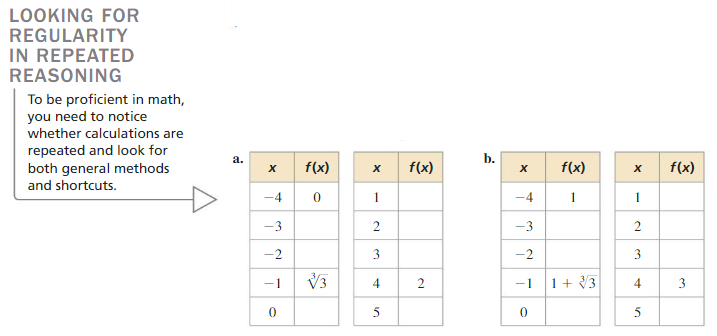
Answer:

Communicate Your Answer

Question 3.
What are some of the characteristics of the graph of a cube root function?
Answer:
I)One to three roots.
ii)Two or zero extrema.
iii)One inflection point.
iv)Point symmetry about the inflection point.
v)Range is the set of real numbers.
vi)Three fundamental shapes.
vii)Four points or pieces of information are required to define a cubic polynomial function.
Roots are solvable by radicals.

Question 4.
Graph each function. Then compare the graph to the graph of f (x) = \(\sqrt [ 3]{ x }\) .
a. g(x) = \(\sqrt [ 3]{ x-1 }\)
b. g(x) = \(\sqrt [ 3]{ x-1 }\)
c. g(x) = 2 \(\sqrt [ 3]{ x }\)
d. g(x) = -2\(\sqrt [ 3]{ x }\)
Answer:

Monitoring Progress

Graph the function. Compare the graph to the graph of f(x) = \(\sqrt [ 3]{ x }\).
Question 1.
h(x) = \(\sqrt [ 3]{ x }\) + 3
Answer:

Question 2.
m(x) = \(\sqrt [ 3]{ x }\) – 5
Answer:

Question 3.
g(x) = 4\(\sqrt [ 3]{ x }\)
Answer:

Graph the function. Compare the graph to the graph of f(x) = \(\sqrt [ 3]{ x }\).
Question 4.
g(x) =\(\sqrt [3]{ 0.5x+5 }\) + 5
Answer:

Question 5.
h(x) = 4\(\sqrt [3]{ x }\) – 1
Answer:

Question 6.
n(x) = \(\sqrt [ 3]{ 4-x }\)
Answer:

Question 7.
Let g(x) = \(-\frac{1}{2} \sqrt[3]{x+2}\) – 4. Describe the transformations from the graph of f (x) = \(\sqrt [ 3]{ x }\) to the graph of g. Then graph g.
Answer:

Question 8.
In Example 4, compare the average rates of change over the interval x = 2 to x = 10.
Answer:

Question 9.
WHAT IF?
Estimate the age of an elephant whose shoulder height is 175 centimeters.
Answer:
h(t) = 62.5\(\sqrt [ 3]{ x }\) + 75.8
h(x) and y = 200

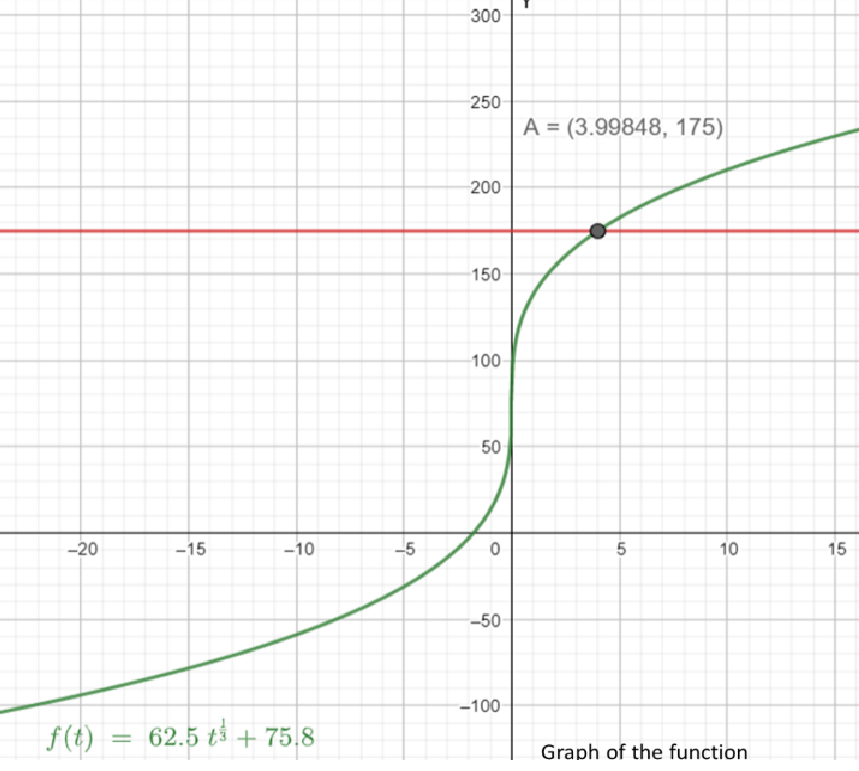
It can be seen from the graph that age of elephant of height 175 cm is 4 years.

Graphing Cube Roots Functions 10.2 Exercises

Vocabulary and Core Concept Check

Question 1.
COMPLETE THE SENTENCE
The __________ of the radical in a cube root function is 3.
Answer:
The index of the radical in a cube root function is 3.

Question 2.
WRITING
Describe the domain and range of the function f(x) = \(\sqrt [3]{ x-4 }\) + 1.
Answer:
Regardless of the transformation, the domain and range of a cubic function are all real numbers.

Monitoring Progress and Modeling with Mathematics

In Exercises 3–6, match the function with its graph.
Question 3.
y = 3\(\sqrt [3]{ x+2 }\)
Answer:

Question 4.
y = 3\(\sqrt [3]{ x-2 }\)
Answer:
The given function is the parent function
y = \(\sqrt [3]{ x }\) that is horizontally shifted 2 units to the right.
Thus the answer is graph A.

Question 5.
y = 3\(\sqrt [3]{ x+2 }\)
Answer:

Question 6.
y = \(\sqrt [3]{ x }\) – 2
Answer:
The given function is the parent function
y = \(\sqrt [3]{ x }\) that is vertically shifted 2 units to the downward.
Thus the answer is graph B

In Exercises 7–12, graph the function. Compare the graph to the graph of f(x) = \(\sqrt [ 3]{ x }\).
Question 7.
h(x) = \(\sqrt [3]{ x-4 }\)
Answer:

Question 8.
g(x) = \(\sqrt [3]{ x+1 }\)
Answer:
1. Make a table of values
2. Plot the ordered pairs
3. Draw a smooth curve through the points.

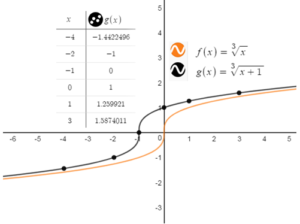
The graph of g(x) is the graph of f(x) that is horizontally shifted 1 unit to the left.

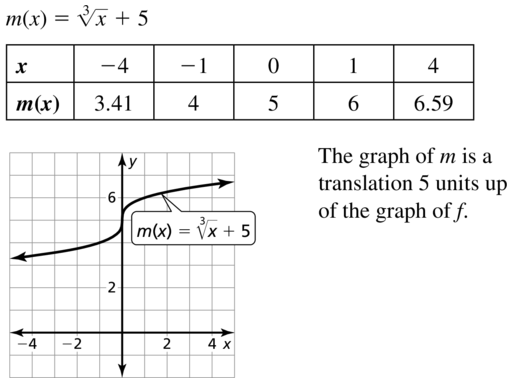
Question 9.
m(x) = \(\sqrt [3]{ x+5 }\)
Answer:

Question 10.
q(x) = \(\sqrt [3]{ x }\) – 3
Answer:
1. Make a table of values
2. Plot the ordered pairs
3. Draw a smooth curve through the points.

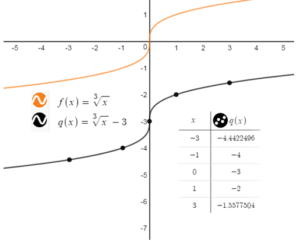
The graph of g(x) is the graph of f(x) that is vertically shifted 3 units downwards.

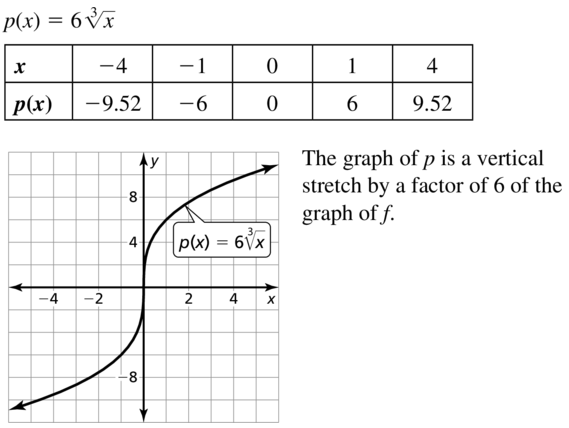
Question 11.
p(x) = 6\(\sqrt [3]{ x }\)
Answer:

Question 12.
j(x) = \(\sqrt[3]{\frac{1}{2} x}\)
Answer:
1. Make a table of values
2. Plot the ordered pairs
3. Draw a smooth curve through the points.

The graph of g(x) is the graph of f(x) that is horizontally stretched by a factor of 2.

In Exercises 13–16, compare the graphs. Find the value of h, k, or a.
Question 13.

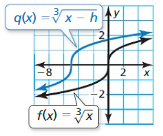
Answer:

Question 14.

Answer:
The graph of g(x) is the graph of f(x) is
– Vertically shifted 4 units upwards
g(x) = \(\sqrt [3]{ x }\) + 4
Therefore k = 4

Question 15.

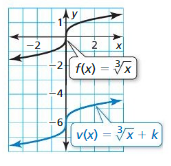
Answer:

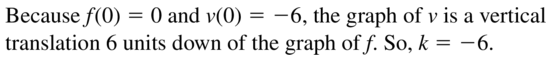
Question 16.

Answer:
The graph of p(x) is the graph of f(x) is vertically stretched by a factor of 3.
p(x) = 3 \(\sqrt [3]{ x }\)
Therefore a = 3

In Exercises 17–26, graph the function. Compare the graph to the graph of f(x) = \(\sqrt [3]{ x }\).
Question 17.
r(x) = – \(\sqrt [3]{ x-2 }\)
Answer:

Question 18.
h(x) = – 3\(\sqrt [3]{ x+3 }\)
Answer:
1. Make a table of values
2. Plot the ordered pairs
3. Draw a smooth curve through the points.

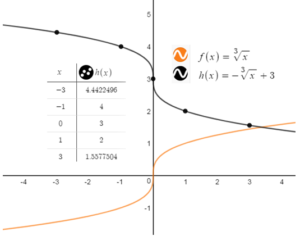
The graph of h(x) is the graph of f(x)
– Reflected in the x-axis
– Vertically shifted 3 units upward

Question 19.
k(x) = 5\(\sqrt [3]{ x+1 }\)
Answer:

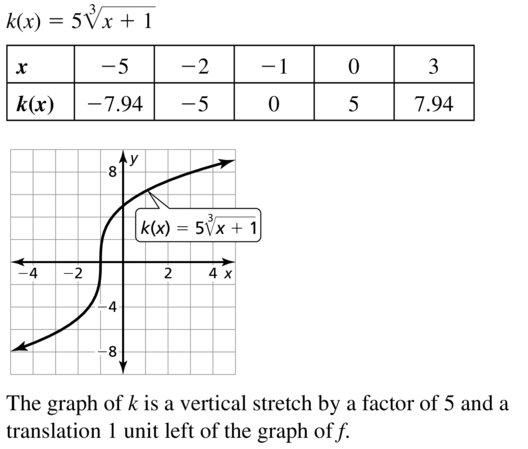
Question 20.
j(x) = 0.5\(\sqrt [3]{ x-4 }\)
Answer:
1. Make a table of values
2. Plot the ordered pairs
3. Draw a smooth curve through the points.

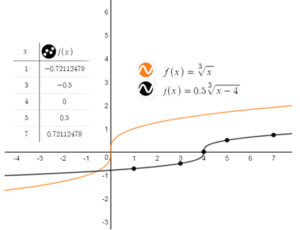
The graph of j(x) is the graph of f(x) is
– Vertically shrinked by a factor of 0.5
– Horizontally shifted 4 units to the right.

Question 21.
g(x) = 4\(\sqrt [3]{ x }\) – 3
Answer:

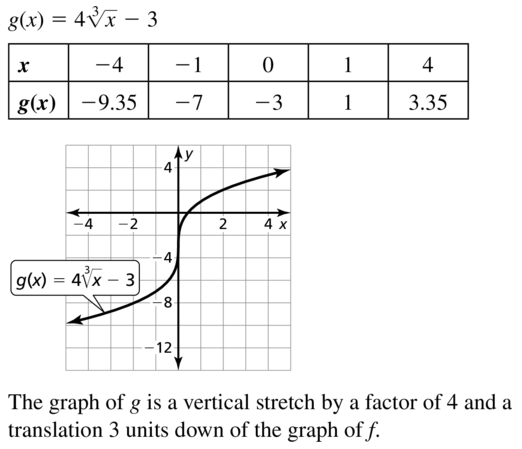
Question 22.
m(x) = 3\(\sqrt [3]{ x }\) + 7
Answer:
1. Make a table of values
2. Plot the ordered pairs
3. Draw a smooth curve through the points.

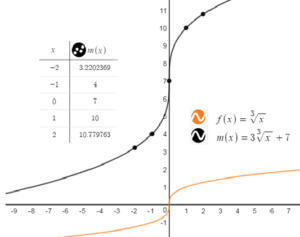
The graph of m(x) is the graph of f(x) is
– Vertically stretched by a factor of 3
– Vertically shifted 7 units upward

Question 23.
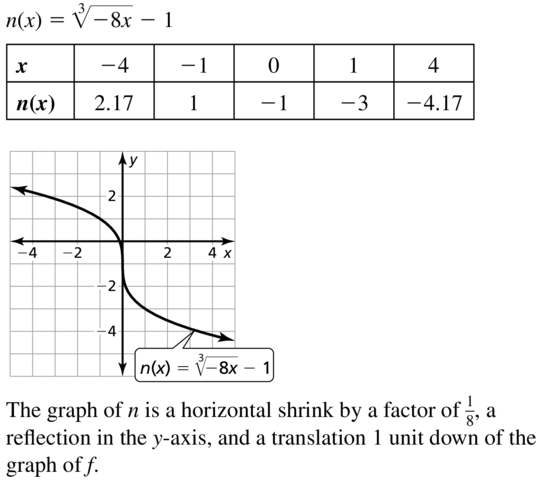
n(x) = \(\sqrt [3]{ -8x }\) – 1
Answer:

Question 24.
v(x) =\(\sqrt [3]{ 5x }\) + 2
Answer:
1. Make a table of values
2. Plot the ordered pairs
3. Draw a smooth curve through the points.

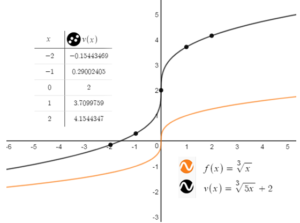
The graph of v(x) is the graph of f(x) is
– Horizontally shrinked by a factor of 1/5
– Vertically shifted 2 units upward

Question 25.
q(x) = \(\sqrt[3]{2(x+3)}\)
Answer:

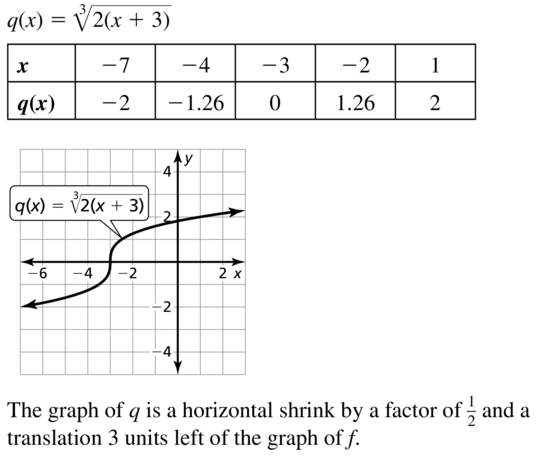
Question 26.
p(x) = \(\sqrt[3]{3(1-x)}\)
Answer:
p(x) = \(\sqrt[3]{3(1-x)}\)
1. Make a table of values
2. Plot the ordered pairs
3. Draw a smooth curve through the points.

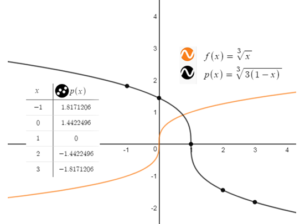
The graph of p(x) is the graph of f(x) is
– Horizontally shrinked by a factor of 1/3
– Reflected in the y-axis
– Horizontally shifted 1 unit to the right

In Exercises 27–32, describe the transformations from the graph of f(x) = \(\sqrt [3]{ x }\) to the graph of the given function. Then graph the given function.
Question 27.
g(x) = \(\sqrt [3]{ x-4 }\) + 2
Answer:

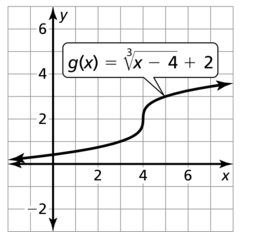
Question 28.
n(x) = \(\sqrt [3]{ x+1 }\) – 3
Answer:
The graph of k(x) is the graph of f(x) is
– Horizontally shifted 1 units to the left → \(\sqrt [3]{ x+1 }\)
– Vertically shifted 3 units downward → \(\sqrt [3]{ x+1 }\) – 3
1. Make a table of values
2. Plot the ordered pairs
3. Draw a smooth curve through the points.

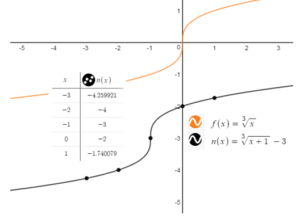
Question 29.
j(x) = -5\(\sqrt [3]{ x+3 }\) + 2
Answer:

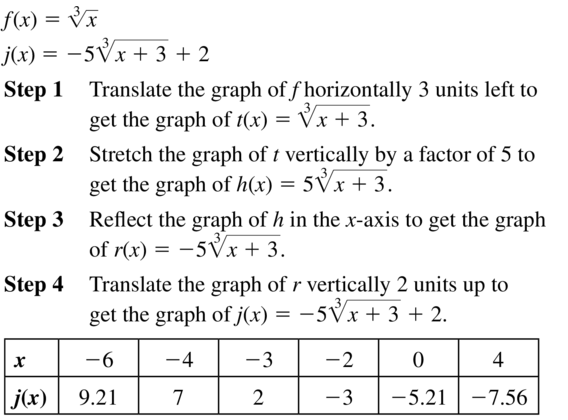
Question 30.
k(x) = 6\(\sqrt [3]{ x-9 }\) – 5
Answer:
The graph of k(x) is the graph of f(x) is
– Vertically stretched by a factor of 6 → 6\(\sqrt [3]{ x }\)
– Horizontally shifted 9 units to the right → 6\(\sqrt [3]{ x-9 }\)
– Vertically shifted 3 units downward → 6\(\sqrt [3]{ x-9 }\) – 5
1. Make a table of values
2. Plot the ordered pairs
3. Draw a smooth curve through the points.

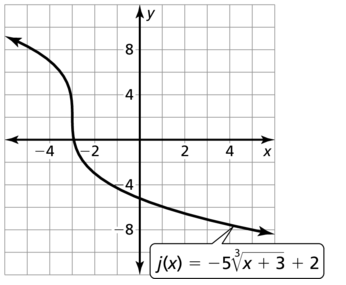
Question 31.
v(x) = \(\frac{1}{3} \sqrt[3]{x-1}\) + 7
Answer:

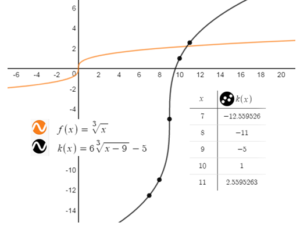
Question 32.
h(x) = -\frac{3}{2} \sqrt[3]{x+4} – 3
Answer:
The graph of v(x) is the graph of f(x) is
– Reflected in the x-axis → \(\sqrt [3]{ x }\)
– Vertically stretched by a factor of 3/2 → -3/2\(\sqrt [3]{ x }\)
– Horizontally shifted 4 units to the left → -\frac{3}{2} \sqrt[3]{x+4}
– Vertically shifted 3 units downward → -\frac{3}{2} \sqrt[3]{x+4} – 3
1. Make a table of values
2. Plot the ordered pairs
3. Draw a smooth curve through the points.

Question 33.
ERROR ANALYSIS
Describe and correct the error in graphing the function f(x) = \(\sqrt [3]{ x-3 }\).

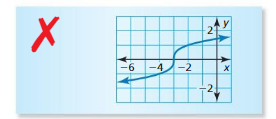
Answer:

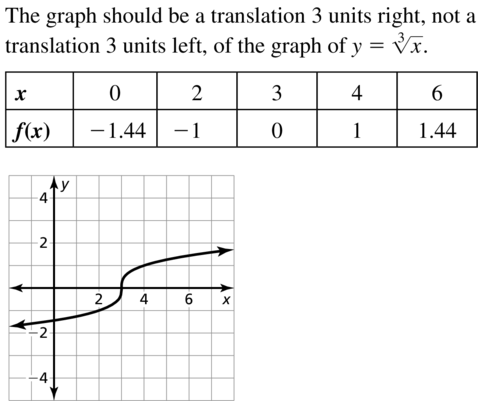
Question 34.
ERROR ANALYSIS
Describe and correct the error in graphing the function h(x) = \(\sqrt [3]{ x }\) + 1.

Answer:
The graph that is translated is the square root function when it should be the cubic function since the index is 3.

Question 35.
COMPARING FUNCTIONS
The graph of cube root function q is shown. Compare the average rate of change of q to the average rate of change of f(x) = 3\(\sqrt [3]{ x }\) over the interval x = 0 to x = 6.

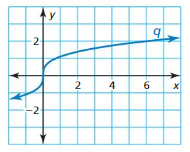
Answer:

Question 36.
COMPARING FUNCTIONS
The graphs of two cube root functions are shown. Compare the average rates of change of the two functions over the interval x = -2 to x = 2.

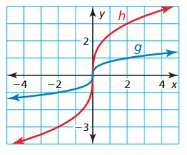
Answer:
For g(x): Use the graph to estimate
Use (-2, -1) and (2, 1)
The average rate of change is
g(2) – g(-2)/2 – (-2) = 0.5
For h(x): Use the graph to estimate
Use (-2, -3) and (2, 3)
h(3) – h(-3)/2 – (-2) = 1.5
The average rate of change of h is 1.5/0.5 = 3.
Thus 3 time greater than the average rate of change of g over the interval x = -2 to x = 2

Question 37.
MODELING WITH MATHEMATICS
For a drag race car that weighs 1600 kilograms, the velocity v (in kilometers per hour) reached by the end of a drag race can be modeled by the function v = 23.8\(\sqrt [3]{ p }\), where p is the car’s power (in horsepower). Use a graphing calculator to graph the function. Estimate the power of a 1600-kilogram car that reaches a velocity of 220 kilometers per hour.
Answer:

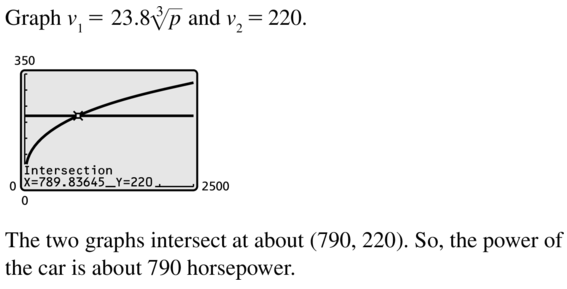
Question 38.
MODELING WITH MATHEMATICS
The radius r of a sphere is given by the function r = \(\sqrt[3]{\frac{3}{4 \pi}} V\), where V is the volume of the sphere. Use a graphing calculator to graph the function. Estimate the volume of a spherical beach ball with a radius of 13 inches.
Answer:
Using a graphing utility, trace where the  y-value is 13

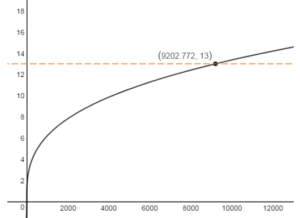
Therefore the volume of a sphere with a radius of 13 inches is approximately 9202.77 cu. in

Question 39.
MAKING AN ARGUMENT
Your friend says that all cube root functions are odd functions. Is your friend correct? Explain.
Answer:

Question 40.
HOW DO YOU SEE IT?
The graph represents the cube root function f(x) = \(\sqrt [3]{ x }\).

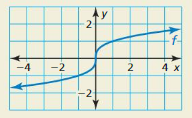
a. On what interval is f negative? positive?
Answer:
Negative on x ≤ 0
Positive on x ≥ 0
b. On what interval, if any, is f decreasing? increasing?
Answer:
Decreasing: None
Increasing: -∞ < x < ∞
c. Does f have a maximum or minimum value? Explain.
Answer:
The graph has neither maximum nor minimum since it is always increasing based on b.
d. Find the average rate of change of f over the interval x = -1 to x = 1.
Answer:
The average rate of change is
f(1) – f(-1)/1 – (-1)
= 1 – (-1)/1 – (-1) = 1

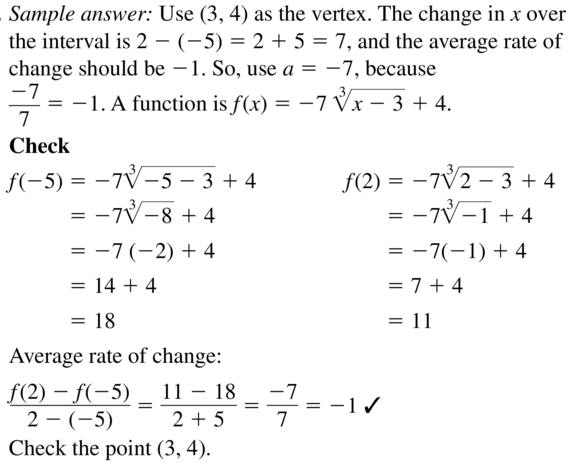
Question 41.
PROBLEM SOLVING
Write a cube root function that passes through the point (3, 4) and has an average rate of change of -1 over the interval x = -5 to x = 2.
Answer:

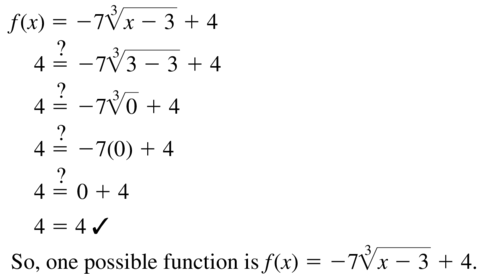
Question 42.
THOUGHT PROVOKING
Write the cube root function represented by the graph. Use a graphing calculator to check your answer.

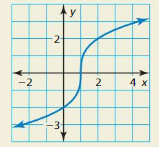
Answer:
Imagine that the coordinate axis is on the center of the cubic function.
This is a vertical stretch by a factor of 2
y = 2 \(\sqrt [3]{ x }\)
Also, from the original graph, it is a translation of 1 unit to the right so tthe function will be
y = 2 \(\sqrt [3]{ x-1 }\)

Maintaining Mathematical Proficiency

Factor the polynomial.(Section 7.6)
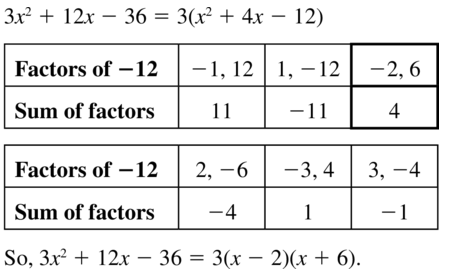
Question 43.
3x² + 12x – 36
Answer:

Question 44.
2x² – 11x + 9
Answer:
2x² – 11x + 9
(2x – 9)(x – 1) factor thr polynomial

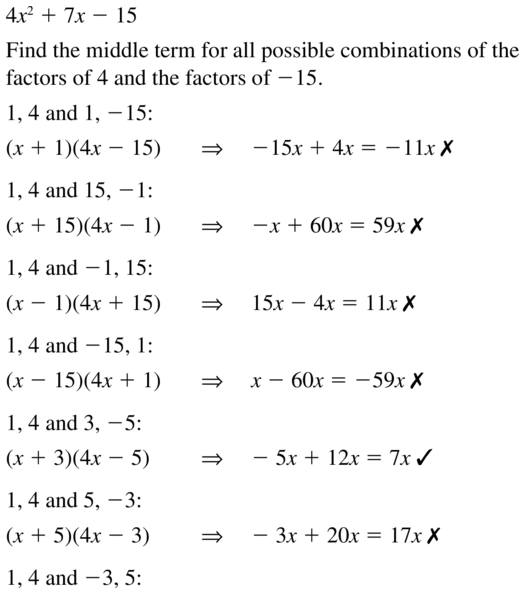
Question 45.
4x² + 7x – 15
Answer:

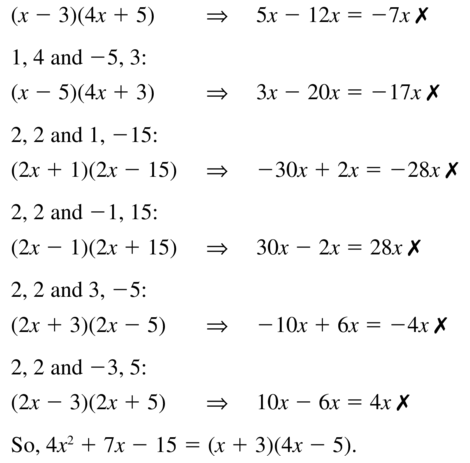
Solve the equation using square roots.(Section 9.3)
Question 46.
x² – 36 = 0
Answer:
x² – 36 = 0
x² = 36
squaring on both sides
x = 6 or x = -6

Question 47.
5x² + 20 = 0
Answer:

Question 48.
(x + 4)² – 81
Answer:
(x + 4)² – 81
(x + 4)²  = 81
squaring on both sides
x + 4 = √81
x + 4 = 9 or x + 4 = -9
x = 9 – 4 or x = -9 – 4
x = 5 or x = -13

Question 49.
25(x – 2)² = 9
Answer:

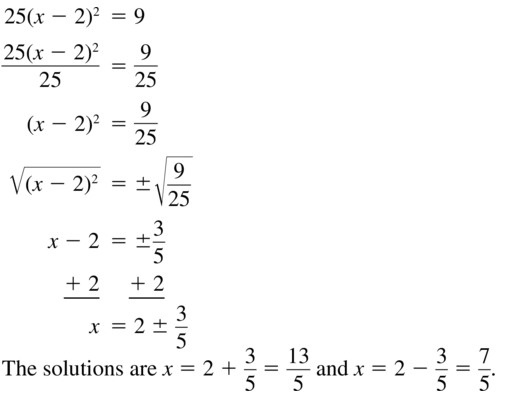
Radical Functions and Equations Study Skills: Making Note Cards

10.1–10.2 What Did YouLearn?

Core Vocabulary
square root function, p. 544
radical function, p. 545
cube root function, p. 552

Core Concepts
Lesson 10.1
Square Root Functions, p. 544
Transformations of Square Root Functions, p. 545
Comparing Square Root Functions Using Average Rates of Change, p. 546

Lesson 10.2
Cube Root Functions, p. 552
Comparing Cube Root Functions
Using Average Rates of Change, p. 554

Mathematical Practices

Question 1.
In Exercise 45 on page 549, what information are you given? What relationships are present? What is your goal?
Answer:

Question 2.
What units of measure did you use in your answer to Exercise 38 on page 556? Explain your reasoning.
Answer:

Study Skills: Making Note Cards

Invest in three different colors of note cards. Use one color for each of the following: vocabulary words, rules, and calculator keystrokes.

• Using the first color of note cards, write a vocabulary word on one side of a card. On the other side, write the definition and an example. If possible, put the definition in your own words.
• Using the second color of note cards, write a rule on one side of a card. On the other side, write an explanation and an example.
• Using the third color of note cards, write a calculation on one side of a card. On the other side, write the keystrokes required to perform the calculation.
  Use the note cards as references while completing your homework. Quiz yourself once a day.
  

Radical Functions and Equations 10.1–10.2 Quiz

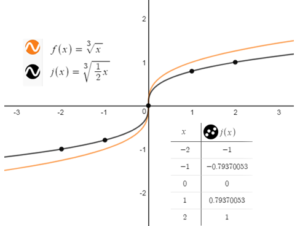
Describe the domain of the function.(Lesson 10.1)
Question 1.
y = \(\sqrt{x-3}\)
Answer:
The domain is determined by the radicand which must be greater than or equal to 0
x – 3 ≥ 0
x ≥ 3

Question 2.
f(x) = 15\(\sqrt{x}\)
Answer:
The domain is determined by the radicand which must be greater than or equal to 0
x ≥ 0

Question 3.
y = \(\sqrt{3-x}\)
Answer:
The domain is determined by the radicand which must be greater than or equal to 0
3 – x ≥ 0
– x ≥ -3
x ≤ 3

Graph the function. Describe the range. Compare the graph to the graph of f(x) = \(\sqrt{x}\). (Lesson 10.1)
Question 4.
g(x) = \(\sqrt{x}\) + 5
Answer:
Step 1: Use the domain to make a table of values.
The radicand must be greater than or equal to 0
x ≥ 0
Step 2: Plot the ordered pairs
Step 3: Draw a smooth curve through the points starting at (0, 5)

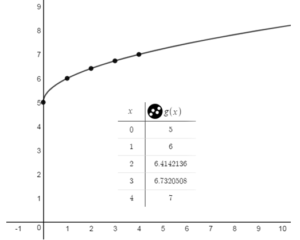
From the graph, we can see that the range is
y ≥ 5
The graph of g(x) is the graph of f(x) is vertically shifted 5 units upward

Question 5.
n(x) = \(\sqrt{x-4}\)
Answer:
Step 1: Use the domain to make a table of values.
The radicand must be greater than or equal to 0
x – 4 ≥ 0
x ≥ 4
Step 2: Plot the ordered pairs
Step 3: Draw a smooth curve through the points starting at (4, 0)

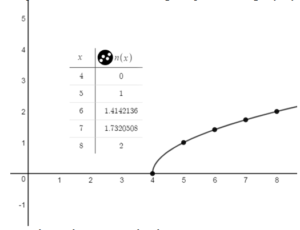
From the graph, we can see that the range is
y ≥ 0
The graph of n(x) is the graph of f(x) is horizontally shifted 4 units to the right.

Question 6.
r(x) = –\(\sqrt{x-1}\) + 1
Answer:
Step 1: Use the domain to make a table of values.
The radicand must be greater than or equal to 0
x – 2 ≥ 0
x ≥ 2
Step 2: Plot the ordered pairs
Step 3: Draw a smooth curve through the points starting at (2, 1)

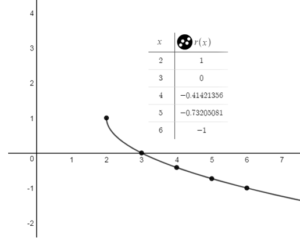
The graph of r(x) is the graph of f(x) is
– Reflected in the x-axis
– Horizontally shifted 2 units to the right
– Vertically shifted 1 unit upward

Graph the function. Compare the graph to the graph of f(x) = \(\sqrt [3]{ x }\). (Lesson 10.2)
Question 7.
b(x) = \(\sqrt [3]{ x+2 }\)
Answer:
Step 1: Make a table of values
Step 2: Plot the ordered pairs
Step 3: Draw a smooth curve through the points

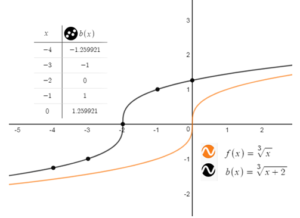
The graph of b(x) is the graph of f(x) is horizontally shifted 2 units to the left.

Question 8.
h(x) = -3\(\sqrt [3]{ x-6 }\)
Answer:
Step 1: Make a table of values
Step 2: Plot the ordered pairs
Step 3: Draw a smooth curve through the points

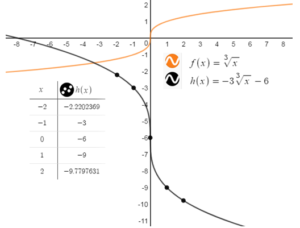
The graph of h(x) is the graph of f(x) is
– Reflected in the x-axis
– Vertically stretched by a factor of 3
– Vertically shifted 6 units downward

Question 9.
q(x) = \(\sqrt [3]{ -4-x }\)
Answer:
Step 1: Make a table of values
Step 2: Plot the ordered pairs
Step 3: Draw a smooth curve through the points

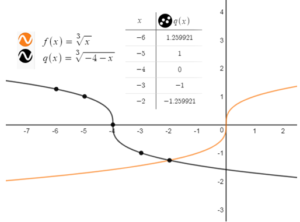
The graph of q(x) is the graph of f(x) is
– Reflected in the y-axis
– Horizontally shifted 4 units to the left

Compare the graphs. Find the value of h, k, or a. (Lesson 10.1 and Lesson 10.2)
Question 10.

Answer:
The graph of v(x) is the graph of f(x) is
– Vertically shifted 3 units upwards
v(x) = \(\sqrt [3]{ x }\) + 3
Thus k = 3

Question 11.

Answer:
The graph of g(x) is the graph of f(x) is
– Reflected in the x-axis
– Vertically stretched by a factor of 2
v(x) = -2√x
Thus a = -2

Question 12.

Answer:
The graph of p(x) is the graph of f(x) is
– Horizontally shifted 1 unit to the left
q(x) = \(\sqrt [3]{ x + 1 }\)
Thus h = -1

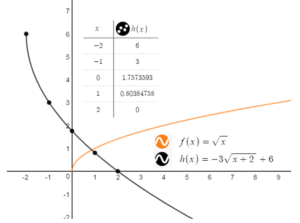
Describe the transformations from the graph of f to the graph of h. Then graph h. (Section 10.1 and Section 10.2)
Question 13.
f(x) = \(\sqrt{x}\); h(x) = -3 \(\sqrt{x+2}\) + 6
Answer:
The graph of h(x) is the graph of f(x) is
– Reflected in the x-axis → \(\sqrt{x}\)
– Vertically stretched by a factor of 3 → -3\(\sqrt{x}\)
– Horizontally shifted 2 units to the left → -3 \(\sqrt{x+2}\)
– Vertically shifted 6 units upward → -3 \(\sqrt{x+2}\) + 6
Step 1: Use the domain to make a table of values
x + 2 ≥ 0
x ≥ -2
Step 2: Plot the ordered pairs
Step 3: Draw a smooth curve through the points starting at (-2, 6)

Question 14.
f(x) = \(\sqrt[3]{x}\); h(x) = \(\frac{1}{2} \sqrt[3]{x}-3\)
Answer:
The graph of h(x) is the graph of f(x) is
– Vertically shrinked by a factor of \(\frac{1}{2}\) → \(\frac{1}{2}\) \(\sqrt[3]{x}\)
– Vertically shifted 3 units downward → \(\frac{1}{2}\) \(\sqrt[3]{x}\) – 3
Step 1: Make a table of values.
Step 2: Plot the ordered pairs.
Step 3: Draw a smooth curve through the points.

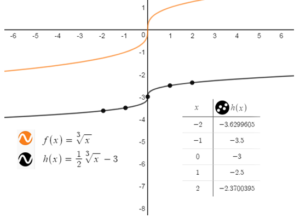
Question 15.
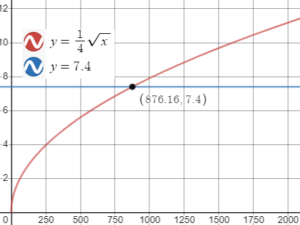
The time t (in seconds) it takes a dropped object to fall h feet is given by t = \(\frac{1}{4} \sqrt{h}\). (Section 10.1)

a. Use a graphing calculator to graph the function. Describe the domain and range.
b. It takes about 7.4 seconds for a stone dropped from the New River Gorge Bridge in West Virginia to reach the water below. About how high is the bridge above the New River?
Answer:
Using a graphing utility, the domain is h ≥ 0 and the range is t ≥ 0.
Graph the function as y = \(\frac{1}{4} \sqrt{h}\) and the line y = 7.4 corresponding to a time of 7.4 seconds.
Use the intersect feature to obtain (876.16, 7.4)
Hence the bridge is about 876 feet high.

Question 16.
The radius r of a sphere is given by the function r = \(\sqrt[3]{\frac{3}{4 \pi} V}\), where V is the volume of the sphere. Spaceship Earth is a spherical structure at Walt Disney World that has an inner radius of about 25 meters. Use a graphing calculator to graph the function. Estimate the volume of Spaceship Earth. (Section 10.2)
Answer:

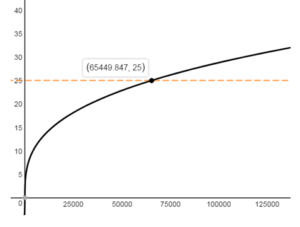
Thus the volume of the spaceship Earth with an inner radius of 25 meters is approximately 65449.85 cu. meters

Question 17.
The graph of square root function g is shown. Compare the average rate of change of g to the average rate of change of h(x) = \(\sqrt[3]{\frac{3}{2} x}\)x over the interval x = 0 to x = 3.

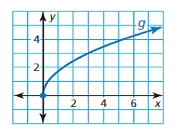
Answer:
For g(x): Use the graph to estimate.
Use (0, 0) and (3, 3)
The average rate of change is
g(3) – g(0)/ 3 – 0
= (3 – 0)/(3 -0) = 1
For h(x) = \(\sqrt[3]{\frac{3}{2} x}\)x:
Evaluate f when x = 0 and x = 3
f(0) = \(\sqrt[3]{\frac{3}{2} (0)}\) = 0
f(3) = \(\sqrt[3]{\frac{3}{2} (3)}\) ≈ 1.65
The average rate of change is
f(3) – f(0)/ 3 – 0
= (1.65 – 0)/(3 -0) ≈ 0.55
The average rate of change of g is \(\frac{1}{0.55}\) ≈ 1.8 times greater than the average rate of change of h over the interval x = 0 to x = 3

Lesson 10.3 Solving Radical Equations

Essential Question How can you solve an equation that contains square roots?

EXPLORATION 1

Analyzing a Free-Falling Object
Work with a partner. The table shows the time t (in seconds) that it takes a free-falling object (with no air resistance) to fall d feet.

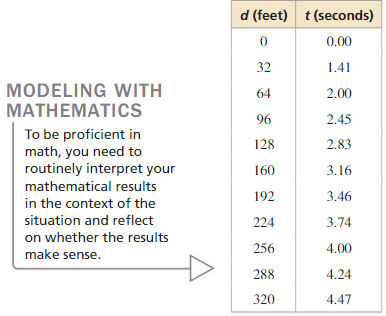
a. Use the data in the table to sketch the graph of t as a function of d. Use the coordinate plane below.
b. Use your graph to estimate the time it takes the object to fall 240 feet.
c. The relationship between d and t is given by the function t = \(\sqrt{\frac{d}{16}}\).
Use this function to check your estimate in part (b).
d. It takes 5 seconds for the object to hit the ground. How far did it fall? Explain your reasoning.

EXPLORATION 2

Solving a Square Root Equation
Work with a partner. The speed s (in feet per second) of the free-falling object in Exploration 1 is given by the functions
s = \(\sqrt{64d}\).
Find the distance the object has fallen when it reaches each speed.
a. s = 8 ft/sec
b. s = 16 ft/sec
c. s = 24 ft/sec

Communicate Your Answer

Question 3.
How can you solve an equation that contains square roots?
Answer:
If an equation has a square root equal to a negative number, that equation will have no solution. To isolate the radical, subtract 1 from both sides.

Question 4.
Use your answer to Question 3 to solve each equation.
a. 5 = \(\sqrt{x}\) + 20
b. 4 = \(\sqrt{x-18}\)
c. \(\sqrt{x}\) + 2 = 3
d. -3 = -2\(\sqrt{x}\)
Answer:

Monitoring Progress

Solve the equation. Check your solution.
Question 1.
\(\sqrt{x}\) = 6
Answer:
Given,
\(\sqrt{x}\) = 6
Squaring on both sides
\(\sqrt{x}\)² = 6²
x = 36
Check:
\(\sqrt{36}\) = 6
Thus x = 6 is the solution

Question 2.
\(\sqrt{x}\) – 7 = 3
Answer:
Given,
\(\sqrt{x}\) – 7 = 3
\(\sqrt{x}\) = 3 + 7
\(\sqrt{x}\) = 10
Squaring on both sides
x = 10²
x = 100
Check:
\(\sqrt{100}\) – 7 = 3
10 – 7 = 3
Thus x = 100 is the solution

Question 3.
\(\sqrt{y}\) + 15 = 22
Answer:
Given,
\(\sqrt{y}\) + 15 = 22
\(\sqrt{y}\) = 22 – 15
\(\sqrt{y}\) = 7
Squaring on both sides
\(\sqrt{y}\)² = 7² = 49
Check:
\(\sqrt{49}\) + 15 = 22
7 + 15 = 22
Thus y = 49 is the solution

Question 4.
1 – \(\sqrt{c}\) = -2
Answer:
Given,
1 – \(\sqrt{c}\) = -2
1 + 2 = \(\sqrt{c}\)
\(\sqrt{c}\) = 3
Squaring on both sides
c = 9
Check:
1 – \(\sqrt{c}\) = -2
1 – \(\sqrt{9}\) = -2
1 – 3 = -2
Thus c = 9 is the solution

Solve the equation. Check your solution.
Question 5.
\(\sqrt{x+4}\) + 7 = 11
Answer:
Given,
\(\sqrt{x+4}\) + 7 = 11
\(\sqrt{x+4}\) = 11 – 7
\(\sqrt{x+4}\) = 4
Squaring on both sides
x + 4 = 4² = 16
x + 4 = 16
x = 16 – 4
x = 12
\(\sqrt{x+4}\) + 7 = 11
\(\sqrt{12+4}\) + 7 = 11
\(\sqrt{16}\) + 7 = 11
4 + 7 = 11
Thus x = 12 is the solution

Question 6.
15 = 6 + \(\sqrt{3w-9}\)
Answer:
Given,
15 = 6 + \(\sqrt{3w-9}\)
15 – 6 = \(\sqrt{3w-9}\)
9 = \(\sqrt{3w-9}\)
Squaring on both sides
81 = 3w – 9
3w = 81 + 9
3w = 90
w = 90/3
w = 30
check:
15 = 6 + \(\sqrt{3(30)-9}\)
15 = 6 + \(\sqrt{81}\)
15 = 6 + 9
15 = 15
Thus w = 30 is the solution

Question 7.
\(\sqrt{3x+1}\) = \(\sqrt{4x-7}\)
Answer:
Given,
\(\sqrt{3x+1}\) = \(\sqrt{4x-7}\)
Squaring on both sides
3x + 1 = 4x – 7
3x – 4x = -7 – 1
-x = -8
x = 8

Question 8.
\(\sqrt{n}\) = \(\sqrt{5n-1}\)
Answer:
Given,
\(\sqrt{n}\) = \(\sqrt{5n-1}\)
Squaring on both sides
n = 5n – 1
5n – n = 1
4n = 1
n = 1/4

Question 9.
\(\sqrt [3]{ y }\) = 4 = 1
Answer:
Given,
\(\sqrt [3]{ y }\) = 4 – 1
\(\sqrt [3]{ y }\) = 3
cubing on both sides
y = 3³ = 27
y = 27

Question 10.
\(\sqrt [3]{ 3c+7 }\) = 10
Answer:
Given,
\(\sqrt [3]{ 3c+7 }\) = 10
cubing on both sides
3c + 7 = 10³
3c + 7 =  1000
3c = 993
c = 993/3
c = 331

Solve the equation. Check your solution(s).
Question 11.
\(\sqrt{4-3x}\) = x
Answer:
Given,
\(\sqrt{4-3x}\) = x
squaring on both sides
4 – 3x = 1
4 – 1 = 3x
3 = 3x
x = 1 is the solution

Question 12.
\(\sqrt{3m}\) + 10 = 1
Answer:
Given,
\(\sqrt{3m}\) + 10 = 1
\(\sqrt{3m}\) = 1 – 10
\(\sqrt{3m}\) = -9
squaring on both sides
3m = 81
m = 81/3
m = 27

Question 13.
p + 1 = \(\sqrt{7p+15}\)
Answer:
Given,
p + 1 = \(\sqrt{7p+15}\)
squaring on both sides
(p + 1)² = \(\sqrt{7p+15}\)²
p² + 2p + 1 = 7p + 15
p² + 2p + 1 – 7p – 15 = 0
p² -5p -14 = 0
p² -7p + 2p -14 = 0
(p – 7)(p – 2) =
p = 7 or p = 2

Question 14.
What is the length of a pendulum that has a period of 2.5 seconds?
Answer:
A simple pendulum with a period of 1 second will have a length of 0.25 meters or 25 centimeters
2.5 sec = 2.5 × 25 centimeters = 62.5 centimeters

Solving Radical Equations 10.3 Exercises

Vocabulary and Core Concept Check

Question 1.
VOCABULARY
Why should you check every solution of a radical equation?
Answer:
You should check every solution of a radical equation because raising each side of a radical equation to an exponent can introduce extraneous solutions.

Question 2.
WHICH ONE DOESN’T BELONG?
Which equation does not belong with the other three? Explain your reasoning.

Answer:
x \(\sqrt{3}\) – 5 = 4 does not belong because it is not a radical equation.
\(\sqrt{3}\) is constant.

Monitoring Progress and Modeling with Mathematics

In Exercises 3–12, solve the equation. Check your solution.
Question 3.
\(\sqrt{x}\) = 9
Answer:

Question 4.
\(\sqrt{x}\) = 4
Answer:
Given,
\(\sqrt{x}\) = 4
Squaring on both sides
\(\sqrt{x}\)² = 4²
x = 16

Question 5.
7 = \(\sqrt{x}\) – 5
Answer:

Question 6.
\(\sqrt{p}\) – 7 = -1
Answer:
\(\sqrt{p}\) – 7 = -1
Add 7 on both sides
\(\sqrt{p}\) – 7 + 7 = -1 + 7
\(\sqrt{p}\) = 6
p = 6² = 36

Question 7.
\(\sqrt{c}\) + 12 = 23
Answer:

Question 8.
\(\sqrt{x}\) + 6 = 8
Answer:
Given,
\(\sqrt{x}\) + 6 = 8
Subtract 6 on both sides
\(\sqrt{x}\) + 6 – 6= 8 – 6
\(\sqrt{x}\) = 2
Squaring on both sides
\(\sqrt{x}\)² = 2²
= 4

Question 9.
4 – \(\sqrt{x}\) = 2
Answer:

Question 10.
-8 = 7 – \(\sqrt{r}\)
Answer:
Given,
-8 = 7 – \(\sqrt{r}\)
Subtract -7 on both sides
-8 – 7 = 7 – \(\sqrt{r}\) – 7
-15 = – \(\sqrt{r}\)
15 = \(\sqrt{r}\)
Squaring on both sides
225 = r

Question 11.
3\(\sqrt{y}\) – 18 = -3
Answer:

Question 12.
2\(\sqrt{q}\) + 5 = 11
Answer:
Given,
2\(\sqrt{q}\) + 5 = 11
Subtract 5 on both sides
2\(\sqrt{q}\) + 5 – 5 = 11 – 5
2\(\sqrt{q}\) = 6
\(\sqrt{q}\) = 6/2 = 3
Squaring on both sides
q = 9

In Exercises 13–20, solve the equation. Check your solution.
Question 13.
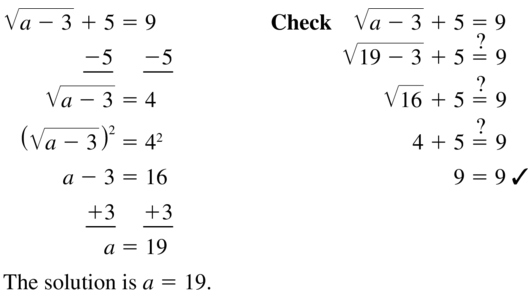
\(\sqrt{a-3}\) + 5 = 9
Answer:

Question 14.
\(\sqrt{b+7}\) – 5 = -2
Answer:
Given,
\(\sqrt{b+7}\) – 5 = -2
Add 5 on both sides
\(\sqrt{b+7}\) – 5 + 5 = -2 + 5
\(\sqrt{b+7}\) = 3
Squaring on both sides
\(\sqrt{b+7}\)² = 3² = 9
b + 7 = 9
b = 9 – 7
b = 2

Question 15.
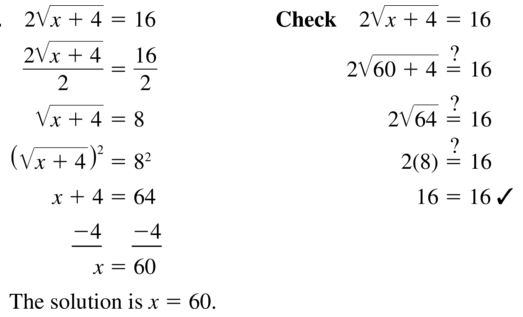
2 \(\sqrt{x+4}\) = 16
Answer:

Question 16.
5\(\sqrt{y-2}\) = 10
Answer:
5\(\sqrt{y-2}\) = 10
Divide both sides by 5
5\(\sqrt{y-2}\)/5 = 10/5
\(\sqrt{y-2}\) = 2
Squaring on both sides
\(\sqrt{y-2}\)² = 2² = 4
y – 2 = 4
Add 2 on both sides
y – 2 + 2 = 4 + 2
y = 6

Question 17.
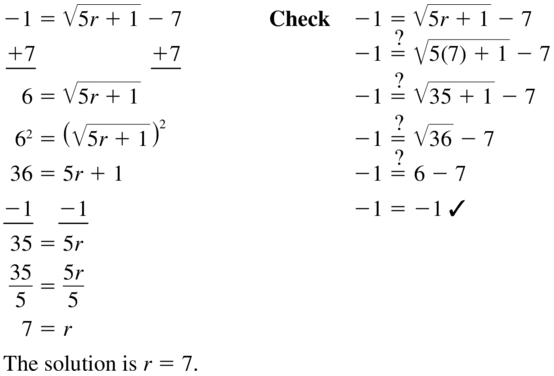
-1 = \(\sqrt{5r+1}\) – 7
Answer:

Question 18.
2 = \(\sqrt{4s-4}\) – 4
Answer:
Given,
2 = \(\sqrt{4s-4}\) – 4
Add 4 to each side
2 + 4 = \(\sqrt{4s-4}\) – 4 + 4
6 = \(\sqrt{4s-4}\)
Squaring on both sides
6² = \(\sqrt{4s-4}\)²
4s – 4 = 36
4s = 36 + 4
4s = 40
s = 40/4
s = 10

Question 19.
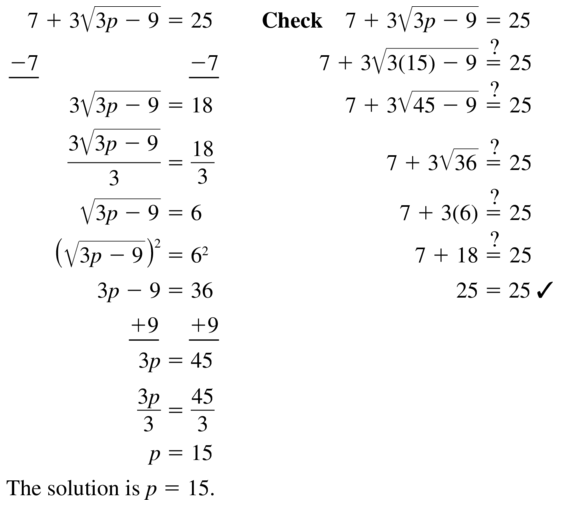
7 + 3\(\sqrt{3p-9}\) = 25
Answer:

Question 20.
19 – 4\(\sqrt{3c-11}\) = 11
Answer:
Given,
19 – 4\(\sqrt{3c-11}\) = 11
Subtract19 on both sides
19 – 4\(\sqrt{3c-11}\) – 19 = 11 – 19
– 4\(\sqrt{3c-11}\) = -8
4\(\sqrt{3c-11}\) = 8
\(\sqrt{3c-11}\) = 8/4
\(\sqrt{3c-11}\) = 2
Squaring on both sides
\(\sqrt{3c-11}\)² = 2² = 4
3c – 11 = 4
3c = 4 + 11
3c = 15
c = 15/3
c = 5

Question 21.
MODELING WITH MATHEMATICS
The Cave of Swallows is a natural open-air pit cave in the state of San Luis Potosí, Mexico. The 1220-foot- deep cave was a popular destination for BASE jumpers. The function t = \(\frac{1}{4} \sqrt{d}\) represents the time t (in seconds) that it takes a BASE jumper to fall d feet. How far does a BASE jumper fall in 3 seconds?

Answer:

Question 22.
MODELING WITH MATHEMATICS
The edge length s of a cube with a surface area of A is given by s = \(\sqrt{\frac{A}{6}}\). What is the surface area of a cube with an edge length of 4 inches?

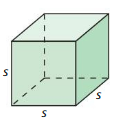
Answer:
Given that,
The edge length s of a cube with a surface area of A is given by s = \(\sqrt{\frac{A}{6}}\)
s = 4
4 = \(\sqrt{\frac{A}{6}}\)
Squaring on both sides
16 = \(\sqrt{\frac{A}{6}}\)²
\({\frac{A}{6}}\) = 16
A = 16 × 6 = 96

In Exercises 23–26, use the graph to solve the equation.
Question 23.

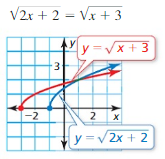
Answer:

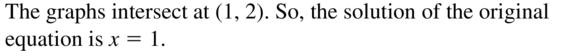
Question 24.

Answer:
The intersection of the two parabolas is the point (5, 4)
Thus the solution of the equation is (5, 4)

Question 25.

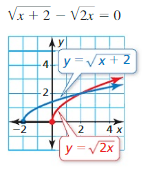
Answer:
The graphs intersect at (2, 2). So, the solution of the original equation is x = 2

Question 26.

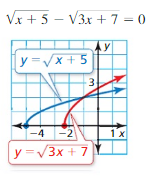
Answer:
The x value where the 2 lines intersect is x = -1

In Exercises 27–34, solve the equation. Check your solution. (See Example 3.)
Question 27.
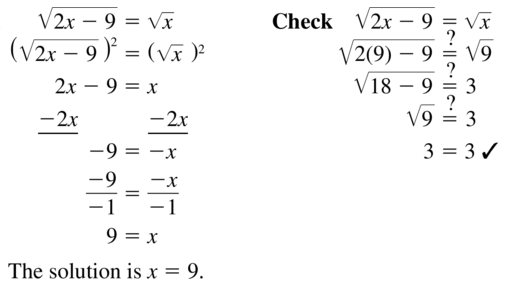
\(\sqrt{2x-9}\) = \(\sqrt{x}\)
Answer:

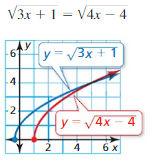
Question 28.
\(\sqrt{y+1}\) = \(\sqrt{4y-8}\)
Answer:
Given,
\(\sqrt{y+1}\) = \(\sqrt{4y-8}\)
Squaring on both sides
\(\sqrt{y+1}\)² = \(\sqrt{4y-8}\)²
y + 1 = 4y – 8
y = 4y – 8 – 1
4y – y = 9
3y = 9
y = 9/3 = 3
y = 3

Question 29.
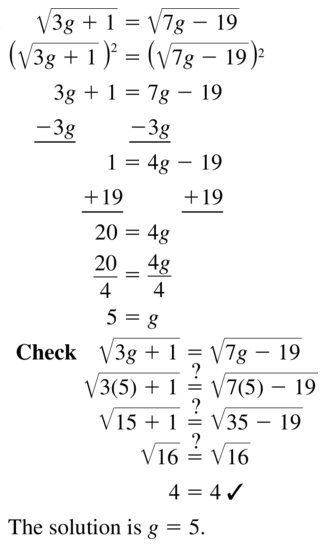
\(\sqrt{3g+1}\) = \(\sqrt{7g-19}\)
Answer:

Question 30.
\(\sqrt{8h-7}\) = \(\sqrt{6h+7}\)
Answer:
Given,
\(\sqrt{8h-7}\) = \(\sqrt{6h+7}\)
Squaring on both sides
\(\sqrt{8h-7}\)² = \(\sqrt{6h+7}\)²
8h – 7 = 6h + 7
8h – 6h = 7 + 7
2h = 14
h = 7

Question 31.
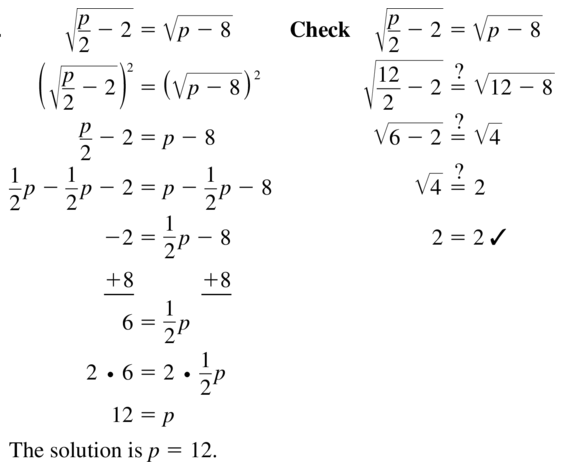
\(\sqrt{\frac{p}{2}-2}\) = \(\sqrt{p-8}\)
Answer:

Question 32.
\(\sqrt{2v-5}\) = \(\sqrt{\frac{v}{3}+5}\)
Answer:
Given,
\(\sqrt{2v-5}\) = \(\sqrt{\frac{v}{3}+5}\)
Squaring on both sides
\(\sqrt{2v-5}\)² = \(\sqrt{\frac{v}{3}+5}\)²
2v – 5 = 5 + v/3
2v = 10 + v/3
6v = 30 + v
6v – v = 30
5v = 30
v = 6

Question 33.
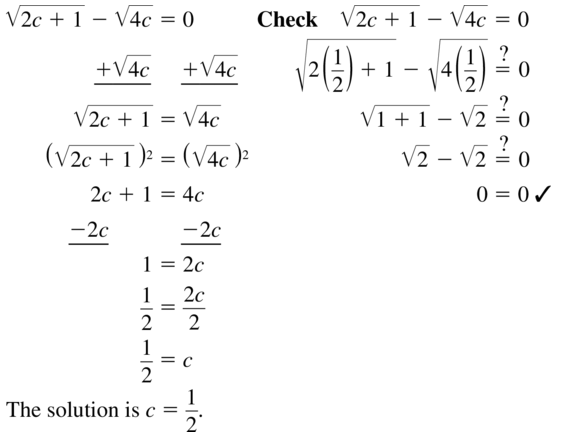
\(\sqrt{2c+1}\) = \(\sqrt{4c}\) = 0
Answer:

Question 34.
\(\sqrt{5r}\) – \(\sqrt{8r-2}\) = 0
Answer:
Given,
\(\sqrt{5r}\) – \(\sqrt{8r-2}\) = 0
\(\sqrt{5r}\) = \(\sqrt{8r-2}\)
Squaring on both sides
\(\sqrt{5r}\)² = \(\sqrt{8r-2}\)²
5r = 8r – 2
2 = 8r – 5r
2 = 3r
r = 2/3

MATHEMATICAL CONNECTIONS In Exercises 35 and 36, find the value of x.
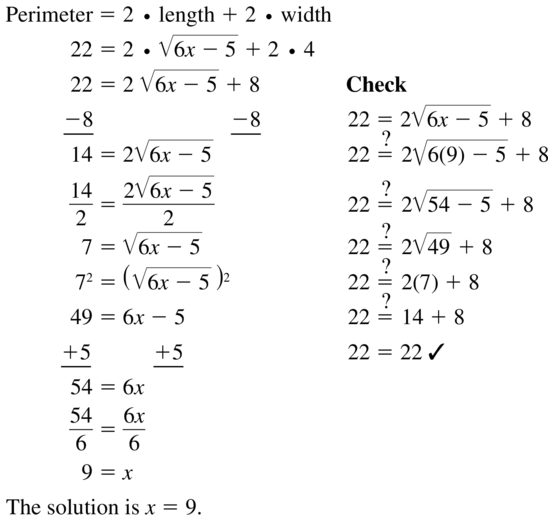
Question 35.
Perimeter = 22 cm

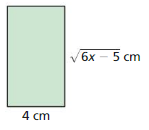
Answer:

Question 36.

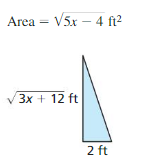
Answer:
\(\sqrt{3x+12}\)(2)(1/2) = \(\sqrt{5x-4}\)
\(\sqrt{3x+12}\) = \(\sqrt{5x-4}\)
Squaring on both sides
3x + 12 = 5x – 4
3x – 5x = -4 – 12
-2x = -16
2x = 16
x = 8

In Exercises 37–44, solve the equation. Check your solution.
Question 37.
\(\sqrt [3]{ x }\) = 4
Answer:

Question 38.
\(\sqrt [3]{ y }\) = 2
Answer:
Given,
\(\sqrt [3]{ y }\) = 2
Cubing on both sides
y = 8

Question 39.
6 = 3\(\sqrt [3]{ 8g }\)
Answer:

Question 40.
\(\sqrt [3]{ r+19 }\) = 3
Answer:
Given,
\(\sqrt [3]{ r+19 }\) = 3
Cubing on both sides
r + 19 = 27
r = 27 – 19
r = 8

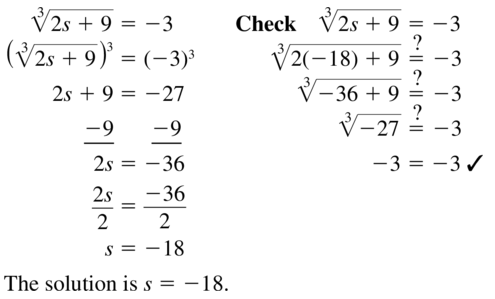
Question 41.
\(\sqrt [3]{ 2x+9 }\) = -3
Answer:

Question 42.
-5 = \(\sqrt [3]{ 10x+15 }\)
Answer:
-5 = \(\sqrt [3]{ 10x+15 }\)
Cubing on both sides
(-5)³ = \(\sqrt [3]{ 10x+15 }\)³
-125 = 10x + 15
-125 – 15 = 10x
-140 = 10x
x = -14

Question 43.
\(\sqrt [3]{ y+6 }\) = \(\sqrt [3]{ 5y-2 }\)
Answer:

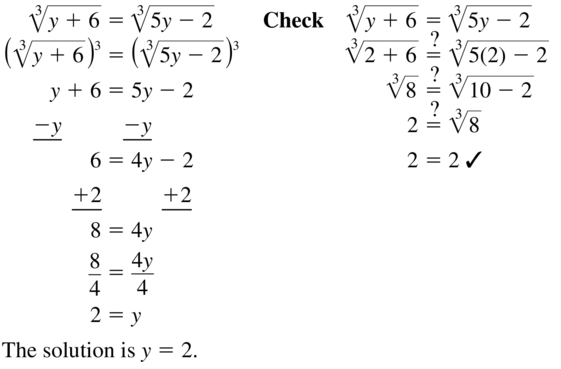
Question 44.
\(\sqrt [3]{ 7j-2 }\) = \(\sqrt [3]{ j+4 }\)
Answer:
Given,
\(\sqrt [3]{ 7j-2 }\) = \(\sqrt [3]{ j+4 }\)
Cubing on both sides
7j – 2 = j + 4
7j – j = 4 + 2
6j = 6
j = 1

In Exercises 45–48, determine which solution, if any, is an extraneous solution.
Question 45.
\(\sqrt{6x-5}\) = x; x = 5, x = 1
Answer:

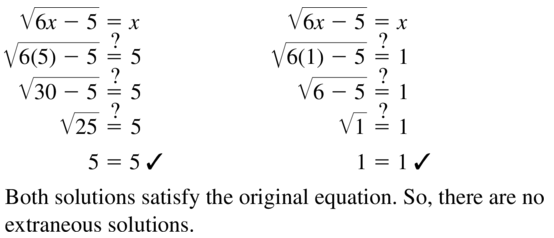
Question 46.
\(\sqrt{2y+3}\) = y; y = -1, y = 3
Answer:
Given,
\(\sqrt{2y+3}\) = y
Substitute y = -1 in the above equation
\(\sqrt{2(-1)+3}\) = -1
\(\sqrt{-2+3}\) = -1
\(\sqrt{1}\) ≠ -1
Now for y = 3
\(\sqrt{2(3)+3}\) = 3
\(\sqrt{6+3}\) = 3
\(\sqrt{9}\) = 3
y = -1 does not satisfy the original equation, it is an extraneous solution.
The only solution is y = 3

Question 47.
\(\sqrt{12p+16}\) = -2p; p = -1, p = 4
Answer:

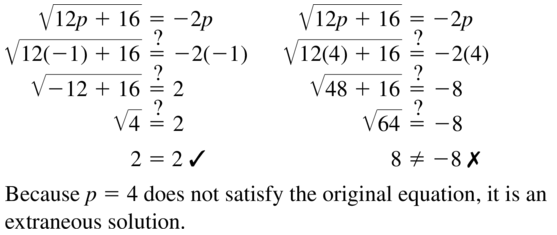
Question 48.
-3g = \(\sqrt{-18-27g}\); g = -2, g = -1
Answer:
Given,
-3g = \(\sqrt{-18-27g}\)
For g = -2
-3(-2) = \(\sqrt{-18-27(-2)}\)
6 = \(\sqrt{36}\)
6 = 6
For g = -1
-3(-1) = \(\sqrt{-18-27(-1)}\)
3 = \(\sqrt{9}\)
3 = 3
There is no extraneous solution.

In Exercises 49–58, solve the equation. Check your solution(s).
Question 49.
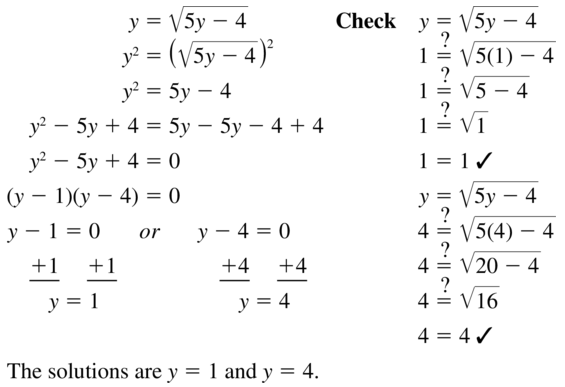
y = \(\sqrt{5y-4}\)
Answer:

Question 50.
\(\sqrt{-14x-9x}\) = x
Answer:
Given,
\(\sqrt{-14x-9x}\) = x
Squaring on both sides
-14x – 9x = x²
x² +14 + 9x = 0
(x + 7)(x + 2) = 0
x + 7 = 0 or x + 2 = 0
x = -7 or x = -2

Question 51.
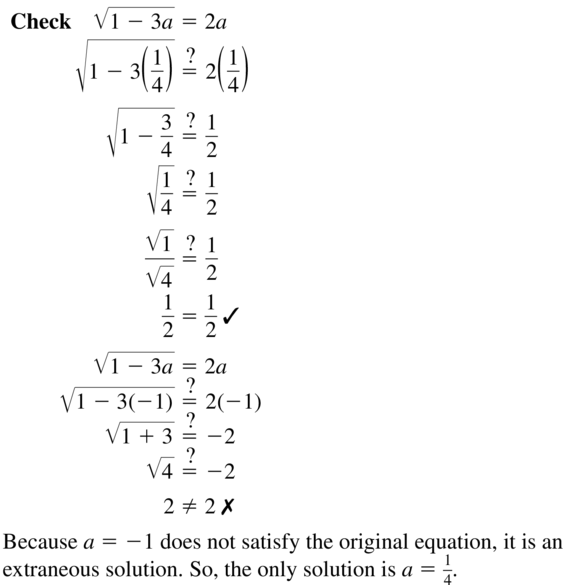
\(\sqrt{1-3a}\) = 2a
Answer:

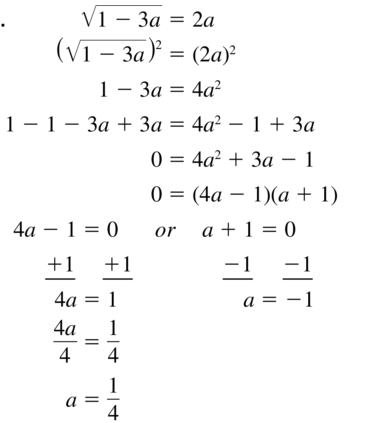
Question 52.
2q = \(\sqrt{10q-6}\)
Answer:
Given,
2q = \(\sqrt{10q-6}\)
Squaring on both sides
(2q)² = \(\sqrt{10q-6}\)²
4q² = 10q – 6
4q² – 10q + 6 = 0
(2q – 3)(q – 1) = 0
2q – 3 = 0 or q – 1 = 0
2q = 3 or q = 1
q = 3/2 or q = 1

Question 53.
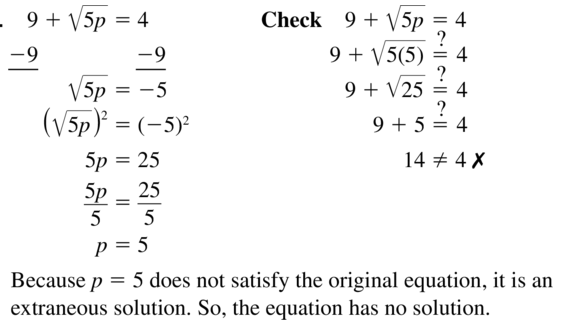
9 + \(\sqrt{5p}\) = 4
Answer:

Question 54.
\(\sqrt{3n}\) – 11 = -5
Answer:
Given,
\(\sqrt{3n}\) – 11 = -5
\(\sqrt{3n}\) = -5 + 11
\(\sqrt{3n}\) = 6
Squaring on both sides
3n = 36
n = 36/3
n = 12

Question 55.
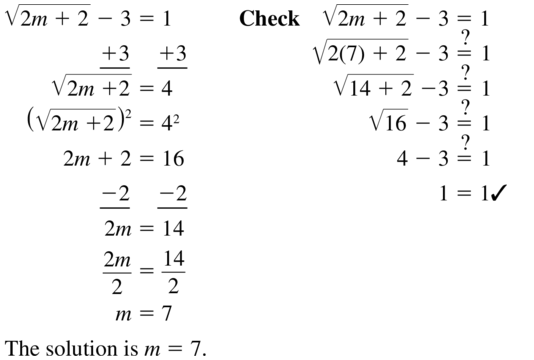
\(\sqrt{2m+2}\) – 3 = 1
Answer:

Question 56.
15 + \(\sqrt{4b-8}\) = 13
Answer:
Given,
15 + \(\sqrt{4b-8}\) = 13
\(\sqrt{4b-8}\) = 13 – 15
\(\sqrt{4b-8}\) = -2
Squaring on both sides
4b – 8 = 4
4b = 4 + 8
4b = 12
b = 12/4
b = 3

Question 57.
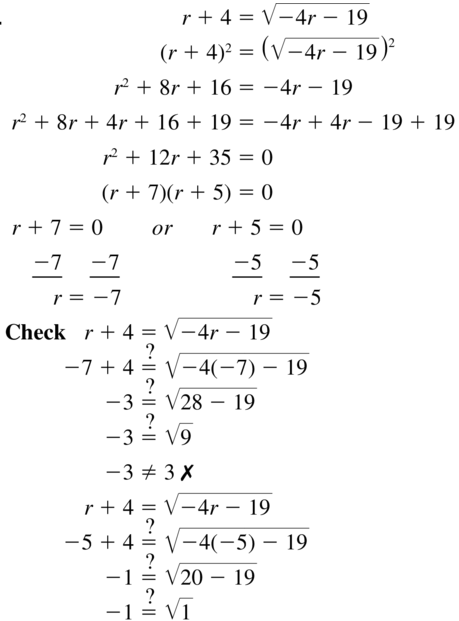
r + 4 = \(\sqrt{-4r-19}\)
Answer:

Question 58.
\(\sqrt{3-s}\) = s – 1
Answer:
Given,
\(\sqrt{3-s}\) = s – 1
Squaring on both sides
3 – s = (s – 1)²
3 – s = s² – 2s + 1
s² – 2s + 1 – 3 + s
s² – s – 2 = 0
(s – 2)(s + 1) = 0
s – 2 = 0 or s + 1 = 0
s = 2 or s = -1

ERROR ANALYSIS In Exercises 59 and 60, describe and correct the error in solving the equation.
Question 59.

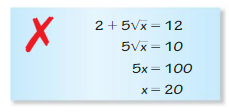
Answer:

Question 60.

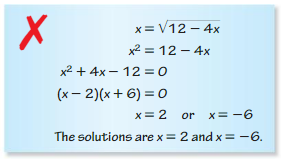
Answer:
Forgot to check the solutioons where x = -6 is an extraneous solution
-6 = \(\sqrt{12-4(-6)}\)
-6 = \(\sqrt{36}\)
– 6 = 6

Question 61.
REASONING
Explain how to use mental math to solve \(\sqrt{2x}\) + 5 = 1.
Answer:

Question 62.
WRITING
Explain how you would solve \(\sqrt [4]{ m+4 }\) – \(\sqrt [4]{ 3m }\) = 0.
Answer:
\(\sqrt [4]{ m+4 }\) – \(\sqrt [4]{ 3m }\) = 0
\(\sqrt [4]{ m+4 }\) = \(\sqrt [4]{ 3m }\)
m + 4 = 3m
m – 3m = -4
-2m = -4
2m = 4
m = 4/2
m = 2

Question 63.
MODELING WITH MATHEMATICS
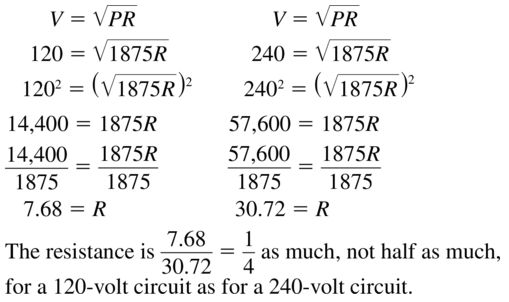
The formula V = \(\sqrt{PR}\) relates the voltage V (in volts), power P (in watts), and resistance R (in ohms) of an electrical circuit. The hair dryer shown is on a 120-volt circuit. Is the resistance of the hair dryer half as much as the resistance of the same hair dryer on a 240-volt circuit? Explain your reasoning.

Answer:

Question 64.
MODELING WITH MATHEMATICS
The time t (in seconds) it takes a trapeze artist to swing back and forth is represented by the function t = 2π \(\sqrt{\frac{r}{32}}\), where r is the rope length (in feet). It takes the trapeze artist 6 seconds to swing back and forth. Is this rope \(\frac{3}{2}\) as long as the rope used when it takes the trapeze artist 4 seconds to swing back and forth? Explain your reasoning.

Answer:
Solve for r at t = 4 and t = 6
At t = 6 seconds
6 = 2π \(\sqrt{\frac{r}{32}}\)
\(\frac{3}{π}\) = \(\sqrt{\frac{r}{32}}\)
r = \(\frac{288}{π²}\)
At t = 4 seconds
4 = 2π \(\sqrt{\frac{r}{32}}\)
\(\frac{4}{π²}\) = \({\frac{r}{32}}\)
r = \(\frac{128}{π²}\)
Therefore, the length of the rope that takes 6 seconds to swing back and forth is 2.25 times the length of the rope that takes 4 seconds to swing back and forth, not 3/2 or 1.5

REASONING In Exercises 65–68, determine whether the statement is true or false. If it is false, explain why.
Question 65.
If \(\sqrt{a}\) = b, then (\(\sqrt{a}\))² = b².
Answer:

Question 66.
If \(\sqrt{a}\) = \(\sqrt{b}\), then a = b.
Answer:
\(\sqrt{a}\) = \(\sqrt{b}\)
Squaring on both sides
\(\sqrt{a}\)² = \(\sqrt{b}\)²
a = b
Thus the above statement is true.

Question 67.
If a² = b², then a = b.
Answer:

Question 68.
If a² = \(\sqrt{b}\), then a⁴ = (\(\sqrt{b}\))²
Answer:
a² = \(\sqrt{b}\)
squaring on both sides
We get a⁴ = (\(\sqrt{b}\))²

Question 69.
COMPARING METHODS
Consider the equation x + 2 = \(\sqrt{2x-3}\).
a. Solve the equation by graphing. Describe the process.
b. Solve the equation algebraically. Describe the process.
c. Which method do you prefer? Explain your reasoning.
Answer:

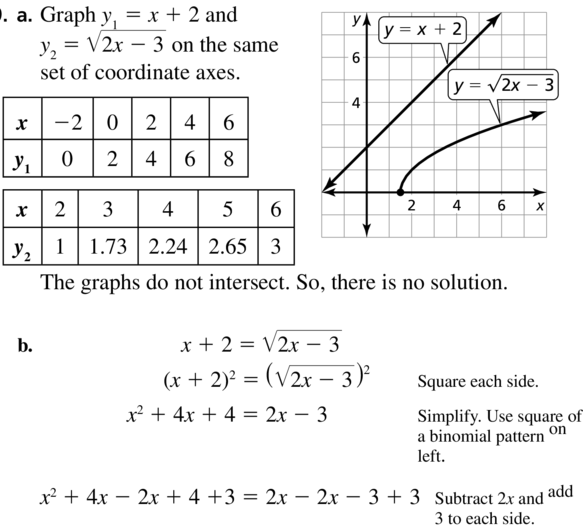
Question 70.
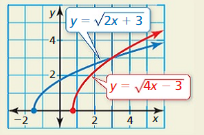
HOW DO YOU SEE IT?
The graph shows two radical functions.

a. Write an equation whose solution is the x-coordinate of the point of intersection of the graphs.
Answer:
Using the above graph, equate the 2 y’s since the point of intersection is the solution
y1 = y2
\(\sqrt{2x+3}\) = \(\sqrt{4x-3}\).

b. Use the graph to solve the equation.
Answer:
From the graph, the solution is x = 3, the x-coordinate of the point of intersection.

Question 71.
MATHEMATICAL CONNECTIONS
The slant height s of a cone with a radius of r and a height of h is given by s = \(\sqrt{r^{2}+h^{2}}\). The slant heights of the two cones are equal. Find the radius of each cone.

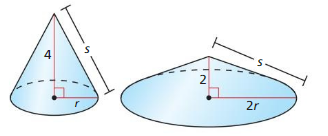
Answer:

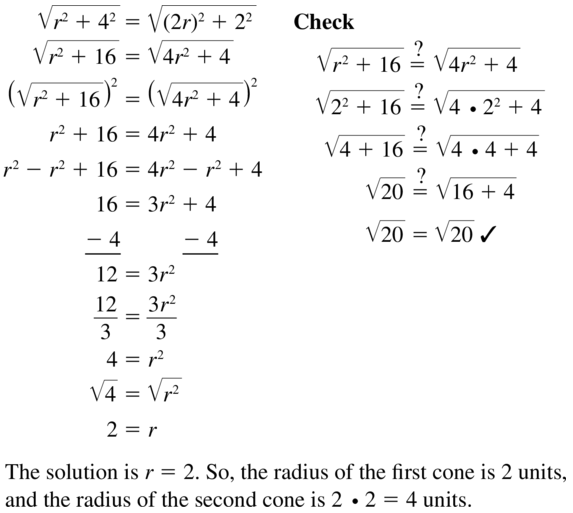
Question 72.
CRITICAL THINKING
How is squaring \(\sqrt{x+2}\) different from squaring \(\sqrt{x}\) + 2?
Answer:
Squaring \(\sqrt{x+2}\) will cancel out the radical symbol and results into x + 2.
Squaring \(\sqrt{x+2}\) is the same as squaring a binomial sum: x + 4 \(\sqrt{x}\) + 4

USING STRUCTURE In Exercises 73–78, solve the equation. Check your solution.
Question 73.
\(\sqrt{m+15}\) = \(\sqrt{m}\) + \(\sqrt{5}\)
Answer:

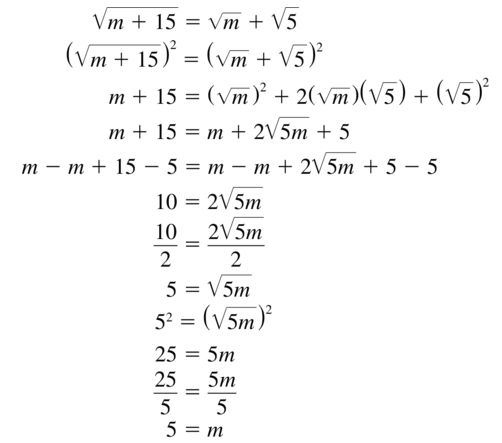
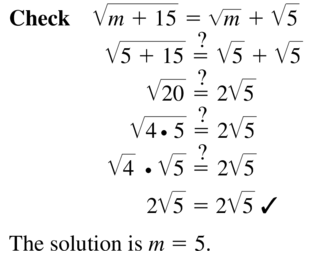
Question 74.
2 – \(\sqrt{x+1}\) = \(\sqrt{x+2}\)
Answer:
Given,
2 – \(\sqrt{x+1}\) = \(\sqrt{x+2}\)
Squaring on both sides
(2 – \(\sqrt{x+1}\))² = \(\sqrt{x+2}\)²
4 – 4 \(\sqrt{x+1}\) + (x + 1) = x + 2
– 4 \(\sqrt{x+1}\) + x + 1 = x + 2 – 4
– 4 \(\sqrt{x+1}\) = x – 2 – x – 1
– 4 \(\sqrt{x+1}\) = -3
4 \(\sqrt{x+1}\) = 3
\(\sqrt{x+1}\) = 3/4
x + 1 = 9/16
x = -7/16

Question 75.
\(\sqrt{5y+9}\) + \(\sqrt{5y}\) = 9
Answer:

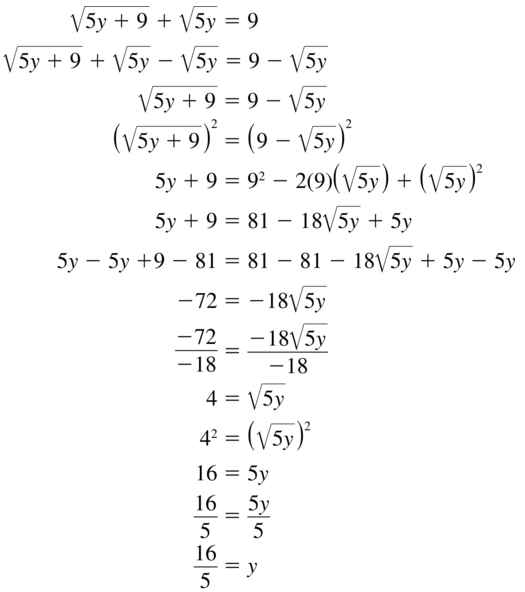
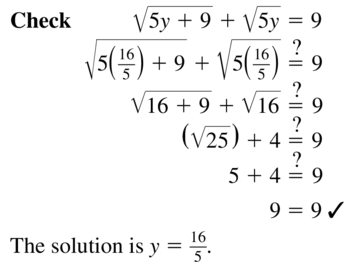
Question 76.
\(\sqrt{2c-8}\) – \(\sqrt{2c}\) – 4 = 0
Answer:
Given,
\(\sqrt{2c-8}\) – \(\sqrt{2c}\) – 4 = 0
\(\sqrt{2c-8}\) = \(\sqrt{2c}\) + 4
Squaring on both sides
\(\sqrt{2c-8}\)² = (\(\sqrt{2c}\) + 4)²
2c – 8 = 2c + 8\(\sqrt{2c}\) + 16
2c – 8 – 2c = 8\(\sqrt{2c}\) + 16
-8 = 8\(\sqrt{2c}\) + 16
Divide by 8 on both sides
-1 = \(\sqrt{2c}\) + 2
-3 = \(\sqrt{2c}\)
Squaring on both sides
9 = 2c
c = 9/2

Question 77.
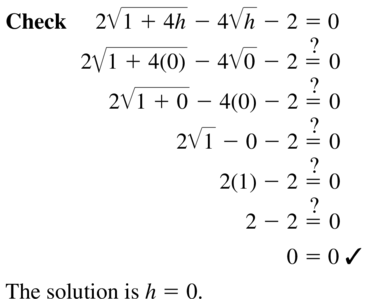
2\(\sqrt{1+4h}\) – 4\(\sqrt{h}\) – 2 = 0
Answer:

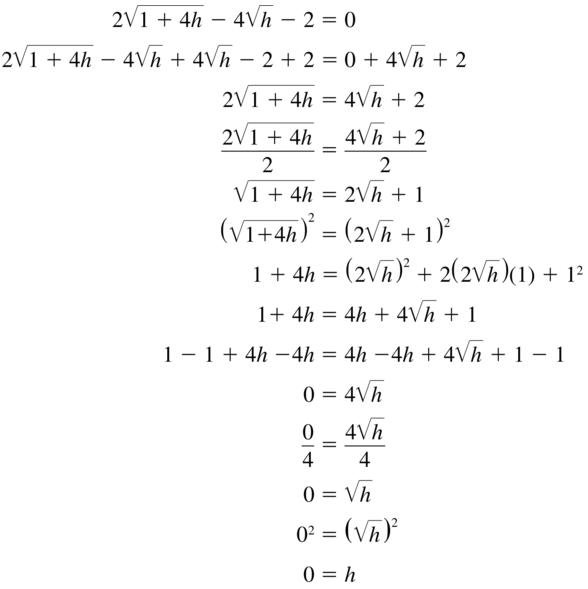
Question 78.
\(\sqrt{20-4z}\) + 2\(\sqrt{-z}\) = 10
Answer:
Given,
\(\sqrt{20-4z}\) + 2\(\sqrt{-z}\) = 10
\(\sqrt{20-4z}\) = 10 – 2\(\sqrt{-z}\)
Squaring on both sides
\(\sqrt{20-4z}\)² = (10 – 2\(\sqrt{-z}\))²
20 – 4z = 100 – 40\(\sqrt{-z}\) – 4z
20 = 100 – 40\(\sqrt{-z}\)
40\(\sqrt{-z}\) = 100 – 20 = 80
40\(\sqrt{-z}\) = 80
\(\sqrt{-z}\) = 2
-z = 4
z = -4

Question 79.
OPEN-ENDED
Write a radical equation that has a solution of x = 5.
Answer:

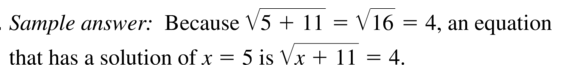
Question 80.
OPEN-ENDED
Write a radical equation that has x = 3 and x = 4 as solutions.
Answer:
The possible answer is writing the quadratic function (x – 3)(x – 4) under the radical symbol and simplify it.
then equate to 0
\(\sqrt{(x-3)(x-4)}\) = 0

Question 81.
MAKING AN ARGUMENT
Your friend says the equation \(\sqrt{(2 x+5)^{2}}\) = 2x + 5 is always true, because after simplifying the left side of the equation, the result is an equation with infinitely many solutions. Is your friend correct? Explain.
Answer:

Question 82.
THOUGHT PROVOKING
Solve the equation \(\sqrt [3]{ x+1 }\) = \(\sqrt{x-3}\). Show your work and explain your steps.
Answer:
\(\sqrt [3]{ x+1 }\) = \(\sqrt{x-3}\)
let y1 = \(\sqrt [3]{ x+1 }\) and y2 = \(\sqrt{x-3}\)
Let x = 7
\(\sqrt [3]{ 7+1 }\) = \(\sqrt{7-3}\)
\(\sqrt [3]{ 8 }\) = \(\sqrt{4}\)
2 = 2
Therefore the solution is x = 7.

Question 83.
MODELING WITH MATHEMATICS
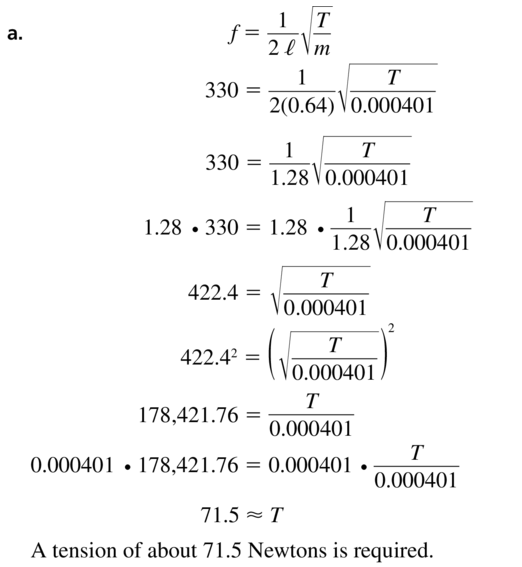
The frequency f (in cycles per second) of a string of an electric guitar is given by the equation f = \(\frac{1}{2 \ell} \sqrt{\frac{T}{m}}\), where ℓ is the length of the string (in meters), T is the string’s tension (in newtons), and m is the string’s mass per unit length (in kilograms per meter). The high E string of an electric guitar is 0.64 meter long with a mass per unit length of 0.000401 kilogram per meter.

a. How much tension is required to produce a frequency of about 330 cycles per second?
b. Would you need more or less tension to create the same frequency on a string with greater mass per unit length? Explain.
Answer:

Maintaining Mathematical Proficiency

Find the product.(Section 7.2)
Question 84.
(x + 8)(x – 2)
Answer:
(x + 8)(x – 2)
x(x – 2) + 8 (x – 2)
x² -2x + 8x – 16
x² +6x – 16

Question 85.
(3p – 1)(4p + 5)
Answer:

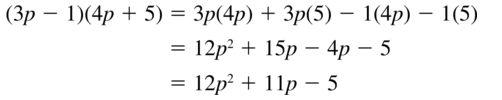
Question 86.
(s + 2)(s² + 3s – 4)
Answer:
Given,
(s + 2)(s² + 3s – 4)
s(s² + 3s – 4) + 2 (s² + 3s – 4)
s³ + 3s² – 4s + 2s² + 6s – 8
s³ + 5s² + 2s – 8

Graph the function. Compare the graph to the graph of f(x) = x².(Section 8.1)
Question 87.
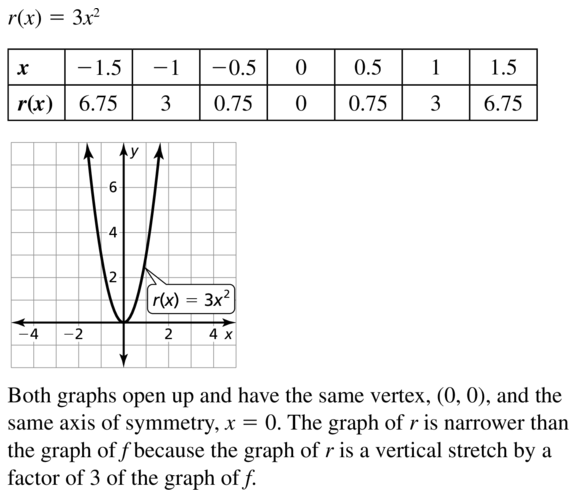
r(x) = 3x²
Answer:

Question 88.
g(x) = \(\frac{3}{4}\)x²
Answer:

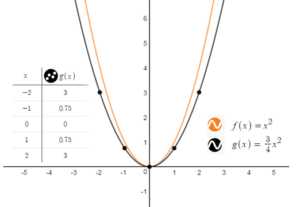
The graph of g(x) is the graph of f(x) that is
– Vertically shrinked by a factor of 3/4

Question 89.
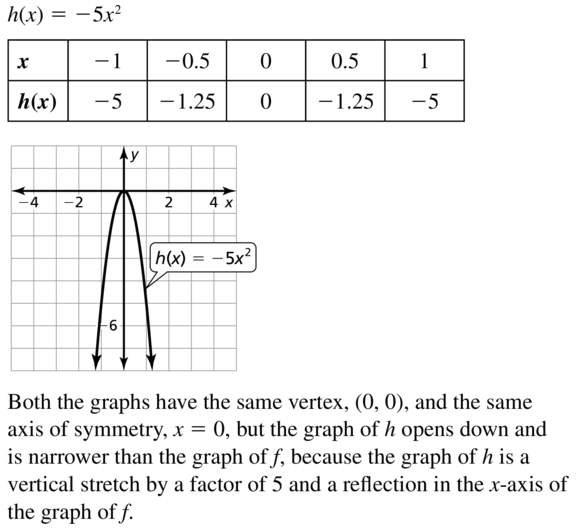
h(x) = -5x²
Answer:

Lesson 10.4 Inverse of a Function

Essential Question How are a function and its inverse related?

EXPLORATION 1

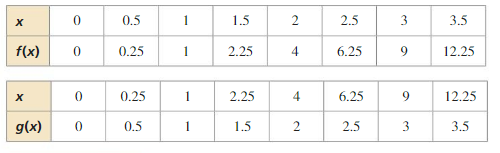
Exploring Inverse Functions
Work with a partner. The functions f and g are inverses of each other. Compare the tables of values of the two functions. How are the functions related?

EXPLORATION 2

Exploring Inverse Functions
Work with a partner.
a. Plot the two sets of points represented by the tables in Exploration 1. Use the coordinate plane below.
b. Connect each set of points with a smooth curve.
c. Describe the relationship between the two graphs.
d. Write an equation for each function.

Communicate Your Answer

Question 3.
How are a function and its inverse related?
Answer:
The inverse of a function is defined as the function that reverses other functions. Suppose f(x) is the function, then its inverse can be represented as f^-1(x).

Question 4.
A table of values for a function f is given. Create a table of values for a function g, the inverse of f.

Answer:

Question 5.
Sketch the graphs of f(x) = x + 4 and its inverse in the same coordinate plane. Then write an equation of the inverse of f. Explain your reasoning.

Answer:

Monitoring Progress

Find the inverse of the relation.
Question 1.
(-3, -4), (-2, 0), (-1, 4), (0, 8), (1, 12), (2, 16), (3, 20)
Answer:
Switch the coordinates of each ordered pair.
(-4, -3), (0, -2), (4, -1), (8, 0), (12, 1), (16, 2), (20, 3)

Question 2.

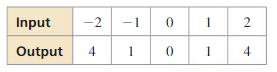
Answer:

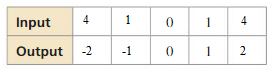
Solve y = f(x) for x. Then find the input when the output is 4.
Question 3.
f(x) = x – 6
Answer:
f(x) = y
y = x – 6
x = y + 6
If y = 4
x = 4 + 6
x = 10

Question 4.
f(x) = \(\frac{1}{2}\)x + 3
Answer:
f(x) = y
y = \(\frac{1}{2}\)x + 3
y – 3 = \(\frac{1}{2}\)x
x = 2y – 6
If y = 4
x = 2(4) – 6
x = 8 – 6
x = 4

Question 5.
f(x) = 4x²
Answer:
f(x) = y
y = 4x²
x² = y/4
If y = 4
x² = 4/4 = 1
x = 1

Find the inverse of the function. Then graph the function and its inverse.
Question 6.
f(x) = 6x
Answer:
Given function is f(x) = 6
f(x) = y
y = 6x
x = 6y
y = x/6
f^-1(x) = x/6

Question 7.
f(x) = -x + 5
Answer:
Given function f(x) = -x + 5
f(x) = y
y = -x + 5
Interchange x and y
x = -y + 5
y = 5 – x
f^-1(x) = 5 – x

Question 8.
f(x) = \(\frac{1}{4}\)x – 1
Answer:
Given function,
f(x) = \(\frac{1}{4}\)x – 1
f(x) = y
y = \(\frac{1}{4}\)x – 1
x = \(\frac{1}{4}\)y – 1
x + 1 = \(\frac{y}{4}\)
4(x + 1) = y

Find the inverse of the function. Then graph the function and its inverse.
Question 9.
f(x) = -x², x ≤ 0
Answer:
f(x) = -x²
f(x) = y
y = -x²
x = -y²
y² = -x
y = √-x

Question 10.
f(x) = 4x² + 3, x ≥ 0
Answer:
Given function,
f(x) = 4x² + 3
f(x) = y
y = 4x² + 3
x = 4y² + 3
x – 3 = 4y²
y² = x – 3/4
y = √(x-3)/2

Question 11.
Is the inverse of f(x) = \(\sqrt{2x-1}\) a function? Find the inverse.
Answer:
f(x) = \(\sqrt{2x-1}\)
f(x) = y
y = \(\sqrt{2x-1}\)
y² = 2x – 1
x² = 2y – 1
x² + 1 = 2y
y = (x²+1)/2

Inverse of a Function 10.4 Exercises

Vocabulary and Core ConceptCheck

Question 1.
COMPLETE THE SENTENCE
A relation contains the point (-3, 10). The ____________ contains the point (10, -3).
Answer:
A relation contains the point (-3, 10). The inverse contains the point (10, -3).

Question 2.
DIFFERENT WORDS, SAME QUESTION
Consider the function f represented by the graph. Which is different? Find “both” answers.

Answer:
The blue points A, B, C and D represent the original function f that was given.
The first, third and forth statement then results in the orange points E, F, G and H while the second statement results in the pink points I, J, K and L.
Thus we can say that the second statement is different.

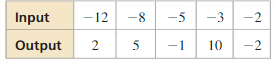
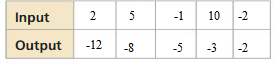
In Exercises 3–8, find the inverse of the relation.
Question 3.
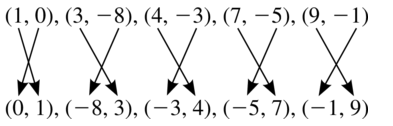
(1, 0), (3, -8), (4, -3), (7, -5), (9, -1)
Answer:

Question 4.
(2, 1), (4, -3), (6, 7), (8, 1), (10, -4)
Answer:
Switch the coordinates of each ordered pair.
(1, 2), (-3, 4), (7, 6), (1, 8), (-4, 10)

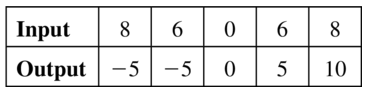
Question 5.

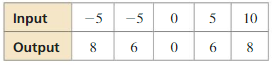
Answer:
Switch the coordinates of each ordered pair.

Question 6.

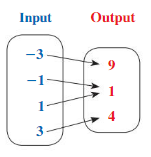
Answer:
Switch the coordinates of each ordered pair.

Question 7.

Answer:
Switch the coordinates of each ordered pair.

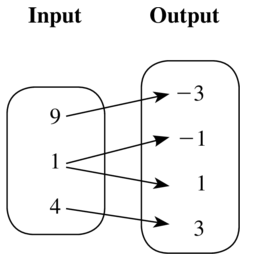
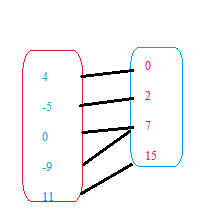
Question 8.

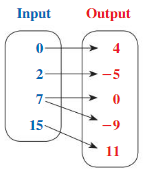
Answer:
Switch the coordinates of each ordered pair.
The inverse relation of the above function is given below.

In Exercises 9–14, solve y = f(x) for x. Then find the input when the output is 2.
Question 9.
f(x) = x + 5
Answer:

Question 10.
f(x) = 2x – 3
Answer:
Given,
f(x) = 2x – 3
y = 2x + 3
To find x:
y – 3 = 2x
x = \(\frac{1}{2}\)y + \(\frac{3}{2}\)
If y = 2:
x = \(\frac{1}{2}\)(2) + \(\frac{3}{2}\)
x = 1 + 1.5
x = 2.5

Question 11.
f(x) = \(\frac{1}{4}\)x – 1
Answer:

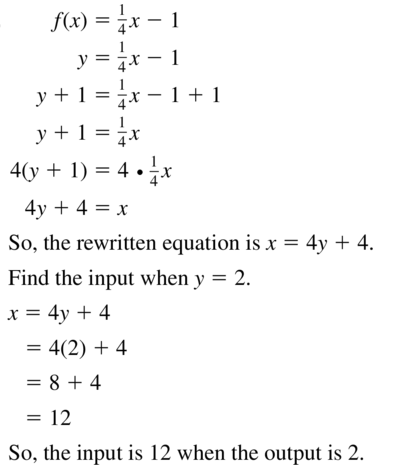
Question 12.
f(x) = \(\frac{2}{3}\)x + 4
Answer:
Given,
f(x) = \(\frac{2}{3}\)x + 4
y = \(\frac{2}{3}\)x + 4
3y = 2x + 12
3y – 12 = 2x
x = \(\frac{3}{2}\)y – 6
If y = 2
x = \(\frac{3}{2}\)(2) – 6
x = 3 – 6
x = -3

Question 13.
f(x) = 9x²
Answer:

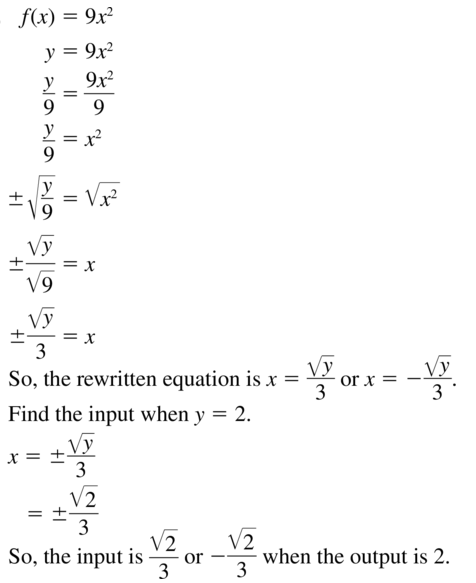
Question 14.
f(x) = \(\frac{1}{2}\)x² – 7
Answer:
Given,
f(x) = \(\frac{1}{2}\)x² – 7
y = \(\frac{1}{2}\)x² – 7
y + 7 = \(\frac{1}{2}\)x²
2y + 14 = x²
x = \(\sqrt{2y+14}\)
If y = 2
x = \(\sqrt{2(2)+14}\)
x = \(\sqrt{18}\)

In Exercises 15 and 16, graph the inverse of the function by reflecting the graph in the line y = x. Describe the domain and range of the inverse.
Question 15.

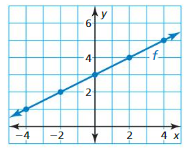
Answer:

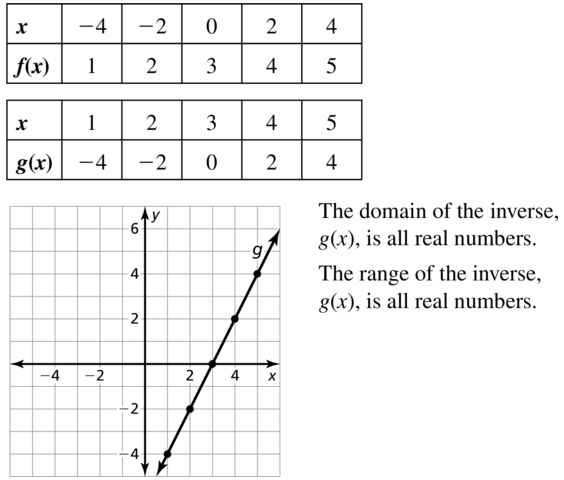
Question 16.

Answer:
The line of the given function passes the two points (-3, -1) and (2, 4)
The function of the line is
y = x + 2
By reflecting the graph in the line y = x, we get the function
y = x – 2
The domain of the inverse is -∞ < x < ∞
The range of the inverse is -∞ < x < ∞

In Exercises 17–22, find the inverse of the function. Then graph the function and its inverse.
Question 17.
f(x) = 4x – 1
Answer:

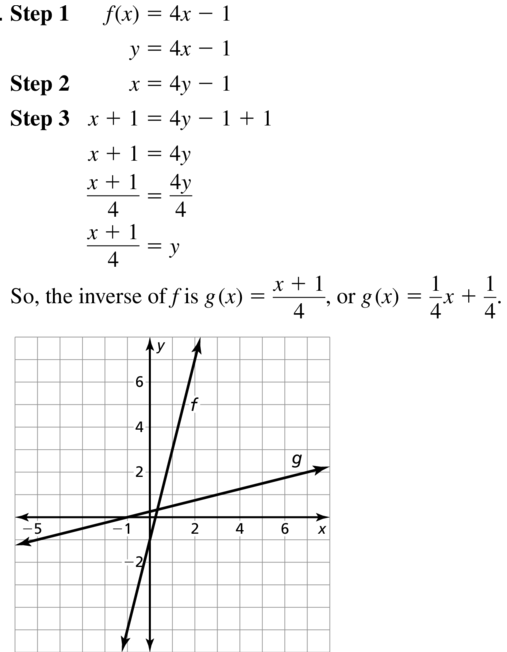
Question 18.
f(x) = -2x + 5
Answer:
f(x) = -2x + 5
y = -2x + 5
Interchange x and y
x = -2y + 5
x – 5 = -2y
(x – 5)/-2 = y
Finally replace y with f^-1 (x) and interchange the left and right side of the equation:
f^-1 (x) = –\(\frac{1}{2}\)x + \(\frac{5}{2}\)

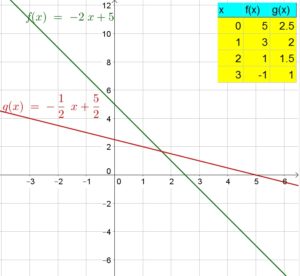
Question 19.
f(x) = -3x – 2
Answer:

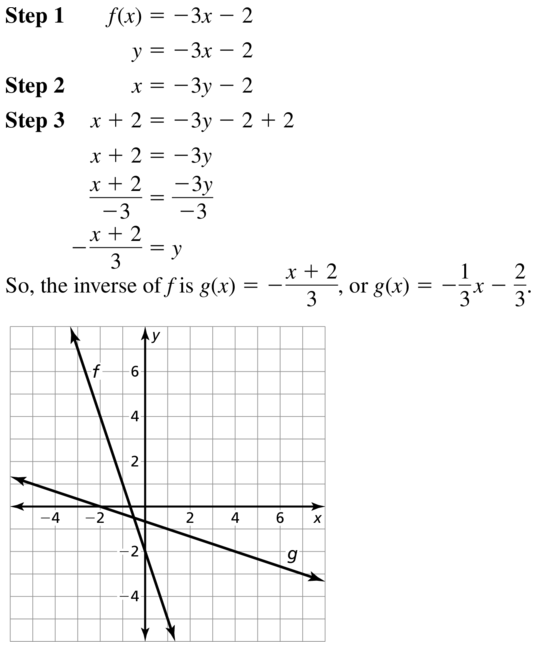
Question 20.
f(x) = 2x + 3
Answer:
Given,
f(x) = 2x + 3
y = 2x + 3
Replace x and y
x = 2y + 3
x – 3 = 2y
y = (x – 3)/2
g(x) = (x – 3)/2
= \(\frac{1}{2}\)x – \(\frac{3}{2}\)

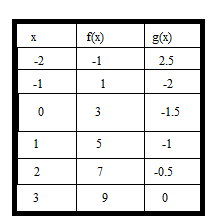
Based on the graphs, we see that the inverse function is symmetric with respect to the line x = y

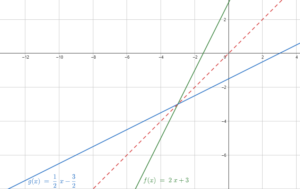
Question 21.
f(x) =\(\frac{1}{3}\)x + 8
Answer:
Given,
f(x) =\(\frac{1}{3}\)x + 8
Interchange x and y
x = \(\frac{1}{3}\)y + 8
x – 8 = \(\frac{1}{3}\)y
3(x – 8) = y
f^-1 (x) = 3(x – 8) = 3x – 24

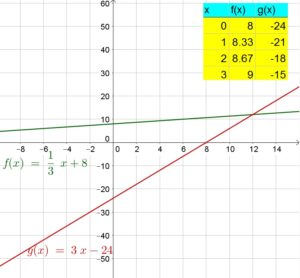
Question 22.
f(x) = – \(\frac{3}{2}\)x + \(\frac{7}{2}\)
Answer:
Given,
f(x) = – \(\frac{3}{2}\)x + \(\frac{7}{2}\)
y = – \(\frac{3}{2}\)x + \(\frac{7}{2}\)
x = – \(\frac{3}{2}\)y + \(\frac{7}{2}\)
x – \(\frac{7}{2}\) = –\(\frac{3}{2}\)y
–\(\frac{2}{3}\)(x – \(\frac{7}{2}\)) = y
f^-1 (x) =-\(\frac{2}{3}\)(x – \(\frac{7}{2}\))

In Exercises 23–28, find the inverse of the function. Then graph the function and its inverse.
Question 23.
f(x) = 4x², x ≥ 0
Answer:

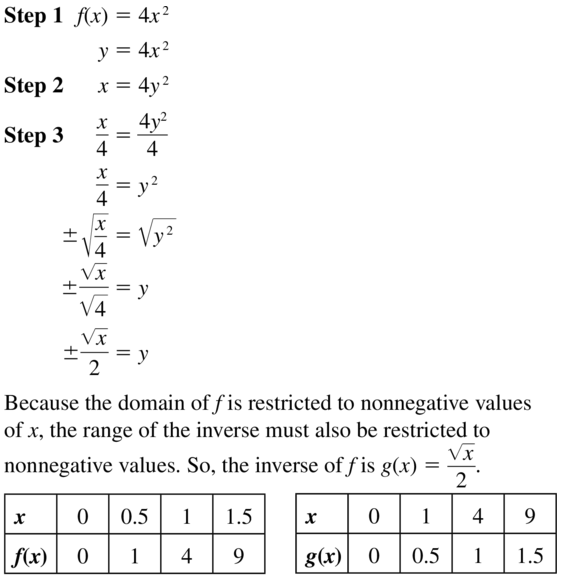
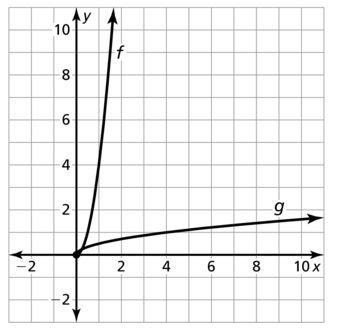
Question 24.
f(x) = \(\frac{2}{25}\)x², x ≤ 0
Answer:
Set y = f(x)
y = –\(\frac{1}{25}\)x²
x = –\(\frac{1}{25}\)y²
-25x = y²
±5\(\sqrt{-x}\) = y
Since the domain of f is restricted to x ≤ 0, then the range of the inverse must also be restricted to y ≤ 0
g(x) = -5\(\sqrt{-x}\)
The function and its inverse are reflections of each other about the line y = x.

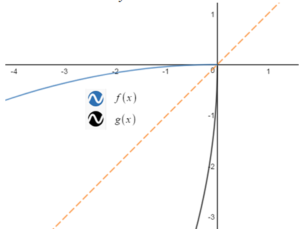
Question 25.
f(x) = -x² + 10, x ≤ 0
Answer:

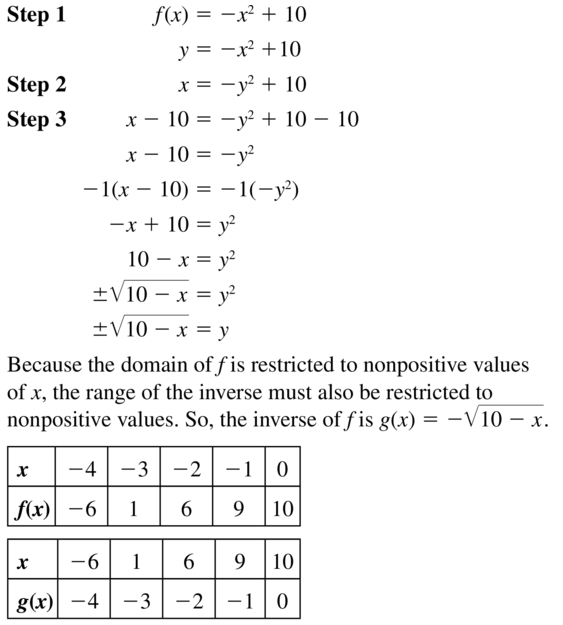
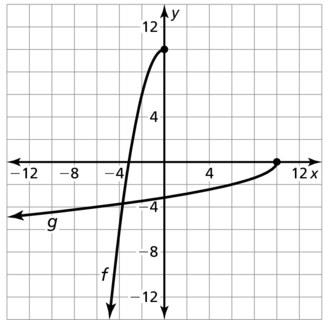
Question 26.
f(x) = 2x² + 6, x ≥ 0
Answer:
y = f(x)
y = 2x² + 6
x = 2y² + 6
x – 6 = 2y²
(x – 6)/2 = y²
± \(\sqrt{x-6/2}\) = y
Since the domain of f is restricted to x ≥ 0, then the range of the inverse must also be restricted to y ≥ 0
g(x) = \(\sqrt{x-6/2}\)
The function and its inverse are reflections of each other about the line y = x.

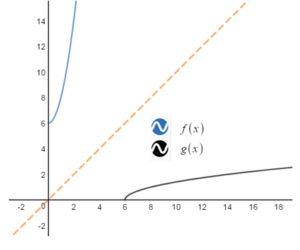
Question 27.
f(x) = \(\frac{1}{9}\)x² + 2, x ≥ 0
Answer:

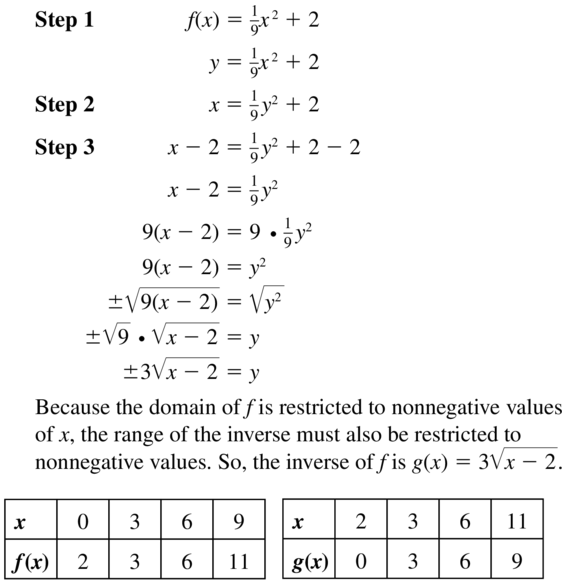
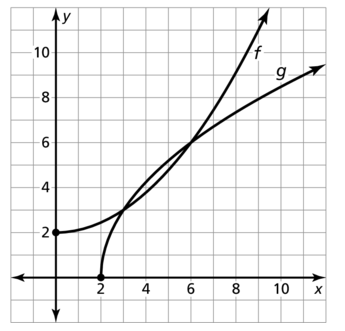
Question 28.
f(x) = -4x² – 8, x ≤ 0
Answer:
y = f(x)
f(x) = -4x² – 8
y = -4x² – 8
x = -4y² – 8
x + 8 = -4y²
–\(\frac{x}{4}\) – 2 = y²
±√-\(\frac{x}{4}\) – 2 = y
Since the domain of f is restricted to x ≥ 0, then the range of the inverse must also be restricted to y ≥ 0
Thus the inverse is
g(x) =- √-\(\frac{x}{4}\) – 2
The function and its inverse are reflections of each other about the line y = x.

In Exercises 29–32, use the Horizontal Line Test to determine whether the inverse of f is a function.
Question 29.

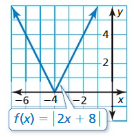
Answer:

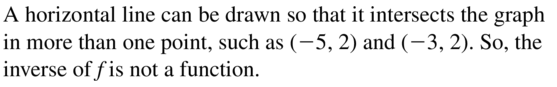
Question 30.

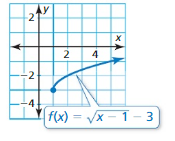
Answer:
The below is the graph of the function:
Because no horizontal line intersects the graph more than once, the inverse is a function.

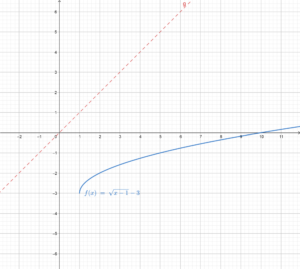
Question 31.

Answer:

Question 32.

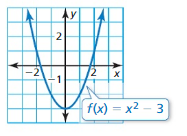
Answer:
The below is the graph of the function:
Because there are horizontal lines that intersect the graph more than once, the inverse is not a function.

In Exercises 33–42, determine whether the inverse of f is a function. Then find the inverse.
Question 33.
f(x) = \(\sqrt{x+3}\)
Answer:

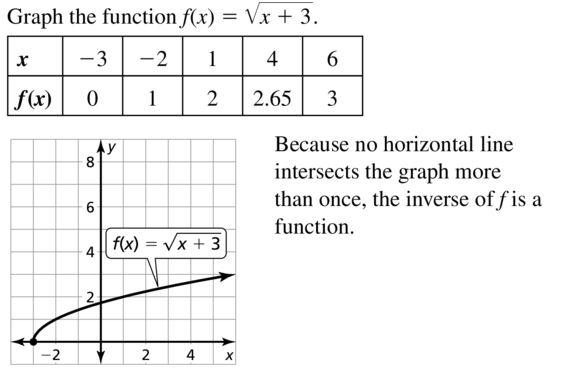
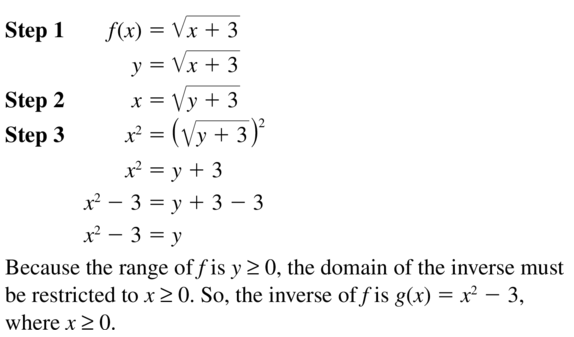
Question 34.
f(x) = \(\sqrt{x-5}\)
Answer:
Graph the function f, because no horizontal line intersects the graph more than once, the inverse of f is a function.

y = f(x)
y = \(\sqrt{x-5}\)
x = \(\sqrt{y-5}\)
Squaring on both sides
x² = y – 5
x² + 5 = y
Since the range of f is restricted to y ≥ 0, then the domain of the inverse must also be restricted to x ≥ 0.
Therefore the inverse is
g(x) = x² + 5, x ≥ 0

Question 35.
f(x) = \(\sqrt{2x-6}\)
Answer:

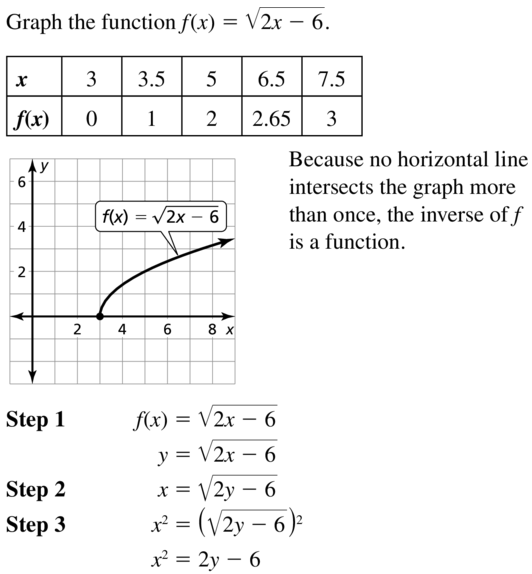
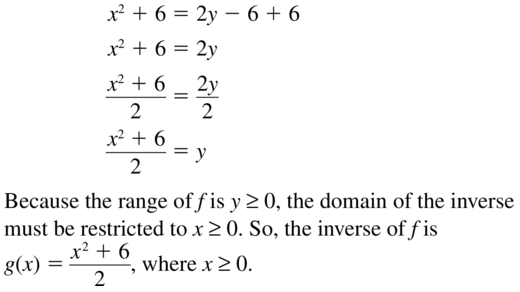
Question 36.
f(x) = \(\sqrt{4x+1}\)
Answer:
Graph the function f, because no horizontal line intersects the graph more than once, the inverse of f is a function.
y = \(\sqrt{4x+1}\)
x = \(\sqrt{4y+1}\)
Squaring on both sides
x² = 4y + 1
x² – 1 = 4y
y = \(\frac{x²}{4}\) – \(\frac{1}{4}\)
Since the range of f is restricted to y ≥ 0, then the domain of the inverse must also be restricted to x ≥ 0.
Thus the inverse is
g(x) = \(\frac{x²}{4}\) – \(\frac{1}{4}\), where x ≥ 0.

Question 37.
f(x) = 3\(\sqrt{x-8}\)
Answer:

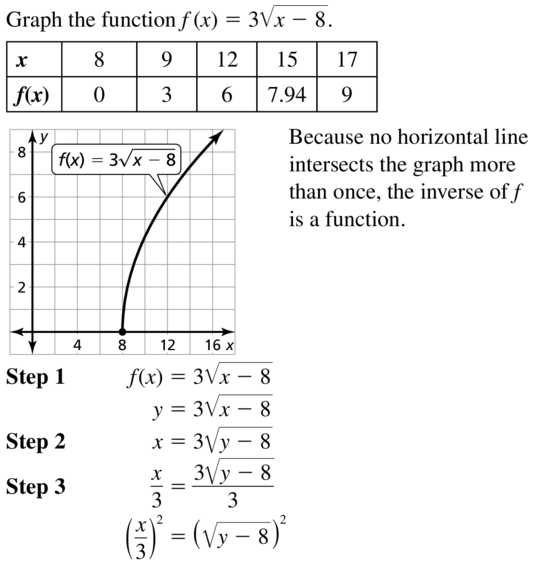
Question 38.
f(x) = –\(\frac{1}{4} \sqrt{5 x+2}\)
Answer:
The below is the graph of the function:
Because no horizontal line intersects the graph more than once, the inverse is a function.

y = f(x)
y = –\(\frac{1}{4} \sqrt{5 x+2}\)
x = –\(\frac{1}{4} \sqrt{5 y+2}\)
Squaring on both sides
x² = \(\frac{1}{16}\)(5y + 2)
16x² = 5y + 2
16x² – 2 = 5y
\(\frac{16x²-2}{5}\) = y
Since the range of f is restricted to y ≥ 0, then the domain of the inverse must also be restricted to x ≥ 0.
Thus the inverse is
g(x) = \(\frac{16x²-2}{5}\), where x ≤ 0

Question 39.
f(x) = –\(\sqrt{3x+5}\) – 2
Answer:

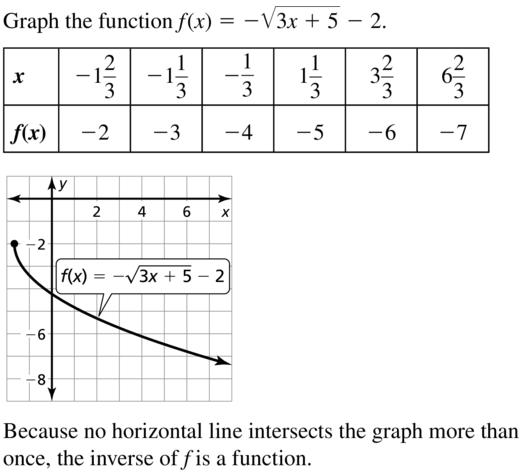
Question 40.
f(x) = 2\(\sqrt{x-7}\) + 6
Answer:
The below is the graph of the function:
Because no horizontal line intersects the graph more than once, the inverse is a function.

y = f(x)
f(x) = 2\(\sqrt{x-7}\) + 6
y = 2\(\sqrt{x-7}\) + 6
x = 2\(\sqrt{y-7}\) + 6
x – 6 = 2\(\sqrt{y-7}\)
Squaring on both sides
(x – 6)² = (2\(\sqrt{y-7}\))²
(x – 6)²/4 = y – 7
(x – 6)²/4 + 7 = y
Since the range of f is restricted to y ≥ 6, then the domain of the inverse must also be restricted to x≥ 6.
g(x) = (x – 6)²/4 + 7, where x ≥ 6

Question 41.
f(x) = 2x²
Answer:

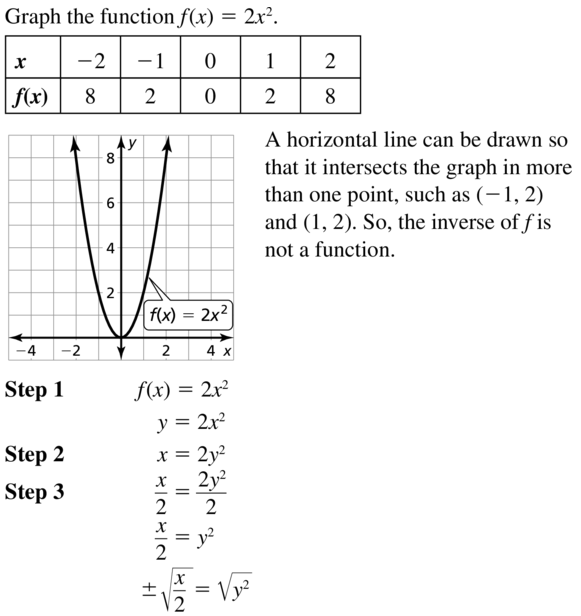
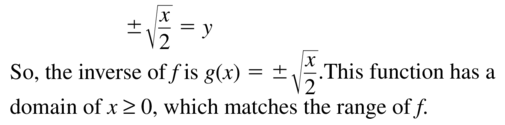
Question 42.
f(x) = |x|
Answer:
Graph the function, Because the horizontal lines intersect the graph more than once, the inverse of f is not a function.
Therefore, it has no inverse.

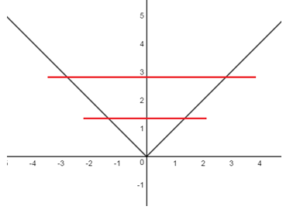
Question 43.
ERROR ANALYSIS
Describe and correct the error in finding the inverse of the function f(x) = – 3x + 5.

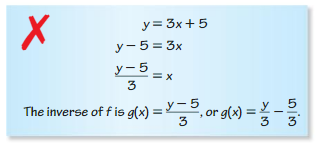
Answer:

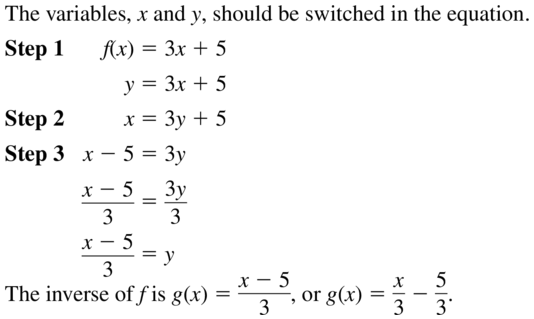
Question 44.
ERROR ANALYSIS
Describe and correct the error in finding and graphing the inverse of the function f(x) = \(\sqrt{x-3}\).

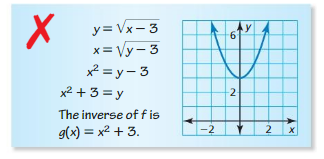
Answer:
Since the range of f is restricted to y ≥ 0, then the domain of the inverse must also be restricted to x ≥ 0.
Therefore, only the right half of the graph should be shown.

Question 45.
MODELING WITH MATHEMATICS
The euro is the unit of currency for the European Union. On a certain day, the number E of euros that could be obtained for D U.S. dollars was represented by the formula shown.
E = 0.74683D
Solve the formula for D. Then find the number of U.S. dollars that could be obtained for 250 euros on that day.
Answer:

Question 46.
MODELING WITH MATHEMATICS
A crow is flying at a height of 50 feet when it drops a walnut to break it open. The height h (in feet) of the walnut above ground can be modeled by h = -16t² + 50, where t is the time (in seconds) since the crow dropped the walnut. Solve the equation for t. After how many seconds will the walnut be 15 feet above the ground?

Answer:
Given,
h = -16t² + 50
h – 50 = -16t²
(h – 50)/-16 = t²
t = ±\(\sqrt{50-h}\)/\(\sqrt{16}\)
Since, we cannot have a negative time, then take the positive root and since the height goes from 50ft to 0ft, then the domain is 0 ≤ h ≤ 50.
Thus t = ±\(\sqrt{50-h}\)/\(\sqrt{16}\)
t = \(\sqrt{2.1875}\)
t ≈ 1.48 seconds

MATHEMATICAL CONNECTIONS In Exercises 47 and 48, s is the side length of an equilateral triangle. Solve the formula for s. Then evaluate the new formula for the given value.

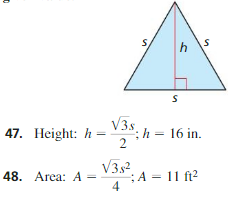
Answer:
47.

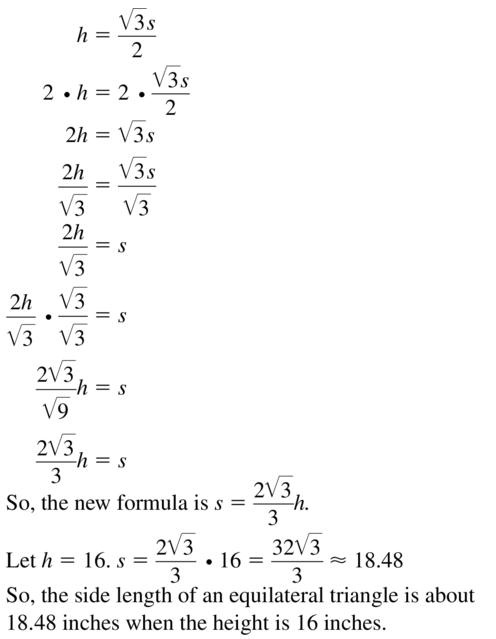
In Exercises 49–54, find the inverse of the function. Then graph the function and its inverse.
Question 49.
f(x) = 2x³
Answer:

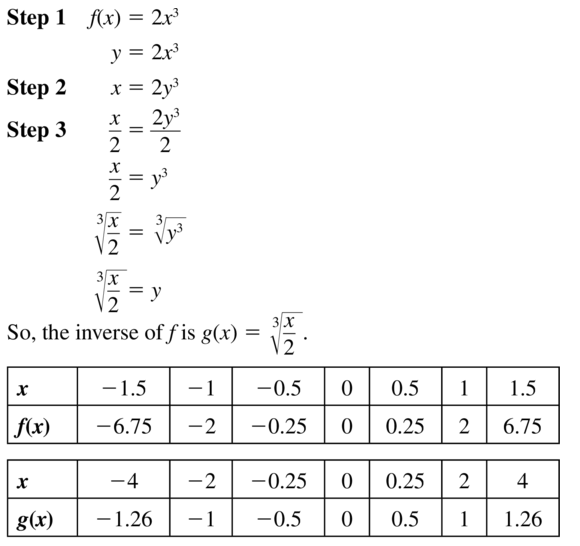
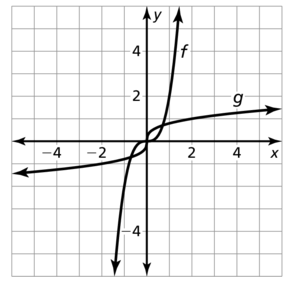
Question 50.
f(x) = x³ – 4
Answer:
y = f(x)
y = x³ – 4
x = = y³ – 4
x + 4 = y³
\(\sqrt [3]{ x+4}\) = y
g(x) = \(\sqrt [3]{ x+4}\)
The function and its inverse are reflections of each other about the line y = x

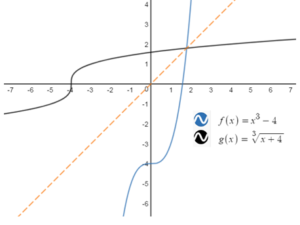
Question 51.
f(x) = (x – 5)³
Answer:

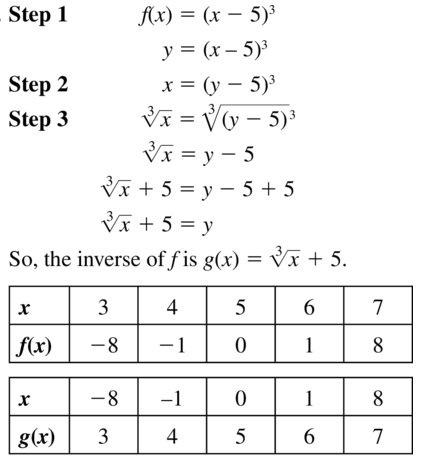
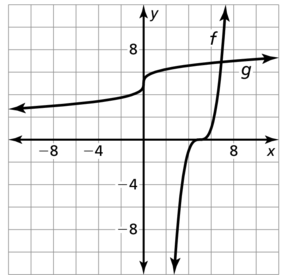
Question 52.
f(x) = 8(x + 2)³
Answer:
y = f(x)
y = 8(x + 2)³
x = 8(y + 2)³
\(\sqrt [3]{ x }\) = 2(y + 2)
\(\sqrt [3]{ x }\)/2 = y + 2
\(\sqrt [3]{ x }\)/2 – 2 = y
g(x) = \(\sqrt [3]{ x }\)/2 – 2
The function and its inverse are reflections of each other about the line y = x

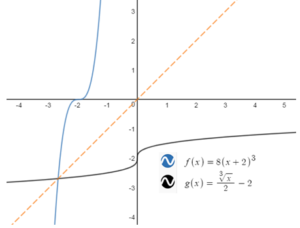
Question 53.
f(x) = 4\(\sqrt [3]{ x }\)
Answer:

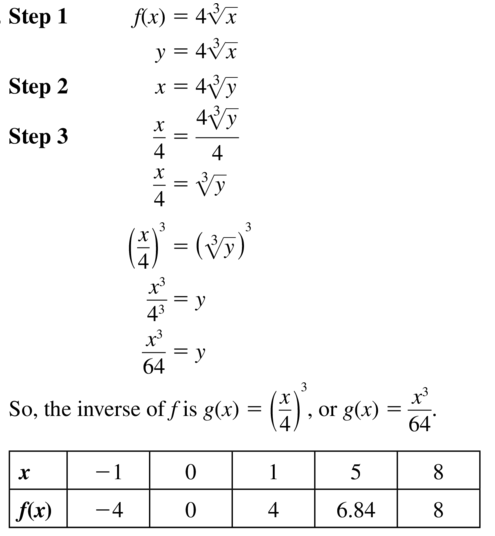
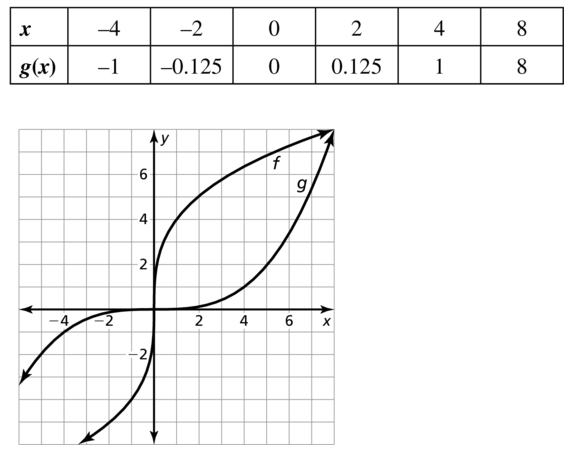
Question 54.
f(x) = –\(\sqrt [3]{ x-1 }\)
Answer:
Given,
f(x) = –\(\sqrt [3]{ x-1 }\)
y = f(x)
y = –\(\sqrt [3]{ x-1 }\)
x = –\(\sqrt [3]{ y-1 }\)
cubing on both sides
x³ = -(y – 1)
-x³ = y – 1
-x³ + 1 = y
g(x) = -x³ + 1
The function and its inverse are reflections of each other about the line y = x

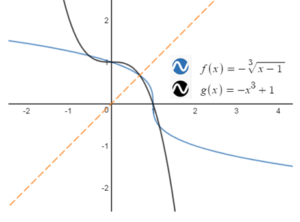
Question 55.
MAKING AN ARGUMENT
Your friend says that the inverse of the function f(x) = 3 is a function because all linear functions pass the Horizontal Line Test. Is your friend correct? Explain.
Answer:

Question 56.
HOW DO YOU SEE IT?
Pair the graph of each function with the graph of its inverse.

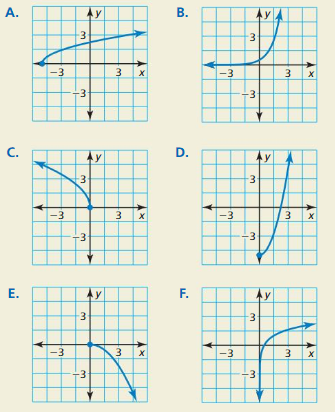
Answer:
The function and its inverse are reflections of each other about the line y = x
Therefore the pairs are A and D, B and F, C and E

Question 57.
WRITING
Describe changes you could make to the function f(x) = x² – 5 so that its inverse is a function. Describe the domain and range of the new function and its inverse.
Answer:

Question 58.
CRITICAL THINKING
Can an even function with at least two values in its domain have an inverse that is a function? Explain.
Answer:
No. Since the graphs of all even functions are reflections of the y-axis.
Thus it will immediately fail the horizontal line test. It can only have an inverse if its domain is restricted.

Question 59.
OPEN-ENDED
Write a function such that the graph of its inverse is a line with a slope of 4.
Answer:

Question 60.
CRITICAL THINKING
Consider the function g(x) = -x.
a. Graph g(x) = -x and explain why it is its own inverse.
Answer: We note that the graph of g is perpendicular to the line y = x and thus reflecting the graph of g will result in the same graph and thus the inverse function of g is g itself.
b. Graph other linear functions that are their own inverses. Write equations of the lines you graph.
Answer:
We note that every function parallel to the line y = -x is also its own inverse because the graph remains perpendicular to the line y = x. However also note that y = x will be its own inverse.

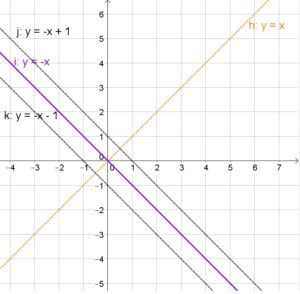
c. Use your results from part (b) to write a general equation that describes the family of linear functions that are their own inverses.
Answer:
Thus y = -x + c and y = x with c a constant are all functions that have itself as inverse.

Question 61.
REASONING
Show that the inverse of any linear function f(x) = mx + b, where m ≠ 0, is also a linear function. Write the slope and y-intercept of the graph of the inverse in terms of m and b.
Answer:

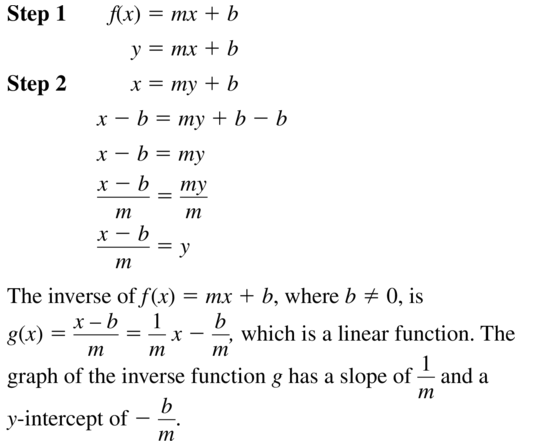
Question 62.
THOUGHT PROVOKING
The graphs of f(x) = x³ – 3x and its inverse are shown. Find the greatest interval -a ≤ x ≤ a for which the inverse of f is a function. Write an equation of the inverse function.

Answer:
Restricting the domain to -1 ≤ x ≤ 1 will make the graph of f(x) pass the horizontal line test.
Find the inverse:
y = f(x)
y = x³ – 3x
x = y³ – 3x
Since we cannot isolate y, then we cannot solve for the inverse function.

Question 63.
REASONING
Is the inverse of f(x) = 2|x + 1|a function? Are there any values of a, h, and k for which the inverse of f(x) = a |x – h| + k is a function? Explain your reasoning.
Answer:

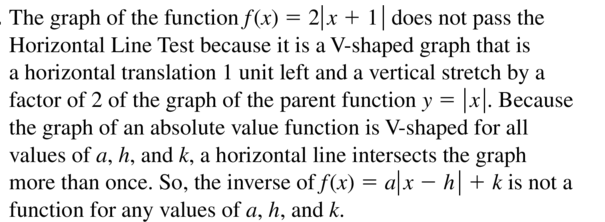
Maintaining Mathematical Proficiency

Find the sum or difference.(Section 7.1)
Question 64.
(2x – 9) – (6x + 5)
Answer:
Given,
(2x – 9) – (6x + 5)
2x – 9 – 6x – 5
add or subtract the like terms
2x – 6x – 9 – 5
-4x – 14

Question 65.
(8y + 1) + (-y – 12)
Answer:

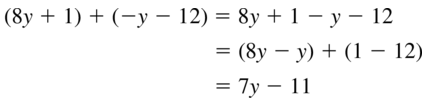
Question 66.
(t² – 4t – 4) + (7t² + 12t + 3)
Answer:
Given,
(t² – 4t – 4) + (7t² + 12t + 3)
t² – 4t – 4 + 7t² + 12t + 3
8t² + 8t -1

Question 67.
(-3d² + 10d – 8) – (7d² – d – 6)
Answer:

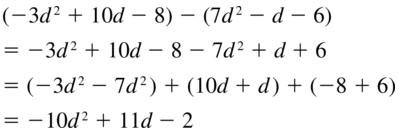
Graph the function. Compare the graph to the graph of f(x) = x². (Section 8.2)
Question 68.
g(x) = x² + 6
Answer:

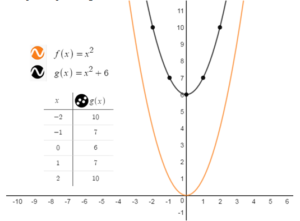
the graph of g(x) is the graph of f(x) that is vertically shifted 6 units upward

Question 69.
h(x) = -x² – 2
Answer:

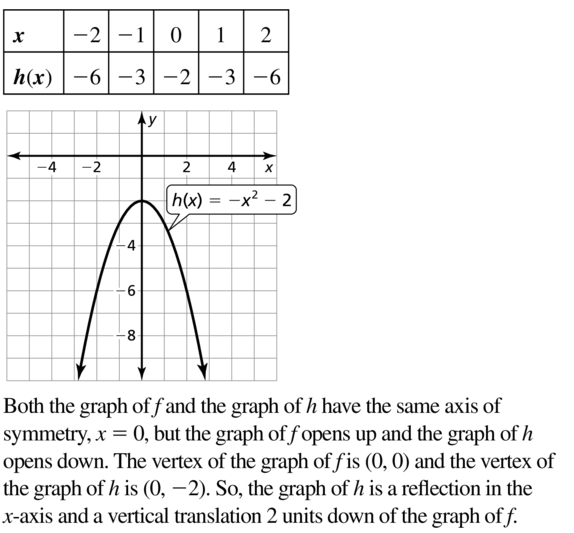
Question 70.
p(x) = -4x² + 5
Answer:

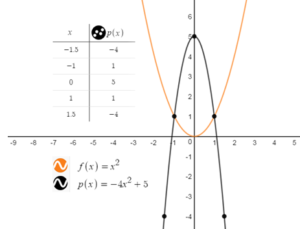
The graph of p(x) is the graph of f(x):
– Reflected in the x-axis
– Veertically stretched by a factor of 4
– Vertically shifted 5 units upward

Question 71.
q(x) = \(\frac{1}{3}\)x² – 1
Answer:

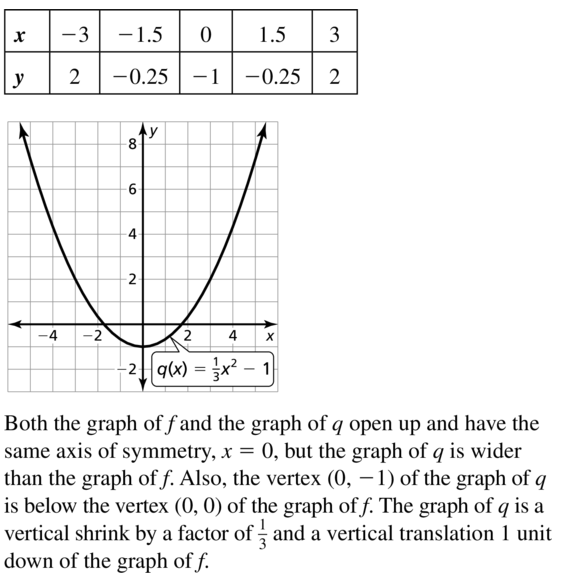
Radical Functions and Equations Performance Task: Medication and the Mosteller Formula

10.3–10.4What Did YouLearn?

Core Vocabulary
radical equation, p. 560
inverse relation, p. 568
inverse function, p. 569

Core Concepts
Lesson 10.3
Squaring Each Side of an Equation, p. 560
Identifying Extraneous Solutions, p. 562

Lesson 10.4
Inverse Relation, p. 568
Finding Inverses of Functions Algebraically, p. 570
Finding Inverses of Nonlinear Functions, p. 570
Horizontal Line Test, p. 571

Mathematical Practices
Question 1.
Could you also solve Exercises 37–44 on page 565 by graphing? Explain.
Answer:

Question 2.
What external resources could you use to check the reasonableness of your answer in Exercise 45 on page 573?
Answer:

Performance Task: Medication and the Mosteller Formula

When taking medication, it is critical to take the correct dosage. For children in particular, body surface area (BSA) is a key component in calculating that dosage. The Mosteller Formula is commonly used to approximate body surface area. How will you use this formula to calculate BSA for the optimum dosage?
To explore the answers to this question and more, go to

Radical Functions and Equations Chapter Review

10.1 Graphing Square Root Functions (pp. 543–550)

Graph the function. Describe the domain and range. Compare the graph to the graph of f(x) = \(\sqrt{x}\).
Question 1.
g(x) = \(\sqrt{x}\) + 7
Answer:
Step 1: Use the domain to make a table of values
The radicand must be greater than or equal to 0
x ≥ 0
Step 2: Plot the ordered pairs
Step 3: Draw a smooth curve through the points starting at (0, 7)

From the graph, we can see that the range is
y ≥ 1
The graph of g(x) is the graph of f(x) is vertically shifted 7 units upwards.

Question 2.
h(x) = \(\sqrt{x-6}\)
Answer:
Step 1: Use the domain to make a table of values
The radicand must be greater than or equal to 0
x – 6 ≥ 0
x ≥ 6
Step 2: Plot the ordered pairs
Step 3: Draw a smooth curve through the points starting at (6, 0)

From the graph, we can see that the range is
y ≥ 0
The graph of h(x) is the graph of f(x) is horizontally shifted 6 units to the right.

Question 3.
r(x) = –\(\sqrt{x+3}\) – 1
Answer:
Step 1: Use the domain to make a table of values
The radicand must be greater than or equal to 0
x + 3 ≥ 0
x ≥ -3
Step 2: Plot the ordered pairs
Step 3: Draw a smooth curve through the points starting at (-3, -1)

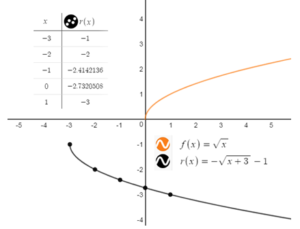
From the graph, we can see that the range is
y ≤ -1
The graph of r(x) is the graph of f(x) is
– Reflected in the x-axis
– Horizontally shifted 3 units to the left
– Vertically shifted 1 unit downward

Question 4.
Let g(x) = \(\frac{1}{4} \sqrt{x-6}\) + 2. Describe the transformations from the graph of f(x) = \(\sqrt{x}\) to the graph of g. Then graph g.
Answer:
The graph of g(x) is the graph of f(x) is
– Vertically shrinked by a factor of 1/4
– Horizontally shifted 6 units to the right
– Vertically shifted 2 units upward
Step 1: Use the domain to make a table of values
The radicand must be greater than or equal to 0
x – 6 ≥ 0
x ≥ 6
Step 2: Plot the ordered pairs
Step 3: Draw a smooth curve through the points starting at (6, 2)

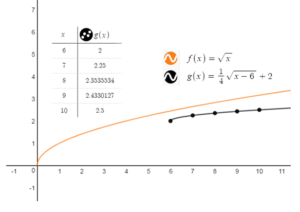
10.2 Graphing Cube Root Functions (pp. 551–556)

Graph the function. Compare the graph to the graph of f(x) = \(\sqrt [3]{ x }\).
Question 5.
g(x) = \(\sqrt [3]{ x }\) + 4
Answer:
Step 1: Make a table of values.
Step 2: Plot the ordered pairs.
Step 3: Draw a smooth curve through the points.

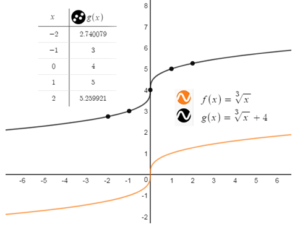
The graph of g(x) is the graph of f(x) is vertically shifted 4 units upward.

Question 6.
h(x) = -8\(\sqrt [3]{ x }\)
Answer:
Step 1: Make a table of values.
Step 2: Plot the ordered pairs.
Step 3: Draw a smooth curve through the points.

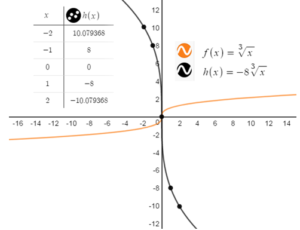
The graph of h(x) is the graph of f(x) is
– Reflected in the x-axis
– Horizontally stretched by a factor of 8
– Vertically shifted 6 unit downward

Question 7.
s(x) = \(\sqrt[3]{-2(x-3)}\)
Answer:
Step 1: Make a table of values.
Step 2: Plot the ordered pairs.
Step 3: Draw a smooth curve through the points.

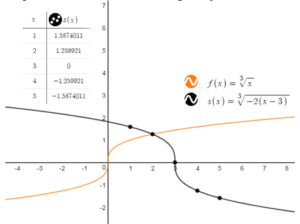
The graph of s(x) is the graph of f(x) is
– Reflected in the y-axis
– Horizontally stretched by a factor of 1/2
– Horizontally shifted 3 unit to the right

Question 8.
Let g(x) = -3\(\sqrt [3]{ x+2 }\) – 1. Describe the transformations from the graph of f(x) = \(\sqrt [3]{ x }\) to the graph of g. Then graph g.
Answer:
Step 1: Make a table of values.
Step 2: Plot the ordered pairs.
Step 3: Draw a smooth curve through the points.

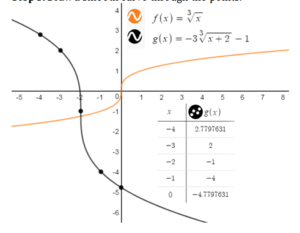
Question 9.
The graph of cube root function r is shown. Compare the average rate of change of r to the average rate of change of p(x) = \(\sqrt[3]{\frac{1}{2} x}\) over the interval x = 0 to x = 8.
Answer:
Use (0, 0) and (8, 5)
The average rate of change is
r(8) – r(0)/8-0 = (5 -0)/(8 – 0) = 0.625
For p(x) = \(\sqrt[3]{\frac{1}{2} x}\)
Evaluate g when x = 0 and x = 8
p(0) = \(\sqrt[3]{\frac{1}{2} (0)}\) = 0
p(8) = \(\sqrt[3]{\frac{1}{2} (8)}\) = \(\sqrt [3]{ 4 }\)
The average rate of change is
p(8) – p(0)/8-0 = (\(\sqrt [3]{ 4 }\)  -0)/(8 – 0) = 0.2
The average rate of change of r is 0.625/0.2 = 3.1 times greater than the average rate of change of p over the interval x = 0 to x = 8.

10.3 Solving Radical Equations (pp. 559-566)

Solve the equation. Check your solution(s).
Question 10.
8 + \(\sqrt{x}\) = 18
Answer:
Given,
8 + \(\sqrt{x}\) = 18
\(\sqrt{x}\) = 18 – 8
\(\sqrt{x}\) = 10
Squaring on both sides
x = 10² = 100
Check:
8 + \(\sqrt{x}\) = 18
8 + \(\sqrt{100}\) = 18
8 + 10 =18
Thus x = 100 is the solution

Question 11.
\(\sqrt [3]{ x-1 }\) = 3
Answer:
Given,
\(\sqrt [3]{ x-1 }\) = 3
Cubing on both sidees
x – 1 = 3³ = 27
x – 1 = 27
x = 27 + 1
x = 28
Check:
\(\sqrt [3]{ x-1 }\) = 3
\(\sqrt [3]{ 28-1 }\) = 3
\(\sqrt [3]{ 27 }\) = 3
3 = 3
Thus x = 28 is the solution

Question 12.
\(\sqrt{5x-9}\) = \(\sqrt{4x}\)
Answer:
Given,
\(\sqrt{5x-9}\) = \(\sqrt{4x}\)
squaring on both sides
5x – 9 = 4x
5x – 4x = 9
x = 9

Question 13.
x = \(\sqrt{3x+4}\)
Answer:
Given,
x = \(\sqrt{3x+4}\)
squaring on both sides
x² = 3x + 4
x² – 3x + 4 = 0
(x – 1) (x – 4) = 0
x = 1 or x = 4

Question 14.
8\(\sqrt{x-5}\) + 34 = 58
Answer:
Given,
8\(\sqrt{x-5}\) + 34 = 58
8\(\sqrt{x-5}\) = 58 – 34
8\(\sqrt{x-5}\) = 24
\(\sqrt{x-5}\) = 24/8
\(\sqrt{x-5}\) = 3
squaring on both sides
x – 5 = 9
x = 9 + 5
x = 14

Question 15.
\(\sqrt{5x}\) + 6 = 5
Answer:
Given,
\(\sqrt{5x}\) + 6 = 5
\(\sqrt{5x}\) = 5 – 6
\(\sqrt{5x}\) = -1
squaring on both sides
5x = 1
x = 1/5

Question 16.
The radius r of a cylinder is represented by the function r = \(\sqrt{\frac{V}{\pi h}}\), where V is the volume and h is the height of the cylinder. What is the volume of the cylindrical can?

Answer:
r = \(\sqrt{\frac{V}{\pi h}}\)
r = 2 in
2 = \(\sqrt{\frac{V}{\pi 4}}\)
4 = \(\frac{V}{4π}\)
16π = V
V ≈ 50.3 cu. in

10.4 Inverse of a Function (pp. 567–574)

Find the inverse of the relation.
Question 17.
(1, -10), (3, -4), (5, 4), (7, 14), (9, 26)
Answer:
Switch the coordinates of each ordered pairs
(1, -10), (3, -4), (5, 4), (7, 14), (9, 26)
(-10, 1), (-4, 3), (4, 5), (14, 7), (26, 9)

Question 18.

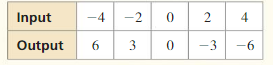
Answer:
Switch the coordinates of each ordered pairs

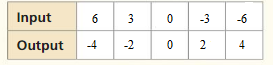
Find the inverse of the function. Then graph the function and its inverse.
Question 19.
f(x) = -5x + 10
Answer:
y = f(x)
y = -5x + 10
x = -5y + 10
x – 10 = -5y
-x/5 + 2 = y
g(x) = -x/5 + 2
The function and its inverse are reflections of each other about the line y = x

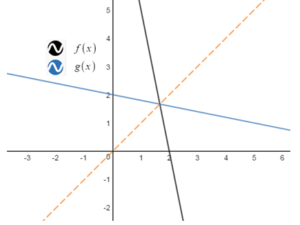
Question 20.
f(x) = 3x² – 1, x ≥ 0
Answer:
y = f(x)
y = 3x² – 1
x = 3y² – 1
x + 1 = 3y²
(x + 1)/3 = y²
y = ± \(\sqrt{\frac{x+1}{\3}}\)
Since the domain of f is restricted to x ≥ 0, then the range of the inverse must also be restricted to y ≥ 0.
Therefore, the inverse is
g(x) = \(\sqrt{x}\)/2
The function and its inverse are reflections of each other about the line y = x

Question 21.
f(x) = \(\frac{1}{2} \sqrt{2 x+6}\)
Answer:
y = f(x)
y = \(\frac{1}{2} \sqrt{2 x+6}\)
x = \(\frac{1}{2} \sqrt{2 y+6}\)
2x = \(\sqrt{2 y+6}\)
Squaring on both sides
4x² = 2y + 6
4x² – 6 = 2y
Taking 2 as a common number
2x² – 3 =y
y = 2x² – 3
Since the range of f is restricted to y ≥ 0, then the domain of the inverse must also be restricted to x ≥ 0.
Therefore the inverse is
g(x) = 2x² – 3
The function and its inverse are reflections of each other about the line y = x

Question 22.
Consider the function f(x) = x² + 4. Use the Horizontal Line Test to determine whether the inverse of f is a function.
Answer:
The inverse of a function f is also a function if and only if no horizontal line intersects the graph of f more than once.

Since the horizontal lines intersect the graph of f more than once, then the inverse of f is not a function.

Question 23.
In bowling, a handicap is an adjustment to a bowler’s score to even out differences in ability levels. In a particular league, you can find a bowler’s handicap h by using the formula h = 0.8(210 – a), where a is the bowler’s average. Solve the formula for a. Then find a bowler’s average when the bowler’s handicap is 28.

Answer:
Given,
h = 0.8(210 – a)
h/0.8 = 210 – a
h/0.8 – 210 = -a
a = -h/0.8 + 210
substitute h = 28 and then find a
a = -28/0.8 + 210
a = 175

Radical Functions and Equations Chapter Test

Find the inverse of the function.
Question 1.
f(x) = 5x – 8
Answer:
f(x) = 5x – 8
y = 5x – 8
y + 8 = 5x
x = (y + 8)/5
Thus f^-1(x) = (y + 8)/5

Question 2.
f(x) = 2\(\sqrt{x+3}\) – 1
Answer:
f(x) = 2\(\sqrt{x+3}\) – 1
y = 2\(\sqrt{x+3}\) – 1
y + 1 = 2\(\sqrt{x+3}\)
(y + 1)/2 = \(\sqrt{x+3}\)
[(y + 1)/2]² = x + 3
[(y + 1)/2]² – 3 = x
Thus f^-1(x) = [(x + 1)/2]² – 3

Question 3.
f(x) = –\(\frac{1}{3}\)x² + 4, x ≥ 0
Answer:
y = f(x)
f(x) = –\(\frac{1}{3}\)x² + 4
y = –\(\frac{1}{3}\)x² + 4
x = –\(\frac{1}{3}\)y² + 4
x – 4 = –\(\frac{1}{3}\)y²
-3x + 12 = y²
y = \(\sqrt{-3x+12}\)
Since the domain of f is restricted to x ≤ 0, then the range of the inverse must also be restricted to y ≤ 0
g(x) = \(\sqrt{-3x+12}\)
The function and its inverse are reflections of each other about the line y = x.

Graph the function f. Describe the domain and range. Compare the graph of f to the graph of g.
Question 4.
f(x) = –\(\sqrt{x+6}\); g(x) = \(\sqrt{x}\)
Answer:
Step 1: Use the domain to make a table of values.
The radicand must be greater than or equal to 0
x + 6 ≥ 0
x ≥ -6
Step 2: Plot the ordered pairs
Step 3: Draw a smooth curve through the points starting at (-6, 0)

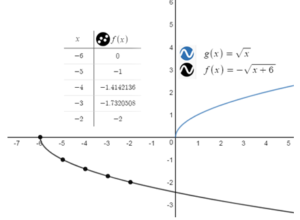
From the graph, we can see that the range is y ≥ 0
The graph of f(x) is the graph of g(x) is
– Reflected in the x-axis
– Horizontally shifted 6 units to the left

Question 5.
f(x) = \(\sqrt{x-3}\) + 2; g(x) = \(\sqrt{x}\)
Answer:
Step 1: Use the domain to make a table of values.
The radicand must be greater than or equal to 0
x – 3 ≥ 0
x ≥ 3
Step 2: Plot the ordered pairs
Step 3: Draw a smooth curve through the points starting at (3, 2)

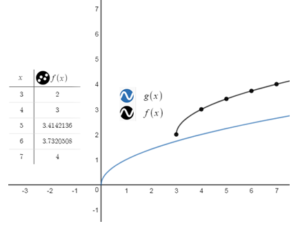
From the graph, we can see that the range is y ≥ 2
The graph of f(x) is the graph of g(x) is
– Horizontally shifted 3 units to the right
– Vertically shifted 2 units upward

Question 6.
f(x) = \(\sqrt [3]{ x }\) – 5; g(x) = \(\sqrt [3]{ x }\)
Answer:
The domain and range of a cubic function are all real numbers.
Step 1: Use the domain to make a table of values.
Step 2: Plot the ordered pairs
Step 3: Draw a smooth curve through the points.

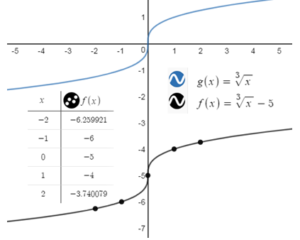
The graph of f(x) is the graph of g(x)
– Vertically shifted 5 units downward

Question 7.
f(x) = -2\(\sqrt [3]{ x+1 }\); g(x) = \(\sqrt [3]{ x }\)
Answer:
The domain and range of a cubic function are all real numbers.
Step 1: Use the domain to make a table of values.
Step 2: Plot the ordered pairs
Step 3: Draw a smooth curve through the points.

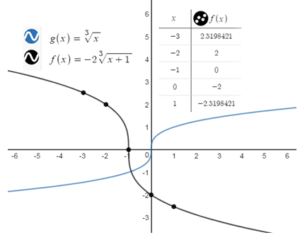
The graph of f(x) is the graph of g(x)
– Reflected in the x-axis
– Vertically stretched by a factor of 2
– Horizontally shifted 1 unit to the right

Solve the equation. Check your solution(s).
Question 8.
9 – \(\sqrt{x}\) = 3
Answer:
Given,
9 – \(\sqrt{x}\) = 3
9 – 3 = \(\sqrt{x}\)
6 = \(\sqrt{x}\)
squaring on both sides
36 = x
check:
9 – \(\sqrt{x}\) = 3
9 – \(\sqrt{36}\) = 3
9 – 6 = 3
Thus x = 36 is the solution

Question 9.
\(\sqrt{2x-7}\) – 3 = 6
Answer:
Given,
\(\sqrt{2x-7}\) – 3 = 6
\(\sqrt{2x-7}\) = 6 + 3
\(\sqrt{2x-7}\) = 9
squaring on both sides
2x – 7 = 9²
2x – 7 = 81
2x = 81 + 7
2x = 88
x = 44
Check:
\(\sqrt{2(44)-7}\) – 3 = 6
\(\sqrt{81}\) – 3 = 6
9 – 3 = 6
6 = 6
Thus x = 44 is the solution

Question 10.
\(\sqrt{8x-21}\) = \(\sqrt{18-5x}\)
Answer:
Given,
\(\sqrt{8x-21}\) = \(\sqrt{18-5x}\)
squaring on both sides
8x – 21 = 18 – 5x
8x + 5x = 18 + 21
13x = 39
x = 3
Check:
\(\sqrt{8x-21}\) = \(\sqrt{18-5x}\)
\(\sqrt{8(3)-21}\) = \(\sqrt{18-5(3)}\)
\(\sqrt{3}\) = \(\sqrt{3}\)
Thus x = 3 is the solution

Question 11.
x + 5 = \(\sqrt{7x+53}\)
Answer:
Given,
x + 5 = \(\sqrt{7x+53}\)
(x + 5)² = 7x + 53
x² + 10x + 25 = 7x + 53
x² + 10x + 25 – 7x – 53 = 0
x² + 3x – 28 = 0
(x + 7)(x – 4) = 0
x + 7 = 0 or x – 4 = 0
x = -7 or x = 4

Question 12.
When solving the equation x – 5 = \(\sqrt{ax+b}\), you obtain x = 2 and x = 8. Explain why at least one of these solutions must be extraneous.
Answer:
x = 2 is an extraneous solution because the left side will be negative but the right side will be positive.

Describe the transformations from the graph of f(x) = \(\sqrt [3]{ x }\) to the graph of the given function. Then graph the given function.
Question 13.
h(x) = 4\(\sqrt [3]{ x-1 }\) + 5
Answer:
Step 1: Use the domain to make a table of values.
Step 2: Plot the ordered pairs
Step 3: Draw a smooth curve through the points.

Question 14.
w(x) = –\(\sqrt [3]{ x+7 }\) – 2
Answer:
Step 1: Use the domain to make a table of values.
Step 2: Plot the ordered pairs
Step 3: Draw a smooth curve through the points.

Question 15.
The velocity v (in meters per second) of a roller coaster at the bottom of a hill is given by v = \(\sqrt{19.6h}\), where h is the height (in meters) of the hill.
(a) Use a graphing calculator to graph the function. Describe the domain and range.
Answer:

Domain: h ≥ 0
Range: v ≥ 0

(b) How tall must the hill be for the velocity of the roller coaster at the bottom of the hill to be at least 28 meters per second?
Answer:
v = 28 meters
28 = \(\sqrt{19.6h}\)
Squaring on both sides
784 = 19.6h
h = 40 meters

(c) What happens to the average rate of change of the velocity as the height of the hill increases?
Answer: The average rate of change of the velocity decreases as the height of the hill increases.

Question 16.
The speed s (in meters per second) of sound through air is given by s = 2\(\sqrt{T+273}\), where T is the temperature (in degrees Celsius).

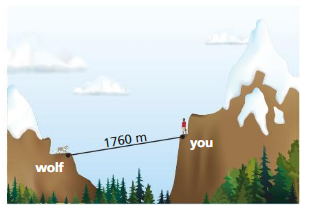
a. What is the temperature when the speed of sound through air is 340 meters per second?
Answer:
s = 340
340 = 20\(\sqrt{T+273}\)
17 = \(\sqrt{T+273}\)
289 = T + 273
T = 16(16°C)
b. How long does it take you to hear the wolf howl when the temperature is -17°C?
Answer:
s = 20\(\sqrt{T+273}\)
s = \(\sqrt{-17+273}\)
s = \(\sqrt{256}\)
s = 20(16)
s = 320
Divide the distance by the speed to find the time
t = d/s = 1760/320
t = 5.5 seconds

Question 17.
How can you restrict the domain of the function f(x) = (x – 3)² so that the inverse of f is a function?
Answer:
We can restrict the domain by taking half of the parabola which occurs at the vertex.
In this case, the vertex is at (3, 0)
Therefore we can restrict the domain as x ≤ 3 or x ≥ 3 for the inverse of f to be a function.

Question 18.
Write a radical function that has a domain of all real numbers less than or equal to 0 and a range of all real numbers greater than or equal to 9.
Answer:
From the statement, the domain is x ≤ 0 which means the function contains the radical \(\sqrt{x}\) and is reflected in the y-axis: \(\sqrt{-x}\)
The range is y ≥ 9 means that this radical is vertically shifted 9 units upward so that the function will be
f(x) = \(\sqrt{-x}\) + 9

Radical Functions and Equations Cumulative Assessment

Question 1.
Fill in the function so that it is represented by the graph.

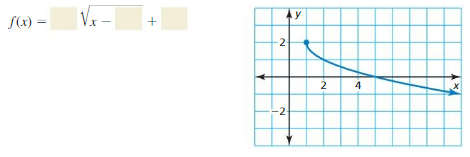
Answer:
The graph of f(x) is the graph of the parent function y = \(\sqrt{x}\) that is
– Reflected in the x-axis → –\(\sqrt{x}\)
– Horizontally shifted 1 unit to the right → –\(\sqrt{x-1}\)
– Vertically shifted 2 units upward → –\(\sqrt{x-1}\) + 2
Thus the function is –\(\sqrt{x-1}\) + 2

Question 2.
Consider the equation y = mx + b. Fill in values for m and b so that each statement is true.
a. When m = ______ and b = ______, the graph of the equation passes through the point (-1, 4).
Answer:
Write any slope say m = 1 and solve for b by using the slope intercept form
y = mx + b
Now substitute slope and given point
4 = 1(-1) + b
4 = -1 + b
Therefore m = 1, b = 5
b. When m = ______ and b = ______, the graph of the equation has a positive slope and passes through the point (-2, -5).
Answer:
Write any slope say m = 1 and solve for b by using the slope intercept form
y = mx + b
Now substitute slope and given point
-5 = 1(-2) + b
-5 = -2 + b
b = -3
Therefore m = 1, b = -3
c. When m = ______ and b = ______, the graph of the equation is perpendicular to the graph of y = 4x – 3 and passes through the point (1, 6).
Answer:
The lines are perpendicular if their slopes are negative reciprocal of each other.
Thus m = -1/4
Solve for b by using the slope intercept form
y = mx + b
Now substitute slope and given point
6 = 1(-1/4) + b
6 = -1/4 + b
b = 25/4
Therefore m = -1/4, b = 25/4

Question 3.
Which graph represents the inverse of the function f(x) = 2x + 4?

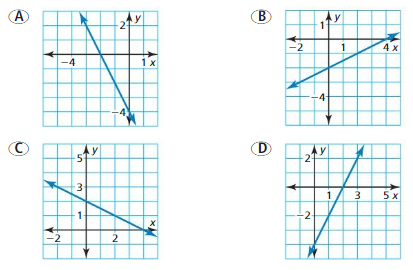
Answer:
y = f(x)
y = 2x + 4
x = 2y + 4
x – 4 = 2y
x/2 – 2 = y
The graph where m = 1/2 and b = -2 is option b

Question 4.
Consider the equation x = \(\sqrt{ax+b}\). Student A claims this equation has one real solution. Student B claims this equation has two real solutions. Use the numbers to answer parts (a)–(c).

a. Choose values for a and b to create an equation that supports Student A’s claim.
Given,
x = \(\sqrt{ax+b}\)
Squaring on both sides
x² = \(\sqrt{ax+b}\)²
x² = ax + b
x² – ax – b = 0
There is one real solution if b² – 4ac = 0
(-a)² – 4(1)(-b) = 0
a² + 4b = 0
The possible answer is a = 4, b = -4
b. Choose values for a and b to create an equation that supports Student B’s claim.
Answer:
There are 2 real solutions if b² – 4ac > 0
(-a)² – 4(1)(-b) > 0
a² + 4b > 0
The possible answer is a = 2, b = 1
c. Choose values for a and b to create an equation that does not support either student’s claim.
Answer:
There are no real solutions if b² – 4ac < 0
(-a)² – 4(1)(-b) < 0
a² + 4b < 0
The possible answer is a = 2, b = -2

Question 5.
Which equation represents the nth term of the sequence 3, 12, 48, 192, . . .?
A. a_n = 3(4)^n-1
B. a_n = 3(9)^n-1
C. a_n = 9n – 6
D. a_n = 9n + 3
Answer:
This is a geometric sequence
a1 = 3 and the common ratio is r = 4
Use the formula for the nth term
a_n = a1(r)^r-1
The equation will be
a_n = 3(4)^n-1
Thus the correct answer is option A.

Question 6.
Consider the function f(x) = \(\frac{1}{2} \sqrt[3]{x+3}\). The graph represents function g. Select all the statements that are true.

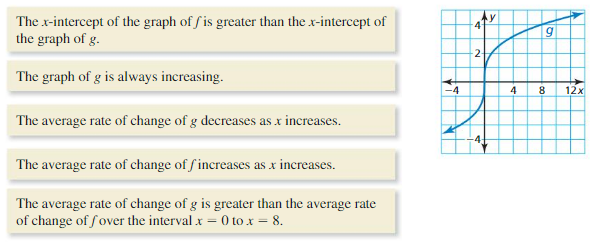
Answer:
The x-intercept of f is -3 while g is 0. (-3 < 0)
The graph of both f and g are always increasing
The average rate of change for both functions decreases as x increases.
For g(x). Estimate using the graph. Use (0, 0) and (8, 4)
The average rate of change us
g(8) – g(0)/8-0
= (4-0)/(8-0) = 0.50
For f(x) = [/latex]\sqrt[3]{x+3}[/latex]. Evaluate f when x = 0 and x = 8
f(0) = 1/2 [/latex]\sqrt[3]{0+3}[/latex] = 0.72
f(8) = 1/2 [/latex]\sqrt[3]{8+3}[/latex] = 1.11
The average rate of change us
f(8) – f(0)/8-0
= (1.11-0.72)/(8-0) = 0.05
The average rate of change of g is 0.5/0.05 = 10 times greater than the average rate of change of f over the interval x = 0 and x = 8

Question 7.
Place each function into one of the three categories.

Answer:
Recall the use of discriminant b² – 4ac to find the number of solutions
b² – 4ac < 0
b² – 4ac = 0
b² – 4ac > 0
f(x) = 3x² + 4x + 2
f(x) = -x² + 2x
f(x) = 4x² – 8x + 4
f(x) = x² – 3x – 21
f(x) = 7x²
f(x) = -6x² – 5

Question 8.
You are making a tabletop with a tiled center and a uniform mosaic border.
a. Write the polynomial in standard form that represents the perimeter of the tabletop.
b. Write the polynomial in standard form that represents the area of the tabletop.
c. The perimeter of the tabletop is less than 80 inches, and the area of tabletop is at least 252 square inches. Select all the possible values of x.

Answer:
From the figure, the dimensions of the tabletop are
L = 2x + 16 and W = 2x + 12
a. The perimeter is
P = 2L + 2W
P = 2(2x + 16 ) + 2(2x + 12)
P = 8x + 56
b. The area is
A = L . W
A = (2x + 16 ) × (2x + 12)
A = 4x² + 56x + 192
c. From the given,
P < 80
8x + 56 < 82
8x < 24
x < 3
A ≥ 252
4x² + 56x + 192 ≥ 252
4x² + 56x – 60 ≥ 0
(x + 15)(x – 1) ≥ 0