Spectrum Math Grade 8 Chapter 2 Lesson 7 Answer Key Comparing and Ordering Irrational Numbers

Students can use the Spectrum Math Grade 8 Answer Key Chapter 2 Lesson 2.7 Comparing and Ordering Irrational Numbers as a quick guide to resolve any of their doubts.

Spectrum Math Grade 8 Chapter 2 Lesson 2.7 Comparing and Ordering Irrational Numbers Answers Key

Rational and irrational numbers can be compared by approximating their value and placing them along a number line.
Place these numbers on a number line: $\sqrt{5}$, 2.5, $\sqrt{3}$
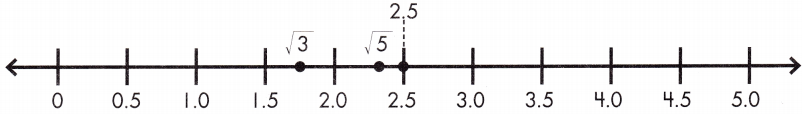
$Spectrum Math Grade 8 Chapter 2 Lesson 7 Answer Key Comparing and Ordering Irrational Numbers 1$

Put the values below in order from least to greatest along a number line.

Question 1.
π², 10, $\sqrt{75}$
$Spectrum Math Grade 8 Chapter 2 Lesson 7 Answer Key Comparing and Ordering Irrational Numbers 7$
Answer:
$Spectrum-Math-Grade-8-Chapter-2-Lesson-7-Answer-Key-Comparing-and-Ordering-Irrational-Numbers-7$
Rational and irrational numbers can be compared by approximating their value and placing them along a number line.

Question 2.
$\sqrt{7}$, $\frac{\sqrt{7}}{2}$, 2
$Spectrum Math Grade 8 Chapter 2 Lesson 7 Answer Key Comparing and Ordering Irrational Numbers 8$
Answer:
$Spectrum-Math-Grade-8-Chapter-2-Lesson-7-Answer-Key-Comparing-and-Ordering-Irrational-Numbers-8$
Rational and irrational numbers can be compared by approximating their value and placing them along a number line.

Question 3.
$\sqrt{10}$, 3.5, 2²
$Spectrum Math Grade 8 Chapter 2 Lesson 7 Answer Key Comparing and Ordering Irrational Numbers 9$
Answer:
$Spectrum-Math-Grade-8-Chapter-2-Lesson-7-Answer-Key-Comparing-and-Ordering-Irrational-Numbers-9$
Rational and irrational numbers can be compared by approximating their value and placing them along a number line.

Question 4.
4, $\sqrt{15}$, 5.2
$Spectrum Math Grade 8 Chapter 2 Lesson 7 Answer Key Comparing and Ordering Irrational Numbers 10$
Answer:
$Spectrum-Math-Grade-8-Chapter-2-Lesson-7-Answer-Key-Comparing-and-Ordering-Irrational-Numbers-10$
Rational and irrational numbers can be compared by approximating their value and placing them along a number line.

Question 5.
$\frac{1}{3}$, $\sqrt{1}$, 0.45
$Spectrum Math Grade 8 Chapter 2 Lesson 7 Answer Key Comparing and Ordering Irrational Numbers 11$
Answer:

$Spectrum-Math-Grade-8-Chapter-2-Lesson-7-Answer-Key-Comparing-and-Ordering-Irrational-Numbers-11$
Rational and irrational numbers can be compared by approximating their value and placing them along a number line.

Question 6.
$\sqrt{72}$, 9, 8²
$Spectrum Math Grade 8 Chapter 2 Lesson 7 Answer Key Comparing and Ordering Irrational Numbers 12$
Answer:
$Spectrum-Math-Grade-8-Chapter-2-Lesson-7-Answer-Key-Comparing-and-Ordering-Irrational-Numbers-12$
Rational and irrational numbers can be compared by approximating their value and placing them along a number line.

Expressions and equations containing irrational numbers can be approximated by testing values.

Compare using <, >, or =.

$\sqrt{3}$ + 5 ____ 3 + $\sqrt{5}$
$\sqrt{3}$ is between 1 and 2, so use 1.5.
$\sqrt{5}$ is between 2 and 3, so use 2.5.
(1.5) + 5 ____ 3 + (2.5)
6.5 > 5.5

Approximate the value of each expression and then compare using <, >, or =.

Question 1.
a. $\sqrt{10}$ + 2 _____ 10 + $\sqrt{2}$
Approximation: ____________
Answer: $\sqrt{10}$ + 2 < 10 + $\sqrt{2}$
$\sqrt{10}$ + 2 _____ 10 + $\sqrt{2}$
$\sqrt{10}$ is between 3 and 4, so use 3.5.
$\sqrt{2}$ is between 1 and 2, so use 1.5.
3.5 + 2 _________ 10 + 1.5
5.5 < 11.5

b. 4 + $\sqrt{2}$ _____ $\sqrt{4}$ + 2
Approximation: ____________
Answer: 4 + $\sqrt{2}$ > $\sqrt{4}$ + 2
4 + $\sqrt{2}$ _____ $\sqrt{4}$ + 2
$\sqrt{2}$ is between 1 and 2, so use 1.5.
$\sqrt{4}$ is 2.
4 + 1.5 _____2 + 2
5.5 > 4

Question 2.
a. 12 + $\sqrt{6}$ _____ $\sqrt{12}$ + 6
Approximation: ____________
Answer: 12 + $\sqrt{6}$ < $\sqrt{12}$ + 6
12 + $\sqrt{6}$ _____ $\sqrt{12}$ + 6
$\sqrt{6}$ is between 2 and 3, so use 2.5.
$\sqrt{12}$ is between 3 and 4, so use 3.5.
12 +2.5 _________ 3.5+6
14.5 < 9.5

b. $\sqrt[3]{8}$ + 6 _____ 8 + $\sqrt[3]{6}$
Approximation: ____________
Answer: $\sqrt[3]{8}$ + 6 < 8 + $\sqrt[3]{6}$
$\sqrt[3]{8}$ + 6 _____ 8 + $\sqrt[3]{6}$
$\sqrt[3]{8}$ is 2.
$\sqrt[3]{6}$ is 1 and 2, so use 1.5.
2 + 6 ______ 8+1.5
8 < 9.5

Question 3.
a. 15 + $\sqrt{12}$ _____ $\sqrt{15}$ + 12
Approximation: ____________
Answer: 15 + $\sqrt{12}$ > $\sqrt{15}$ + 12
15 + $\sqrt{12}$ _____ $\sqrt{15}$ + 12
$\sqrt{12}$ is between 3 and 4, so use 3.5.
$\sqrt{15}$ is between 3 and 4, so use 3.5.
15 + 3.5 ________ 3.5+12
18.5 > 15.5

b. $\sqrt{7}$ + 3 _____ 7 + $\sqrt{3}$
Approximation: ____________
Answer: $\sqrt{7}$ + 3 < 7 + $\sqrt{3}$
$\sqrt{7}$ + 3 _____ 7 + $\sqrt{3}$
$\sqrt{7}$ is between 2 and 3, so use 2.5.
$\sqrt{3}$ is between 1 and 2, so use 1.5.
2.5 + 3 ________ 7+1.5
5.5 < 8.5

Question 4.
a. 4 + $\sqrt[3]{7}$ _____ $\sqrt[3]{4}$ + 7
Approximation: ____________
Answer: 4 + $\sqrt[3]{7}$ < $\sqrt[3]{4}$ + 7
4 + $\sqrt[3]{7}$ _____ $\sqrt[3]{4}$ + 7
$\sqrt[3]{7}$ is between 1 and 2, so use 1.5.
$\sqrt[3]{4}$ is between 1 and 2, so use 1.5.
4 + 1.5 ________ 1.5 + 7
5.5 < 8.5

b. $\sqrt{3}$ + 5 _____ 3 + $\sqrt{5}$
Approximation: ____________
Answer: $\sqrt{3}$ + 5 > 3 + $\sqrt{5}$
$\sqrt{3}$ + 5 _____ 3 + $\sqrt{5}$
$\sqrt{3}$ is between 1 and 2, so use 1.5.
$\sqrt{5}$ is between 2 and 3, so use 2.5.
1.5 + 5 ________ 3 + 2.5
6.5 > 5.5

Spectrum Math Grade 8 Chapter 2 Lesson 2.7 Comparing and Ordering Irrational Numbers Answers Key

Leave a Comment Cancel Reply