We included HMH Into Math Grade 6 Answer Key PDF Module 4 Lesson 1 Add and Subtract Multi-Digit Decimals to make students experts in learning maths.
HMH Into Math Grade 6 Module 4 Lesson 1 Answer Key Add and Subtract Multi-Digit Decimals
I Can add and subtract multi-digit decimals to the thousandths with or without a model.
Step It Out
The 10 × 10 grid represents 1 whole. There are loo squares, so each square represents 0.01 or \(\frac{1}{100}\) of the whole.
1. Find the sum of 0.13 + 0.58 using a 10 × 10 grid.
A. How can you represent 0.13 on the grid?
_______________________

Answer:
To represent 0.13 on a hundredths grid, you can place 13 counters on a hundredths grid – one counter per square
B. Shade the grid to represent 0.13.
________________

C. How can you represent 0.58 on the grid?
________________
Answer:
To represent 0.58 on a hundredths grid, you can place 58 counters on a hundredths grid – one counter per square
D. Shade the grid to represent 0.58.
Answer:

E. How many total squares are shaded? ________________
So, 0.13 + 0.58 = _____.
Answer:
Count both the shades in both the grids.
13 + 58 = 71.
0.13 + 0.58 = 0.71
Adding decimals is similar to adding whole numbers. You must first write the numbers so that like places are aligned. Then add from right to left and regroup when necessary.

2. While at a grocery store, Robert bought 0.26 pound of red grapes and 0.34 pound of green grapes. How many total pounds of grapes did Robert buy?
A. You can use a table to align the places of decimals to make it easier to add. Write 0.34 in the table at the right. Add from right to left, regrouping when necessary.
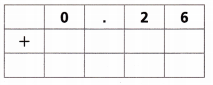
Answer:
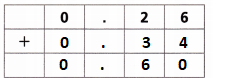
B. Did you need to regroup? Explain.
Answer:
0.26 + 0.34 = 0.60
Yes, you need to regroup ten ones as 1 ten.
C. Robert bought ![]() pound of grapes.
pound of grapes.
Answer: Robert bought 0.6 pounds of grapes.
Turn and Talk How is adding decimals different from adding whole numbers? Explain.
3. Find the difference of 0.42 – 0.19 using a 10 × 10 grid.
A. How can you represent 0.42 on the grid? How can you represent subtracting 0.19?

_______________________
_______________________
Answer:
To represent 0.42 on a hundredths grid, you can place 42 counters on a hundredths grid – one counter per square

B. How many shaded squares remain?
_______________________
Answer:
C. So, 0.42 – 0.19 = ____
0.42 – 0.19 = 0.23
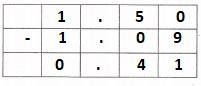
4. A cardinal weighs 1.5 ounces. Find the difference in ounces between the weights of a cardinal and a bluebird. The weight of a bluebird is shown.

A. What subtraction problem can you write to represent this situation?
Answer: 1.50 – 1.09
B. Write 1.5 in the table. Add a zero as a placeholder.

Answer:
C. Write 1.09 in the table. Subtract from right to left, regrouping when necessary.
ounce
Answer:
1.50 – 1.09 = 0.41
D. So. the weight of the cardinal is ___ ounce more than the weight of the bluebird.
Answer:
So, the weight of the cardinal is 0.41 ounces more than the weight of the bluebird.
Turn and Talk How can you subtract a decimal from a whole number?
Check Understanding
Question 1.
Julia has $1 for a snack. She buys an apple for $0.49. How much does she have left after buying the apple?
Answer:
Given,
Julia has $1 for a snack.
She buys an apple for $0.49.
We need to subtract the cost of the apple from the total amount.
1 – 0.49 = 0.51
$0.51 have left with Julia after buying the apple.
Question 2.
A group of friends spent $31.95 on movie tickets and $12.54 on refreshments. How much did they spend in all?
Answer:
Given,
A group of friends spent $31.95 on movie tickets and $12.54 on refreshments.
31.95 + 12.54 = $44.49
Thus they spent $44.49 in all.
On Your Own
Question 3.
Chu rides his bike 1.39 miles from his home to baseball practice. On the way home he takes a shorter route than the route he took to baseball practice. How far does he ride his bicycle from baseball practice to home?

Answer:
Given,
Chu rides his bike 1.39 miles from his home to baseball practice.
On the way home, he takes a shorter route than the route he took to baseball practice.
1.39 – 0.18 = 1.21 miles
Thus he rides 1.21 miles his bicycle from baseball practice to home.
Question 4.
Matias is working on a science project in school. He needs 0.33 kilogram of dry ice and 0.55 kilogram of regular ice for his project. How many total kilograms of ice does Matias need for his project?
Answer:
Given,
Matias is working on a science project in school.
He needs 0.33 kilogram of dry ice and 0.55 kilogram of regular ice for his project.
0.33 + 0.55 = 0.88 kilograms
Therefore Matias needs 0.88 kilograms for his project.
Question 5.
When two decimals are added or subtracted, in what order should you add or subtract the digits in the decimals?
_______________________
Answer:
1. Line up the decimal points vertically. Fill in any 0’s where necessary.
2. Add or subtract the numbers as if they were whole numbers.
3. Place the decimal point in the sum or difference so that it lines up vertically with the numbers being added or subtracted.
Question 6.
Use the table to subtract 378.5 – 26.19.

Answer:
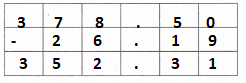
378.5 – 26.19 = 352.31
Question 7.
Use Structure Add 2.31 + 0.89 using the 10 × 10 grids.
A. Shade the grids to model the problem 2.31 + 0.89.
B. How many total squares are shaded?
____________
C. What is the sum?
____________
Answer:
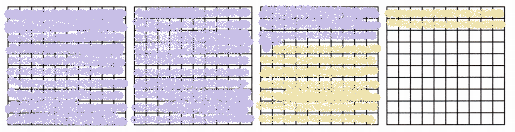
To represent 2.31 on a hundredths grid, you can place 231 counters on a 2 hundredths grid – one counter per square.
To represent 0.89 on a hundredths grid, you can place 89 counters on a hundredths grid – one counter per square.
231 + 89 = 320
2.31 + 0.89 = 3.20
For Problems 8-11, find the sum or difference.
Question 8.
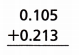
Answer:
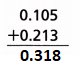
Question 9.
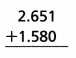
Answer:
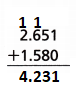
Question 10.
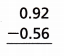
Answer:
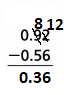
Question 11.

Answer:
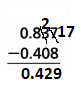
Question 12.
STEM In the periodic table each element is shown with its atomic mass. The atomic mass of silicon is approximately 28.09. The atomic mass of potassium is approximately 39.10. About how much greater is the atomic mass of potassium than that of silicon?
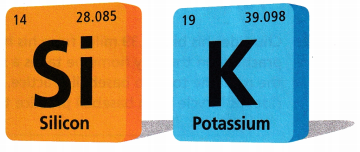
_______________________
Answer:
Given,
The atomic mass of silicon is approximately 28.09.
The atomic mass of potassium is approximately 39.10.
The atomic mass of potassium is greater than the atomic mass of silicon.
39.1 > 28.09
39.10 – 28.09 = 11.01
Question 13.
Open Ended Describe a general method for adding a decimal with two decimal places to a decimal with three decimal places. Give an example.
________________________
Answer:
In order to add two decimal numbers, first, check if they have the same number of digits to the right of the decimal point. If they don’t, add zeros to the right of one of the numbers until they do. Then, write one number on top of the other, lining up the decimal points vertically.
Question 14.
Look at the subtraction problem shown. Explain how to regroup so you can subtract in the hundredths place.
![]()
__________________________
__________________________
__________________________
__________________________
Answer:
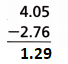
Borrow 1 from 4 and then make it as ten ones and again borrow 1 from hundredths place and make it as 15 ones.
4.05 – 2.76 = 1.29
Question 15.
Critique Reasoning Look at the addition problem shown. Is the sum correct? Why or why not?
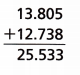
_______________________
_______________________
Answer: No the sum is not correct.
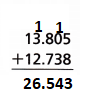
For Problems 16-19, find the sum or difference.
Question 16.
0.35 + 0.26 = ____
Answer: 0.61
0.35
+0.26
0. 61
The sum of two decimal numbers 0.35 and 0.26 is 0.61
Question 17.
1.60 + 1.98 = _____
Answer: 3.58
1.60
1.98
3.58
The sum of two decimal numbers 1.60 and 1.98 is 3.58
Question 18.
0.88 – 0.49 = ____
Answer: 0.39
0.88
0.49
0.39
The difference between the two decimal numbers 0.88 and 0.49 is 0.39
Question 19.
2.212 – 1.304 = ____
Answer: 0.908
2.212
1.304
0.908
The difference between the two decimal numbers 2.212 and 1.304 is 0.908
Lesson 4.1 More Practice/Homework
Add and Subtract Multi-Digit Decimals
Question 1.
Deangelo babysits for his neighbor. Over a two-week period, he babysat 3.8 hours the first week and 5.25 hours the second week. How many total hours did Deangelo babysit over the two weeks?
Answer:
Given,
Deangelo babysits for his neighbor.
Over a two-week period, he babysat for 3.8 hours the first week and
5.25 hours the second week.
3.8 + 5.25 = 9.05 hours
Deangelo babysits for 9.05 hours over the two weeks.
Question 2.
Babe Ruth. a professional baseball player known for hitting home runs, had a batting average of 0.342. Hank Aaron, another record home run hitter, had a batting average of 0.305. How much greater was Babe Ruth’s batting average than Hank Aaron’s?
Answer:
Given,
Babe Ruth. a professional baseball player known for hitting home runs, had a batting average of 0.342.
Hank Aaron, another record home run hitter, had a batting average of 0.305.
0.342 – 0.305 = 0.037
Question 3.
STEM The pH level of a substance is a measure of how acidic or alkaline it is. The pH of lemonade is 2.6, while orange juice has a pH of 4.09. What is the difference in the pH levels of orange juice and lemonade?
Answer:
Given that,
The pH level of a substance is a measure of how acidic or alkaline it is.
The pH of lemonade is 2.6, while orange juice has a pH of 4.09.
4.09 – 2.6 = 1.49
The difference in the pH levels of orange juice and lemonade is 1.49
Question 4.
A recipe for blintzes, a very thin type of pancake, calls for 0.06 liter of melted butter and 0.236 liter of milk. What is the total amount of butter and milk needed for the recipe?

Answer:
Given,
A recipe for blintzes, a very thin type of pancake, calls for 0.06 liter of melted butter and 0.236 liter of milk.
0.06 + 0.236 = 0.296
The total amount of butter and milk needed for the recipe is 0.296 liters
Question 5.
Math on the Spot Jared ran two sprints in track practice. His time for the first sprint was 4.64 seconds. His time for the second sprint was 4.3 seconds. What was Jared’s total time for the two sprints?
________________
Answer:
Given,
Jared ran two sprints in track practice.
His time for the first sprint was 4.64 seconds.
His time for the second sprint was 4.3 seconds.
To find the total time for the two spirits we have to add both the timings.
4.64 + 4.3 = 8.94 seconds
Jared’s total time for the two sprints is 8.94 seconds.
For Problems 6-11, find the sum or difference.
Question 6.

Answer: 7.79
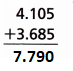
The sum of two decimal points 4.105 and 3.685 is 7.790
Question 17.
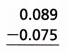
Answer:0.014
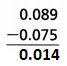
The difference between the two decimal points 0.089 and 0.075 is 0.014
Question 18.
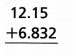
Answer: 18.982
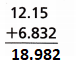
The sum of two decimal points 12.15 and 6.832 is 18.982
Question 19.
1.25 + 2.39
____________
Answer: 3.64
1.25 + 2.39 = 3.64
The sum of two decimal points 1.25 and 2.39 is 3.64
Question 20.
4.08 – 3.975
____________
Answer: 0.105
4.08 – 3.975 = 0.105
The difference between the two decimal points 4.08 and 3.975 is 0.105
Question 21.
0.91 – 0.487
____________
Answer: 0.423
0.91 – 0.487 = 0.423
The difference between the two decimal points 0.91 and 0.487 is 0.423
Test Prep
For Problems 12-13, use the following information.
Several teams, each consisting of 4 athletes, ran a relay race. The top two teams won ribbons. Team Rocket finished in 3.48 minutes and Team Jaguar finished in 3.471 minutes.
Question 12.
How much faster was Team Jaguar’s finish compared to Team Rocket’s finish?
![]() minute
minute
Answer:
Given,
Team Jaguar finished in 3.471 minutes.
Team Rocket finished in 3.48 minutes
3.48 – 3.471 = 0.009
Team rockets are 0.009 minutes faster than Team Jaguars.
Question 13.
Team Tortoise took twice as long to finish the race as Team Jaguar. How long did it take Team Tortoise to finish the race?
![]() minutes
minutes
Answer:
Team Jaguar finished in 3.471 minutes.
Given that Team Tortoise took twice as long to finish the race as Team Jaguar
3.471 × 2 = 6.942 minutes
Team Tortoise took 6.942 minutes to finish the race.
Question 14.
In 2018, the state sales tax in Maine was 5.5%, or 0.055. The state sales tax in Florida was 6%, or 0.06. How much greater was the sales tax in Florida than in Maine?
________________
Answer:
Given,
In 2018, the state sales tax in Maine was 5.5%, or 0.055.
The state sales tax in Florida was 6%, or 0.06.
0.060 – 0.055 = 0.05
Question 15.
The Nurburgring in Germany is a racetrack that hosts racing events all year. Two of the fastest laps ever driven on the track are 6 minutes 47.30 seconds and 6 minutes 52.01 seconds. How much faster is the 6 minutes 47.30 seconds time than the 6 minutes 52.01 seconds time?
________________
Answer:
Given,
The Nurburgring in Germany is a racetrack that hosts racing events all year.
Two of the fastest laps ever driven on the track are 6 minutes 47.30 seconds and 6 minutes 52.01 seconds.
52.01 – 47.30 = 4.71 seconds
Question 16.
A hectare is a measure of area. There are 2.471 acres in 1 hectare. What is the area of 3 hectares?
A. 0.529 acre
B. 5.471 acres
C. 4.942 acres
D. 7.413 acres
Answer:
Given,
A hectare is a measure of area. There are 2.471 acres in 1 hectare.
2.471 × 3 = 7.413 acres
Option D is the correct answer.
Spiral Review
Question 17.
What is the quotient of \(\frac{1}{5}\) ÷ \(\frac{2}{3}\)?
________________
Answer:
\(\frac{1}{5}\) ÷ \(\frac{2}{3}\)
Take the reciprocal of the second fraction by flipping the numerator and denominator and changing the operation to multiplication.
Then the equation becomes
\(\frac{1}{5}\) × \(\frac{3}{2}\) = \(\frac{3}{10}\)
Thus the quotient is \(\frac{3}{10}\)
Question 18.
A garden bed has 4 sections of vegetables. The garden is 8\(\frac{1}{2}\) yards long. If each section is equal in length, what is the length of each section?
Answer:
Given,
A garden bed has 4 sections of vegetables.
The garden is 8\(\frac{1}{2}\) yards long.
4 + 8\(\frac{1}{2}\) = 12 \(\frac{1}{2}\)
Question 19.
The elevation of a coral reef is 12 feet below sea level. The elevation of a snorkeler is 2 feet below sea level. Write an inequality to compare the elevations using integers.
Answer:
Given that the elevation of a coral reef is 12 feet below sea level. The elevation of a snorkeler is 2 feet below sea level.
We know that elevation below the sea level is negative and elevation above the sea level is positive.
The elevation of the coral reef would be -12 feet and the elevation of the snorkeler would be -2.
We know that a larger negative number has less value than a smaller negative number. -12 is more negative, so it will be less than -2.
Therefore, our required inequality would be -12 < -2