We included HMH Into Math Grade 5 Answer Key PDF Module 4 Review to make students experts in learning maths.
HMH Into Math Grade 5 Module 4 Review Answer Key
Vocabulary
Question 1.
Draw lines to match the term with its description.

Answer:
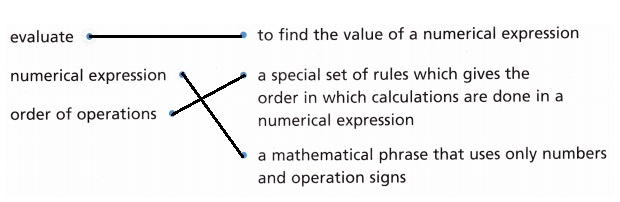
Explanation:
Drawn lines to match the term with its description as shown above.
Concepts and Skills
Question 2.
Write a numerical expression to model the words. Add 5 and 25, and then multiply the sum by 4.
Answer:
(5 + 25) X 4,
Explanation:
Given to find a numerical expression to model the words.
Add 5 and 25, and then multiply the sum by 4,
so (5 + 25) X 4.
Question 3.
Which of the following can be modeled by the numerical expression (20 – 15) ÷ 5? Select all that apply.
A. Find the difference of 20 and the quotient of 15 and 5.
B. Subtract 15 from 20, and then divide the difference by 5.
C. Divide 20 by 5, and then subtract 15.
D. Divide the difference of 20 and 15 by 5.
E. Subtract the quotient of 15 and 5 from 20.
Answer:
B. Subtract 15 from 20, and then divide the difference by 5,
D. Divide the difference of 20 and 15 by 5,
Explanation:
Given to check the numerical expression (20 – 15) ÷ 5 that can apply first we see the value of
(20 – 15) ÷ 5 is 5 ÷ 5 = 1,
A. The difference of 20 and the quotient of 15 and 5 is
20 – (15 ÷ 5) = 20 – 3 = 17 ≠ 1,
B. Subtract 15 from 20, and then divide the difference by 5 is
(20 – 15) ÷ 5 is 5 ÷ 5 = 1 = 1,
C. Divide 20 by 5, and then subtract 15 is
(20 ÷ 5) – 15 = 4 – 15 = -11 ≠ 1,
D. Divide the difference of 20 and 15 by 5 is
(20 – 15) ÷ 5 = 5 ÷ 5 = 1 = 1,
E. Subtract the quotient of 15 and 5 from 20 is
(15 ÷ 5) – 20 = 3 – 20 = -17 ≠ 1.
therefore the models B. Subtract 15 from 20, and then divide the difference by 5,
D. Divide the difference of 20 and 15 by 5 can be applied for the numerical expression (20 – 15) ÷ 5.
Question 4.
Use Tools Compare the numerical expressions 9 × (15 – 8) and 15 – 8. Tell what strategy or tool you will use to solve the problem, explain, your choice, and then find the answer.
Answer:
9 X (15 – 8) > 15 – 8,
9 times greater than 15 – 8,
Explanation:
Given to compare the numerical expressions 9 × (15 – 8) and 15 – 8. I use > < and = to solve the problem as
9 X (15 – 8) is 9 times greater than (15 – 8) so
9 X (15 – 8) > 15 – 8.
Question 5.
Which is the value of the numerical expression 6 + 2 × (8 – 4)?
A. 10
B. 12
C. 14
D. 32
Answer:
D. 32,
Explanation:
Given to find the value of the numerical expression 6 + 2 × (8 – 4) the order I follow is first parentheses then left to right addition and multiplication as 8 X (4) = 32 matches with
D.32.
Question 6.
Which is the value of the numerical expression 30 ÷ 5 – (3 + 1)?
A. 2
B. 4
C. 16
D. 30
Answer:
A. 2,
Explanation:
Given to find the value of the numerical expression 30 ÷ 5 – (3 + 1) is
30 ÷ 5 – (3 + 1) the order I follow is first parentheses then left to right division and then subtraction as
6 – (4) = 2 which matches with A. 2.
Question 7.
Select all the numerical expressions that have a value of 9.
A. 6 × (5 + 2 × 9 – 3)
B. 6 × 5 – 2 × 9 – 3
C. 6 × (5 – 2) × 9 – 3
D. (6 × 5 – 2) × 9 + 3
E. (6 × 5) – (2 × 9) – 3
F. (6 × 5) – 2 × (9 – 3)
Answer:
B. 6 × 5 – 2 × 9 – 3 and
E. (6 × 5) – (2 × 9) – 3,
Explanation:
Checking all numerical expressions that have a value of 9,
A. 6 × (5 + 2 × 9 – 3) = 6 X (7 X 6) = 6 X 42 = 252 ≠ 9,
B. 6 × 5 – 2 × 9 – 3 = 30 – 18 – 3 = 30 – 21 = 9 = 9,
C. 6 × (5 – 2) × 9 – 3 = 6 X 3 X 6= 108 ≠ 9,
D. (6 × 5 – 2) × 9 + 3 = 28 X 9 + 3 = 252 + 3 = 255 ≠ 9,
E. (6 × 5) – (2 × 9) – 3 = 30 – 18 – 3 = 30 – 21 = 9 = 9,
F. (6 × 5) – 2 × (9 – 3) = 30 – 2 X (6) = 30 – 12 = 18 ≠ 9.
therefore B. 6 × 5 – 2 × 9 – 3 and E. (6 × 5) – (2 × 9) – 3 numerical expressions that have a value of 9.
Question 8.
Use parentheses to rewrite the numerical expression 18 + 3 × 6 ÷ 3 to have a value of 12.
Answer:
The numerical expression 18 + 3 × 6 ÷ 3 to have a value of 12 using parentheses is
(18 +(3 X 6)) ÷ 3,
Explanation:
Given to rewrite the numerical expression 18 + 3 × 6 ÷ 3 to have a value of 12 using parentheses is
1. (18 + 3) X (6 ÷ 3) = 21 X 2 = 42 ≠ 12,
2. 18 + ((3 X 6) ÷ 3) = 18 + (18 ÷ 3) = 18 + 6 = 24 ≠ 12,
3. (18 +(3 X 6)) ÷ 3 = (18 + 18) ÷ 3 = 36 ÷ 3 = 12 = 12,
So the numerical expression 18 + 3 × 6 ÷ 3 to have a value of 12 using parentheses is
(18 +(3 X 6)) ÷ 3.
Question 9.
Use parentheses to rewrite the numerical expression 4 × 7 – 2 × 3 to have a value of 60.
Answer:
The numerical expression 4 X 7 – 2 X 3 to have a value of 60 using parentheses is
(4 X (7 – 2)) X 3 or 4 X ((7 – 2) X 3),
Explanation:
Given to rewrite the numerical expression 4 X 7 – 2 X 3 to have a value of 60 using parentheses is
1. (4 X 7) – ( 2 X 3) = 28 – 6 = 22 ≠ 60,
2. (4 X (7 – 2)) X 3 = (4 X 5) X 3 = 20 X 3 = 60 = 60,
3. 4 X ((7 – 2) X 3) = 4 X (5 X 3) = 4 X 15 = 60 = 60,
therefore the numerical expression 4 X 7 – 2 X 3 to have a value of 60 using parentheses is
(4 X (7 – 2)) X 3 or 4 X ((7 – 2) X 3).