We included HMH Into Math Grade 4 Answer Key PDF Module 15 Lesson 4 Rename Mixed Numbers to Subtract to make students experts in learning maths.
HMH Into Math Grade 4 Module 15 Lesson 4 Answer Key Rename Mixed Numbers to Subtract
I Can rename mixed numbers to subtract fractions with like denominators to solve real-world problems.
Spark Your Learning
At a petting zoo, a baby goat is on a special diet. She receives 3\(\frac{1}{4}\) cups of food each day. The baby goat eats 1\(\frac{3}{4}\) cups of the food in the morning. How much is left for her evening feeding?

Represent the problem with a fraction model.
Answer:
1\(\frac{2}{4}\) = 1\(\frac{1}{2}\)
Explanation:
3\(\frac{1}{4}\) – 1\(\frac{3}{4}\)
= \(\frac{13}{4}\) – \(\frac{7}{4}\)
= \(\frac{13 – 7}{4}\)
= \(\frac{6}{4}\)
= 1\(\frac{2}{4}\)
Turn and Talk A classmate says that you just need to subtract the whole number from the whole number and the fraction from the fraction to solve this problem. How would you respond?
Answer:
\(\frac{2}{4}\)
Explanation:
3\(\frac{1}{4}\) – 1\(\frac{3}{4}\)
subtract the whole number from the whole number
3 – 1 = 2
the fraction from the fraction to solve this problem.
= \(\frac{1}{4}\) – \(\frac{3}{4}\)
= \(\frac{1 – 3}{4}\)
= \(\frac{2}{4}\)
Build Understanding
Question 1.
Bessie the cow drinks 4\(\frac{1}{8}\) gallons of water each day. She drinks 1\(\frac{7}{8}\) gallons before noon. How much water will Bessie still need to drink before the end of the day?

A. Model the situation with a visual representation.
Answer:
2 \(\frac{2}{8}\)
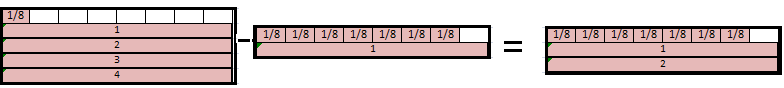
B. What equation can you use to model the problem?
Use d to represent the amount Bessie still needs to drink.
Answer:
2 \(\frac{2}{8}\)
Explanation:
d= 4\(\frac{1}{8}\) – 1\(\frac{7}{8}\)
d= \(\frac{33}{8}\) – \(\frac{15}{8}\)
d= \(\frac{33 – 15}{8}\)
d = \(\frac{18}{8}\)
d = 2 \(\frac{2}{8}\)
C. Explain how you could model the problem with an equation using only fractions greater than one. Show your equation.
Answer:
Subtract the whole number from the whole number
4 – 1 = 3
Explanation:
d= 4\(\frac{1}{8}\) – 1\(\frac{7}{8}\)
d= \(\frac{33}{8}\) – \(\frac{15}{8}\)
d= \(\frac{33 – 15}{8}\)
d = \(\frac{18}{8}\)
d = 2 \(\frac{2}{8}\)
D. What mixed number equals the number of gallons of water that are left for Bessie to drink?
Answer:
2 \(\frac{2}{8}\)
Explanation:
4\(\frac{1}{8}\) – 1\(\frac{7}{8}\)
= \(\frac{33}{8}\) – \(\frac{15}{8}\)
= \(\frac{33 – 15}{8}\)
= \(\frac{18}{8}\)
= 2 \(\frac{2}{8}\)
Turn and Talk What equation could you have written to model the problem using another operation? How do the two equations relate to each other?
Answer:
2 \(\frac{2}{8}\)
Explanation:
4\(\frac{1}{8}\) – 1\(\frac{7}{8}\)
= \(\frac{33}{8}\) – \(\frac{15}{8}\)
= \(\frac{33 – 15}{8}\)
= \(\frac{18}{8}\)
= 2 \(\frac{2}{8}\)
Step It Out
Question 2.
Two kittens get 4\(\frac{2}{4}\) ounces of food. The bigger kitten gets 2\(\frac{3}{4}\) ounces. How much food does the smaller kitten get?

You sometimes need to rename mixed numbers to subtract. One way ¡s to rename a mixed number so the fractional part is greater than 1.
A. Rename the mixed number that is the amount of food given to the pair so its fractional part is greater than 1. Show your work.
Answer:
1\(\frac{3}{4}\)
Explanation:
4\(\frac{2}{4}\) – 2\(\frac{3}{4}\)
= \(\frac{18}{4}\) – \(\frac{11}{4}\)
= \(\frac{18 – 11}{4}\)
= \(\frac{7}{4}\)
= 1\(\frac{3}{4}\)
B. Model the problem with an equation using the renamed mixed number and solve the problem.
Answer:
1\(\frac{3}{4}\)
Explanation:
4\(\frac{2}{4}\) – 2\(\frac{3}{4}\)
= \(\frac{18}{4}\) – \(\frac{11}{4}\)
= \(\frac{7}{4}\)
= 1\(\frac{3}{4}\)
Another way is to rename both mixed numbers as fractions greater than 1, and then subtract.
C. Write an equation modeling the problem and its solution using only fractions greater than 1.
Answer:
\(\frac{7}{4}\)
Explanation:
4\(\frac{2}{4}\) – 2\(\frac{3}{4}\)
4 – 2 = 2
4\(\frac{2}{4}\) – 2\(\frac{3}{4}\)
= \(\frac{18}{4}\) – \(\frac{11}{4}\)
= \(\frac{7}{4}\)
Turn and Talk Which method do you prefer if you have to rename a mixed number to subtract: renaming one mixed number or renaming both mixed numbers? Why?
Answer:
One way ¡s to rename a mixed number so the fractional part is greater than 1.
To rename a mixed number if the fraction we are subtracting is larger than the first fraction. We have two mixed numbers.
Explanation:
For example, we find common denominators first, convert fractions into decimals and then back into fractions, or simplify fractions first and then subtract.
Check Understanding
Question 1.
Max has 6\(\frac{3}{6}\) pounds of seed. He used some and now has 4\(\frac{5}{6}\) pounds left. How many pounds of seed did Max use? Write an equation to model the problem.
Answer:
6\(\frac{3}{6}\) – 4\(\frac{5}{6}\) = 1\(\frac{4}{6}\)
Explanation:
Max has 6\(\frac{3}{6}\) pounds of seed.
He used some and now has 4\(\frac{5}{6}\) pounds left.
Total pounds of seed Max use = 6\(\frac{3}{6}\) – 4\(\frac{5}{6}\)
= \(\frac{39}{6}\) – \(\frac{29}{6}\)
= \(\frac{10}{6}\) = 1\(\frac{4}{6}\)
On Your Own
Question 2.
Pablo spends 3\(\frac{1}{6}\) hours painting, and Miguel spends 1\(\frac{5}{6}\) hours painting. How many fewer hours does Miguel spend painting than Pablo spends? Show your work.
Answer:
\(\frac{8}{6}\) hours
Explanation:
Pablo spends 3\(\frac{1}{6}\) hours painting,
Miguel spends 1\(\frac{5}{6}\) hours painting.
Total fewer hours Miguel spend painting than Pablo spends,
= 3\(\frac{1}{6}\) – 1\(\frac{5}{6}\)
= \(\frac{19}{6}\) – \(\frac{11}{6}\)
= \(\frac{8}{6}\)
Question 3.
Open-Ended Write three mixed numbers that are between 8 and 10 that would require renaming in order to subtract 7\(\frac{6}{8}\) from them.
Answer:
\(\frac{6}{8}\)
Explanation:
x = 8 – 7\(\frac{6}{8}\)
= 8 – \(\frac{62}{8}\)
= \(\frac{64 – 62}{8}\) = \(\frac{2}{8}\)= \(\frac{6}{8}\)
Question 4.
Reason Susie has 5\(\frac{2}{8}\) cups of flour. She uses 2\(\frac{7}{8}\) cups of flour to make pasta. To make bread, she needs 2\(\frac{4}{8}\) cups of flour. Does she have enough flour to make both? Explain.
Answer:
No, she don’t have enough flour to make.
Explanation:
Susie has 5\(\frac{2}{8}\) cups of flour.
She uses 2\(\frac{7}{8}\) cups of flour to make pasta.
= 5\(\frac{2}{8}\) – 2\(\frac{7}{8}\)
= \(\frac{42}{8}\) – \(\frac{23}{8}\)
= \(\frac{42-23}{8}\) = \(\frac{19}{8}\)
= 2\(\frac{3}{8}\)
Find the difference. If possible, write your answer as a mixed number.
Question 5.
1\(\frac{6}{8}\) – \(\frac{7}{8}\)
Answer:
\(\frac{7}{8}\)
Explanation:
1\(\frac{6}{8}\) – \(\frac{7}{8}\)
= \(\frac{14}{8}\) – \(\frac{7}{8}\)
= \(\frac{14-7}{8}\) – \(\frac{7}{8}\)
Question 6.
3\(\frac{2}{8}\) – 2\(\frac{7}{8}\)
Answer:
\(\frac{3}{8}\)
Explanation:
3\(\frac{2}{8}\) – 2\(\frac{7}{8}\)
= \(\frac{26}{8}\) – \(\frac{23}{8}\)
= \(\frac{26-23}{8}\) – \(\frac{3}{8}\)
Question 7.
4\(\frac{6}{12}\) – 1\(\frac{11}{12}\)
Answer:
2\(\frac{7}{12}\)
Explanation:
4\(\frac{6}{12}\) – 1\(\frac{11}{12}\)
= \(\frac{54}{12}\) – \(\frac{23}{12}\)
= \(\frac{54-23}{12}\) = \(\frac{31}{12}\)
= 2\(\frac{7}{12}\)
Question 8.
9\(\frac{1}{4}\) – \(\frac{3}{4}\)
Answer:
8\(\frac{2}{4}\)
Explanation:
9\(\frac{1}{4}\) – \(\frac{3}{4}\)
= \(\frac{37}{4}\) – \(\frac{3}{4}\)
= \(\frac{37-3}{4}\) = \(\frac{34}{4}\)
= 8\(\frac{2}{4}\)
Question 9.
6\(\frac{2}{5}\) – 4\(\frac{4}{5}\)
Answer:
1\(\frac{3}{5}\)
Explanation:
6\(\frac{2}{5}\) – 4\(\frac{4}{5}\)
= \(\frac{32}{5}\) – \(\frac{24}{5}\)
= \(\frac{32-24}{5}\) = \(\frac{8}{5}\)
= 1\(\frac{3}{5}\)
Question 10.
8\(\frac{2}{6}\) – 4\(\frac{5}{6}\)
Answer:
3\(\frac{3}{6}\)
Explanation:
8\(\frac{2}{6}\) – 4\(\frac{5}{6}\)
= \(\frac{50}{6}\) – \(\frac{29}{6}\)
= \(\frac{50-29}{6}\) = \(\frac{21}{6}\)
= 3\(\frac{3}{6}\)
I’m in a Learning Mindset!
How does a fraction model help me when subtracting mixed numbers that need renaming?
Answer:
Fraction model shows a whole shape split into equal parts.
Explanation:
Mixed number is a number represented by a whole number and a fraction.
Use renaming to subtract mixed number from a whole number,
when the fraction is greater than 1 have numerators that are greater than their denominators.