Engage NY Eureka Math Precalculus Module 5 Lesson 7 Answer Key
Eureka Math Precalculus Module 5 Lesson 7 Exploratory Challenge 1/Exercise Answer Key
Exploratory Challenge 1/Exercises 1–5
A new game, Six Up, involves two players. Each player rolls her die, counting the number of times a six is rolled. The players roll their dice for up to one minute. The first person to roll 15 sixes wins. If no player rolls 15 sixes in the one-minute time limit, then the player who rolls the greatest number of sixes wins that round. The player who wins the most rounds wins the game.
Suppose that your class will play this game. Your teacher poses the following question:
How many sixes would you expect to roll in one round?
Exercise 1.
How would you answer this question?
Answer:
Answers will vary. Anticipate a wide range of answers to this question.
Exercise 2.
What discrete random variable should you investigate to answer this question?
Answer:
The discrete random variable is the number of sixes rolled in a round.
Exercise 3.
What are the possible values for this discrete random variable?
Answer:
The values are 0, 1, 2, 3, 4, …, 15.
Exercise 4.
Do you think these possible values are all equally likely to be observed?
Answer:
No. Some outcomes are more likely than others. For example, it would be unlikely to see only 0 or 1 six rolled in a round.
Exercise 5.
What might you do to estimate the probability of observing each of the different possible values?
Answer:
We could play many rounds of the game to see how often each number occurs. This would allow us to estimate the probabilities.
Exploratory Challenge 1/Exercises 6–8
You and your partner will play the Six Up game. Roll the die until the end of one minute or until you or your partner rolls 15 sixes. Count the number of sixes you rolled. Remember to stop rolling if either you or your partner rolls 15 sixes before the end of one minute.
Exercise 6.
Play five rounds. After each round, record the number of sixes that you rolled.
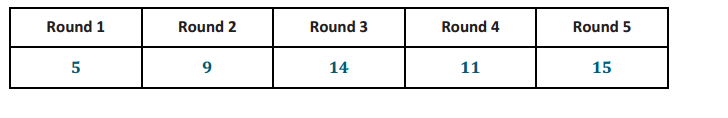
Answer:
Student answers will vary. Values range from 0 to 15. One possible answer is shown.
The number of sixes rolled in each round is shown below.
Exercise 7.
On the board, put a tally mark for the number of sixes rolled in each round.
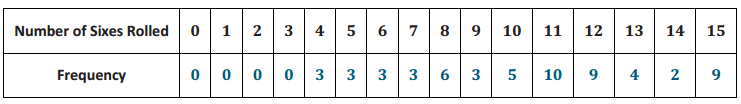
Answer:
Student answers will vary. Values range from 0 to 15. One possible answer is shown.
The frequencies for the number of sixes rolled by the class are shown below.
Exercise 8.
Answer:
Using the data summarized in the frequency chart on the board, find the mean number of sixes rolled in a round.
Answers will vary. For the example given in Exercise 6:
The total number of observations is 60.
\(\frac{\mathbf{0}(0) + 1(0) + 2(0) + 3(0) + 4(3) + 5(3) + 6(3) + 7(3) + 8(6) + 9(3) + 10(5) + 11(10) + 12(9) + 13(4) + 14(2) + 15(9)}{60}\)
= 10.4
The mean is 10.4 sixes.
Exploratory Challenge 1/Exercises 9–13
Exercise 9.
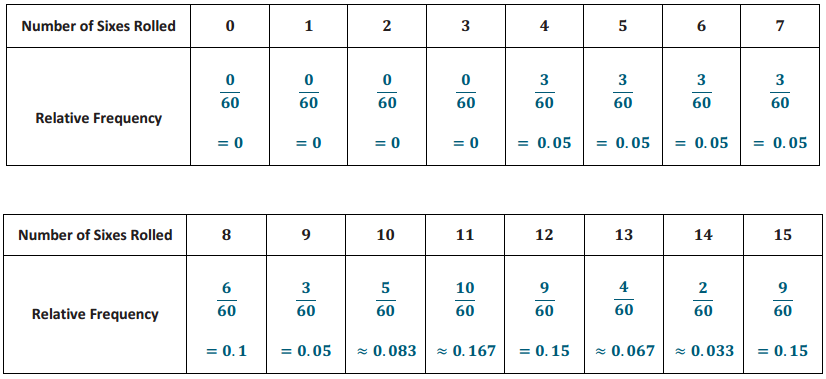
Calculate the relative frequency (proportion) for each value of the discrete random variable (i.e., the number of sixes rolled) by dividing the frequency for each possible value of the number of sixes rolled by the total number of rounds (the total number of tally marks). (The relative frequencies can be interpreted as estimates of the probabilities of observing the different possible values of the discrete random variable.)
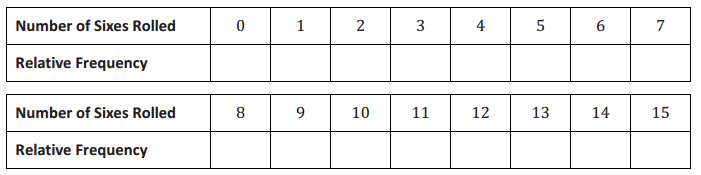
Answer:
Student answers will vary. For the above example:
The proportions are displayed in the table below. The observed number for each x-value is divided by 60, the total number of observations of the variable.
Exercise 10.
Multiply each possible value for the number of sixes rolled by the corresponding probability (relative frequency).
Answer:
Answers will vary. The products for the example given above are the following:
0(0) = 0
1(0) = 0
2(0) = 0
3(0) = 0
4(0.05) = 0.2
5(0.05) = 0.25
6(0.05) = 0.3
7(0.05) = 0.35
8(0.1) = 0.8
9(0.05) = 0.45
10(0.083) = 0.83
11(0.167) = 1.837
12(0.15) = 1.8
13(0.067) = 0.871
14(0.033) = 0.462
15(0.15) = 2.25
Exercise 11.
Find the sum of the calculated values in Exercise 10. This number is called the expected value of the discrete random variable.
Answer:
Student answers will vary. For the above example:
0 + 0 + 0 + 0 + 0.2 + 0.25 + 0.3 + 0.35 + 0.8 + 0.45 + 0.83 + 1.837 + 1.8 + 0.871 + 0.462 + 2.25
= 10.4
The expected value is 10.4 sixes.
Exercise 12.
What do you notice about the sum in Exercise 11 and the mean that you calculated in Exercise 8?
Answer:
The two values are the same. The procedures in Exercises 9, 10, and 11 combine to create a procedure for calculating the expected value of a discrete random variable.
Exercise 13.
The expected value of a random variable, x, is also called the mean of the distribution of that random variable. Why do you think it is called the mean?
Answer:
Because it is like calculating a long-run average value for the random variable.
Exploratory Challenge 1/Exercise 14
The expected value for a discrete random variable is computed using the following equation:
expected value = ∑(each value of the random variable (x))⋅(the corresponding probability (p))
or
expected value = ∑xp
where x is a possible value of the random variable, and p is the corresponding probability.
The following table provides the probability distribution for the number of heads occurring when two coins are flipped.
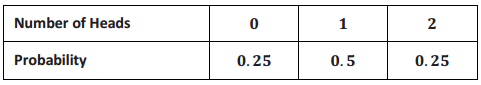
Exercise 14.
If two coins are flipped many times, how many heads would you expect to occur, on average?
Answer:
0(0.25) + 1(0.5) + 2(0.25) = 1
You would expect 1 head to occur when two coins are flipped.
Exploratory Challenge 1/Exercises 15–16
Exercise 15.
The estimated expected value for the number of sixes rolled in one round of the Six Up game was 10.4. Write a sentence interpreting this value.
Answer:
If a player were to play many rounds of the Six Up game, the average number of sixes rolled by that player would be about 10.4 sixes per round.
Exercise 16.
Suppose that you plan to change the rules of the Six Up game by increasing the one-minute time limit for a round. You would like to set the time so that most rounds will end by a player reaching 15 sixes. Considering the estimated expected number of sixes rolled in a one-minute round, what would you recommend for the new time limit? Explain your choice.
Answer:
Answers will vary. Look for a justification based on the expected value. For example, a student might say 1.5 minutes, reasoning that if the average number of sixes rolled in one minute is about 10, increasing the time by 10% to 1.5 minutes should be enough time because the average would then be around 15. Or a student might say two minutes should be enough time because if the average number of sixes in one minute is around 19, in two minutes, it would be very likely that one of the players would get to 15 sixes.
Exploratory Challenge 2/Exercises 17–19
Suppose that we convert the table on the previous page displaying the discrete distribution for the number of heads occurring when two coins are flipped to two vectors.
Let vector a be the number of heads occurring.
Let vector b be the corresponding probabilities.
a = (0, 1, 2)
b = (0.25, 0.5, 0.25)
Exercise 17.
Find the dot product of these two vectors.
Answer:
0(0.25) + 1(0.5) + 2(0.25) = 1
Exercise 18.
Explain how the dot product computed in Exercise 17 compares to the expected value computed in Exercise 14.
Answer:
The dot product and the expected value are equal.
Exercise 19.
How do these two processes, finding the expected value of a discrete random variable and finding the dot product of two vectors, compare?
Answer:
The two processes are the same.
Eureka Math Precalculus Module 5 Lesson 7 Problem Set Answer Key
Question 1.
The number of defects observed in the paint of a newly manufactured car is a discrete random variable. The probability distribution of this random variable is shown in the table below.

If large numbers of cars were inspected, what would you expect to see for the average number of defects per car?
Answer:
0(0.02) + 1(0.15) + 2(0.40) + 3(0.35) + 4(0.05) + 5(0.03) = 2.35
The expected number of defects is 2.35.
Note: Since the expected number of defects is the mean of the probability distribution, we do not round to a whole number.
Question 2.
a. Interpret the expected value calculated in Problem 1. Be sure to give your interpretation in context.
Answer:
If many cars were inspected and the number of defects was observed for each car, the average number of defects per car would be about 2.35.
b. Explain why it is not reasonable to say that every car will have the expected number of defects.
Answer:
The expected value is an average. It represents a typical value for a random variable, but individual values may be greater than or less than the expected value.
Question 3.
Students at a large high school were asked how many books they read over the summer. The number of books read is a discrete random variable. The probability distribution of this random variable is shown in the table below.
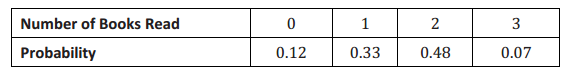
If a large number of students were asked how many books they read over the summer, what would you expect to see for the average number of books read?
Answer:
0(0.12) + 1(0.33) + 2(0.48) + 3(0.07) = 1.5
The expected number of books is 1.5.
Question 4.
Suppose two dice are rolled. The sum of the two numbers showing is a discrete random variable. The following table displays the probability distribution of this random variable:
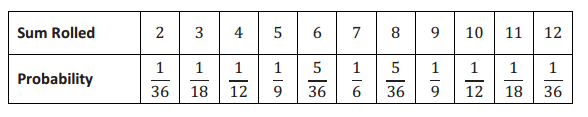
If you rolled two dice a large number of times, what would you expect the average of the sum of the two numbers showing to be?
Answer:
The expected sum of the two rolled dice is
2(\(\frac{1}{36}\)) + 3(\(\frac{1}{18}\)) + 4(\(\frac{1}{12}\)) + 5(\(\frac{1}{9}\)) + 6(\(\frac{5}{36}\)) + 7(\(\frac{1}{6}\)) + 8(\(\frac{5}{36}\)) + 9(\(\frac{1}{9}\)) + 10(\(\frac{1}{12}\)) + 11(\(\frac{1}{18}\)) + 12(\(\frac{1}{36}\)) = 7
Question 5.
Explain why it is not possible for a random variable whose only possible values are 3, 4, and 5 to have an expected value greater than 6.
Answer:
Expected value is an average. The average of a large number of observations of this variable cannot be greater than 6 if the variable can only have values that are 6 or less.
Question 6.
Consider a discrete random variable with possible values 1, 2, 3, and 4. Create a probability distribution for this variable so that its expected value would be greater than 3 by entering probabilities into the table below. Then, calculate the expected value to verify that it is greater than 3.
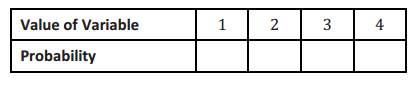
Answer:
Answers will vary. One possible answer is as follows:

1(0.1) + 2(0.1) + 3(0.1) + 4(0.7) = 3.4
The expected value is 3.4, which is greater than 3.
Eureka Math Precalculus Module 5 Lesson 7 Exit Ticket Answer Key
At a carnival, one game costs $1 to play. The contestant gets one shot in an attempt to bust a balloon. Each balloon contains a slip of paper with one of the following messages:
Sorry, you do not win, but you get your dollar back. (The contestant has not lost the $1 cost.)
Congratulations, you win $2. (The contestant has won $1.)
Congratulations, you win $5. (The contestant has won $4.)
Congratulations, you win $10. (The contestant has won $9.)
If the contestant does not bust a balloon, then the $1 cost is forfeited. The table below displays the probability distribution of the discrete random variable, or net winnings for this game.
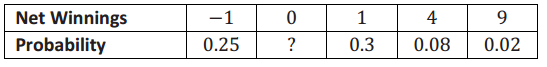
Question 1.
What is the sum of the probabilities in a discrete probability distribution? Why?
Answer:
The sum of the probabilities in a discrete probability distribution is one. In a discrete distribution, every possible x-value of the random variable is listed. Thus, the sum of the corresponding probabilities must equal one.
Question 2.
What is the probability that a contestant will bust a balloon and receive the message, “Sorry, you do not win, but you get your dollar back”?
Answer:
If you receive the message, “Sorry, you do not win, but you get your dollar back,” then your net winnings is $0. The probability of winning $0 is
1-(0.25 + 0.3 + 0.08 + 0.02) = 0.35.
Question 3.
What is the net amount that a contestant should expect to win per game if the game were to be played many times?
Answer:
The net amount that a contestant should expect to win is the expected value of the probability distribution, which is $0.55:
-1(0.25) + 0(0.35) + 1(0.3) + 4(0.08) + 9(0.02) = 0.55