Engage NY Eureka Math Precalculus Module 4 Lesson 13 Answer Key
Eureka Math Precalculus Module 4 Lesson 13 Example Answer Key
Example
The Statue of Liberty is 151 feet tall and sits on a pedestal that is 154 feet above the ground. An observer who is 6 feet tall wants to stand at the ideal viewing distance in front of the statue.
a. Sketch the statue and observer. Label all appropriate measurements on the sketch, and define them in context.
Answer:
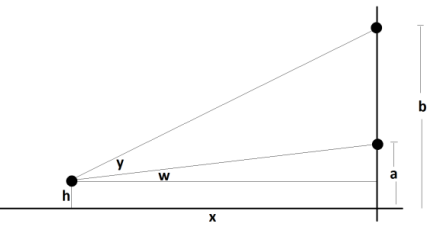
h: height of the observer at eye level
a: height of pedestal
b: height of the pedestal and statue
x: horizontal distance between viewer and base of the statue
y: viewing angle
w: angle formed between line of sight from viewer to the base of the statue and a line segment parallel to x from the viewer’s eye level to the statue
b. How far back from the statue should the observer stand so that his or her viewing angle (from the feet of the statue to the tip of the torch) is largest? What is the value of the largest viewing angle?
Answer:
h = 6
a = 151
b = 154 + 151 = 305
y = tan-1(\(\frac{b-h}{x}\)) – tan-1(\(\frac{a-h}{k}\))
y = tan-1(\(\frac{299}{x}\)) – tan-1(\(\frac{148}{x}\))
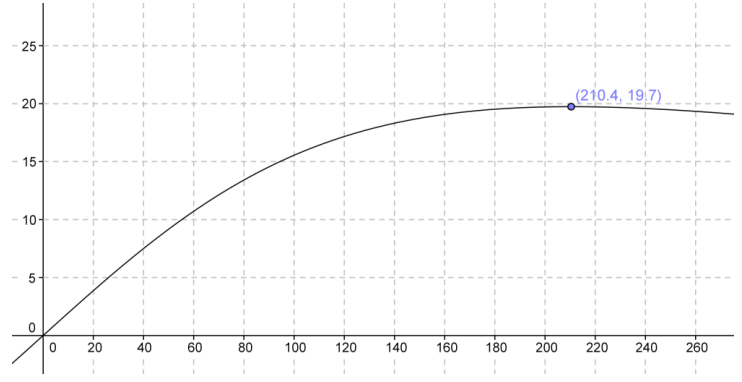
The ideal viewing distance is approximately 210.4 feet from the base of the statue, which produces a maximum viewing angle of approximately 19.7°.
c. What would be your best viewing distance from the statue?
Answer:
Answers will vary. For example, for a viewer whose height is 5.5 feet:
y = tan-1 (\(\frac{299.5}{x}\)) – tan-1 (\(\frac{148.5}{x}\)), which has a maximum y value of 19.7° when x = 210.9. Therefore, the best viewing distance is 210.9 feet from the base of the statue.
d. If there are 66 meters of dry land in front of the statue, is the viewer still on dry land at the best viewing distance?
Answer:
Since 66 meters is approximately 216.5 feet, the observer would be on land at our best viewing angle with about 6 feet between him or her and the water.
Eureka Math Precalculus Module 4 Lesson 13 Exercise Answer Key
Exercise
Hanging on a museum wall is a picture with base a inches above a viewer’s eye level and top b inches above the viewer’s eye level.
a. Model the situation with a diagram.
Answer:
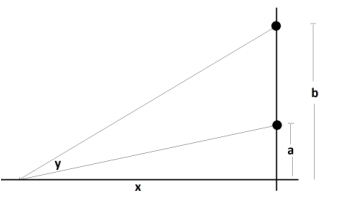
b. Determine an expression that could be used to find the ideal viewing distance x that maximizes the viewing angle y.
Answer:
y = tan-1 (\(\frac{b}{x}\)) – tan-1 (\(\frac{a}{x}\))
c. Find the ideal viewing distance, given the a and b values assigned to you. Calculate the maximum viewing angle in degrees.
Answer:
Answers will vary. An example of an appropriate response is shown.
For a = 1 and b = 60, y = tan-1 (\(\frac{60}{x}\)) – tan-1 (\(\frac{1}{x}\))
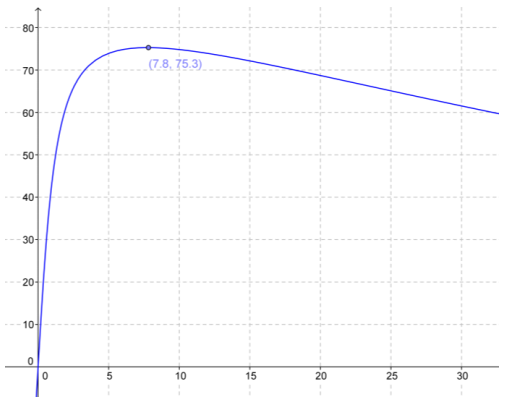
The ideal viewing distance is approximately 7.8 inches, with a maximized viewing angle of 75.3°.
d. Complete the table using class data, which indicates the ideal values for x given different assigned values of a and b. Note any patterns you see in the data.
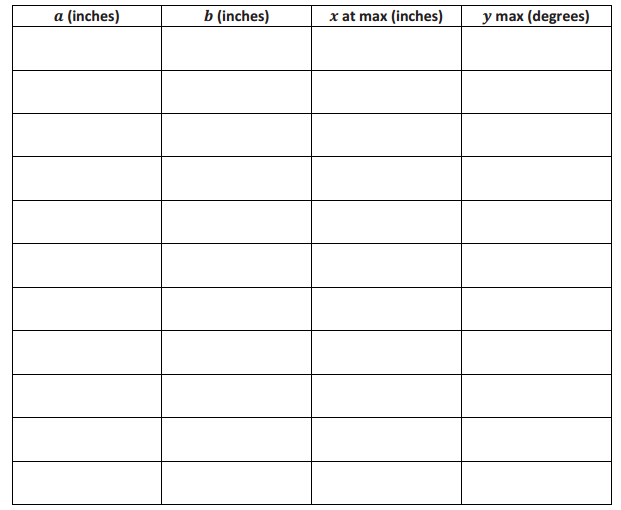
Answer:
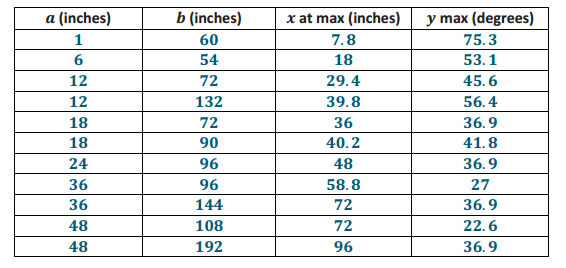
It appears that x is the geometric mean of a and b.
Eureka Math Precalculus Module 4 Lesson 13 Problem Set Answer Key
Question 1.
Consider the situation of sitting down with eye level at 46 in. Find the missing distances and heights for the following:
a. The bottom of the picture is at 50 in. and the top is at 74 in. What is the optimal viewing distance?
Answer:
\(\sqrt{4 \cdot 28}\) = 4\(\sqrt{7}\) ≈ 10.583
About 10.6 in. away
b. The bottom of the picture is at 52 in. and the top is at 60 in. What is the optimal viewing distance?
Answer:
\(\sqrt{6 \cdot 14}\) = 2\(\sqrt{21}\) ≈ 9.165
About 9.2 in. away
c. The bottom of the picture is at 48 in. and the top is at 64 in. What is the optimal viewing distance?
Answer:
\(\sqrt{2 \cdot 18}\) = 6
6 in. away
d. What is the height of the picture if the optimal viewing distance is 1 ft. and the bottom of the picture is hung at 47 in.?
Answer:
12 = \(\sqrt{1 \cdot x}\)
x = 144
12 ft. above eye level, so 15 ft. 10 in. tall
Question 2.
Consider the situation where you are looking at a painting a inches above your line of sight and b inches below your line of sight.
a. Find the optimal viewing distance if it exists.
Answer:
It is not humanly possible to maximize the optimal viewing distance. The closer you get to the painting, the larger the angles become. Eventually, the picture fully encompasses the field of view of the person.
b. If the average standing eye height of Americans is 61.4 in., at what height should paintings and other works of art be hung?
Answer:
The center of the artwork should be hung at eye level so that viewers can get the largest image of the picture they want by getting closer to the image without having to worry about finding the optimal viewing distance.
Question 3.
The amount of daylight per day is periodic with respect to the day of the year. The function y = -3.016 cos(\(\frac{2 \pi x}{365}\)) + 12.25 gives the number of hours of daylight in New York, y, as a function of the number of days since the winter solstice (December 22), which is represented by x.
a. On what days will the following hours of sunlight occur?
i. 15 hours, 15 minutes
Answer:
15.25 = -3.016 cos(\(\frac{2 \pi x}{365}\)) + 12.25
3 = -3.016 cos(\(\frac{2 \pi x}{365}\))
-0.995 = cos(\(\frac{2 \pi x}{365}\))
cos-1 (-0.995) = cos-1(cos(\(\frac{2 \pi x}{365}\)))
(\(\frac{2 \pi x}{365}\)) = 3.0416 and 3.2416
x ≈ 177 and 188
On the 177th and 188th days
ii. 12 hours
Answer:
On the 86th and 279th days
iii. 9 hours, 15 minutes
Answer:
On the 6th and 359th days
iv. 10 hours
Answer:
On the 42nd and 323rd days
v. 9 hours
Answer:
This will never happen. The function does not go that low.
b. Give a function that will give the day of the year from the solstice as a function of the hours of daylight.
Answer:
y = \(\frac{365}{2 \pi}\) ⋅ cos-1(-\(\frac{x-12.25}{3.016}\))
c. What is the domain of the function you gave in part (b)?
Answer:
This function is only accurate for as long as the argument to the inverse cosine stays between -1 and 1, so |x – 12.25|≤3.016, which tells us 9.234 ≤ x ≤ 15.266.
d. What does the domain tell you in the context of the problem?
Answer:
The hours of sunlight for the year vary between about 9 hours, 14 minutes and 15 hours, 16 minutes.
e. What is the range of the function? Does this make sense in the context of the problem? Explain.
Answer:
The range of the function is 0 to 182.5. This only covers half of the year because if the entire year was covered, the inverse would not be a function. We can find other dates with the same amount of daylight by subtracting the number of the day from 365.
Question 4.
Ocean tides are an example of periodic behavior. At a particular harbor, data was collected over the course of
24 hours to create the following model: y = 1.236 sin(\(\frac{\pi}{3}\) x) + 1.798, which gives the water level, y, in feet above the MLLW (mean lower low water) as a function of the time, x, in hours.
a. How many periods are there each day?
Answer:
Four
b. Write a function that gives the time in hours as a function of the water level. How many other times per day will have the same water levels as those given by the function?
Answer:
y = \(\frac{3}{\pi}\) sin-1(\(\frac{x-1.798}{1.236}\))
There are potentially eight times per day that have the same water level (with the exception of peaks and troughs, which only occur four times per day).
Eureka Math Precalculus Module 4 Lesson 13 Exit Ticket Answer Key
Question 1.
The pedestal that the Statue of Liberty sits on is 89 ft. tall with a foundation fashioned in the shape of an eleven-point star making up the rest of the height. The front point of the star juts out about 145 ft. from the front of the statue and stands about 35.2 ft. tall.
How far from the Statue of Liberty does someone whose eye-height is 6 ft. need to stand in order to see the base of the statue without being obscured by the foundation? Include a diagram and appropriate work to justify your answer.
Answer:
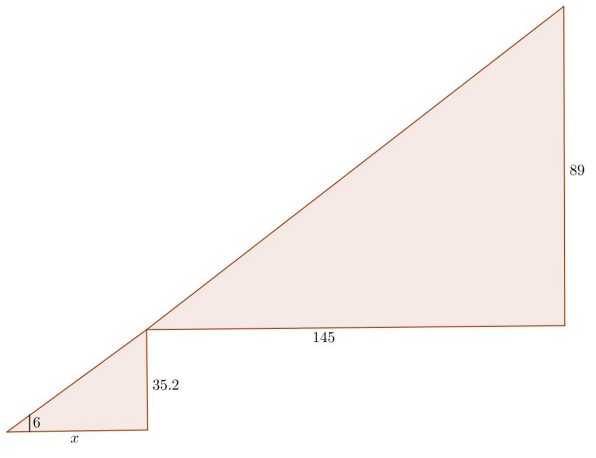
Note that the larger triangle is similar to the smaller triangle, so the angles are equal. This tells us that
tan-1(\(\frac{89 + 35.2-6}{x + 145}\)) = tan-1(\(\frac{35.2-6}{x}\))
\(\frac{118.2}{x + 142}\) = \(\frac{29.2}{x}\)
118.2x = 29.2x + 4234
89x = 4234
x ≈ 47.6
The person will have to stand about 47.6 ft. away from the point of the star.