Engage NY Eureka Math Precalculus Module 2 Lesson 17 Answer Key
Eureka Math Precalculus Module 2 Lesson 17 Opening Exercise Answer Key
Opening Exercise:
When an earthquake hits, the ground shifts abruptly due to forces created when the tectonic plates along fault lines rub together. As the tectonic plates shift and move, the intense shaking can even cause the physical movement of objects as large as buildings.
Suppose an earthquake causes all points in a town to shift 10 feet to the north and 5 feet to the east.
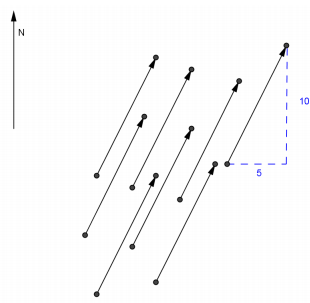
a. Explain how the diagram shown above could be said to represent the shifting caused by the earthquake.
Answer:
The arrows show the amount and direction of the shift. Each point, not just the ones represented in this diagram, would be shifted 5 feet east and 10 feet north.
b. Draw another arrow that shows the same shift. Explain how you drew your arrow.
Answer:
The new arrow would be shifted 5 feet east and 10 feet north from its initial point.
Eureka Math Precalculus Module 2 Lesson 17 Exercise Answer Key
Exercises 1 – 3:
Several vectors are represented in the coordinate plane below using arrows.
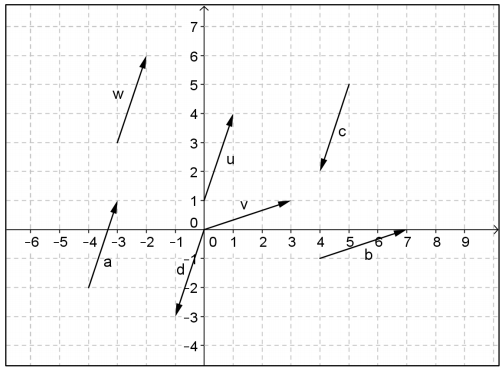
Exercise 1.
Which arrows represent the same vector? Explain how you know.
Answer:
Arrows w, u, and a represent the same vector because they indicate a translation of 1 unit right and 3 units up. Arrows y and b represent the same vector because these arrows indicate a translation of 3 units right and 1 unit up. Arrows c and d represent the same vector because they represent a translation of 1 unit left and 3 units down.
Exercise 2.
Why do arrows c and u not represent the same vector?
Answer:
These arrows have the same magnitude but opposite directions.
Exercise 3.
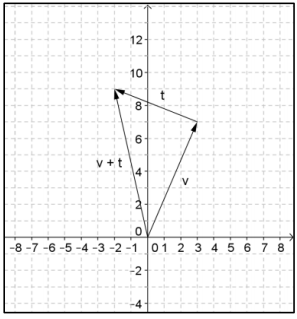
After the first earthquake shifted points 5 feet east and 10 feet north, suppose a second earthquake hits the town and all points shift 6 feet east and 9 feet south.
a. Write and draw a vector t that represents this shift caused by the second earthquake.
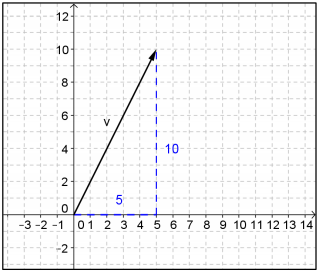
Answer:
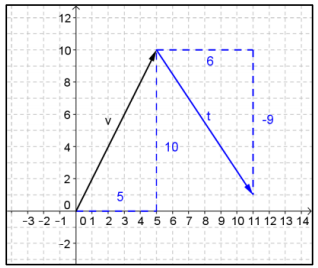
b. Which earthquake, the first one or the second one, shifted all the points in the town further? Explain your reasoning.
Answer:
The length of the arrow that represents the vector y is \(\sqrt{5^{2}+10^{2}}\), or \(\sqrt{125}\) feet. The length of the arrow that represents the vector t is \(\sqrt{6^{2}+(-9)^{2}}\), or \(\sqrt{117}\) feet. The first quake shifted the points further. You can also see from the diagram that if we rotated t from the tip of v to align with v, then t would be slightly shorter.
Exercises 4 – 10:
Exercise 4.
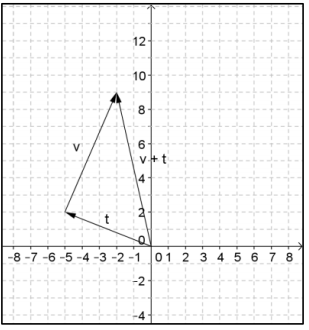
Given that v (3, 7) and t (-5, 2)
a. What is v + t?
Answer:
v + t = (3 + (-5), 7 + 2) = (-2, 9)
b. Draw a diagram that represents this addition and shows the resulting sum of the two vectors.
Answer:
c. What is t + v?
Answer:
t + v = (-2, 9)
d. Draw a diagram that represents this addition and shows the resulting sum of the two vectors.
Answer:
Exercise 5.
Explain why vector addition is commutative.
Answer:
Since we are combining two horizontal and two vertical translations when we add vectors, the end result will be the same regardless of the order in which we apply the translations. Thus, when we add two vectors, it doesn’t matter which comes first and which comes second. Using the rule we were given, we can see that the components represent real numbers, and thus, the commutative property should apply to each component of the resulting sum vector.
Exercise 6.
Given v = (3, 7) and t = (-5, 2).
a. Show numerically that ||v|| + ||t|| ≠ ||v + t||.
Answer:
||v|| = \(\sqrt{3^{2}+7^{2}}=\sqrt{58}\) and ||t|| = \(\sqrt{(-5)^{2}+2^{2}}\) = √29
v + t = (-2, 9) and ||v + t|| = \(\sqrt{(-2)^{2}+(9)^{2}}\) = √85
√58 + √29 ≠ √85. This can be confirmed quickly using approximations for each square root.
b. Provide a geometric argument to explain, in general, why the sum of the magnitudes of two vectors is not equal to the magnitude of the sum of the vectors.
Answer:
When added end-to-end, two vectors and the resulting sum vector lie on the sides of a triangle. Since the sum of any two sides of a triangle must be longer than the third side, and the magnitude of the vectors would correspond to the lengths of the sides of the triangle, this statement cannot be true.
c. Can you think of an example of when the statement would be true? Justify your reasoning.
Answer:
This statement would be true if one of the vectors had a magnitude of 0. The sum of the vectors would be equal to the original nonzero vector, so they would have the same magnitude.
Exercise 7.
Why is the vector o = (0, 0) called the zero vector? Describe its geometric effect when added to another vector.
Answer:
The magnitude of this vector is o, and its components are 0. It maps the pre-image vector onto itself and essentially has no translational effect on the original vector, It has the same effect as add0ing the real-number 0 to any other real number.
Exercise 8.
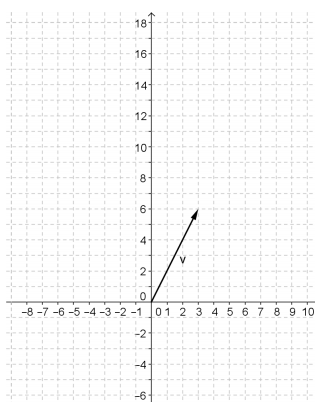
Given the vectors shown below
y = (3, 6)
u = (9, 18)
w = (-3, -6)
s = (1, 2)
t = (-1.5, -3)
r = (6, 12)
a. Draw each vector with its initial point located at (0, 0). The vector v is already shown. How are all of these vectors related?
Answer:
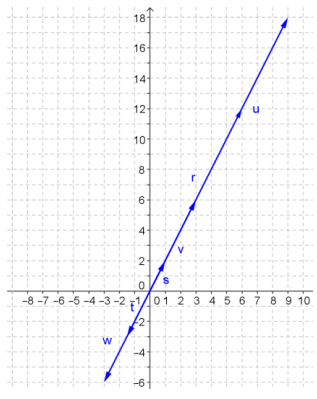
All of these vectors lie on the same line that passes through the origin. If you dilate with center (0, 0), then each vector is a dilation of every other vector in the list.
b. Which vector is 2v? Explain how you know.
Answer:
The vector 2v is twice as long as v and points in the same direction. The components would be doubled so r = 2v.
c. Describe the remaining vectors as a scalar multiple of y = (3, 6) and explain your reasoning.
u = 3v
w = -v
s = \(\frac{1}{3}\)v
t = –\(\frac{1}{2}\)v
d. Is the vector p = (3√2, 6√2) a scalar multiple of v? Explain.
Answer:
Yes; p = √2v. You can see that if the initial point were located at (0, 0), then the vector p would also lie on the line through the origin that contains the other vectors in this exercise.
Exercise 9.
Which vector from Exercise 8 would It make sense to call the opposite of y = (3, 6)?
Answer:
You could call w the opposite of v because w has the same length as v, and it has the opposite direction.
Exercise 10.
Describe a rule that defines vector subtraction. Use the vectors v = (5, 7) and u = (6, 3) to support your reasoning.
Answer:
Since adding two real numbers is adding the opposite of the second number to the first number, it makes sense that
vector subtraction would work in a similar way. To subtract two vectors, you add the opposite of the second vector or, more simply, just subtract the components.
We can create the opposite of u by multiplying by the scalar -1.
v – u = v + (-u) = (5 + (-6), 7 + (-3)) = (-1, 4)
or simply subtracting the components gives
v – u = (5 – 6, 7 – 3) = (-1, 4)
Eureka Math Precalculus Module 2 Lesson 17 Example Answer Key
The magnitude of a vector y = (a, b) is the length of the line segment from the origin to the point (a, b) in the coordinate plane, which we denote by ||v||. Using the language of translation, the magnitude of y is the distance between any point and its image under the translation a units horizontally and b units vertically. It is denoted ||v||.
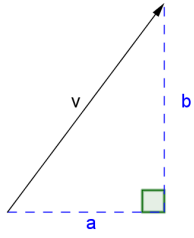
a. Find the magnitude of y = (5, 10) and t = (6, -9). Explain your reasoning.
Answer:
||v|| = \(\sqrt{5^{2}+10^{2}}\) = \(\sqrt{125}\)
||t|| = \(\sqrt{6^{2}+(-9)^{2}}\) = \(\sqrt{117}\)
We use the Pythagorean theorem (or distance formula) to find the length of the hypotenuse of a triangle with side lengths a and b
b. Write the general formula for the magnitude of a vector.
Answer:
||v|| = \(\sqrt{a^{2}+b^{2}}\)
Eureka Math Precalculus Module 2 Lesson 17 Problem Set Answer Key
Question 1.
Sasha says that a vector has a direction component in it; therefore, we cannot add two vectors or subtract one from the other. His argument is that we cannot add “east” to “north” nor subtract “east” from “north,” for instance. Therefore, he claims, we cannot add or subtract vectors.
a. Is he correct? Explain your reasons.
Answer:
No, Sasha is not correct. Although a vector has a magnitude and direction, and it is numerically suited to do translation of an object, it has horizontal and vertical components indicating how many units for translation. Therefore, we can add and subtract vectors.
b. What would you do if you need to add two vectors, u and y, or subtract vector y from vector arithmetically?
Answer:
For addition, we add the same corresponding vector components. For example, u = (u1, u2), v = (v1, v2)
u + v = (u1 + v1, u2 + v2).
For subtraction, u – v = u + (-v) = (u1 + (-v1), u2 + (-v2) = (u1 – v1, u2 – v2).
Question 2.
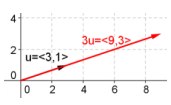
Given u = (3, 1) and y = (-4, 2), write each vector in component form, graph it, and explain the geometric effect.
a. 3u
Answer:
3u = (9, 3)
The vector is dilated by a factor of 3 and the direction stays the same.
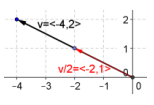
b. \(\frac{1}{2}\)v
Answer:
\(\frac{1}{2}\)v = (-2, 1)
The vector is dilated by a factor of \(\frac{1}{2}\), and the direction stays the same.
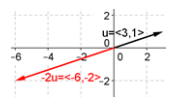
c. -2u
Answer:
2u = (-6, -2)
The vector is dilated by a factor of 2, and the direction is reversed.
d. -v
Answer:
-v = (4, -2).
The length of the vector is unchanged, and the direction is reversed.
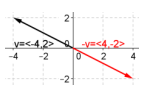
e. u + v
Answer:
u + v = (-1, 3)
When adding vector u = (3, 1) onto vector v = (-4, 2), from the tip of vector v, we move 3 units to the right and 1 unit upward, and the resultant vector is (-1, 3).
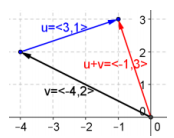
f. 2u + 3v
Answer:
2u + 3v = (-6, 8)
When adding vector 2u = (6, 2) onto vector 3v = (-12, 6), from the tip of vector 3v, we move 6 units to the right and 2 units upward, and the resultant vector is (-6, 8).
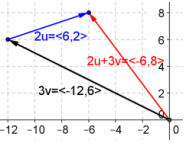
g. 4u – 3v
Answer:
4u – 3v = 4u + (-3v) = (24, -2)
When adding vector 4u = (12, 4) onto vector -3v = (12, -6), from the tip of vector -3v, we move 12 units to the right and 4 units upward. The resultant vector is (24, -2).
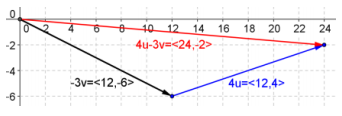
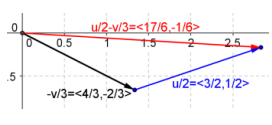
h. \(\frac{1}{2}\)u – \(\frac{1}{3}\)v
Answer:
\(\frac{1}{2}\)u – \(\frac{1}{3}\)v = \(\frac{1}{2}\)u + (-\(\frac{1}{3}\)v) = (\(\frac{17}{6}\),-\(\frac{1}{6}\))
When adding vector \(\frac{1}{2}\)u = (\(\frac{3}{2}\), \(\frac{1}{2}\)) onto vector –\(\frac{1}{3}\)v = (-\(\frac{4}{3}\), 1), from the tip of vector –\(\frac{1}{3}\)v, we move \(\frac{3}{2}\) units to the right and unit upward, and the resultant vector is (\(\frac{17}{6}\), –\(\frac{1}{6}\)).
Question 3.
Given u = (3, 1) and v = (-4, 2), find the following.
a. ||u||
Answer:
√10
b. ||v||
Answer:
2√5
c. ||2u|| and 2||u||
Answer:
||2u|| = √40 = 2√10. 2||u|| = 2√10.
d.||\(\frac{1}{2}\)v|| and \(\frac{1}{2}\)||v||
Answer:
\(\frac{1}{2}\)v = (-2, 1), ||\(\frac{1}{2}\)v|| = √5 . \(\frac{1}{2}\)||v|| = \(\frac{1}{2}\)√5.
e. Is ||u + v|| equal to ||u|| + ||v||? Expalin how you know.
Answer:
No. We have
u + v = (-1, 3) ||u + v|| = √10 and ||u|| + ||v|| = √10 + 2√5.
g. Is ||u – v|| equal to |u|| – ||v||? Explain how you know.
Answer:
No. u – v = (7, -1). we have ||u – v|| = √50 = 5√2, but ||u|| – ||v|| = √10 – 2√5.
Question 4.
Given u = (1, 2), y = (3, -4), and w = (-4, 6), show that (u + v) + w = u + (v + w).
Answer:
(u + v) + w = (4, -2) + (-4, 6) = (0, 4)
u + (v + w) = (1, 2) +(-1, 2) = (0, 4)
Question 5.
Tyiesha says that if the magnitude of a vector u is zero, then u has to be a zero vector. Is she correct? Explain how you know.
Answer:
Yes. Suppose that u = (u1, u2), and ||u|| = \(\sqrt{\left(\mathbf{u}_{1}\right)^{2}+\left(\mathbf{u}_{2}\right)^{2}}\) = 0, so (u1)2 + (u2)2 = 0. Then (u1)2 and (u2)2 are non-negative numbers, and if two non-negative numbers sum to zero, then both numbers must be zero. Then u1 = 0 and u2 = 0, which proves u is a zero vector.
Question 6.
Sergei experienced one of the biggest earthquakes when visiting Taiwan in 1999. He noticed that his refrigerator moved on the wooden floor and made marks on it. By measuring the marks he was able to trace how the refrigerator moved. The first move was northeast with a distance of 20 cm. The second move was northwest with a distance of 10 cm.
The final move was northeast with a distance of 5 cm. Find the vectors that would re-create the refrigerator’s movement on the floor and find the distance that the refrigerator moved from its original spot to its resting place. Draw a diagram of these vectors.
Answer:
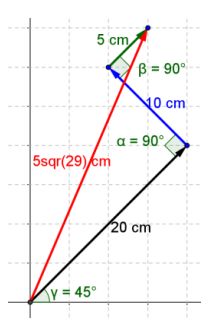
The first vector v1 = (10√2, 10√2).
The second vector v2 = (-5√2, 5√2).
The third vector v3 = \(\left\langle\frac{5 \sqrt{2}}{2}, \frac{5 \sqrt{2}}{2}\right\rangle\)
The resultant vector is
v = v1 + v2 + v3 = \(\left\langle\frac{15 \sqrt{2}}{2}, \frac{35 \sqrt{2}}{2}\right\rangle\).
||v|| = \(\sqrt{\left(\frac{15 \sqrt{2}}{2}\right)^{2}+\left(\frac{35 \sqrt{2}}{2}\right)^{2}}\) = 5√29
The magnitude is 5√29 cm.
Eureka Math Precalculus Module 2 Lesson 17 Exit Ticket Answer Key
Question 1.
Vector v = (3, 4) and the vector u is represented by the arrow shown below. How are the vectors the same? How are they different?
Answer:
Both u and v have the same length and direction, so they are different representations of the same vector. They both represent a translation of 3 units right and 4 units up.
Question 2.
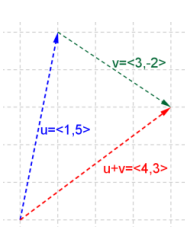
Let u = (1, 5) and y = (3, -2). Write each vector in component form and draw an arrow to represent the vector.
a. u + v
Answer:
u + v = (4, 3)
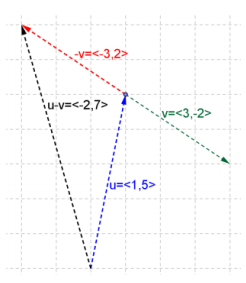
b. u – v
Answer:
u – v = u + (-v) = (-2, 7)
c. 2u + 3v
Answer:
2u + 3v = (11, 4)
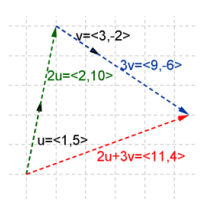
Question 3.
For u = (1, 5) and v = (3, -2) as in problem 2, part (a), What is the magnitude of +v?
Answer:
||u + v|| = (4, 3) = -5