Engage NY Eureka Math 8th Grade Module 7 Lesson 8 Answer Key
Eureka Math Grade 8 Module 7 Lesson 8 Example Answer Key
Example 1.
Show that the decimal expansion of \(\frac{26}{4}\) is 6.5.
Answer:
Use the example with students so they have a model to complete Exercises 1–5.
→ Show that the decimal expansion of \(\frac{26}{4}\) is 6.5.
Students might use the long division algorithm, or they might simply observe \(\frac{26}{4}\) = \(\frac{13}{2}\) = 6.5.
→ Here is another way to see this: What is the greatest number of groups of 4 that are in 26?
There are 6 groups of 4 in 26.
→ Is there a remainder?
Yes, there are 2 left over.
→ This means we can write 26 as
26 = 6 × 4 + 2.
This means we could also compute \(\frac{26}{4}\) as follows:
\(\frac{26}{4}\) = \(\frac{6 \times 4 + 2}{4}\)
\(\frac{26}{4}\) = \(\frac{6 \times 4}{4}\) + \(\frac{2}{4}\)
\(\frac{26}{4}\) = 6 + \(\frac{2}{4}\)
\(\frac{26}{4}\) = 6\(\frac{2}{4}\) = 6 \(\frac{1}{2}\).
(Some students might note we are simply rewriting the fraction as a mixed number.)
The fraction \(\frac{26}{4}\) is equal to the finite decimal 6.5. When the fraction is not equal to a finite decimal, then we need to use the long division algorithm to determine the decimal expansion of the number.
Eureka Math Grade 8 Module 7 Lesson 8 Exploratory Challenge/Exercise Answer Key
Exploratory Challenge/Exercises 1–5
Exercise 1.
a. Use long division to determine the decimal expansion of \(\frac{142}{2}\).
Answer:
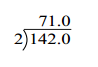
b. Fill in the blanks to show another way to determine the decimal expansion of \(\frac{142}{2}\).
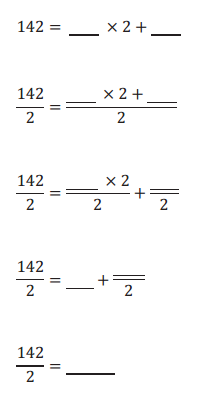
Answer:
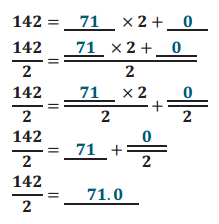
c. Does the number \(\frac{142}{2}\) have a finite or an infinite decimal expansion?
Answer:
The decimal expansion of \(\frac{142}{2}\) is 71.0 and is finite.
Exercise 2.
a. Use long division to determine the decimal expansion of \(\frac{142}{4}\).
Answer:
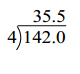
b. Fill in the blanks to show another way to determine the decimal expansion of \(\frac{142}{4}\).
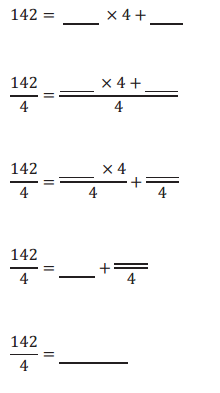
Answer:
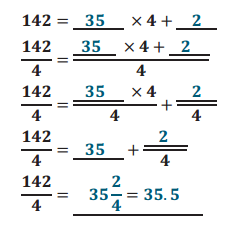
c. Does the number \(\frac{142}{4}\) have a finite or an infinite decimal expansion?
Answer:
The decimal expansion of \(\frac{142}{4}\) is 35.5 and is finite.
Exercise 3.
a. Use long division to determine the decimal expansion of \(\frac{142}{6}\).
Answer:
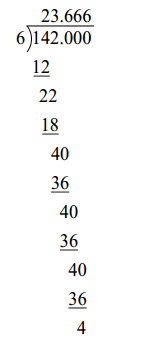
b. Fill in the blanks to show another way to determine the decimal expansion of \(\frac{142}{6}\).
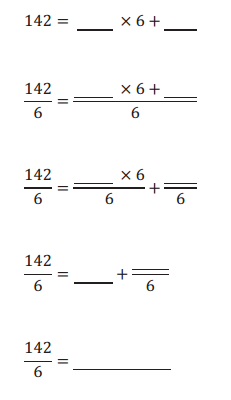
Answer:
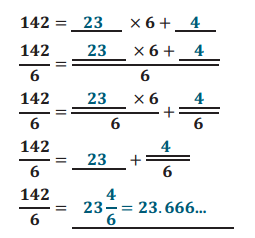
c. Does the number \(\frac{142}{6}\) have a finite or an infinite decimal expansion?
Answer:
The decimal expansion of \(\frac{142}{6}\) is 23.666… and is infinite.
Exercise 4.
a. Use long division to determine the decimal expansion of \(\frac{142}{11}\).
Answer:
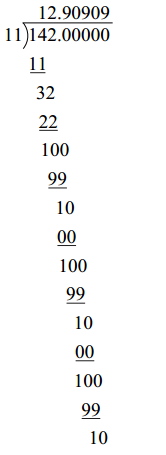
b. Fill in the blanks to show another way to determine the decimal expansion of \(\frac{142}{11}\).
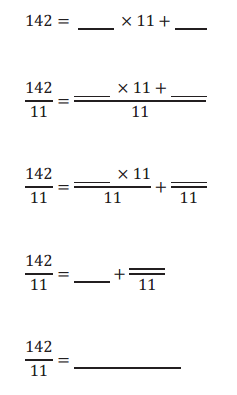
Answer:
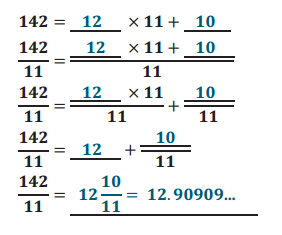
c. Does the number \(\frac{142}{11}\) have a finite or an infinite decimal expansion?
Answer:
The decimal expansion of \(\frac{142}{11}\) is 12.90909… and is infinite.
Exercise 5.
In general, which fractions produce infinite decimal expansions?
Answer:
We discovered in Lesson 6 that fractions equivalent to ones with denominators that are a power of 10 are precisely the fractions with finite decimal expansions. These fractions, when written in simplified form, have denominators with factors composed of 2‘s and 5‘s. Thus any fraction, in simplified form, whose denominator contains a factor different from 2 or 5 must yield an infinite decimal expansion.
Exercises 6–10
Exercise 6.
Does the number \(\frac{65}{13}\) have a finite or an infinite decimal expansion? Does its decimal expansion have a repeating pattern?
Answer:
The number \(\frac{65}{13}\) is rational and so has a decimal expansion with a repeating pattern. Actually, \(\frac{65}{13}\) = \(\frac{5 \times 13}{13}\) = 5, so it is a finite decimal. Viewed as an infinite decimal, \(\frac{65}{13}\) is 5.0000… with a repeat block of 0.
Exercise 7.
Does the number \(\frac{17}{11}\) have a finite or an infinite decimal expansion? Does its decimal expansion have a repeating pattern?
Answer:
The rational \(\frac{17}{11}\) is in simplest form, and we see that it is not equivalent to a fraction with a denominator that is a power of 10. Thus, the rational has an infinite decimal expansion with a repeating pattern.
Exercise 8.
Is the number 0.212112111211112111112… rational? Explain. (Assume the pattern you see in the decimal expansion continues.)
Answer:
Although the decimal expansion of this number has a pattern, it is not a repeating pattern. The number cannot be rational. It is irrational.
Exercise 9.
Does the number \(\frac{860}{999}\) have a finite or an infinite decimal expansion? Does its decimal expansion have a
repeating pattern?
Answer:
The number is rational and so has a decimal expansion with a repeating pattern. Since the fraction is not equivalent to one with a denominator that is a power of 10, it is an infinite decimal expansion.
Exercise 10.
Is the number 0.1234567891011121314151617181920212223… rational? Explain. (Assume the pattern you see in the decimal expansion continues.)
Answer:
Although the decimal expansion of this number has a pattern, it is not a repeating pattern. The number cannot be rational. It is irrational.
Eureka Math Grade 8 Module 7 Lesson 8 Problem Set Answer Key
Question 1.
Write the decimal expansion of \(\frac{7000}{9}\) as an infinitely long repeating decimal.
Answer:
\(\frac{7000}{9}\) = \(\frac{777 \times 9}{9}\) + \(\frac{7}{9}\)
= \(\frac{7777}{9}\)
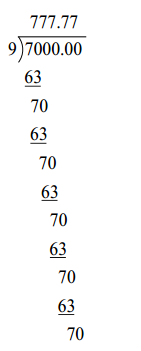
The decimal expansion of \(\frac{7000}{9}\) is \(777 . \overline{7}\)
Question 2.
Write the decimal expansion of \(\frac{6555555}{3}\) as an infinitely long repeating decimal.
Answer:
\(\frac{6555555}{3}\) = \(\frac{2185185 \times 3}{3}\) + \(\frac{0}{3}\)
= 2 185 185
The decimal expansion of \(\frac{6555555}{3}\) is \(2,185,185 . \overline{0}\).
Question 3.
Write the decimal expansion of \(\frac{350000}{11}\) as an infinitely long repeating decimal.
Answer:
\(\frac{350000}{11}\) = \(\frac{31818 \times 11}{11}\) + \(\frac{2}{11}\)
= 31818\(\frac{2}{11}\)
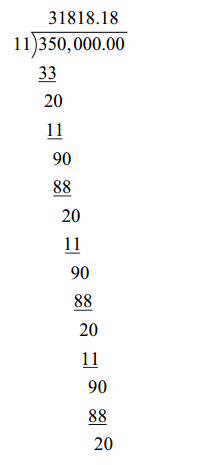
The decimal expansion of \(\frac{350000}{11}\) is \(31,818 . \overline{18}\).
Question 4.
Write the decimal expansion of \(\frac{12000000}{37}\) as an infinitely long repeating decimal.
Answer:
\(\frac{12000000}{37}\) = \(\frac{324324 \times 37}{37}\) + \(\frac{12}{37}\)
= 324324\(\frac{12}{37}\)
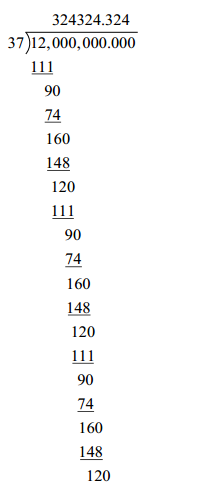
The decimal expansion of \(\frac{12000000}{37}\) is \(324,324 . \overline{324}\).
Question 5.
Someone notices that the long division of 2,222,222 by 6 has a quotient of 370,370 and a remainder of 2 and wonders why there is a repeating block of digits in the quotient, namely 370. Explain to the person why this happens.
Answer:
\(\frac{2222222}{6}\) = \(\frac{370370 \times 6}{6}\) + \(\frac{2}{6}\)
= 370370 \(\frac{2}{6}\)
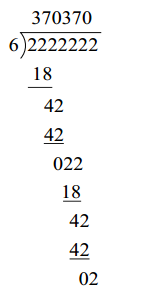
The block of digits 370 keeps repeating because the long division algorithm leads us to perform the same division over and over again. In the algorithm shown above, we see that there are three groups of 6 in 22, leaving a remainder of 4. When we bring down the next 2, we see that there are exactly seven groups of 6 in 42. When we bring down the next 2, we see that there are zero groups of 6 in 2, leaving a remainder of 2. It is then that the process starts over because the next step is to bring down another 2, giving us 22, which is what we started with. Since the division repeats, then the digits in the quotient will repeat.
Question 6.
Is the answer to the division problem 10÷3.2 a rational number? Explain.
Answer:
Yes. This is equivalent to the division problem 100÷32, which can be written as \(\frac{100}{32}\), and so it is a rational number.
Question 7.
Is \(\frac{3 \pi}{77 \pi}\) a rational number? Explain.
Answer:
Yes. \(\frac{3 \pi}{77 \pi}\) is equal to \(\frac{3}{77}\) and so it is a rational number.
Question 8.
The decimal expansion of a real number x has every digit 0 except the first digit, the tenth digit, the hundredth digit, the thousandth digit, and so on, are each 1. Is x a rational number? Explain.
Answer:
No. Although there is a pattern to this decimal expansion, it is not a repeating pattern. Thus, x cannot be rational.
Eureka Math Grade 8 Module 7 Lesson 8 Exit Ticket Answer Key
Question 1.
Will the decimal expansion of \(\frac{125}{8}\) be finite or infinite? Explain. If we were to write the decimal expansion of this rational number as an infinitely long decimal, which block of numbers repeat?
Answer:
The decimal expansion of \(\frac{125}{8}\) will be finite because \(\frac{125}{8}\) is equivalent to a fraction with a denominator that is a power of 10. (Multiply the numerator and denominator each by 5 × 5 × 5.) If we were to write the decimal as an infinitely long decimal, then we’d have a repeating block consisting of 0.
Question 2.
Write the decimal expansion of \(\frac{13}{7}\) as an infinitely long repeating decimal.
Answer:
\(\frac{13}{7}\) = \(\frac{1 \times 7}{7}\) + \(\frac{6}{7}\)
= 1\(\frac{6}{7}\)
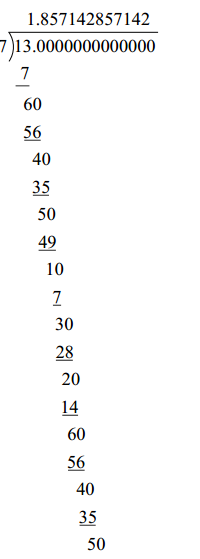
The decimal expansion of \(\frac{13}{7}\) is \(1 . \overline{857142}\).