Eureka Math Grade 8 Module 7 Lesson 20 Answer Key

Engage NY Eureka Math 8th Grade Module 7 Lesson 20 Answer Key

Eureka Math Grade 8 Module 7 Lesson 20 Example Answer Key

Example 1.
Determine the volume of the truncated cone shown below.

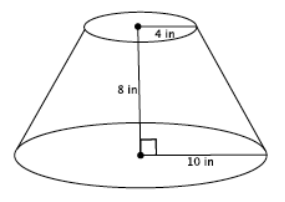
Answer:
Since we know that the original cone and the portion that has been removed to make this truncated cone are similar, let’s begin by drawing in the missing portion.

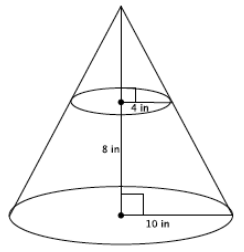
→ We know the formula to find the volume of a cone. Is there enough information in the new diagram for us to find the volume? Explain.
→ No, there’s not enough information. We would have to know the height of the cone, and at this point we only know the height of the truncated cone, 8 inches.
→ Recall our conversation about the similar right triangles. We can use what we know about similarity to determine the height of the cone with the following proportion. What does each part of the proportion represent in the diagram?
Since the triangles are similar, we will let x inches represent the height of the cone that has been removed.
\(\frac{4}{10}\) = \(\frac{x}{x + 8}\)
The 4 is the radius of the small cone. The 10 is the radius of the large cone. The x represents the height of the small cone. The expression x + 8 represents the height of the large cone.
Work in your groups to determine the height of the small cone.
4(x + 8) = 10x
4x + 32 = 10x
32 = 6x
\(\frac{32}{6}\) = x
\(5 . \overline{3}\) = x
→ Now that we know the height of the cone that has been removed, we also know the total height of the cone. How might we use these pieces of information to determine the volume of the truncated cone?
We can find the volume of the large cone, find the volume of the small cone that was removed, and then subtract the volumes. What will be left is the volume of the truncated cone.

→ Write an expression that represents the volume of the truncated cone. Use approximations for the heights since both are infinite decimals. Be prepared to explain what each part of the expression represents in the situation.
The volume of the truncated cone is given by the expression
\(\frac{1}{3}\) π(10)² (13.3) – \(\frac{1}{3}\) π(4)² (5.3),
where \(\frac{1}{3}\) π(10)² (13.3) is the volume of the large cone, and \(\frac{1}{3}\) π(4)² (5.3) is the volume of the smaller cone. The difference in the volumes will be the volume of the truncated cone.
Determine the volume of the truncated cone. Use the approximate value of the number \(5 . \overline{3}\) when you compute the volumes.

The volume of the small cone is
V ≈ \(\frac{1}{3}\) π(4)² (5.3)
≈ \(\frac{1}{3}\) π(84.8)
≈ \(\frac{84.8}{3}\) π
The volume of the large cone is
V ≈ \(\frac{1}{3}\)π(10)² (13.3)
≈ \(\frac{1330}{3}\) π
The volume of the truncated cone is
\(\frac{1330}{3}\) π – \(\frac{84.8}{3}\) π = (\(\frac{1330}{3}\) – \(\frac{84.8}{3}\))π
= \(\frac{1245.2}{3}\) π
The volume of the truncated cone is approximately \(\frac{1245.3}{3}\) π in ³.

Write an equivalent expression for the volume of a truncated cone that shows the volume is \(\frac{1}{3}\) of the difference between two cylinders. Explain how your expression shows this.
The expression \(\frac{1}{3}\) π(10)²(13.3) – \(\frac{1}{3}\) π(4)²(5.3) can be written as \(\frac{1}{3}\)(π(10)²(13.3) – π(4)²(5.3)), where π(10)²(13.3) is the volume of the larger cylinder, and π(4)²(5.3) is the volume of the smaller cylinder. One – third of the difference is the volume of a truncated cone with the same base and height measurements as the cylinders.

Eureka Math Grade 8 Module 7 Lesson 20 Exercise Answer Key

Exercises 1–5

Exercise 1.
Find the volume of the truncated cone.

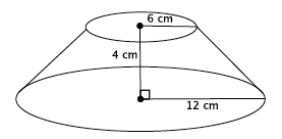
a. Write a proportion that will allow you to determine the height of the cone that has been removed. Explain what all parts of the proportion represent.
Answer:
\(\frac{6}{12}\) = \(\frac{x}{x + 4}\)
Let x cm represent the height of the small cone. Then, x + 4 is the height of the large cone (with the removed part included). The 6 represents the base radius of the removed cone, and the 12 represents the base radius of the large cone.

b. Solve your proportion to determine the height of the cone that has been removed.
Answer:
6(x + 4) = 12x
6x + 24 = 12x
24 = 6x
4 = x

c. Write an expression that can be used to determine the volume of the truncated cone. Explain what each part of the expression represents.
Answer:
\(\frac{1}{3}\) π(12)² (8) – \(\frac{1}{3}\) π(6)² (4)
The expression \(\frac{1}{3}\) π(12)² (8) is the volume of the large cone, and \(\frac{1}{3}\) π(6)² (4) is the volume of the small cone. The difference of the volumes gives the volume of the truncated cone.

d. Calculate the volume of the truncated cone.
Answer:
The volume of the small cone is
V = \(\frac{1}{3}\) π(6)² (4)
= \(\frac{144}{3}\) π.

The volume of the large cone is
V = \(\frac{1}{3}\) π(12)² (8)
= \(\frac{1152}{3}\) π.
The volume of the truncated cone is
\(\frac{1152}{3}\) π – \(\frac{144}{3}\) π = (\(\frac{1152}{3}\) – \(\frac{144}{3}\))π
= \(\frac{1008}{3}\) π
= 336π.
The volume of the truncated cone is 336π cm².

Exercise 2.
Find the volume of the truncated cone.

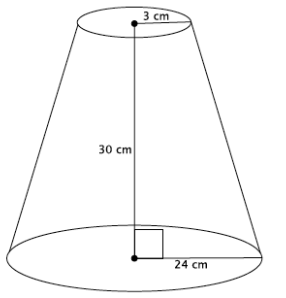
Answer:
Let x cm represent the height of the small cone.
\(\frac{3}{24}\) = \(\frac{x}{x + 30}\)
3x + 90 = 24x
90 = 21x
\(\frac{30}{7}\) = x
4.3 ≈ x

The volume of the small cone is
V ≈ \(\frac{1}{3}\) π(3)² (4.3)
≈ \(\frac{38.7}{3}\) π
= 12.9π.

The volume of the large cone is
V ≈ \(\frac{1}{3}\) π(24)² (34.3)
≈ \(\frac{19756.8}{3}\) π
= 6585.6π.

The volume of the truncated cone is
6585.6π – 12.9π = (6585.6 – 12.9)π
= 6572.7π.
The volume of the truncated cone is approximately 6572.7π cm³.

Exercise 3.
Find the volume of the truncated pyramid with a square base.

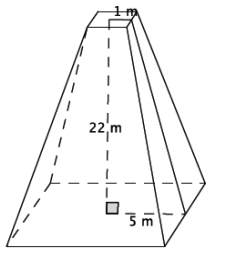
a. Write a proportion that will allow you to determine the height of the cone that has been removed. Explain what all parts of the proportion represent.
Answer:
\(\frac{1}{5}\) = \(\frac{x}{x + 22}\)
Let x m represent the height of the small pyramid. Then x + 22 is the height of the large pyramid. The 1 represents half of the length of the base of the small pyramid, and the 5 represents half of the length of the base of the large pyramid.

b. Solve your proportion to determine the height of the pyramid that has been removed.
Answer:
x + 22 = 5x
22 = 4x
5.5 = x

c. Write an expression that can be used to determine the volume of the truncated pyramid. Explain what each part of the expression represents.
Answer:
\(\frac{1}{3}\) (100)(27.5) – \(\frac{1}{3}\) (4)(5.5)
The expression \(\frac{1}{3}\) (100)(27.5) is the volume of the large pyramid, and \(\frac{1}{3}\) (4)(5.5) is the volume of the small pyramid. The difference of the volumes gives the volume of the truncated pyramid.

d. Calculate the volume of the truncated pyramid.
Answer:
The volume of the small pyramid is
V = \(\frac{1}{3}\) (4)(5.5)
= \(\frac{22}{3}\) .

The volume of the large pyramid is
V = \(\frac{1}{3}\) (100)(27.5)
= \(\frac{2750}{3}\) .

The volume of the truncated pyramid is
\(\frac{2750}{3}\) – \(\frac{22}{3}\) = \(\frac{2728}{3}\)

The volume of the truncated pyramid is \(\frac{2728}{3}\) m³.

Exercise 4.
A pastry bag is a tool used to decorate cakes and cupcakes. Pastry bags take the form of a truncated cone when filled with icing. What is the volume of a pastry bag with a height of 6 inches, large radius of 2 inches, and small radius of 0.5 inches?
Answer:
Let x in. represent the height of the small cone.
\(\frac{x}{x + 6}\) = \(\frac{0.5}{2}\)
2x = 0.5(x + 6)
2x = \(\frac{1}{2}\) x + 3
\(\frac{3}{2}\) x = 3
x = 2
The volume of the small cone is
V = \(\frac{1}{3}\) π(\(\frac{1}{2}\))² (2)
= \(\frac{1}{6}\) π.

The volume of the large cone is
V = \(\frac{1}{3}\) π(2)² (8)
= \(\frac{32}{3}\) π.

The volume of the truncated cone is
\(\frac{32}{3}\) π – \(\frac{1}{6}\) π = (\(\frac{32}{3}\) – \(\frac{1}{6}\))π
= \(\frac{63}{6}\) π
= \(\frac{21}{2}\) π.
The volume of the pastry bag is \(\frac{21}{2}\) π in³ when filled.

Exercise 5.
Explain in your own words what a truncated cone is and how to determine its volume.
Answer:
A truncated cone is a cone with a portion of the top cut off. The base of the portion that is cut off needs to be parallel to the base of the original cone. Since the portion that is cut off is in the shape of a cone, then to find the volume of a truncated cone, you must find the volume of the cone (without any portion cut off), find the volume of the cone that is cut off, and then find the difference between the two volumes. That difference is the volume of the truncated cone.

Eureka Math Grade 8 Module 7 Lesson 20 Problem Set Answer Key

Question 1.
Find the volume of the truncated cone.

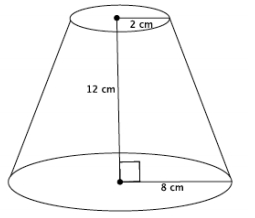
a. Write a proportion that will allow you to determine the height of the cone that has been removed. Explain what each part of the proportion represents.
Answer:
\(\frac{2}{8}\) = \(\frac{x}{x + 12}\)
Let x cm represent the height of the small cone. Then x + 12 is the height of the large cone. The 2 represents the base radius of the small cone, and the 8 represents the base radius of the large cone.

b. Solve your proportion to determine the height of the cone that has been removed.
Answer:
2(x + 12) = 8x
2x + 24 = 8x
24 = 6x
4 = x

c. Show a fact about the volume of the truncated cone using an expression. Explain what each part of the expression represents.
Answer:
\(\frac{1}{3}\) π(8)² (16) – \(\frac{1}{3}\) π(2)² (4)
The expression \(\frac{1}{3}\) π(8)² (16) represents the volume of the large cone, and \(\frac{1}{3}\) π(2)² (4) is the volume of the small cone. The difference in volumes gives the volume of the truncated cone.

d. Calculate the volume of the truncated cone.
Answer:
The volume of the small cone is
V = \(\frac{1}{3}\) π(2)² (4)
= \(\frac{16}{3}\) π.

The volume of the large cone is
V = \(\frac{1}{3}\) π(8)² (16)
= \(\frac{1024}{3}\) π.

The volume of the truncated cone is
\(\frac{1024}{3}\) π – \(\frac{16}{3}\) π = (\(\frac{1024}{3}\) – \(\frac{16}{3}\))π
= \(\frac{1008}{3}\) π
= 336π.
The volume of the truncated cone is 336π cm³.

Question 2.
Find the volume of the truncated cone.

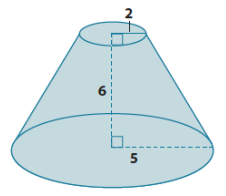
Answer:
Let x represent the height of the small cone.
\(\frac{2}{5}\) = \(\frac{x}{x + 6}\)
2(x + 6) = 5x
2x + 12 = 5x
12 = 3x
4 = x
The volume of the small cone is
V = \(\frac{1}{3}\) π(2)² (4)
= \(\frac{16}{3}\)π.

The volume of the large cone is
V = \(\frac{1}{3}\) π(5)² (10)
= \(\frac{250}{3}\) π.

The volume of the truncated cone is
\(\frac{250}{3}\) π – \(\frac{16}{3}\) π = (\(\frac{250}{3}\) – \(\frac{16}{3}\))π
= \(\frac{234}{3}\) π
= 78π.
The volume of the truncated cone is 8π units³.

Question 3.
Find the volume of the truncated pyramid with a square base.

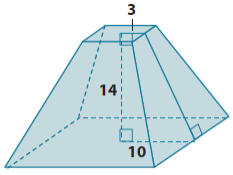
Answer:
Let x represent the height of the small pyramid.
\(\frac{3}{10}\) = \(\frac{x}{x + 14}\)
3(x + 14) = 10x
3x + 42 = 10x
42 = 7x
6 = x
The volume of the small pyramid is
V = \(\frac{1}{3}\) (36)(6)
= \(\frac{216}{3}\).

The volume of the large pyramid is
V = \(\frac{1}{3}\) (400)(20)
= \(\frac{8000}{3}\).

The volume of the truncated pyramid is
\(\frac{8000}{3}\) – \(\frac{216}{3}\) = \(\frac{7784}{3}\).

The volume of the truncated pyramid is \(\frac{7784}{3}\) units³.

Question 4.
Find the volume of the truncated pyramid with a square base. Note: 3 mm is the distance from the center to the edge of the square at the top of the figure.

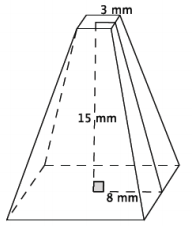
Answer:
Let x mm represent the height of the small pyramid.
\(\frac{3}{8}\) = \(\frac{x}{x + 15}\)
3(x + 15) = 8x
3x + 45 = 8x
45 = 5x
9 = x
The volume of the small pyramid is
V = \(\frac{1}{3}\) (36)(9)
= 108.

The volume of the large pyramid is
V = \(\frac{1}{3}\) (256)(24)
= 2048.

The volume of the truncated pyramid is
2048 – 108 = 1940.
The volume of the truncated pyramid is 1,940 mm³.

Question 5.
Find the volume of the truncated pyramid with a square base. Note: 0.5 cm is the distance from the center to the edge of the square at the top of the figure.

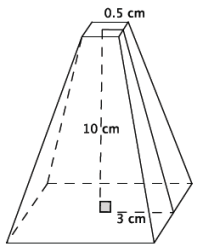
Answer:
Let x cm represent the height of the small pyramid.
\(\frac{0.5}{3}\) = \(\frac{x}{x + 10}\)
\(\frac{1}{2}\) (x + 10) = 3x
\(\frac{1}{2}\) x + 5 = 3x
5 = \(\frac{5}{2}\) x
2 = x
The volume of the small pyramid is
V = \(\frac{1}{3}\) (1)(2)
= \(\frac{2}{3}\).

The volume of the large pyramid is
V = \(\frac{1}{3}\) (36)(12)
= \(\frac{432}{3}\).

The volume of the truncated pyramid is
\(\frac{432}{3}\) – \(\frac{2}{3}\) = \(\frac{430}{3}\).

The volume of the truncated pyramid is \(\frac{430}{3}\) cm³.

Question 6.
Explain how to find the volume of a truncated cone.
Answer:
The first thing you have to do is use the ratios of corresponding sides of similar triangles to determine the height of the cone that was removed to make the truncated cone. Once you know the height of that cone, you can determine its volume. Then, you can find the height of the cone (the truncated cone and the portion that was removed). Once you know both volumes, you can subtract the smaller volume from the larger volume. The difference is the volume of the truncated cone.

Question 7.
Challenge: Find the volume of the truncated cone.

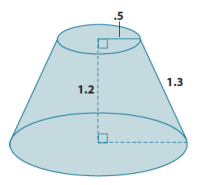
Answer:
Since the height of the truncated cone is 1.2 units, we can drop a perpendicular line from the top of the cone to the bottom of the cone so that we have a right triangle with a leg length of 1.2 units and a hypotenuse of 1.3 units. Then, by the Pythagorean theorem, if b is the length of the leg of the right triangle, then
1.2² + b² = 1.3²
1.44 + b² = 1.69
b² = 0.25
b = 0.5.
The part of the radius of the bottom base found by the Pythagorean theorem is 0.5. When we add the length of the upper radius (because if you translate along the height of the truncated cone, then it is equal to the remaining part of the lower base), then the radius of the lower base is 1.
Let x represent the height of the small cone.
\(\frac{0.5}{1}\) = \(\frac{x}{x + 1.2}\)
\(\frac{1}{2}\) (x + 1.2) = x
\(\frac{1}{2}\) x + 0.6 = x
0.6 = \(\frac{1}{2}\) x
1.2 = x
The volume of the small cone is
V = \(\frac{1}{3}\) π(0.5)² (1.2)
= \(\frac{0.3}{3}\) π
= 0.1π.

The volume of the large cone is
V = \(\frac{1}{3}\) π(1)² (2.4)
= \(\frac{2.4}{3}\) π
= 0.8π.

The volume of the truncated cone is
\(\frac{2.4}{3}\) π – \(\frac{0.3}{3}\) π = (\(\frac{2.4}{3}\) – \(\frac{0.3}{3}\))π
= \(\frac{2.1}{3}\) π
= 0.7π.
The volume of the truncated cone is 7π units³.

Eureka Math Grade 8 Module 7 Lesson 20 Exit Ticket Answer Key

Question 1.
Find the volume of the truncated cone.

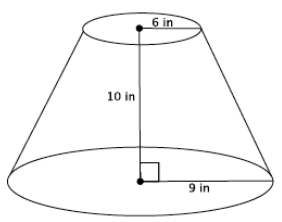
a. Write a proportion that will allow you to determine the height of the cone that has been removed. Explain what all parts of the proportion represent.
Answer:
\(\frac{6}{9}\) = \(\frac{x}{x + 10}\)
Let x in. represent the height of the small cone. Then
x + 10 is the height of the large cone. Then 6 is the base radius of the small cone, and 9 is the base radius of the large cone.

b. Solve your proportion to determine the height of the cone that has been removed.
Answer:
6(x + 10) = 9x
6x + 60 = 9x
60 = 3x
20 = x

c. Write an expression that can be used to determine the volume of the truncated cone. Explain what each part of the expression represents.
Answer:
\(\frac{1}{3}\) π(9)² (30) – \(\frac{1}{3}\) π(6)² (20)
The expression \(\frac{1}{3}\) π(9)² (30) represents the volume of the large cone, and \(\frac{1}{3}\) π(6)² (20) is the volume of the small cone. The difference in volumes represents the volume of the truncated cone.

d. Calculate the volume of the truncated cone.
Answer:
The volume of the small cone is
V = \(\frac{1}{3}\) π(6)² (20)
= \(\frac{720}{3}\) π.

The volume of the large cone is
V = \(\frac{1}{3}\) π(9)² (30)
= \(\frac{2430}{3}\) π.

The volume of the truncated cone is
\(\frac{2430}{3}\) π – \(\frac{720}{3}\) π = (\(\frac{2430}{3}\) – \(\frac{720}{3}\))π
= \(\frac{1710}{3}\) π
= 570π.
The volume of the truncated cone is 570π in³.