Eureka Math Grade 7 Module 6 Lesson 22 Answer Key

Engage NY Eureka Math 7th Grade Module 6 Lesson 22 Answer Key

Eureka Math Grade 7 Module 6 Lesson 22 Example Answer Key

Example 1.
a. The circle to the right has a diameter of 12 cm. Calculate the area of the shaded region.

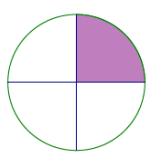
b. Sasha, Barry, and Kyra wrote three different expressions for the area of the shaded region. Describe what each student was thinking about the problem based on his or her expression.
Sasha’s expression: \(\frac{1}{4}\) π(6²)
Barry’s expression: π(6² ) – \(\frac{3}{4}\) π(6²)
Kyra’s expression: \(\frac{1}{2}\)(\(\frac{1}{2}\) π(6²))
Answer:
a.
→ What information do we need to calculate the area?
We need the radius of the circle and the shaded fraction of the circle.

→ What is the radius of the circle? How do you know?
The radius of the circle is 6 cm since the length of the radius is half the length of the diameter.

→ Now that we know the radius, how can we find the area of the shaded region?
Find the area of one quarter of the circle because the circle is divided into four identical parts, and only one part is shaded.

→ Choose a method discussed, and calculate the area of the shaded region.
A_{quarter circle} = \(\frac{1}{4}\)πr²
The area in squared centimeters:
\(\frac{1}{4}\)π(6)² = 9π ≈ 28.27
The area of the shaded region is about 28.27 cm².

b. Sasha’s expression: \(\frac{1}{4}\) π(6²)
Sasha’s expression gets directly to the shaded area as it represents a quarter of the area of the whole circle.

Barry’s expression: π(6² ) – \(\frac{3}{4}\) π(6²)
Barry’s expression shows the area of the whole circle minus the unshaded area of the circle or three – quarters of the area of the circle.

Kyra’s expression: \(\frac{1}{2}\)(\(\frac{1}{2}\) π(6²))
Kyra’s expression arrives at the shaded area by taking half the area of the whole circle, which is half of the circle, and taking half of that area, which leaves a quarter of the area of the whole circle.

Example 2.
The square in this figure has a side length of 14 inches. The radius of the quarter circle is 7 inches.
a. Estimate the shaded area.

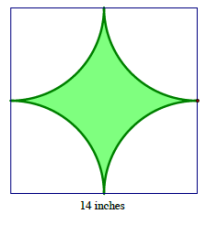
b. What is the exact area of the shaded region?
c. What is the approximate area using π ≈ \(\frac{22}{7}\)?
Answer:
→ Describe a strategy to find the area of the shaded region.
Find the area of the entire square, and subtract the area of the unshaded region because the unshaded region is four quarter circles of equal radius or a whole circle.

→ What is the difference between parts (b) and (c) in Example 2?
Part (b) asks for the exact area, which means the answer must be left in terms of pi; part (c) asks for the approximate area, which means the answer must be rounded off.

→ How would you estimate the shaded area?
Responses will vary. One possible response might be that the shaded area looks to be approximately one – quarter the area of the entire square, roughly \(\frac{1}{4}\)(14 in.)² = 49 in².

→ What is the area of the square?
A = 14 in. ∙ 14 in. = 196 in²
The area of the square is 196 in².

→ What is the area of each quarter circle?
A = \(\frac{\pi}{4}\) r² = \(\frac{\pi}{4}\)(7 in.)² = \(\frac{49 \pi}{4}\) in²
The area of each quarter circle is \(\frac{49 \pi}{4}\) in².
The area of the entire unshaded region, or all four quarter circles, is 49π in².

→ What is the exact area of the shaded region?
A = 196 in² – 49π in²
The area of the shaded region is (196 – 49π) in².

→ What is the approximate area using π ≈ \(\frac{22}{7}\)?
A ≈ 196 in² – (\(\frac{22}{7}\)) (7 in.)²
≈ 196 in² – 154 in²
≈ 42 in²
The area of the shaded region is approximately 42 in².

Eureka Math Grade 7 Module 6 Lesson 22 Exercise Answer Key

Exercise 1.
a. Find the area of the shaded region of the circle to the right.

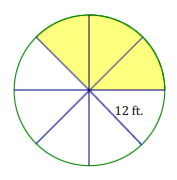
b. Explain how the expression you used represents the area of the shaded region.
Answer:
a. A = \(\frac{3}{8}\)πr²
A = \(\frac{3}{8}\)(π) (12 ft)²
A = 54π ft²
A≈169.65 ft²
The shaded area of the circle is approximately 169.65 ft².

b. The expression \(\frac{3}{8}\)(π) (12)² takes the area of a whole circle with a radius of 12 ft., which is just the portion that reads (π) (12)², and then multiplies that by \(\frac{3}{8}\). The shaded region is just three out of eight equal pieces of the circle.

Exercise 2.
Calculate the area of the figure below that consists of a rectangle and two quarter circles, each with the same radius. Leave your answer in terms of pi.

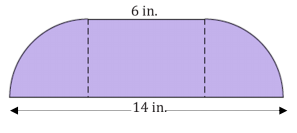
Answer:
A_rectangle = l ∙ w
A = 6 in. ∙ 4 in.
A = 24 in²
The area of the rectangle is 24 in².

A_{half circle} = \(\frac{1}{2}\)πr²
A = \(\frac{1}{2}\)(π)(4 in.)²
A = 8π in²

The area of the two quarter circles, or one semicircle, is 8π in².
The area of the entire figure is A = (24 + 8π) in².

Exercise 3.
The vertices A and B of rectangle ABCD are centers of circles each with a radius of 5 inches.
a. Find the exact area of the shaded region.

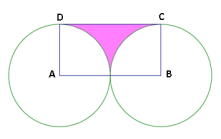
b. Find the approximate area using π ≈ \(\frac{22}{7}\).
c. Find the area to the nearest hundredth using the π key on your calculator.
Answer:
a. A_rectangle = 10 in. ∙ 5 in. = 50 in²
A_semicircle = \(\frac{1}{2}\) π(5 in.)²
A_semicircle = 25\(\frac{\pi}{2}\) in²
A_{shaded area} = (50 – 25\(\frac{\pi}{2}\)) in²

b. A_{shaded area} = (50 – 25\(\frac{\pi}{2}\) in²
A_{shaded area} ≈ (50 – \(\frac{25}{2}\)(\(\frac{22}{7}\))) in²
A_{shaded area} ≈ (50 – \(\frac{275}{7}\)) in²
A_{shaded area} ≈ 10 \(\frac{5}{7}\)in²
The area of the shaded region in the figure is approximately 10\(\frac{5}{7}\) in².

A_{shaded area} = (50 – \(\frac{25 \pi}{2}\)) in²
A_{shaded area} ≈ 10.73 in²
The area of the shaded region in the figure is approximately 10.73 in².

Exercise 4.
The diameter of the circle is 12 in. Write and explain a numerical expression that represents the area of the shaded region.

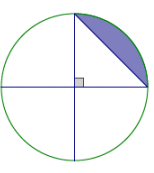
Answer:
A_shaded = A_{quarter circle} – A_triangle
A_shaded = \(\frac{\pi}{4}\)(6in.)² – \(\frac{1}{2}\)(6 in. ∙ 6 in.)
A_shaded = (9π – 18) in²
The expression represents the area of one quarter of the entire circle less the area of the right triangle, whose legs are formed by radii of the circle.

Eureka Math Grade 7 Module 6 Lesson 22 Problem Set Answer Key

Question 1.
A circle with center O has an area of 96 in². Find the area of the shaded region.

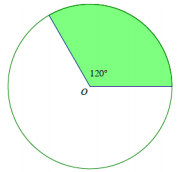
Peyton’s Solution
A = \(\frac{1}{3}\)(96 in² ) = 32 in²

Monte’s Solution
A = \(\frac{96}{120}\) in² = 0.8 in²
Answer:
Peyton solved the problem correctly because he correctly identified the shaded region as one – third of the area of the entire circle. The shaded region represents \(\frac{1}{3}\) of the circle because 120° is one third of 360°. To find the area of the shaded region, one – third of the area of the entire circle, 96 in², must be calculated, which is what Peyton did to get his solution.

Question 2.
The following region is bounded by the arcs of two quarter circles, each with a radius of 4 cm, and by line segments 6 cm in length. The region on the right shows a rectangle with dimensions 4 cm by 6 cm. Show that both shaded regions have equal areas.

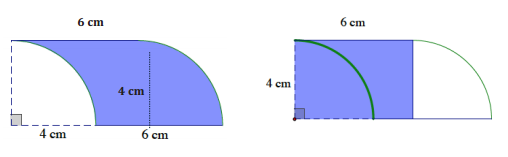
Answer:
A = ((4 cm ∙ 6 cm) – \(\frac{1}{4}\)π(4 cm)² ) + \(\frac{1}{4}\)π(4 cm)²
A = 24 cm²

A = 4 cm ∙ 6 cm
A = 24 cm²

Question 3.
A square is inscribed in a paper disc (i.e., a circular piece of paper) with a radius of 8 cm. The paper disc is red on the front and white on the back. Two edges of the circle are folded over. Write and explain a numerical expression that represents the area of the figure. Then, find the area of the figure.

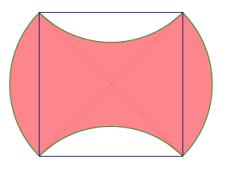
Answer:

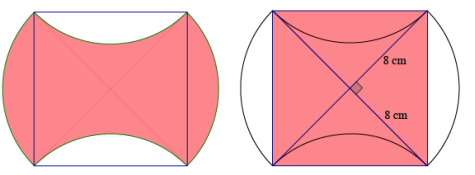
Numeric expression for the area: 4(\(\frac{1}{2}\) ∙ 8 cm ∙ 8 cm)
The shaded (red) area is the same as the area of the square. The radius is 8 cm, which is the length of one leg of each of the four equal – sized right triangles within the square. Thus, we find the area of one triangle and multiply by 4.
The area of the shaded region is 128 cm².

Question 4.
The diameters of four half circles are sides of a square with a side length of 7 cm.

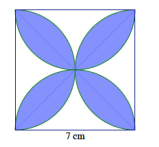
a. Find the exact area of the shaded region.
b. Find the approximate area using π ≈ \(\frac{22}{7}\).
c. Find the area using the π button on your calculator and rounding to the nearest thousandth.
Answer:

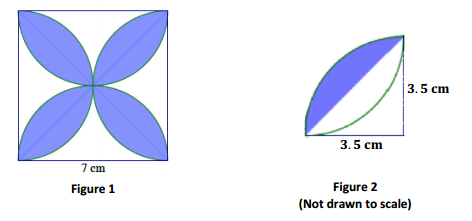
a. Figure 2 isolates one quarter of Figure 1. The shaded area in Figure 2 can be found as follows:
Shaded area = Area of the quarter circle – Area of the isosceles right triangle.
Shaded area:
(\(\frac{ \pi}{4}\) (\(\frac{7}{2}\) cm)² ) – (\(\frac{1}{2}\) ∙ \(\frac{7}{2}\) cm ∙ \(\frac{7}{2}\) cm) = \(\frac{49 \pi}{16}\) cm² – \(\frac{49}{8}\) cm²
= \(\frac{49}{16}\) (π – 2) cm²
The area of the shaded region is \(\frac{49}{16}\)(π – 2) cm². There are 8 such regions in the figure, so we multiply this answer by 8.
Total shaded area:
8(\(\frac{49}{16}\) (π – 2)) = \(\frac{49}{2}\) (π – 2) = \(\frac{49 \pi}{2}\) – 49
The exact area of the shaded region is (\(\frac{49 \pi}{2}\) – 49) cm².

b. A_{total shaded} ≈ \(\frac{49}{2}\) (\(\frac{22}{7}\) – 2) cm²
A_{total shaded} ≈ (77 – 49) cm²
A_{total shaded} ≈ 28 cm²
The approximate area of the shaded region is 28 cm².

c. A_{total shaded} = \(\frac{49}{2}\) (π – 2) cm²
A_{total shaded} ≈ 27.969 cm²
The approximate area of the shaded region is 27.969 cm².

Question 5.

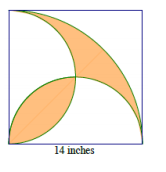
A square with a side length of 14 inches is shown below, along with a quarter circle (with a side of the square as its radius) and two half circles (with diameters that are sides of the square). Write and explain a numerical expression that represents the area of the figure.
Answer:

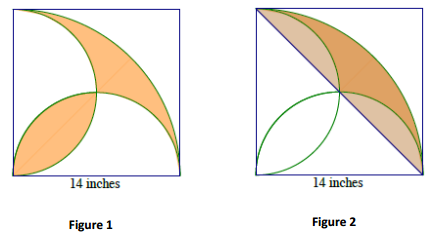
Numeric expression for the area: 1\(\frac{1}{4}\)π(14 in.)² – (\(\frac{1}{2}\) ∙ 14 in. ∙ 14 in.)
The shaded area in Figure 1 is the same as the shaded area in Figure 2. This area can be found by subtracting the area of the right triangle with leg lengths of 14 in. from the area of the quarter circle with a radius of 14 in.
\(\frac{1}{4}\) π(14 in.)² – (\(\frac{1}{2}\) ∙ 14 in. ∙ 14 in.) = (49π – 98) in²

Question 6.
Three circles have centers on segment AB. The diameters of the circles are in the ratio 3:2:1. If the area of the largest circle is 36 ft², find the area inside the largest circle but outside the smaller two circles.

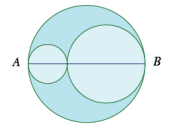
Answer:
Since all three circles are scale drawings of each other, the ratio of the areas of the circles is 9:4:1. This ratio provides a means to find the areas of the two smaller circles.
Area of medium – sized circle in ft²:
\(\frac{9}{4}\) = \(\frac{36}{x}\)
x = 16
The area of the medium – sized circle is 16 ft².

Area of small – sized circle in ft²:
\(\frac{9}{1}\) = \(\frac{36}{y}\)
y = 4
The area of the small – sized circle is 4 ft².

The area inside the largest circle but outside the smaller two circles is
A = 36 ft² – 16 ft² – 4 ft²
A = 16 ft².
The area inside the largest circle but outside the smaller two circles is 16 ft².

Question 7.
A square with a side length of 4 ft. is shown, along with a diagonal, a quarter circle (with a side of the square as its radius), and a half circle (with a side of the square as its diameter). Find the exact, combined area of regions I and II.

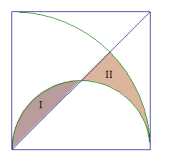
Answer:
The area of I is the same as the area of III in the following diagram.

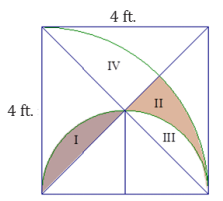
Since the area of I is the same as the area of III, we need to find the combined area of II and III. The combined area of II and III is half the area of II, III, and IV. The area of II, III, and IV is the area of the quarter circle minus the area of the triangle.
A_{II and III} = \(\frac{1}{2}\)(\(\frac{1}{4}\)π(4 ft.)² – \(\frac{1}{2}\) ∙ 4 ft ∙ 4 ft.)
A_{II and III} = \(\frac{1}{2}\)(\(\frac{16 \pi}{4}\) ft² – (\(\)\frac{4 \mathrm{ft} \cdot 4 \mathrm{ft}}{2}/\(\))
A_{II and III} = (2π – 4) ft²
The combined area of I and II is (2π – 4) ft².

Eureka Math Grade 7 Module 6 Lesson 22 Exit Ticket Answer Key

Question 1.
A circle with a 10 cm radius is cut into a half circle and two quarter circles. The three circular arcs bound the region below.
a. Write and explain a numerical expression that represents the area.
b. Then, find the area of the figure.

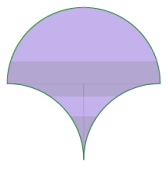
Answer:
a. Numeric expression 1 for the area:
10 cm ∙ 20 cm
The expression for the area represents the region when it is cut into three pieces and rearranged to make a complete rectangle as shown.

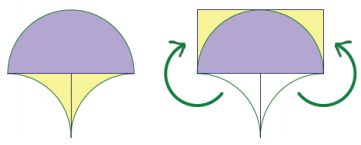
Numeric expression 2 for the area: (50π) cm² + (200 – 50π) cm²
The expression for the area is calculated as is; in other words, by finding the area of the semicircle in the top portion of the figure and then the area of the carved – out regions in the bottom portion of the figure.

b. The area of the figure is 200 cm².