Engage NY Eureka Math 7th Grade Module 5 Lesson 10 Answer Key
Eureka Math Grade 7 Module 5 Lesson 10 Example Answer Key
Example 1: Families
How likely is it that a family with three children has all boys or all girls?
Let’s assume that a child is equally likely to be a boy or a girl. Instead of observing the result of actual births, a toss of a fair coin could be used to simulate a birth. If the toss results in heads (H), then we could say a boy was born; if the toss results in tails (T), then we could say a girl was born. If the coin is fair (i.e., heads and tails are equally likely), then getting a boy or a girl is equally likely.
Answer:
Pose the following questions to the class one at a time, and allow for multiple responses:
→ How could a number cube be used to simulate getting a boy or a girl birth?
An even-number outcome represents boy, and an odd-number outcome represents girl; a prime-number outcome represents boy, and a non-prime outcome represents girl; or any three-number cube digits represents boy, while the rest represents girl.
→ How could a deck of cards be used to simulate getting a boy or a girl birth?
The most natural option is to allow black cards to represent one gender and red cards to represent the other.
Example 2.
Simulation provides an estimate for the probability that a family of three children would have three boys or three girls by performing three tosses of a fair coin many times. Each sequence of three tosses is called a trial. If a trial results in either HHH or TTT, then the trial represents all boys or all girls, which is the event that we are interested in. These trials would be called a success. If a trial results in any other order of H’s and T’s, then it is called a failure.
The estimate for the probability that a family has either three boys or three girls based on the simulation is the number of successes divided by the number of trials. Suppose 100 trials are performed, and that in those 100 trials, 28 resulted in either HHH or TTT. Then, the estimated probability that a family of three children has either three boys or three girls would be \(\frac{28}{100}\), or 0.28.
Answer:
→ What is the estimated probability that the three children are not all the same gender?
1 – 0.28 = 0.72
Example 3: Basketball Player
Suppose that, on average, a basketball player makes about three out of every four foul shots. In other words, she has a 75% chance of making each foul shot she takes. Since a coin toss produces equally likely outcomes, it could not be used in a simulation for this problem.
Instead, a number cube could be used by specifying that the numbers 1, 2, or 3 represent a hit, the number 4 represents a miss, and the numbers 5 and 6 would be ignored. Based on the following 50 trials of rolling a fair number cube, find an estimate of the probability that she makes five or six of the six foul shots she takes.
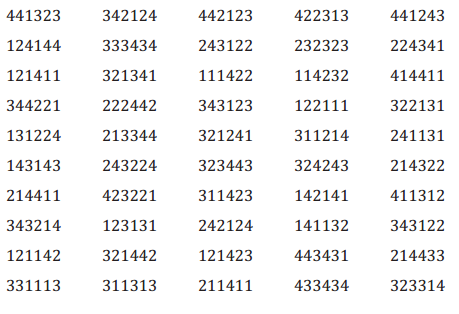
Answer:
50 trials of six numbers each are shown. Students are to estimate the probability that the player makes five or six of the six shots she takes. So, they should count how many of the trials have five or six of the numbers 1, 2, 3 in them as successes. They should find the estimated probability to be \(\frac{27}{50}\), or 0.54.
The estimate of the probability that she will make five or six foul shots is \(\frac{27}{50}\).
Eureka Math Grade 7 Module 5 Lesson 10 Exercise Answer Key
Exercises 1–2
Suppose that a family has three children. To simulate the genders of the three children, the coin or number cube or a card would need to be used three times, once for each child. For example, three tosses of the coin resulted in HHT, representing a family with two boys and one girl. Note that HTH and THH also represent two boys and one girl.
Exercise 1.
Suppose that when a prime number (P) is rolled on the number cube, it simulates a boy birth, and a non-prime (N) simulates a girl birth. Using such a number cube, list the outcomes that would simulate a boy birth and those that simulate a girl birth. Are the boy and girl birth outcomes equally likely?
Answer:
The outcomes are 2, 3, 5 for a boy birth and 1, 4, 6 for a girl birth. The boy and girl births are thereby equally likely.
Exercise 2.
Suppose that one card is drawn from a regular deck of cards. A red card (R) simulates a boy birth, and a black card (B) simulates a girl birth. Describe how a family of three children could be simulated.
Answer:
The key response has to include the drawing of three cards with replacement. If a card is not replaced and the deck shuffled before the next card is drawn, then the probabilities of the genders have changed (ever so slightly, but they are not 50/50 from draw to draw). Simulating the genders of three children requires three cards to be drawn
with replacement.
Exercises 3–5
Exercise 3.
Find an estimate of the probability that a family with three children will have exactly one girl using the following outcomes of 50 trials of tossing a fair coin three times per trial. Use H to represent a boy birth and T to represent a girl birth.

Answer:
T represents a girl. I went through the list, counted the number of times that HHT, HTH, or THH appeared and divided that number of successes by 50. The simulated probability is \(\frac{16}{50}\), or 0.32
Exercise 4.
Perform a simulation of 50 trials by rolling a fair number cube in order to find an estimate of the probability that a family with three children will have exactly one girl.
a. Specify what outcomes of one roll of a fair number cube will represent a boy and what outcomes will represent a girl.
b. Simulate 50 trials, keeping in mind that one trial requires three rolls of the number cube. List the results of your 50 trials.
c. Calculate the estimated probability.
Answer:
Answers will vary. For example, students could identify a girl birth as 1, 2, 3 outcome on one roll of the number cube and roll the number cube three times to simulate three children (one trial). They need to list their 50 trials. Note that an outcome of 412 would represent two girls, 123 would represent three girls, and 366 would represent one girl, as would 636 and 663. Be sure that they are clear about how to do all five steps of the simulation process.
Exercise 5.
Calculate the theoretical probability that a family with three children will have exactly one girl.
a. List the possible outcomes for a family with three children. For example, one possible outcome is BBB (all three children are boys).
b. Assume that having a boy and having a girl are equally likely. Calculate the theoretical probability that a family with three children will have exactly one girl.
c. Compare it to the estimated probabilities found in parts (a) and (b).
Answer:
a. The sample space is BBB, BBG, BGB, GBB, BGG, GBG, GGB, GGG.
b. Each is equally likely, so the theoretical probability of getting exactly one girl is \(\frac{3}{8}\), or 0.375 (BBG, BGB, GBB).
c. Answers will vary. The estimated probabilities from the first two parts of this exercise should be around 0.375. If not, suggest that students conduct more trials.
Eureka Math Grade 7 Module 5 Lesson 10 Problem Set Answer Key
Question 1.
A mouse is placed at the start of the maze shown below. If it reaches station B, it is given a reward. At each point where the mouse has to decide which direction to go, assume that it is equally likely to go in either direction. At each decision point 1, 2, 3, it must decide whether to go left (L) or right (R). It cannot go backward.
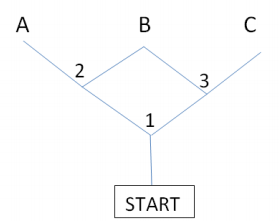
a. Create a theoretical model of probabilities for the mouse to arrive at terminal points A, B, and C.
i) List the possible paths of a sample space for the paths the mouse can take. For example, if the mouse goes left at decision point 1 and then right at decision point 2, then the path would be denoted LR.
ii) Are the paths in your sample space equally likely? Explain.
iiii) What are the theoretical probabilities that a mouse reaches terminal points A, B, and C? Explain.
b. Based on the following set of simulated paths, estimate the probabilities that the mouse arrives at points A, B, and C.

c. How do the simulated probabilities in part (b) compare to the theoretical probabilities of part (a)?
Answer:
a. i) The possible paths in the sample space are {LL, LR, RL, RR}.
ii) Each of these outcomes has an equal probability of 1/4 since at each decision point there are only two possible choices, which are equally likely.
iii) The probability of reaching terminal point A is \(\frac{1}{4}\) since it is accomplished by path LL. Similarly, reaching terminal point C is \(\frac{1}{4}\) since it is found by path RR. However, reaching terminal point B is \(\frac{1}{2}\) since it is reached via LR or RL.
b. Students need to go through the list and count the number of paths that go to A, B, and C. They should find the estimated probabilities to be \(\frac{8}{40}\), or 0.2, for A, \(\frac{22}{40}\), or 0.55, for B, and \(\frac{10}{40}\), or 0.25, for C.
c. The probabilities are reasonably close for parts (a) and (b). Probabilities based on taking 400 trials should be closer than those based on 40, but the probabilities based on 40 are in the ballpark.
Question 2.
Suppose that a dartboard is made up of the 8 × 8 grid of squares shown below. Also, suppose that when a dart is thrown, it is equally likely to land on any one of the 64 squares. A point is won if the dart lands on one of the 16 black squares. Zero points are earned if the dart lands in a white square.

a. For one throw of a dart, what is the probability of winning a point? Note that a point is won if the dart lands on a black square.
b. Lin wants to use a number cube to simulate the result of one dart. She suggests that 1 on the number cube could represent a win. Getting 2, 3, or 4 could represent no point scored. She says that she would ignore getting a 5 or 6. Is Lin’s suggestion for a simulation appropriate? Explain why you would use it, or if not, how you would change it.
c. Suppose a game consists of throwing a dart three times. A trial consists of three rolls of the number cube. Based on Lin’s suggestion in part (b) and the following simulated rolls, estimate the probability of scoring two points in three darts.

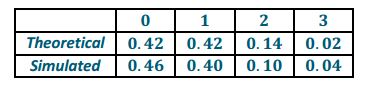
d. The theoretical probability model for winning 0, 1, 2, and 3 points in three throws of the dart as described in this problem is:
i) Winning 0 points has a probability of 0.42.
ii) Winning 1 point has a probability of 0.42.
iii) Winning 2 points has a probability of 0.14.
iv) Winning 3 points has a probability of 0.02.
Use the simulated rolls in part (c) to build a model of winning 0, 1, 2, and 3 points, and compare it to the theoretical model.
Answer:
a. The probability of winning a point is \(\frac{16}{64}\), or 0.25.
b. Lin correctly suggests that to simulate the result of one throw, a number cube could be used with the 1 representing a hit; 2, 3, 4 representing a missed throw; and 5 and 6 being ignored. (As an aside, a tetrahedron could be used by using the side facing down as the result.)
c. The probability of scoring two points in three darts is \(\frac{5}{50}\), or 0.1. (Students need to count the number of trials that contain exactly two 1’s.)
d. To find the estimated probability of 0 points, count the number of trials that have no 1’s in them
(\(\frac{23}{50}\) = 0.46).
To find the estimated probability of 1 point, count the number of trials that have one 1 in them (\(\frac{20}{50}\) = 0.4).
From part (c), the estimated probability of 2 points is 0.1.
To find the estimated probability of 3 points, count the number of trials that have three 1’s in them
(\(\frac{2}{50}\) = 0.04).
The theoretical and simulated probabilities are reasonably close.
Eureka Math Grade 7 Module 5 Lesson 10 Exit Ticket Answer Key
Question 1.
Nathan is your school’s star soccer player. When he takes a shot on goal, he typically scores half of the time. Suppose that he takes six shots in a game. To estimate the probability of the number of goals Nathan makes, use simulation with a number cube. One roll of a number cube represents one shot.
a. Specify what outcome of a number cube you want to represent a goal scored by Nathan in one shot.
b. For this problem, what represents a trial of taking six shots?
c. Perform and list the results of ten trials of this simulation.
d. Identify the number of goals Nathan made in each of the ten trials you did in part (c).
e. Based on your ten trials, what is your estimate of the probability that Nathan scores three goals if he takes six shots in a game?
Answer:
a. Answers will vary; students need to determine which three numbers on the number cube represent scoring a goal.
b. Rolling the cube six times represents taking six shots on goal or 1 simulated trial.
c. Answers will vary; students working in pairs works well for these problems. Performing only ten trials is a function of time. Ideally, many more trials should be done. If there is time, have the class pool their results.
d. Answers will vary.
e. Answers will vary; the probability of scoring per shot is \(\frac{1}{2}\).
Question 2.
Suppose that Pat scores 40% of the shots he takes in a soccer game. If he takes six shots in a game, what would one simulated trial look like using a number cube in your simulation?
Answer:
Students need to realize that 40% is 2 out of 5. In order to use the number cube as the device, 1 and 2 could represent goals, while 3, 4, and 5 could represent missed shots, and 6 is ignored. Rolling the number cube six times creates 1 simulated trial.