Engage NY Eureka Math 7th Grade Module 3 Lesson 6 Answer Key
Eureka Math Grade 7 Module 3 Lesson 6 Example Answer Key
Example 1.
Rewrite the expression in standard form by collecting like terms.
\(\frac{2}{3}\) n-\(\frac{3}{4}\) n+\(\frac{1}{6}\) n+2 \(\frac{2}{9}\) n
Answer:
\(\frac{2}{3}\) n-\(\frac{3}{4}\) n+\(\frac{1}{6}\) n+2 \(\frac{2}{9}\) n
\(\frac{24}{36}\) n-\(\frac{27}{36}\) n+\(\frac{6}{36}\) n+2 \(\frac{8}{36}\) n
2 \(\frac{11}{36}\) n
→ What are various strategies for adding, subtracting, multiplying, and dividing rational numbers?
→ Find common denominators; change from mixed numbers and whole numbers to improper fractions, and then convert back.
Example 2.
At a store, a shirt was marked down in price by $10.00. A pair of pants doubled in price. Following these changes, the price of every item in the store was cut in half. Write two different expressions that represent the new cost of the items, using s for the cost of each shirt and p for the cost of a pair of pants. Explain the different information each one shows.
Answer:
For the cost of a shirt:
\(\frac{1}{2}\) (s-10) The cost of each shirt is \(\frac{1}{2}\) of the quantity of the original cost of the shirt, minus 10.
\(\frac{1}{2}\) s-5 The cost of each shirt is half off the original price, minus 5, since half of 10 is 5.
For the cost of a pair of pants:
\(\frac{1}{2}\) (2p) The cost of each pair of pants is half off double the price.
p The cost of each pair of pants is the original cost because \(\frac{1}{2}\) is the multiplicative inverse of 2.
→ Describe a situation in which either of the two expressions in each case would be more useful.
→ Answers may vary. For example, p would be more useful than \(\frac{1}{2}\)(2p) because it is converted back to an isolated variable, in this case the original cost.
Example 3.
Write this expression in standard form by collecting like terms. Justify each step.
5 \(\frac{1}{3}\)-(3 \(\frac{1}{3}\))(\(\frac{1}{2}\) x-\(\frac{1}{4}\))
Answer:
\(\frac{16}{3}\)+(-\(\frac{10}{3}\))(\(\frac{1}{2}\) x)+(-\(\frac{10}{3}\))(-\(\frac{1}{4}\)) Write mixed numbers as improper fractions, then distribute.
\(\frac{16}{3}\)+(-\(\frac{5}{3}\) x)+\(\frac{5}{6}\) Any grouping (associative) and arithmetic rules for multiplying rational numbers
–\(\frac{5}{3}\) x+(\(\frac{36}{6}\)+\(\frac{5}{6}\)) Commutative property and associative property of addition, collect like terms
–\(\frac{5}{3}\) x+\(\frac{37}{6}\) Apply arithmetic rule for adding rational numbers
→ A student says he created an equivalent expression by first finding this difference: 5 \(\frac{1}{3}\)-3 \(\frac{1}{3}\) . Is he correct? Why or why not?
→ Although they do appear to be like terms, taking the difference would be incorrect. In the expression (3 \(\frac{1}{3}\))(\(\frac{1}{2}\) x-\(\frac{1}{4}\)), 3 \(\frac{1}{3}\) must be distributed before applying any other operation in this problem.
→ How should 3 \(\frac{1}{3}\) be written before being distributed?
→ The mixed number can be rewritten as an improper fraction \(\frac{10}{3}\). It is not necessary to convert the mixed number, but it makes the process more efficient and increases the likelihood of getting a correct answer.
Example 4.
Model how to write the expression in standard form using rules of rational numbers.
\(\frac{x}{20}\)+\(\frac{2x}{5}\)+\(\frac{(x+1)}{2}\)+\(\frac{(3x-1)}{10}\)
→ What are other equivalent expressions of \(\frac{x}{20}\)? How do you know?
→ Other expressions include 1\(\frac{x}{20}\) and \(\frac{1}{2}\)0 x because of the arithmetic rules of rational numbers.
→ What about \(\frac{1}{20x}\)? How do you know?
→ It is not equivalent because if x=2, the value of the expression is \(\frac{1}{40}\), which does not equal \(\frac{1}{10}\).
→ How can the distributive property be used in this problem?
→ For example, it can be used to factor out \(\frac{1}{20}\) from each term of the expression.
→ Or, for example, it can be used to distribute \(\frac{1}{10}\): \(\frac{3x-1}{10}\)=\(\frac{1}{10}\) (3x-1) = \(\frac{3x}{10}\)–\(\frac{1}{10}\).
Below are two solutions. Explore both with the class.
\(\frac{x}{20}\)+\(\frac{4(2x)}{20}\)+\(\frac{10(x+1)}{20}\)+\(\frac{2(3x-1)}{20}\)
\(\frac{x+8 x+10 x+10+6 x-2}{20}\)
\(\frac{25 x+8}{20}\)
\(\frac{5}{4}\)x + \(\frac{2}{5}\)
\(\frac{1}{20}\)x+\(\frac{2}{5}\) x+\(\frac{1}{2}\) x+\(\frac{1}{2}\)+\(\frac{3}{10}\) x-\(\frac{1}{10}\)
(\(\frac{1}{20}\)+\(\frac{2}{5}\)+\(\frac{1}{2}\)+\(\frac{3}{10}\))x+(\(\frac{1}{2}\)–\(\frac{1}{10}\))
(\(\frac{1}{20}\)+\(\frac{8}{20}\)+\(\frac{10}{20}\)+\(\frac{6}{20}\))x+(\(\frac{5}{10}\)–\(\frac{1}{10}\))
\(\frac{5}{4}\) x+\(\frac{2}{5}\)
Ask students to evaluate the original expression and the answers when x=20 to see if they get the same number.
Evaluate the original expression and the answers when x=20. Do you get the same number?
\(\frac{x}{20}\)+\(\frac{2x}{5}\)+\(\frac{x+1}{2}\)+\(\frac{3x-1}{10}\)
\(\frac{20}{20}\)+\(\frac{2(20)}{5}\)+\(\frac{20+1}{2}\)+\(\frac{3(20)-1}{10}\)
1+8+\(\frac{21}{2}\)+\(\frac{59}{10}\)
9+\(\frac{105}{10}\)+\(\frac{59}{10}\)
9+\(\frac{164}{10}\)
9+\(\frac{164}{10}\)
25 \(\frac{2}{5}\)
\(\frac{5}{4}\) x+\(\frac{2}{5}\)
\(\frac{5}{4}\) (20)+\(\frac{2}{5}\)
25+\(\frac{2}{5}\)
25 \(\frac{2}{5}\)
Example 5.
Rewrite the following expression in standard form.
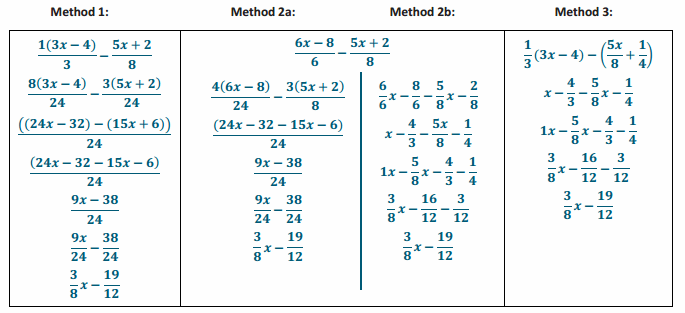
\(\frac{2(3 x-4)}{6}-\frac{5 x+2}{8}\)
Answer:
→ How can we start to rewrite this problem?
→ There are various ways to start rewriting this expression, including using the distributive property, renaming \(\frac{2}{6}\), rewriting the subtraction as an addition, distributing the negative in the second term, rewriting each term as a fraction (e.g., \(\frac{2}{6}\)(3x-4)-(\(\frac{5x}{8}\)+\(\frac{2}{8}\))), or finding the lowest common denominator.
Eureka Math Grade 7 Module 3 Lesson 6 Opening Exercise Answer Key
Solve each problem, leaving your answers in standard form. Show your steps.
a. Terry weighs 40 kg. Janice weighs 2 \(\frac{3}{4}\) kg less than Terry. What is their combined weight?
Answer:
40+(40-2 \(\frac{3}{4}\))=80-2\(\frac{3}{4}\)=78-\(\frac{3}{4}\)=77 \(\frac{1}{4}\). Their combined weight is 77 \(\frac{1}{4}\) kg.
b. 2\(\frac{2}{3}\)-1 \(\frac{1}{2}\)–\(\frac{4}{5}\)
Answer:
\(\frac{8}{3}\)–\(\frac{3}{2}\)–\(\frac{4}{5}\)
\(\frac{80}{30}\)–\(\frac{45}{30}\)–\(\frac{24}{30}\)
\(\frac{11}{30}\)
c. \(\frac{1}{5}\)+(-4)
Answer:
-3 \(\frac{4}{5}\)
d. 4(\(\frac{3}{5}\))
Answer:
\(\frac{4}{1}\) (\(\frac{3}{5}\))
\(\frac{12}{5}\)
2 \(\frac{2}{5}\)
e. Mr. Jackson bought 1 \(\frac{3}{5}\) lb. of beef. He cooked \(\frac{3}{4}\) of it for lunch. How much does he have left?
Answer:
If he cooked \(\frac{3}{4}\) of it for lunch, he had \(\frac{1}{4}\) of the original amount left. Since (1 \(\frac{3}{5}\))(\(\frac{1}{4}\))=\(\frac{8}{5}\)⋅\(\frac{1}{4}\)=\(\frac{2}{5}\), he had \(\frac{2}{5}\) lb. left. Teachers: You can also show your students how to write the answer as one expression.
(1\(\frac{3}{5}\))(1-\(\frac{3}{4}\))
→ How is the process of writing equivalent expressions by combining like terms in this Opening Exercise different from the previous lesson?
→ There are additional steps to find common denominators, convert mixed numbers to improper numbers (in some cases), and convert back.
Eureka Math Grade 7 Module 3 Lesson 6 Exercise Answer Key
Exercise 1.
For the following exercises, predict how many terms the resulting expression will have after collecting like terms. Then, write the expression in standard form by collecting like terms.
a. \(\frac{2}{5}\) g-\(\frac{1}{6}\)-g+\(\frac{3}{10}\) g-\(\frac{4}{5}\)
Answer:
There will be two terms.
\(\frac{2}{5}\) g-1g+\(\frac{3}{10}\) g-\(\frac{1}{6}\)–\(\frac{4}{5}\)
(\(\frac{2}{5}\)-1+\(\frac{3}{10}\))g-(\(\frac{1}{6}\)+\(\frac{4}{5}\))
–\(\frac{3}{10}\) g-\(\frac{29}{30}\)
b. i+6i-\(\frac{3}{7}\) i+\(\frac{1}{3}\) h+\(\frac{1}{2}\) i-h+\(\frac{1}{4}\) h
Answer:
i+6i-\(\frac{3}{7}\) i+\(\frac{1}{3}\) h+\(\frac{1}{2}\) i-h+\(\frac{1}{4}\) h
There will be two terms.
\(\frac{1}{3}\) h+\(\frac{1}{4}\) h-h+i-\(\frac{3}{7}\) i+6i+\(\frac{1}{2}\) i
(\(\frac{1}{3}\)+\(\frac{1}{4}\)+(-1))h+(1-\(\frac{3}{7}\)+6+\(\frac{1}{2}\))i
–\(\frac{5}{12}\) h+7 \(\frac{1}{14}\) i
Exercise 2.
Write two different expressions that represent the total cost of the items if tax was \(\frac{1}{10}\) of the original price. Explain the different information each shows.
For the cost of a shirt:
\(\frac{1}{2}\) (s-10)+\(\frac{1}{10}\) s The cost of each shirt is \(\frac{1}{2}\) of the quantity of the original cost of the shirt, minus 10, plus \(\frac{1}{10}\) of the cost of the shirt.
\(\frac{3}{5}\) s-5 The cost of each shirt is \(\frac{3}{5}\) of the original price (because it is \(\frac{1}{2}\) s+\(\frac{1}{10}\) s=\(\frac{6}{10}\) s), minus 5, since half of 10 is 5.
For the cost of a pair of pants:
\(\frac{1}{2}\) (2p)+\(\frac{1}{10}\) p The cost of each pair of pants is half off double the price plus \(\frac{1}{10}\) of the cost of a pair of pants.
1 \(\frac{1}{10}\) p The cost of each pair of pants is 1 \(\frac{1}{10}\) (because 1p+\(\frac{1}{10}\) p=1 \(\frac{1}{10}\) p) times the number of pair of pants.
Exercise 3.
Rewrite the following expressions in standard form by finding the product and collecting like terms.
a. -6 \(\frac{1}{3}\)–\(\frac{1}{2}\) (\(\frac{1}{2}\)+y)
Answer:
-6 \(\frac{1}{3}\)+(-\(\frac{1}{2}\))(\(\frac{1}{2}\))+(-\(\frac{1}{2}\))y
-6 \(\frac{1}{3}\)+(-\(\frac{1}{4}\))+(-\(\frac{1}{2}\) y)
–\(\frac{1}{2}\) y-(6 \(\frac{1}{3}\)+\(\frac{1}{4}\))
–\(\frac{1}{2}\) y-(6 \(\frac{4}{12}\)+\(\frac{3}{12}\))
–\(\frac{1}{2}\) y-6 \(\frac{7}{12}\)
b. \(\frac{2}{3}\)+\(\frac{1}{3}\) (\(\frac{1}{4}\) f-1 \(\frac{1}{3}\))
Answer:
\(\frac{2}{3}\)+\(\frac{1}{3}\) (\(\frac{1}{4}\) f)+\(\frac{1}{3}\) (-\(\frac{4}{3}\))
\(\frac{2}{3}\)+\(\frac{1}{12}\) f-\(\frac{4}{9}\)
\(\frac{1}{12}\) f+(\(\frac{6}{9}\)–\(\frac{4}{9}\))
\(\frac{1}{12}\) f+\(\frac{2}{9}\)
Exercise 4.
Rewrite the following expression in standard form by finding common denominators and collecting like terms.
\(\frac{2h}{3}\)–\(\frac{h}{9}\)+\(\frac{h-4}{6}\)
Answer:
\(\frac{6(2h)}{18}\)–\(\frac{2(h)}{18}\)+\(\frac{3(h-4)}{18}\)
\(\frac{12 h-2 h+3 h-12}{18}\)
\(\frac{13 h-12}{18}\)
\(\frac{13}{18}\)h – \(\frac{2}{3}\)
Exercise 5.
Write the following expression in standard form.
\(\frac{2 x-11}{4}-\frac{3(x-2)}{10}\)
Answer:
\(\frac{5(2 x-11)}{20}-\frac{2 \cdot 3(x-2)}{20}\)
\(\frac{(10 x-55)-6(x-2)}{20}\)
\(\frac{10 x-55-6 x+12}{20}\)
\(\frac{4 x-43}{20}\)
\(\frac{1}{5} x-2 \frac{3}{20}\)
Eureka Math Grade 7 Module 3 Lesson 6 Exit Ticket Answer Key
For the problem \(\frac{1}{5}\) g-\(\frac{1}{10}\)-g+1 \(\frac{3}{10}\) g-\(\frac{1}{10}\) , Tyson created an equivalent expression using the following steps.
Answer:
\(\frac{1}{5}\) g+-1g+1 \(\frac{3}{10}\) g+-\(\frac{1}{10}\)+-\(\frac{1}{10}\)
–\(\frac{4}{5}\) g+1 \(\frac{1}{10}\)
Is his final expression equivalent to the initial expression? Show how you know. If the two expressions are not equivalent, find Tyson’s mistake and correct it.
For the problem \(\frac{1}{5}\) g-\(\frac{1}{10}\)-g+1 \(\frac{3}{10}\) g-\(\frac{1}{10}\) , Tyson created an equivalent expression using the following steps.
\(\frac{1}{5}\) g+-1g+1 \(\frac{3}{10}\) g+-\(\frac{1}{10}\)+-\(\frac{1}{10}\)
–\(\frac{4}{5}\) g+1 \(\frac{1}{10}\)
Is his final expression equivalent to the initial expression? Show how you know. If the two expressions are not equivalent, find Tyson’s mistake and correct it.
Answer:
No, he added the first two terms correctly, but he forgot the third term and added to the other like terms.
If g=10,
\(\frac{1}{5}\) g+-1g+1 \(\frac{3}{10}\) g+-\(\frac{1}{10}\)+-\(\frac{1}{10}\)
\(\frac{1}{5}\)(10)+-1(10)+1 \(\frac{3}{10}\) (10)+-\(\frac{1}{10}\)+-\(\frac{1}{10}\)
2+(-10)+13+(-\(\frac{2}{10}\))
4\(\frac{4}{5}\)
–\(\frac{4}{5}\)g +1 \(\frac{1}{10}\)
–\(\frac{4}{5}\)(10)+1 \(\frac{1}{10}\)
-8+1 \(\frac{1}{10}\)
-6\(\frac{9}{10}\)
The expressions are not equal.
He should factor out the g and place parentheses around the values using the distributive property in order to make it obvious which rational numbers need to be combined.
\(\frac{1}{5}\) g+-1g+1 \(\frac{3}{10}\) g+-\(\frac{1}{10}\)+-\(\frac{1}{10}\)
(\(\frac{1}{5}\) g+-1g+1 \(\frac{3}{10}\) g)+(-\(\frac{1}{10}\)+-\(\frac{1}{10}\))
(\(\frac{1}{5}\)+-1+1 \(\frac{3}{10}\))g+(-\(\frac{2}{10}\))
(\(\frac{2}{10}\)+\(\frac{3}{10}\))g+(-\(\frac{1}{5}\))
\(\frac{1}{2}\) g-\(\frac{1}{5}\)
Eureka Math Grade 7 Module 3 Lesson 6 Problem Set Answer Key
Question 1.
Write the indicated expressions.
a. \(\frac{1}{2}\) m inches in feet
Answer:
\(\frac{1}{2}\) m×\(\frac{1}{12}\)=\(\frac{1}{24}\)m. It is \(\frac{1}{24}\)m ft.
b. The perimeter of a square with \(\frac{2}{3}\) g cm sides
Answer:
4×\(\frac{2}{3}\) g=\(\frac{8}{3}\) g. The perimeter is \(\frac{8}{3}\) g cm.
c. The number of pounds in 9 oz.
Answer:
9×\(\frac{1}{16}\)=\(\frac{9}{16}\). It is \(\frac{9}{16}\) lb.
d. The average speed of a train that travels x miles in \(\frac{3}{4}\) hour
Answer:
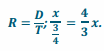 . The average speed of the train is \(\frac{4}{3}\) x miles per hour.
. The average speed of the train is \(\frac{4}{3}\) x miles per hour.
e. Devin is 1 \(\frac{1}{4}\) years younger than Eli. April is \(\frac{1}{5}\) as old as Devin. Jill is 5 years older than April. If Eli is E years old, what is Jill’s age in terms of E?
Answer:
D=E-1 \(\frac{1}{4}\), A=\(\frac{D}{5}\), A+5=J, so J=(\(\frac{D}{5}\))+5. J=\(\frac{1}{5}\)(E-1 \(\frac{1}{4}\))+5. J=\(\frac{E}{5}\)+4 \(\frac{3}{4}\).
Question 2.
Rewrite the expressions by collecting like terms.
a. \(\frac{1}{2}\) k-\(\frac{3}{8}\) k
Answer:
\(\frac{4}{8}\) k-\(\frac{3}{8}\) k
\(\frac{1}{8}\) k
b. \(\frac{2r}{5}\)+\(\frac{7r}{15}\)
Answer:
\(\frac{6r}{15}\)+\(\frac{7r}{15}\)
\(\frac{13r}{15}\)
c. –\(\frac{1}{3}\) a-\(\frac{1}{2}\) b-\(\frac{3}{4}\)+\(\frac{1}{2}\) b-\(\frac{2}{3}\) b+\(\frac{5}{6}\) a
Answer:
–\(\frac{1}{3}\) a+\(\frac{5}{6}\) a-\(\frac{1}{2}\) b+\(\frac{1}{2}\) b-\(\frac{2}{3}\) b-\(\frac{3}{4}\)
–\(\frac{2}{6}\) a+\(\frac{5}{6}\) a-\(\frac{2}{3}\) b-\(\frac{3}{4}\)
\(\frac{1}{2}\) a-\(\frac{2}{3}\) b-\(\frac{3}{4}\)
d. -p+\(\frac{3}{5}\) q-\(\frac{1}{10}\) q+\(\frac{1}{9}\)–\(\frac{1}{9}\) p+2 \(\frac{1}{3}\) p
Answer:
-p-\(\frac{1}{9}\) p+2 \(\frac{1}{3}\) p+\(\frac{3}{5}\) q-\(\frac{1}{10}\) q+\(\frac{1}{9}\)
–\(\frac{9}{9}\) p-\(\frac{1}{9}\) p+2 \(\frac{3}{9}\) p+\(\frac{6}{10}\) q-\(\frac{1}{10}\) q+\(\frac{1}{9}\)
1\(\frac{1}{9}\) p+\(\frac{5}{10}\)q+\(\frac{1}{9}\)
1 \(\frac{2}{9}\) p+\(\frac{1}{2}\) q+\(\frac{1}{9}\)
e. \(\frac{5}{7}\) y-\(\frac{y}{14}\)
Answer:
\(\frac{10}{14}\) y-\(\frac{1}{14}\) y
\(\frac{9}{14}\)y
f. \(\frac{3n}{8}\)–\(\frac{n}{4}\)+2 \(\frac{n}{2}\)
Answer:
\(\frac{3n}{8}\)–\(\frac{2n}{8}\)+2\(\frac{4n}{8}\)
2\(\frac{5n}{8}\)
Question 3.
Rewrite the expressions by using the distributive property and collecting like terms.
a. \(\frac{4}{5}\) (15x-5)
Answer:
12x-4
b. \(\frac{4}{5}\) (\(\frac{1}{4}\) c-5)
Answer:
\(\frac{1}{5}\)c-4
c. 2 \(\frac{4}{5}\) v-\(\frac{2}{3}\) (4v+1 \(\frac{1}{6}\))
Answer:
\(\frac{2}{5}\) v-\(\frac{7}{9}\)
d. 8-4(\(\frac{1}{8}\) r-3 \(\frac{1}{2}\))
Answer:
–\(\frac{1}{2}\) r+22
e. \(\frac{1}{7}\) (14x+7)-5
Answer:
2x-4
f. \(\frac{1}{5}\) (5x-15)-2x
Answer:
-x-3
g. \(\frac{1}{4}\) (p+4)+\(\frac{3}{5}\) (p-1)
Answer:
\(\frac{17}{20}\) p+\(\frac{2}{5}\)
h. \(\frac{7}{8}\) (w+1)+\(\frac{5}{6}\)(w-3)
Answer:
\(\frac{41}{24}\)w-\(\frac{39}{24}\) or \(\frac{41}{24}\) w-\(\frac{13}{8}\)
i. \(\frac{4}{5}\) (c-1)-\(\frac{1}{8}\)(2c+1)
Answer:
\(\frac{11}{20}\)c – \(\frac{37}{40}\)
j. \(\frac{2}{3}\) (h+\(\frac{3}{4}\))-\(\frac{1}{3}\) (h+\(\frac{3}{4}\))
Answer:
\(\frac{1}{3}\) h+\(\frac{1}{4}\)
k. \(\frac{2}{3}\) (h+\(\frac{3}{4}\))-\(\frac{2}{3}\) (h-\(\frac{3}{4}\))
Answer:
1
l. \(\frac{2}{3}\) (h+\(\frac{3}{4}\))+\(\frac{2}{3}\) (h-\(\frac{3}{4}\))
Answer:
\(\frac{4}{3}\) h
m. \(\frac{k}{2}\)–\(\frac{4k}{5}\)-3
Answer:
–\(\frac{3k}{10}\)-3
n. \(\frac{3t+2}{7}\)+\(\frac{t-4}{14}\)
Answer:
\(\frac{1}{2}\) t
o. \(\frac{9x-4}{10}\)+\(\frac{3x+2}{5}\)
Answer:
\(\frac{3x}{2}\) or 1 \(\frac{1}{2}\)x
p. \(\frac{3(5 g-1)}{4}\) – \(\frac{2 g+7}{6}\)
Answer:
3\(\frac{5}{12}\)g – 1\(\frac{11}{12}\)
q. \(\frac{3 d+1}{5}\) + \(\frac{d-5}{2}\) + \(\frac{7}{10}\)
Answer:
\(\frac{-d}{10}\)-2
r. \(\frac{9 w}{6}+\frac{2 w-7}{3}-\frac{w-5}{4}\)
Answer:
\(\frac{23 w-13}{12}\)
\(\frac{23}{12}\)w-\(\frac{13}{12}\)
s. \(\frac{1+f}{5}-\frac{1+f}{3}+\frac{3-f}{6}\)
Answer:
\(\frac{11}{30}\)–\(\frac{3}{10}\)f