Engage NY Eureka Math 5th Grade Module 5 End of Module Assessment Answer Key
Eureka Math Grade 5 Module 5 End of Module Assessment Task Answer Key
Question 1.
Use your ruler to draw a rectangle that measures 4\(\frac{1}{2}\) by 2\(\frac{3}{4}\) inches, and find its area.
Answer:
Area =
9/2 x 11/4
= 99/8
= 12 3/8
Therefore, area = 12 3/8 square inches.
Question 2.
Heather has a rectangular yard. She measures it and finds out it is 24\(\frac{1}{2}\) feet long by 12\(\frac{1}{2}\) feet wide.
a. She wants to know how many square feet of sod she will need to completely cover the yard. Draw the yard, and label the measurements.
b. How much sod will Heather need to cover the yard?
c. If each square foot of sod costs 65 cents, how much will she have to pay to cover her yard?
Answer:
a.
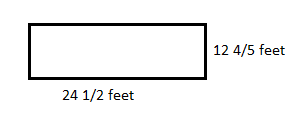
b.
The amount of Sod Heather need to cover the yard =
24 1/2 x 12 4/5
= 49/2 x 64/5
= 3136/10
= 1568/ 5
= 313 3/5
Therefore, Heather need 313 3/5 square feet of Sod to cover the yard.
c.
313 3/5 = 313.6
Now, Given that each square feet of sod cost 65 cents
So,
313.6 x 65
= 203.840
Therefore, Heather needs to pay $203.840 to cover her yard.
Question 3.
A rectangular container that has a length of 30 cm, a width of 20 cm, and a height of 24 cm is filled with water to a depth of 15 cm. When an additional 6.5 liters of water are poured into the container, some water overflows. How many liters of water overflow the container? Use words, pictures, and numbers to explain your answer. (Remember: 1 cm3 = 1 mL.)
Answer:
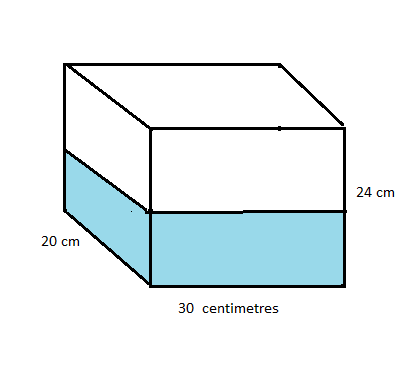
Volume of the rectangular container =
= 30 x 20 x 24
= 14,400 cubic centimetres
Volume of water =
30 x 20 x 15
= 9000
Therefore, water in the container = 9000 cubic centimetres
The leftover part in the container =
14,400 – 9000
= 5400 cubic centimetres or 5.4 litres
According to the given condition,
If 6.5 litres of water is poured into the container
Then, the amount of overflown water =
6.5 litres – 5.4 litres
= 1.1 litres
Therefore, the amount of water overflown = 1.1 litres.
Question 4.
Jim says that a 2\(\frac{1}{2}\) inch by 3\(\frac{1}{2}\) inch rectangle has a section that is 2 inches × 3 inches and a section that is \(\frac{1}{2}\)inch × \(\frac{1}{4}\)inch. That means the total area is just the sum of these two smaller areas, or 6\(\frac{1}{8}\) in2. Why is Jim incorrect? Use an area model to explain your thinking. Then, give the correct area of the rectangle.
Answer:
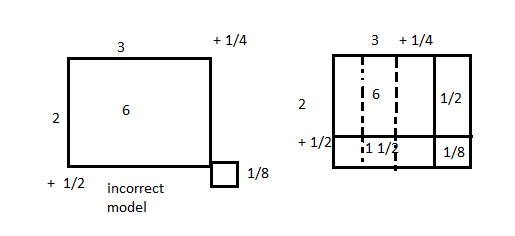
The area of the rectangle according to the correct model =
6 + 1 1/2 + 1/2 + 1/8
=8 1/8
Therefore, the area of the rectangle = 8 1/8 square inches.
Question 5.
Miguel and Jacqui built towers out of craft sticks. Miguel’s tower had a 4-inch square base. Jacqui’s tower had a 6-inch square base. If Miguel’s tower had a volume of 128 cubic inches and Jacqui’s had a volume of 288 cubic inches, whose tower was taller? Explain your reasoning.
Answer:
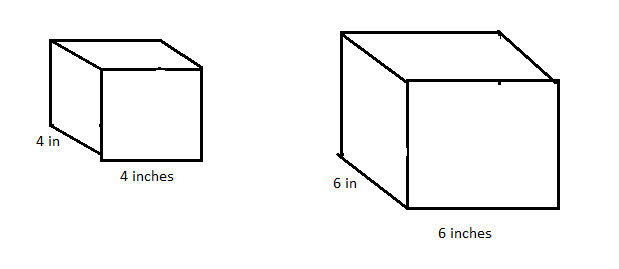
Given volume = 128 cubic centimetres
Now, Height of Miguel tower =
Base area = 4 x 4 = 16
height = 128/16
= 8 inches
Height of Jacqui tower =
Base area = 6 x 6 = 36
Height =
128/36
= 8 inches
Therefore, both towers have same height of 8 inches
To calculate height, I divided volume by base area.
Question 6.
Read the statements. Circle True or False. Explain your choice for each using words and/or pictures.

Answer:
a.
True ,
All parallelograms have 4 straight sides.
So, all parallelograms area a type of quadrilateral
b.
True,
All squares are rhombuses because squares have equal sides and same 4 right angles, but some rhombuses do not have 4 right angles so, not all rhombuses are squares.
c.
False,
Squares and rhombuses both have 4 equal sides and also squares and rectangles both have 4 right angles.
Therefore, Squares are both rhombuses and rectangles.
d.
True,
The four angles are added up to 360 degrees.
In parallelogram, the opposite sides are equal and parallel. Opposite angles are also equal.
e.
False,
All rectangles have 2 pairs of parallel sides. Therefore, All rectangles are parallelograms.
f.
False,
The sum of all angles in any parallelograms is always 360 degrees.
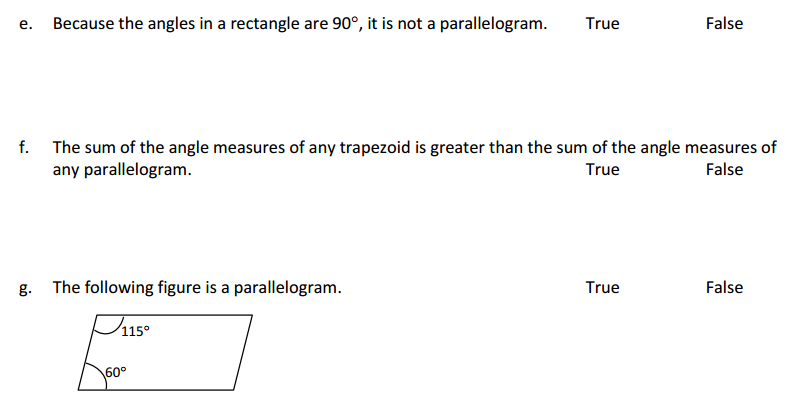
g.
False,
In a parallelogram, the sum of angles is equal to 360 degrees and also opposite sides are always equal.
So, the given figure is not a parallelogram.