Eureka Math Geometry Module 4 Lesson 6 Answer Key

Engage NY Eureka Math Geometry Module 4 Lesson 6 Answer Key

Eureka Math Geometry Module 4 Lesson 6 Example Answer Key

Example
Given points A(2, 2), B(10, 16), C( – 3, 1), and D(4, – 3), are \(\overline{A B}\) and \(\overline{C D}\) perpendicular? Are the lines containing the segments perpendicular? Explain.
Answer:
One possible solution would be to translate \(\overline{A B}\) so that A’ is on the origin (using the vector ( – 2, – 2), or left 2 units and down 2 units) and to translate \(\overline{C D}\) so that C’ is on the origin (using the vector (3, – 1), right 3 units and down 1 unit):

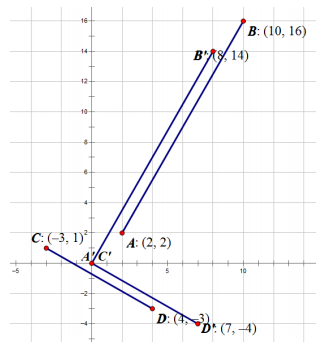
A(2, 2) → A'(2 – 2, 2 – 2) → A'(0, 0)
B(10, 16) → B'(10 – 2, 16 – 2) → B'(8, 14)
C( – 3, 1) → C'( – 3 – ( – 3), 1 – 1) → C'(0, 0)
D(4, – 3) → D'(4 – ( – 3), – 3 – 1) → D'(7, – 4)
\(\overline{A B}\) will be perpendicular to \(\overline{C D}\) if \(\overline{A^{\prime} B^{\prime}}\) is perpendicular to \(\overline{C^{\prime} D^{\prime}}{\prime}\).
8(7) + 14( – 4) = 0; therefore, \(\overline{A^{\prime} B^{\prime}}\) is perpendicular to \(\overline{C^{\prime} D^{\prime}}\), which means \(\overline{A B}\) ⊥ \(\overline{C D}\).

Eureka Math Geometry Module 4 Lesson 6 Exercise Answer Key

Opening Exercise
Carlos thinks that the segment having endpoints A(0, 0) and B(6, 0) is perpendicular to the segment with endpoints A(0, 0) and C( – 2, 0). Do you agree? Why or why not?
Answer:
No, the two segments are not perpendicular. If they were perpendicular, then 6( – 2) + 0(0) = 0 would be true.

Working with a partner, given A(0, 0) and B(3, – 2), find the coordinates of a point C so that \(\overline{A C}\) ⊥ \(\overline{A B}\).
Answer:
Let the other endpoint be C(c, d). If \(\overline{A C}\) ⊥ \(\overline{A B}\), then 3c + – 2d = 0. This means that \(\overline{A C}\) will be perpendicular to \(\overline{A B}\) as long as we choose values for c and d that satisfy the equation d = \(\frac{1}{2}\) c.
Answers may vary but may include (2, 3), (4, 6), and (6, 9) or any other coordinates that meet the requirement stated above.

Exercises
Exercise 1.
Given A(a₁, a₂), B(b₁, b₂), C(c₁, c₂), and D(d₁, d₂), find a general formula in terms of a₁, a₂, b₁, b₂, c₁, c₂, d₁, and d₂ that will let us determine whether \(\overline{A B}\) and \(\overline{C D}\) are perpendicular.
Answer:
After translating the segments so that the image of points A and C lie on the origin, we get A’ (0, 0), B’ (b₁ – a₁, b₂ – a₂ ), C’ (0, 0), and D’ (d₁ – c₁, d₂ – c₂ ).
\(\overline{A^{\prime} B^{\prime}}\) ⊥ \(\overline{A^{\prime} B^{\prime}}\)⟺ (b₁ – a₁ )(d₁ – c₁ ) + (b₂ – a₂ )(d₂ – c₂ ) = 0

Exercise 2.
Recall the Opening Exercise of Lesson 4 in which a robot is traveling along a linear path given by the equation
y = 3x – 600. The robot hears a ping from a homing beacon when it reaches the point F(400, 600) and turns to travel along a linear path given by the equation y – 600 = – \(\frac{1}{3}\)(x – 400). If the homing beacon lies on the x – axis, what is its exact location? (Use your own graph paper to visualize the scenario.)

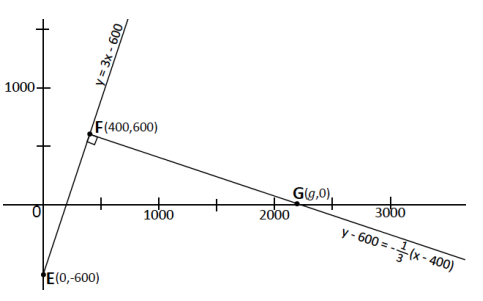
a. If point E is the y – intercept of the original equation, what are the coordinates of point E?
Answer:
E(0, – 600)

b. What are the endpoints of the original segment of motion?
Answer:
E(0, – 600) and F(400, 600)

c. If the beacon lies on the x – axis, what is the y – value of this point, G?
Answer:
0

d. Translate point F to the origin. What are the coordinates of E’, F’, and G’?
Answer:
E'( – 400, – 1200), F’ (0, 0), and G'(g – 400, – 600)

e. Use the formula derived in this lesson to determine the coordinates of point G.
Answer:
We know that \(\overline{E F}\) ⊥ \(\overline{F G}\), so – 400(g – 400) + ( – 1200)( – 600) = 0 ⟺ g = 2200.
Given G(g, 0) and we found g = 2000, then the coordinates of point G are (2200, 0).

Exercise 3.
A triangle in the coordinate plane has vertices A(0, 10), B( – 8, 8), and C( – 3, 5). Is it a right triangle? If so, at which vertex is the right angle? (Hint: Plot the points, and draw the triangle on a coordinate plane to help you determine which vertex is the best candidate for the right angle.)
Answer:
The most likely candidate appears to be vertex C. By translating the figure so that C is mapped to the origin, we can use the formula

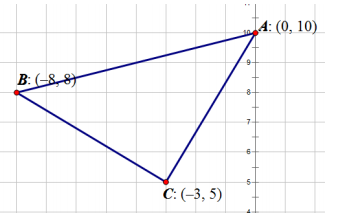
( – 8 + 3)(0 + 3) + (8 – 5)(10 – 5) = 0
– 5(3) + 3(5) = 0
0 = 0
Yes, \(\overline{B C}\) and \(\overline{A C}\) are perpendicular. The right angle is ∠C.

Exercise 4.
A( – 7, 1), B( – 1, 3), C(5, – 5), and D( – 5, – 5) are vertices of a quadrilateral. If \(\overline{A C}\) bisects \(\overline{B D}\), but \(\overline{B D}\)does not bisect \(\overline{A C}\), determine whether ABCD is a kite.
Answer:
We can show ABCD is a kite if \(\overline{A C}\) ⊥ \(\overline{B D}\)
Translating \(\overline{A C}\) so that the image of point A lies on the origin and translating \(\overline{B D}\) so that the image of point B lies at the origin lead to the equation:
(5 + 7)( – 5 + 1) + ( – 5 – 1)( – 5 – 3) = 0
(12)( – 4) + ( – 6)( – 8) = 0
– 48 + 48 = 0
This is a true statement; therefore, \(\overline{A C}\) ⊥ \(\overline{B D}\), and ABCD is a kite.

Eureka Math Geometry Module 4 Lesson 6 Problem Set Answer Key

Question 1.
Are the segments through the origin and the points listed perpendicular? Explain.
a. A(9, 10), B(10, 9)
Answer:
No 9∙10 + 10∙9 ≠ 0

b. C(9, 6), D(4, – 6)
Answer:
Yes 9∙4 + 6∙( – 6) = 0

Question 2.
Given M(5, 2), N(1, – 4), and L listed below, are \(\overline{L M}\) and \(\overline{M N}\) perpendicular? Translate M to the origin, write the coordinates of the images of the points, and then explain without using slope.
a. L( – 1, 6)
Answer:
M'(0, 0), N'( – 4, – 6), L'( – 6, 4)
Yes ( – 4)( – 6) + ( – 6)(4) = 0

b. L(11, – 2)
Answer:
M'(0, 0), N'( – 4, – 6), L'(6, – 4)
Yes ( – 4)(6) + ( – 6)( – 4) = 0

c. L(9, 8)
Answer:
M'(0, 0), N'( – 4, – 6), L'(4, 6)
No. ( – 4)(4) + ( – 6)(6) ≠ 0

Question 3.
Is triangle PQR, where P( – 7, 3), Q( – 4, 7), and R(1, – 3), a right triangle? If so, which angle is the right angle? Justify your answer.
Answer:
Yes. If the points are translated to P'(0, 0), Q'(3, 4), and R'(8, – 6), 3(8) + 4( – 6) = 0, meaning the segments are perpendicular. The right angle is ∠P.

Question 4.
A quadrilateral has vertices (2 + \(\sqrt{2}\), – 1), (8 + \(\sqrt{2}\), 3), (6 + \(\sqrt{2}\), 6), and (\(\sqrt{2}\), 2). Prove that the quadrilateral is a rectangle.
Answer:
Answers will vary, but it is a rectangle because it has 4 right angles.

Question 5.
Given points G( – 4, 1), H(3, 2), and I( – 2, – 3), find the x – coordinate of point J with y – coordinate 4 so that the \(\overleftrightarrow{G H}\) and \(\overleftrightarrow{I J}\) are perpendicular.
Answer:
– 3

Question 6.
A robot begins at position ( – 80, 45) and moves on a path to (100, – 60). It turns 90° counterclockwise.
a. What point with y – coordinate 120 is on this path?
Answer:
(205, 120)

b. Write an equation of the line after the turn.
Answer:
y + 60 = \(\frac{12}{7}\) (x – 100)

c. If it stops to charge on the x – axis, what is the location of the charger?
Answer:
(135, 0)

Question 7.
Determine the missing vertex of a right triangle with vertices (6, 2) and (5, 5) if the third vertex is on the y – axis. Verify your answer by graphing.
Answer:
(0, \(\frac{10}{3}\))

Question 8.
Determine the missing vertex for a rectangle with vertices (3, – 2), (5, 2), and ( – 1, 5), and verify by graphing. Then, answer the questions that follow.
Answer:
( – 3, 1)

a. What is the length of the diagonal?
Answer:
Approximately 8.06 units

b. What is a point on both diagonals in the interior of the figure?
Answer:
(1, \(\frac{3}{2}\))

Question 9.
Leg \(\overline{A B}\) of right triangle ABC has endpoints A(1, 3) and B(6, – 1). Point C(x, y) is located in Quadrant IV.
a. Use the perpendicularity criterion to determine at which vertex the right angle is located. Explain your reasoning.
Answer:
Assume that the right angle is at A(1, 3). Then a translation 1 unit left and 3 units down maps point A to the origin. A(1, 3) → A’ (1 – 1, 3 – 3) → A’ (0, 0)
B(6, – 1) → B'(6 – 1, – 1 – 3) → B'(5, – 4)
C(x, y) → C'(x – 1, y – 3)
By the criterion for perpendicularity,
5(x – 1) + – 4(y – 3) = 0
5x – 5 – 4y + 12 = 0
5x – 4y + 7 = 0
4y = 5x + 7
y = \(\frac{5}{4}\) x + \(\frac{7}{4}\)
The solutions to this equation form a line that had a positive slope and a positive y – intercept so no point on this line lies in Quadrant IV. Therefore, the right angle cannot be at A(1, 3) and so must be at B(6, – 1).

b. Determine the range of values that x is limited to and why?
Answer:
If the right angle is at B(6, – 1), then a translation 6 units left and 1 unit up maps point B to the origin.
B(6, – 1) → B'(6 – 6, – 1 – ( – 1)) → B'(0, 0)
A(1, 3) → A'(1 – 6, 3 – ( – 1)) → A'( – 5, 4)
C(x, y) → C'(x – 6, y – ( – 1)) → C'(x – 6, y + 1)
By the criterion for perpendicularity,
– 5(x – 6) + 4(y + 1) = 0
– 5x + 30 + 4y + 4 = 0
– 5x + 4y + 34 = 0
4y = 5x – 34
y = \(\frac{5}{4}\) x – \(\frac{17}{2}\)
The point must lie on the graph of y = \(\frac{5}{4}\) x – \(\frac{17}{2}\) and in Quadrant IV. The y – intercept of the graph is (0, – \(\frac{17}{2}\)), however since this point is not in the fourth quadrant, x > 0. Substituting 0 for y in the equation, it is determined that the x – intercept of the graph is 6.8. This point does not lie in Quadrant IV so x < 6.8. The remaining vertex cannot coincide with another vertex, so x ≠ 6. Therefore, the value of the x – coordinate of the remaining vertex is limited to 0 < x < 6.8 and x≠6.

c. Find the coordinates of point C if they are both integers.
Answer:
The value of x must be 1, 2, 3, 4, or 5. Substituting each into the equation y = \(\frac{5}{4}\) x – \(\frac{17}{2}\), the only value of x that yields an integer value for y is x = 2.
y = \(\frac{5}{4}\) (2) – \(\frac{17}{2}\)
y = \(\frac{5}{2}\) – \(\frac{17}{2}\)
y = – 6
The coordinates of vertex C are (2, – 6).

Eureka Math Geometry Module 4 Lesson 6 Exit Ticket Answer Key

Question 1.
Given points S(2, 4), T(7, 6), U( – 3, – 4), and V( – 1, – 9):

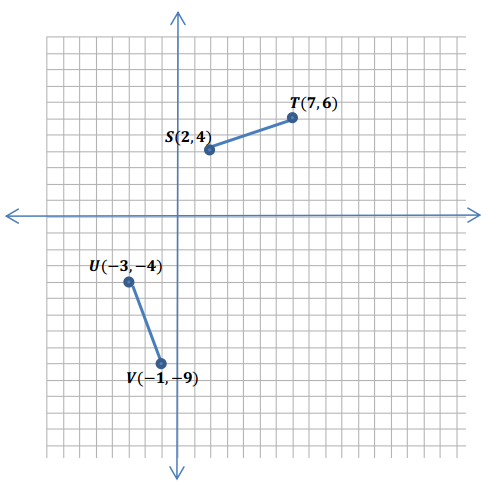
a. Translate \(\overline{S T}\) and \(\overline{U V}\) so that the image of each segment has an endpoint at the origin.
Answer:
Answers can vary slightly. Students have to choose one of the first two and one of the second two.
If we translate \(\overline{S T}\) so that the image of S is at the origin, we get S'(0, 0), T'(5, 2).
If we translate \(\overline{S T}\) so that the image of T is at the origin, we get S'( – 5, – 2), T'(0, 0).
If we translate \(\overline{U V}\) so that the image of U is at the origin, we get U'(0, 0), V'(2, – 5).
If we translate \(\overline{U V}\) so that the image of V is at the origin, we get V'(0, 0), U'( – 2, 5).

b. Are the segments perpendicular? Explain.
Answer:
Yes. By choosing any two of the translated \(\overline{S^{\prime} T^{\prime}}\) and \(\overline{U^{\prime} V^{\prime}}\), we determine whether the equation yields a true statement: (b₁ – a₁)(d₁ – c₁ ) + (b₂ – a₂)(d₂ – c₂) = 0.
For example, using S( – 5, – 2), T'(0, 0), and U'(0, 0), V'(2, – 5):
– 5(2) + ( – 2)( – 5) = 0 is a true statement; therefore, \(\overline{S^{\prime} T^{\prime}}\) ⊥ \(\overline{U^{\prime} V^{\prime}}\) and \(\overline{S T}\) ⊥ \(\overline{U V}\).

c. Are the lines \(\overleftrightarrow{S T}\) and \(\overleftrightarrow{U V}\) perpendicular? Explain.
Answer:
Yes, lines containing perpendicular segments are also perpendicular.