Engage NY Eureka Math Geometry Module 1 Lesson 8 Answer Key
Eureka Math Geometry Module 1 Lesson 8 Exercise Answer Key
Opening Exercise
Find the measure of angle x in the figure to the right. Explain your calculations. (Hint: Draw an auxiliary line segment.)
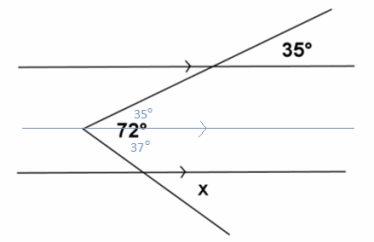
Answer:
m∠x=37°
The angle with the measure of 72° can be divided two angles. One measures 35° (corresponding angles). Then the other angle has a measure of 37° (partition property).
Discussion
The sum of the 3 angle measures of any triangle is ___.
Answer:
The sum of the 3 angle measures of any triangle is 180°.
INTERIOR OF A TRIANGLE: A point lies in the interior of a triangle if it lies in the interior of each of the angles of the triangle.
In any triangle, the measure of the exterior angle is equal to the sum of the measures of the ___ angles. These are sometimes also known as ___ angles.
Answer:
In any triangle, the measure of the exterior angle is equal to the sum of the measures of the opposite interior angles. These are sometimes also known as remote interior angles.
Base angles of an __ triangle are equal in measure.
Answer:
Base angles of an isosceles triangle are equal in measure.
Each angle of an __ triangle has a measure equal to 60°.
Answer:
Each angle of an equilateral triangle has a measure equal to 60°.
Exercises 1–11
Exercise 1.
Find the measures of angles a and b in the figure to the right. Justify your results.
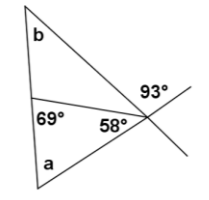
Answer:
m∠a=53°
m∠b=40°
In each figure, determine the measures of the unknown (labeled) angles. Give reasons for your calculations.
Exercise 2.
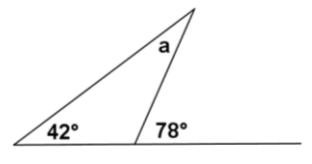
m∠a=
Answer:
m∠a=36°
The exterior angle of a triangle equals the sum of the two interior opposite angles.
Exercise 3.
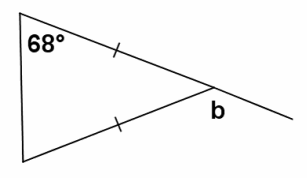
m∠b=
Answer:
m∠b=136°
The base angles of an isosceles triangle are equal in measure.
The sum of the angle measures in a triangle is 180°.
Linear pairs form supplementary angles.
Exercise 4.
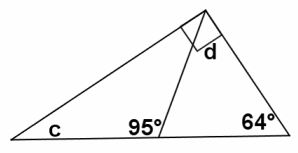
m∠c=
Answer:
m∠c=26°
The sum of the angle measures in a triangle is 180°.
m∠d=
Answer:
m∠d=31°
Linear pairs form supplementary angles.
The sum of the angle measures in a triangle is 180°.
Exercise 5.
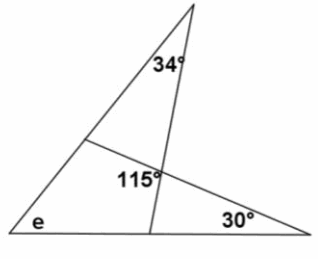
m∠e=
Answer:
m∠e=51°
Linear pairs form supplementary angles.
The sum of the angle measures in a triangle is 180°.
Exercise 6.
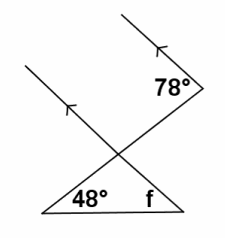
m∠f=
Answer:
m∠f=30°
If parallel lines are cut by a transversal, then corresponding angles are equal in measure.
Linear pairs form supplementary angles.
The sum of the angle measures in a triangle is 180°.
Exercise 7.
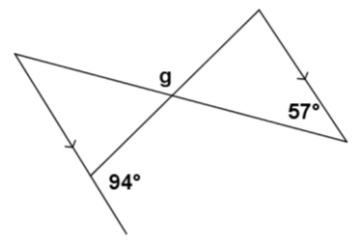
m∠g=
Answer:
m∠g= 143°
If parallel lines are cut by a transversal, then alternate interior angles are equal in measure.
Linear pairs form supplementary angles.
The sum of the angle measures in a triangle is 180°.
Exercise 8.
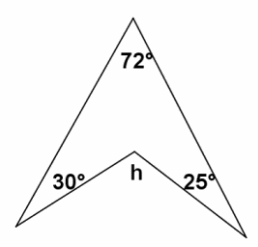
m∠h=
Answer:
m∠h=127°
Draw an auxiliary line, and then use the facts that linear pairs form supplementary angles and the sum of the angle measures in a triangle is 180°.
Exercise 9.
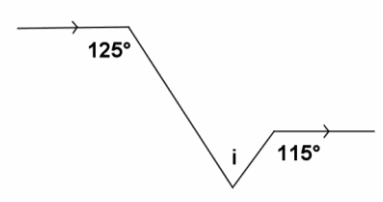
m∠i=
Answer:
m∠i=60°
If parallel lines are cut by a transversal, then alternate interior angles are equal in measure.
Linear pairs form supplementary angles (twice).
The sum of the angle measures in a triangle is 180°.
Exercise 10.
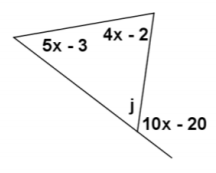
m∠j=
Answer:
m∠j=50°
If parallel lines are cut by a transversal, then alternate interior angles are equal in measure.
Linear pairs form supplementary angles.
Exercise 11.
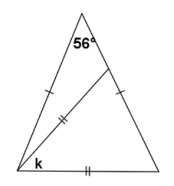
m∠k=
Answer:
m∠k=56°
Eureka Math Geometry Module 1 Lesson 8 Problem Set Answer Key
Find the unknown (labeled) angle in each figure. Justify your calculations.
Question 1.
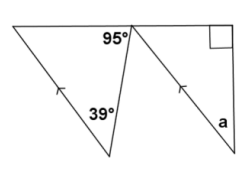
m∠a=
Answer:
m∠a=44°
If parallel lines are cut by a transversal, then alternate interior angles are equal in measure.
Linear pairs form supplementary angles.
The sum of the angle measures in a triangle is 180°.
Exercise 2.
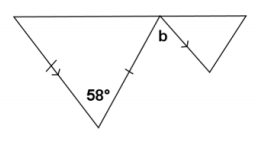
m∠b=
Answer:
m∠b=58°
If parallel lines are cut by a transversal, then alternate interior angles are equal in measure.
Exercise 3.
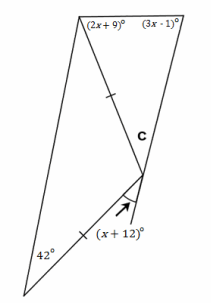
m∠c=
Answer:
m∠c=47°
The base angles of an isosceles triangle are equal in measure.
The sum of the angle measures in a triangle is 180°.
The exterior angle of a triangle equals the sum of the two interior opposite angles.
The sum of the angle measures in a triangle is 180°.
Eureka Math Geometry Module 1 Lesson 8 Exit Ticket Answer Key
Find the value of d and x.
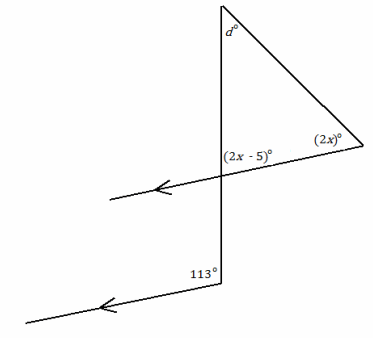
d =
x =
Answer:
d=41
x=36