Engage NY Eureka Math Algebra 2 Module 3 Lesson 26 Answer Key
Eureka Math Algebra 2 Module 3 Lesson 26 Exercise Answer Key
Exercise 1:
Answer the following questions.
The youth group from Example 1 is given the option of investing their money at 2.976% interest per year, compounded monthly instead of depositing it in the original account earning 3. 0% compounded yearly.
a. With an initial deposit of $800, how much would be in each account after two years?
Answer:
The account from the beginning of the lesson would contain $848.72, and the new account would contain
$800 \(\left(1+\frac{0.02976}{12}\right)^{12 \cdot 2}\) ≈ $849.00
b. Compare the total amount from part (a) to how much they would have made using the interest rate of 3% compounded yearly for two years. Which account would you recommend the youth group invest its money in? Why?
Answer:
The 3% compounded yearly yields $848. 72, while the 2.976% compounded monthly yields $849.00 after two years. The yield from the two accounts are very close to each other, but the account compounded monthly earns $0.28 more over the two-year period.
Eureka Math Algebra 2 Module 3 Lesson 26 Problem Set Answer Key
Question 1.
Write each recursive sequence in explicit form. Identify each sequence as arithmetic, geometric, or neither.
a. a1 = 3, an + 1 = an + 5
Answer:
an = 3 + 5(n – 1), arithmetic
b. a1 = -1, an + 1 = -2an
Answer:
an = -(-2)n – 1, geometric
c. a1 = 30, an + 1 = a – 3
Answer:
an = 30 – 3(n – 1), arithmetic
d. a1 = ± √2 an + 1 = \(\frac{a_{n}}{\sqrt{2}}\)
Answer:
an = √2\(\left(\frac{1}{\sqrt{2}}\right)^{n-1}\), geometric.
e. a1 = 1 , an + 1 = cos(πan)
Answer:
a1 = 1, an = -1 for n > 1, neither.
Question 2.
Write each sequence in recursive form. Assume the first term is when n = 1.
a. an = \(\frac{3}{2}\)n + 3
Answer:
a1 = \(\frac{9}{2}\), an + 1 = an + \(\frac{3}{2}\)
b. an = 3(\(\frac{3}{2}\))n
Answer:
a1 = \(\frac{9}{2}\), an + 1 = \(\frac{3}{2}\) . an
c. an = n2
Answer:
a1 = 1, an + 1 = an + 2n + 1
d. an = cos(2πn)
Answer:
a1 = 1, an + 1 = an
Question 3.
Consider two bank accounts. Bank A gives simple interest on an initial investment in savings accounts at a rate of 3% per year. Bank B gives compound interest on savings accounts at a rate of 2.5% per year. Fill out the following table.
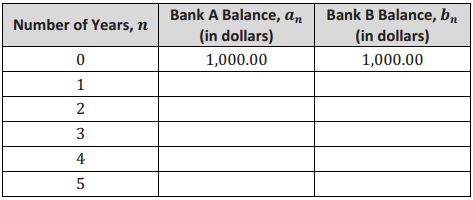
Answer:
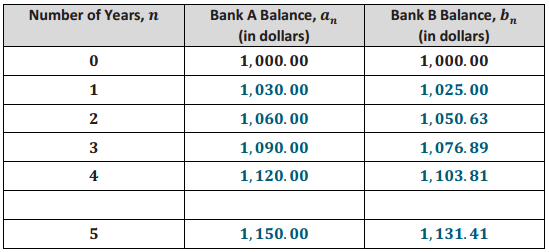
a. What type of sequence do the Bank A balances represent?
Answer:
The balances in Bank A represent an arithmetic sequence with constant difference of $30.
b. Give both a recursive and an explicit formula for the Bank A balances.
Answer:
Recursive: a0 = 1000, an = an – 1 + 30
Explicit: an = 1000 + 30n or fA(n) = 1000 + 30n.
c. What type of sequence do the Bank B balances represent?
Answer:
The balances in Bank B represent a geometric sequence with common ratio of 1.025.
d. Give both a recursive and an explicit formula for the Bank B balances.
Answer:
Recursive: b1 = 1000, bn = bn – 1 . 1.025
Explicit: bn = 1000 . 1.025n or f8(n)= 1000 . 1.025n
e. Which bank account balance is increasing faster in the first five years?
Answer:
During the first five years, the balance in Bank A is increasing faster at a constant rate of $30 per year.
f. If you were to recommend a bank account for a long-term investment, which would you recommend?
Answer:
The balance in Bank B would eventually outpace the balance in Bank A since the balance in Bank B is increasing geometrically.
g. At what point is the balance in Bank B larger than the balance in Bank A?
Answer:
Once the balance in Bank B overtakes the balance in Bank A, It will always be larger, so we just have to find when they are equal. This can only be done by graphing functions fA and fB and estimating the intersection point.
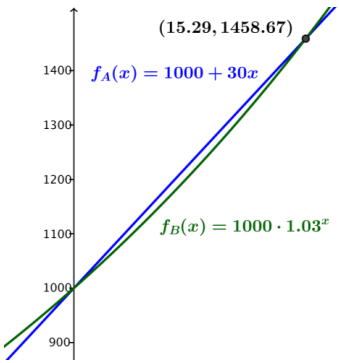
It appears that the balance in Bank B overtakes the balance in Bank A in the l6th year and be larger from then on. Any investment made for 0 to 15 years would perform better in Bank A than Bank B.
Question 4.
You decide to invest your money in a bank that uses continuous compounding at 5.5% Interest per year. You have $500.
a. Ja’mie decides to invest $1,000 in the same bank for one year. She predicts she will have double the amount in her account than you will have. Is this prediction correct? Explain.
Answer:
F = 1000 . e0.055 ≈ 1056.54
F = 500 . e0.055 ≈ 528.27
Her prediction is correct. Evaluating the formula with 1,000, we can see that 1000e0.055 = 2 . 500 . e0.055
b. Jonas decides to invest $500 in the same bank as well, but for two years. He predicts that after two years he will have double the amount of cash that you will after one year. Is this prediction correct? Explain.
Answer:
Jonas will earn more than double the amount of interest since the value increasing is in the exponent but will not have more than double the amount of cash.
Question 5.
Use the properties of exponents to identify the percent rate of change of the functions below, and classify them as modeling exponential growth or decay. (The first two problems are done for you.)
a. f(t) = (1.02)t
Answer:
The percent rate of change is 2% and models exponential growth.
b. f(t) = (1.01)12t
Answer:
Since (1.01)t = ((1.01)12)t ≈ (1.1268)t, the percent rate of change is 12.68% and models exponential growth.
c. f(t) = (0.97))t
Answer:
Since (0.97))t = (1 – 0.03))t, the percent rate of change is -3% and models exponential decay.
d. f(t) = 1000(1.2))t
Answer:
The percent rate of change is 20% and models exponential growth.
e. f(t) = \(\frac{(1.07)^{t}}{1000}\)
Answer:
The percent rate of change is 7% and models exponential growth.
f. f(t) = 100 . 3t
Answer:
Since 3t = (1 + 2)t, the percent rate of change is 200% and models exponential growth.
g. f(t) = 1.05.\(\left(\frac{1}{2}\right)^{t}\)
Answer:
Since \(\left(\frac{1}{2}\right)^{t}\) = (o.5)t = (1 – 0.5)t, the percent rate of change is -50% and models exponential decay.
h. f(t) = 80\(\left(\frac{49}{64}\right)^{\frac{1}{2} t}\)
Answer:
Since \(\left(\frac{49}{64}\right)^{\frac{1}{2} t}\) = \(\left(\left(\frac{49}{64}\right)^{\frac{1}{2}}\right)^{t}=\left(\frac{7}{8}\right)^{t}\) = (1 – \(\frac{1}{8}\))t = (1 – 0.125)t, the percent rate of change is -12.5% and models exponential decay.
i. f(t) = 1.02 . (1.13)πt
Answer:
Since (1.13)πt = ((1.13)π)t ≈ (1.468)πt, the percent rate of change is 46.8% and models exponential growth.
Question 6.
The effective rate of an investment is the percent rate of change per year associated with the nominal APR. The effective rate is very useful in comparing accounts with different interest rates and compounding periods. In general, the effective rate can be found with the following formula: rE = \(\left(1+\frac{r}{k}\right)^{k}\) – 1. The effective rate presented here is the interest rate needed for annual compounding to be equal to compounding n times per year.
a. For investing, which account Is better: an account earning a nominal APR of 7% compounded monthly or an account earning a nominal APR of 6. 875% compounded daily? Why?
Answer:
The 7% account is better. The effective rate for the 7% account is \($\left(1+\frac{0.07}{12}\right)^{12}$\) – 1 ≈ 0.07229 compared to the effective rate for the 6.875% account, which is 0.07116.
b. The effective rate formula for an account compounded continuously is rE = er – 1. Would an account earning 6. 875% interest compounded continuously be better than the accounts in part (a)?
Answer:
The effective rate of the account continuously compounded at 6.875% is e0.06875 – 1 ≈ 0.07117, which is
less than the effective rate of the 7% account, so the 7% account is better.
Question 7.
Radioactive decay is the process in which radioactive elements decay into more stable elements. A half-life is the time it takes for half of an amount of an element to decay into a more stable element. For instance, the half-life of uranium-235 is 704 million years.
This means that half of any sample of uranium-235 transforms into lead-207 in 704 million years. We can assume that radioactive decay is modeled by an exponential decay with a constant decay rate.
a. Suppose we have a sample of A g of uranium-235. Write an exponential formula that gives the amount of uranium-235 remaining after m half-lives.
Answer:
The decay rate is constant on average and is 0.5. If the present value is A, then we have
F = A(1 + (-o.50))m, which simplifies to F = A(\(\frac{1}{2}\))m.
b. Does the formula that you wrote in part (a) work for any radioactive element? Why?
Answer:
Since m represents the number of half-lives, this should be an appropriate formula for any decaying element.
c. Suppose we have a sample of A g of uranium-235. What is the decay rate per million years? Write an exponential formula that gives the amount of uranium-235 remaining after t million years.
Answer:
The decay rate is 0.5 every 704 million years. If the present value is A, then we have F = A(1 + (-0.5))\(\frac{t}{704}\) = A(0.5)\(\frac{t}{704}\). This tells us that the growth rate per million years is (0.5)\(\frac{t}{704}\) ≈ 0.9990159005, and the decay rate is 0.0009840995 per million years. Written with this decay rate, the formula becomes F = A(0.9990159005)t
d. How would you calculate the number of years it takes to get to a specific percentage of the original amount of material? For example, how many years will it take for there to be 80% of the original amount of uranium-235 remaining?
Answer:
Set F = 0.80A in our formula and solve for t. For this example, this gives
0.80A = A\(\left(\frac{1}{2}\right)^{\frac{t}{704}}\)
0.80 = \(\left(\frac{1}{2}\right)^{\frac{t}{704}}\)
ln(0.80) = \(\frac{t}{704}\)(ln(\(\frac{1}{2}\)))
t = 704 \(\frac{\ln (0.80)}{\ln (0.5)}\)
t ≈ 226.637
Remember that t represents the number of millions of years. So, it takes approximately 227,000,000 years.
e. How many millions of years would it take 2.35 kg of uranium-235 to decay to 1 kg of uranium?
Answer:
For our formula, the future value is 1 kg, and the present value is 2.35.
1 = 2.35\(\left(\frac{1}{2}\right)^{\frac{t}{704}}\)
\(\frac{1}{2.35}\) = \(\left(\frac{1}{2}\right)^{\frac{t}{704}}\)
ln(\(\frac{1}{2.35}\)) = \(\frac{t}{704}\) . ln(\(\frac{1}{2}\))
t = 704\(\frac{\ln \left(\frac{1}{2.35}\right)}{\ln \left(\frac{1}{2}\right)}\)
t ≈ 867.793
Since t is the number of millions of years, it would take approximately 868 million years for 2.35 kg of uranium-235 to decay to 1 kg.
Question 8.
Doug drank a cup of tea with 130 mg of caffeine. Each hour, the caffeine in Doug’s body diminishes by about 12%. (This rate varies between 6% and 14% depending on the person.)
a. Write a formula to model the amount of caffeine remaining in Doug’s system after each hour.
Answer:
c(t)= 130.(1 – 0.12)t
c(t) = 130 (0.88)t
b. About how long should it take for the level of caffeine in Doug’s system to drop below 30 mg?
Answer:
30 = 130 . (0.88)t
\(\frac{3}{13}\) = 0.88t
ln(\(\frac{3}{13}\)) = t. ln(0.88)
t = \(\frac{\ln \left(\frac{3}{13}\right)}{\ln (0.88)}\)
t ≈ 11.471
The caffeine level is below 30 mg after about 11 hours and 28 minutes.
c. The time it takes for the body to metabolize half of a substance is called its half-life. To the nearest 5 minutes, how long is the half-life for Doug to metabolize caffeine?
Answer:
65 = 130 . (0.88)t
\(\frac{1}{2}\) = 0.88t
ln(\(\frac{1}{2}\)) = t . ln(0.88)
t = \(\frac{\ln \left(\frac{1}{2}\right)}{\ln (0.88)}\)
t ≈ 5.422
The half – life of caffeine in Dough’s system is about 5 hours and 25 minutes.
d. Write a formula to model the amount of caffeine remaining in Doug’s system after m half-lives.
Answer:
c = 130 (\(\frac{1}{2}\))m
Question 9.
A study done from 1950 through 2000 estimated that the world population increased on average by 1.77% each year. In 1950, the world population was 2.519 billion.
a. Write a function p for the world population t years after 1950.
Answer:
p(t) = 2.519 . (1 + 0.0177)t
p(t) = 2. 519 . (1.0177)t
b. If this trend continued, when should the world population have reached 7 billion?
Answer:
7 = 2.519 . (1.0177)t
\(\frac{7}{2.519}\) = 1.0177t
ln(\(\frac{7}{2.519}\)) = t ln(1. 0177)
t = \(=\frac{\ln \left(\frac{7}{2.519}\right)}{\ln (1.0177)}\)
t ≈ 58.252
The model says that the population should reach 7 billion sometime roughly 58 years after 1950. This would be around April 2008.
c. The world population reached 7 billion October 31, 2011, according to the United Nations. Is the model reasonably accurate?
Answer:
Student responses will vary. The model was accurate to within three years, so, yes, it is reasonably accurate.
d. According to the model, when should the world population be greater than 12 billion people?
Answer:
12 = 2.519 . (1.0177)t
\(\frac{12}{2.519}\) = 1.0177t
ln(\(\frac{7}{2.519}\)) = t . ln(1.0177)
t = \(\frac{\ln \left(\frac{12}{2.519}\right)}{\ln (1.0177)}\)
t ≈ 88.973
According to the model, it will take a little less than 89 years from 1950 to get a world population of 12 billion. This would be the year 2039.
Question 10.
A particular mutual fund offers 4.5% nominal APR compounded monthly. Trevor wishes to deposit $1,000.
a. What is the percent rate of change per month for this account?
Answer:
There are twelve months in a year, so \(\frac{4.5 \%}{12}\) = 0.375% = 0.00375.
b. Write a formula for the amount Trevor will have in the account after m months.
Answer:
A = 1000 . (1 + 0.00375)m
A = 1000 . (1.00375))m
c. Doubling time is the amount of time it takes for an investment to double. What is the doubling time of Trevor’s investment?
Answer:
2000 = 1000 . (1. 00375)m
2 = 1.00375m
ln(2) = m ln(1.00375)
m = \(\frac{\ln (2)}{\ln (\mathbf{1 . 0 0 3 7 5})}\)
m ≈ 185.186
It will take 186 months for Trevor’s investment to double. This is 15 years and 6 months.
Question 11.
When paying off loans, the monthly payment first goes to any interest owed before being applied to the remaining balance. Accountants and bankers use tables to help organizing their work.
a. Consider the situation that Fred is paying off a loan of $125,000 with an interest rate of 6% per year compounded monthly. Fred pays $749.44 every month. Complete the following table:
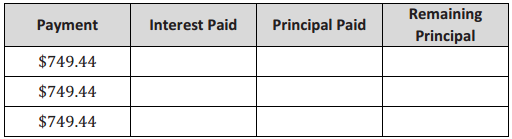
Answer:
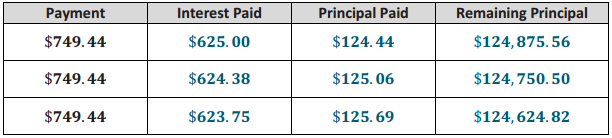
b. Fred’s loan is supposed to last for 30 years. How much will Fred end up paying if he pays $749.44 every month for 30 years? How much of this is interest if his loan was originally for $125,000?
Answer:
$749.44(30)(12) = $269,798.40
Fred will pay $269, 798.40 for his loan. The interest paid will be $269,798.40 – $125,000.00, which is $144,798.40.
Eureka Math Algebra 2 Module 3 Lesson 26 Exit Ticket Answer Key
Question 1.
April would like to invest $200 in the bank for one year. Three banks all have a nominal APR of 1.5% but compound the interest differently.
a. Bank A computes interest just once at the end of the year. What would April’s balance be after one year with this bank?
Answer:
I = 200 . 0.015 = 3
April would have $203 at the end of the year.
b. Bank B compounds interest at the end of each six-month period. What would April’s balance be after one year with this bank?
Answer:
F = 200e0.015 ≈ 203.01
April would have $203.01 at the end of the year.
c. Bank C compounds interest continuously. What would April’s balance be after one year with this bank?
Answer:
F = 200e0.015 ≈ 203.02
April would have $203.02 at the end of the year.
d. Each bank decides to double the nominal APR It offers for one year. That is, they offer a nominal APR of 3%. Each bank advertises, “DOUBLE THE AMOUNT YOU EARN !“ For which of the three banks, if any, is this advertised claim correct?
Answer:
Bank A:
I = 200 . 0.03 = 6
Bank B:
F = 200(1 + \(\frac{0.03}{2}\))2 ≈ 206.045
Bank C:
F = 200e0.015 ≈ 206.09
All three banks earn at least twice as much with a double interest rate. Bank A earns exactly twice as much, Bank B earns 2 cents more than twice as much, and Bank C earns 5 cents more than twice as much.