Eureka Math Algebra 1 Module 4 Lesson 11 Answer Key

Engage NY Eureka Math Algebra 1 Module 4 Lesson 11 Answer Key

Eureka Math Algebra 1 Module 4 Lesson 11 Example Answer Key

Example
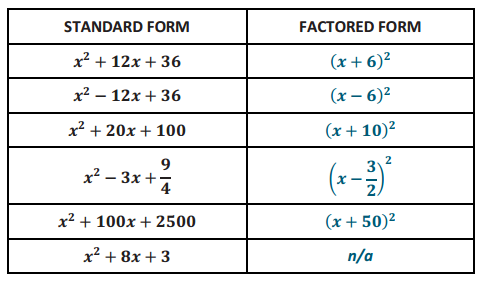
Now try working backward. Rewrite the following standard form quadratic expressions as perfect squares.
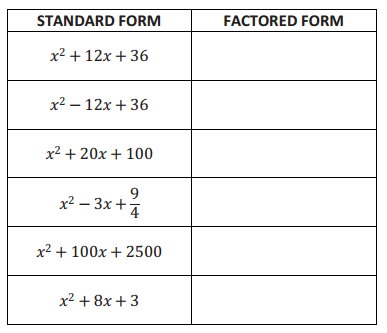
$Engage NY Math Algebra 1 Module 4 Lesson 11 Example Answer Key 1$
Answer:
$Engage NY Math Algebra 1 Module 4 Lesson 11 Example Answer Key 2$

Eureka Math Algebra 1 Module 4 Lesson 11 Exploratory Challenge Answer Key

Exploratory Challenge
Find an expression equivalent to x² + 8x + 3 that includes a perfect square binomial.
Answer:
(x + 4)² – 13

Eureka Math Algebra 1 Module 4 Lesson 11 Exercise Answer Key

Opening Exercise
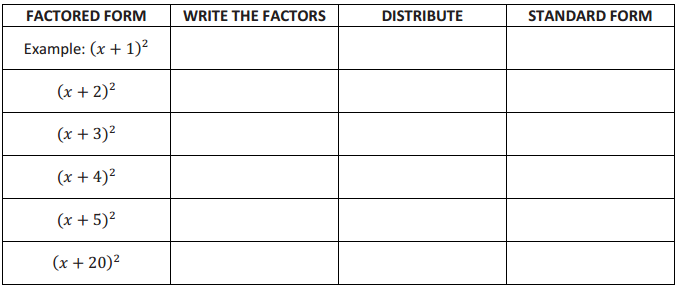
Rewrite the following perfect square quadratic expressions in standard form. Describe patterns in the coefficients for the factored form, (x + A)², and the standard form, x² + bx + c.
$Engage NY Math Algebra 1 Module 4 Lesson 11 Exercise Answer Key 1$
Answer:
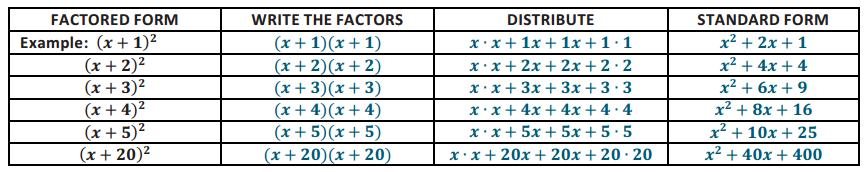
$Engage NY Math Algebra 1 Module 4 Lesson 11 Exercise Answer Key 2$
For each row, the factored form and standard form are equivalent expressions, so (x + A)²=x² + bx + c. A, the constant in factored form of the equation, is always half of b, the coefficient of the linear term in the standard form. c, the constant term in the standard form of the quadratic equation, is always the square of the constant in the factored form, A.

Exercises
Rewrite each expression by completing the square.
Exercise 1.
a² – 4a + 15
Answer:
(a – 2)² + 11
(Note: Since the constant term required to complete the square is less than the constant term, + 15, students may notice that they just need to split the + 15 strategically.)

Exercise 2.
n² – 2n – 15
Answer:
(n – 1)² – 16

Exercise 3.
c² + 20c – 40
Answer:
(c + 10)² – 140

Exercise 4.
x² – 1000x + 60 000
Answer:
(x – 500)² – 190 000

Exercise 5.
y² – 3y + 10
Answer:
(y – $\frac{3}{2}$)² + $\frac{31}{4}$

Exercise 6.
k² + 7k + 6
Answer:
(k + $\frac{7}{2}$)² – $\frac{25}{4}$

Exercise 7.
z² – 0.2z + 1.5
Answer:
(z – 0.1)² + 1.49

Exercise 8.
p² + 0.5p + 0.1
Answer:
(p + 0.25)² + 0.0375

Exercise 9.
j² – $\frac{3}{4}$ j + $\frac{3}{4}$
Answer:
(j – $\frac{3}{8}$)² + $\frac{39}{64}$

Exercise 10.
x² – bx + c
Answer:
(x – $\frac{b}{2}$)² + c – $\frac{b^{2}}{4}$

Eureka Math Algebra 1 Module 4 Lesson 11 Problem Set Answer Key

Rewrite each expression by completing the square.
Question 1.
q² + 12q + 32
Answer:
(q + 6)² – 4

Question 2.
m² – 4m – 5
Answer:
(m – 2)² – 9

Question 3.
x² – 7x + 6.5
Answer:
(x – $\frac{7}{2}$)² – 5.75

Question 4.
a² + 70a + 1225
Answer:
(a + 35)²

Question 5.
z² – 0.3z + 0.1
Answer:
(z – 0.15)² + 0.0775

Question 6.
y² – 6by + 20
Answer:
(y – 3b)² + 20 – 9b²

Question 7.
Which of these expressions would be most easily rewritten by factoring? Justify your answer.
Answer:
Students may respond with either Problem 1, 2, or 4, and justifications may range from a demonstration of the factoring process to a written explanation where students show that the product – sum rule can be applied to either of these expressions.

Eureka Math Algebra 1 Module 4 Lesson 11 Exit Ticket Answer Key

Question 1.
Rewrite the expression r² + 4r + 3, first by factoring and then by completing the square. Which way is easier?
Explain why you think so.
Answer:
By factoring: (r + 3)(r + 1)
By completing the square: (r + 2)² – 1
Both options are fairly simple, and students may select either for their preference. The important thing is that they are thinking about efficiency in their methods and the various options available for rewriting quadratic expressions.