Looking for better preparation opportunities regarding the Geometry Concepts then try out our Big Ideas Math Geometry Answers Chapter 3 Parallel and Perpendicular Lines. Practice using the BIM Book Geometry Solution Key and clear all your doubts on the Ch 3 Parallel and Perpendicular Lines. All the Questions prepared in the Parallel and Perpendicular Lines Big Ideas Math Geometry Ch 3 Answer Key are as per the common core curriculum.
Big Ideas Math Geometry Answer Key Chapter 3 Parallel and Perpendicular Lines covers questions from Exercises, Chapter Tests, Review Tests, Assessments, Cumulative Practice, etc. Learn Math in a fun way and practice Big Ideas Math Geometry Chapter 3 Parallel and Perpendicular Lines Answers on a daily basis. Enhance your confidence levels by solving from the Parallel and Perpendicular Lines Big Ideas Math Geometry Answers Chapter 3 and attempt the exams well.
Big Ideas Math Book Geometry Answer Key Chapter 3 Parallel and Perpendicular Lines
Learn the concepts quickly using the BIM Book Geometry Answer Key Chapter 3 Parallel and Perpendicular Lines. For a better learning experience, we have compiled all the Big Ideas Math Geometry Answers Chapter 3 as per the Big Ideas Math Geometry Textbooks format. You can find all the concepts via the quick links available below. Simply tap on them and learn the fundamentals involved in the Parallel and Perpendicular Lines Chapter.
- Parallel and Perpendicular Lines Maintaining Mathematical Proficiency – Page 123
- Parallel and Perpendicular Lines Mathematical Practices – Page 124
- 3.1 Pairs of Lines and Angles – Page(125-130)
- Lesson 3.1 Pairs of Lines and Angles – Page(126-128)
- Exercise 3.1 Pairs of Lines and Angles – Page(129-130)
- 3.2 Parallel Lines and Transversals – Page(131-136)
- Lesson 3.2 Parallel Lines and Transversals – Page(132-134)
- Exercise 3.2 Parallel Lines and Transversals – Page(135-136)
- 3.3 Proofs with Parallel Lines – Page(137-144)
- Lesson 3.3 Proofs with Parallel Lines – Page(138-141)
- Exercise 3.3 Proofs with Parallel Lines – Page(142-144)
- 3.1 – 3.3 Study Skills: Analyzing Your Errors – Page 145
- 3.1 – 3.3 Quiz – Page 146
- 3.4 Proofs with Perpendicular Lines – Page(147-154)
- Lesson 3.4 Proofs with Perpendicular Lines – Page(148-151)
- Exercise 3.4 Proofs with Perpendicular Lines – Page(152-154)
- 3.5 Equations of Parallel and Perpendicular Lines – Page(155-162)
- Lesson 3.5 Equations of Parallel and Perpendicular Lines – Page(156-159)
- Exercise 3.5 Equations of Parallel and Perpendicular Lines – Page(160-162)
- 3.4 – 3.5 Performance Task: Navajo Rugs – Page 163
- Parallel and Perpendicular Lines Chapter Review – Page(164-166)
- Parallel and Perpendicular Lines Test – Page 167
- Parallel and Perpendicular Lines Cumulative Assessment – Page(168-169)
Parallel and Perpendicular Lines Maintaining Mathematical Proficiency
Find the slope of the line.
Question 1.
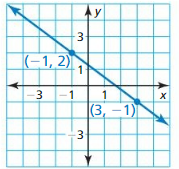
Answer:
From the given coordinate plane,
Let the given points are:
A (-1, 2), and B (3, -1)
Compare the given points with
A (x1, y1), B (x2, y2)
We know that,
Slope of the line (m) = \(\frac{y2 – y1}{x2 – x1}\)
So,
Slope of the line (m) = \(\frac{-1 – 2}{3 + 1}\)
= \(\frac{-3}{4}\)
Hence, from the above,
We can conclude that the slope of the given line is: \(\frac{-3}{4}\)
Question 2.
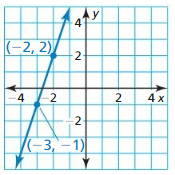
Answer:
From the given coordinate plane,
Let the given points are:
A (-2, 2), and B (-3, -1)
Compare the given points with
A (x1, y1), B (x2, y2)
We know that,
Slope of the line (m) = \(\frac{y2 – y1}{x2 – x1}\)
So,
Slope of the line (m) = \(\frac{-1 – 2}{-3 + 2}\)
= \(\frac{-3}{-1}\)
= 3
Hence, from the above,
We can conclude that the slope of the given line is: 3
Question 3.
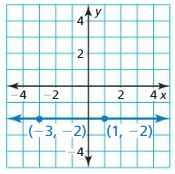
Answer:
From the given coordinate plane,
Let the given points are:
A (-3, -2), and B (1, -2)
Compare the given points with
A (x1, y1), B (x2, y2)
We know that,
Slope of the line (m) = \(\frac{y2 – y1}{x2 – x1}\)
So,
Slope of the line (m) = \(\frac{-2 + 2}{3 + 1}\)
= \(\frac{0}{4}\)
= 0
Hence, from the above,
We can conclude that the slope of the given line is: 0
Write an equation of the line that passes through the given point and has the given slope.
Question 4.
(6, 1); m = – 3
Answer:
The given point is: (6, 1)
The slope of the given line is: m = -3
We know that,
The equation of a line is:
y = mx + b
Where,
m is the slope
b is the y-intercept
So,
y = -3x + b ——–(1)
To find the value of b,
Substitute the given point in eq. (1)
So,
1 = -3 (6) + b
1 = -18 + b
1 + 18 = b
b = 19
Hence,
The equation of the line along with y-intercept is:
y = -3x + 19
Question 5.
(-3, 8); m = – 2
Answer:
The given point is: (-3, 8)
The slope of the given line is: m = -2
We know that,
The equation of a line is:
y = mx + b
Where,
m is the slope
b is the y-intercept
So,
y = -2x + b ——–(1)
To find the value of b,
Substitute the given point in eq. (1)
So,
8 = -2 (-3) + b
8 = 6 + b
8 – 6 = b
b = 2
Hence,
The equation of the line along with y-intercept is:
y = -2x + 2
Question 6.
(- 1, 5); m = 4
Answer:
The given point is: (-1, 5)
The slope of the given line is: m = 4
We know that,
The equation of a line is:
y = mx + b
Where,
m is the slope
b is the y-intercept
So,
y = 4x + b ——–(1)
To find the value of b,
Substitute the given point in eq. (1)
So,
5 = 4 (-1) + b
5 = -4 + b
5 + 4 = b
b = 9
Hence,
The equation of the line along with y-intercept is:
y = 4x + 9
Question 7.
(2, – 4); m = \(\frac{1}{2}\)
Answer:
The given point is: (2, -4)
The slope of the given line is: m = \(\frac{1}{2}\)
We know that,
The equation of a line is:
y = mx + b
Where,
m is the slope
b is the y-intercept
So,
y = \(\frac{1}{2}\)x + b ——–(1)
To find the value of b,
Substitute the given point in eq. (1)
So,
-4 = \(\frac{1}{2}\) (2) + b
-4 = 1 + b
-4 – 1 = b
b = -5
Hence,
The equation of the line along with y-intercept is:
y = \(\frac{1}{2}\)x – 5
Question 8.
(- 8, – 5); m = –\(\frac{1}{4}\)
Answer:
The given point is: (-8, -5)
The slope of the given line is: m = –\(\frac{1}{4}\)
We know that,
The equation of a line is:
y = mx + b
Where,
m is the slope
b is the y-intercept
So,
y = –\(\frac{1}{4}\)x + b ——–(1)
To find the value of b,
Substitute the given point in eq. (1)
So,
-5 = –\(\frac{1}{4}\) (-8) + b
-5 = 2 + b
-5 – 2 = b
b = -7
Hence,
The equation of the line along with y-intercept is:
y = –\(\frac{1}{4}\)x – 7
Question 9.
(0, 9); m = \(\frac{2}{3}\)
Answer:
The given point is: (0, 9)
The slope of the given line is: m = \(\frac{2}{3}\)
We know that,
The equation of a line is:
y = mx + b
Where,
m is the slope
b is the y-intercept
So,
y = \(\frac{2}{3}\)x + b ——–(1)
To find the value of b,
Substitute the given point in eq. (1)
So,
9 = \(\frac{2}{3}\) (0) + b
9 = 0 + b
9 – 0 = b
b = 9
Hence,
The equation of the line along with y-intercept is:
y = \(\frac{2}{3}\)x + 9
Question 10.
ABSTRACT REASONING
Why does a horizontal line have a slope of 0, but a vertical line has an undefined slope?
Answer:
We know that,
Slope of the line (m) = \(\frac{y2 – y1}{x2 – x1}\)
We know that,
For a horizontal line,
The coordinates of y are the same. i.e.,
y1 = y2 = y3 ………
For a vertical line,
The coordinates of x are the same. i.e.,
x1 = x2 = x3 …….
So,
The slope of the horizontal line (m) = \(\frac{y2 – y2}{x2 – x1}\)
We know that,
Any fraction that contains 0 in the numerator has its value equal to 0
So,
The slope of horizontal line (m) = 0
The slope of vertical line (m) = \(\frac{y2 – y1}{x2 – x1}\)
We know that,
Any fraction that contains 0 in the denominator has its value undefined
So,
The slope of the vertical line (m) = Undefined
Parallel and Perpendicular Lines Mathematical Practices
Use a graphing calculator to graph the pair of lines. Use a square viewing window. Classify the lines as parallel, perpendicular, coincident, or non-perpendicular intersecting lines. Justify your answer.
Question 1.
x + 2y = 2
2x – y = 4
Answer:
The given pair of lines are:
x + 2y = 2
2x – y = 4
Hence,
The representation of the given pair of lines in the coordinate plane is:
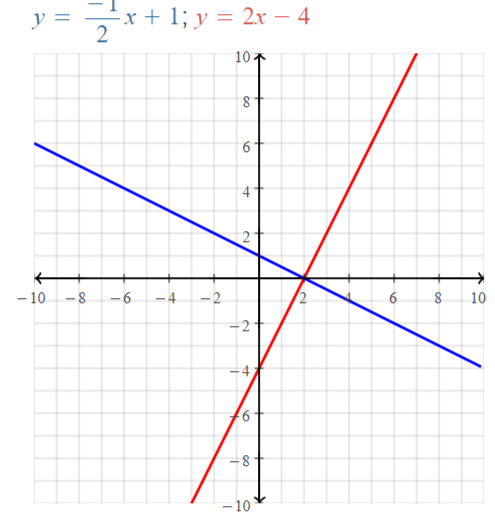
We know that,
For a pair of lines to be perpendicular, the product of the slopes i.e., the product of the slope of the first line and the slope of the second line will be equal to -1
So,
By comparing the given pair of lines with
y = mx + b
We get
The slope of first line (m1) = –\(\frac{1}{2}\)
The slope of second line (m2) = 2
So,
m1 ×m2 = –\(\frac{1}{2}\) × 2
= -1
Hence, from the above,
We can conclude that the given pair of lines are perpendicular lines
Question 2.
x + 2y = 2
2x + 4y = 4
Answer:
The give pair of lines are:
x + 2y = 2
2x + 4y = 4
Hence,
The representation of the given pair of lines in the coordinate plane is:
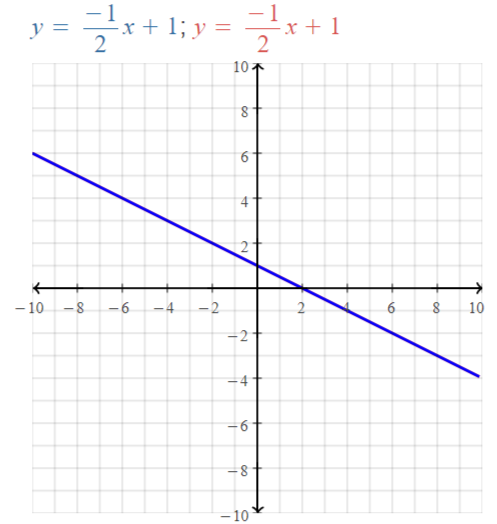
We know that,
For a pair of lines to be coincident, the pair of lines have the same slope and the same y-intercept
So,
By comparing the given pair of lines with
y = mx + b
We get
m1 = –\(\frac{1}{2}\), b1 = 1
m2 = –\(\frac{1}{2}\), b2 = 1
Hence, from the above,
We can conclude that the given pair of lines are coincident lines
Question 3.
x + 2y = 2
x + 2y = – 2
Answer:
The given pair of lines are:
x + 2y = 2
x + 2y = -2
Hence,
The representation of the given pair of lines in the coordinate plane is:
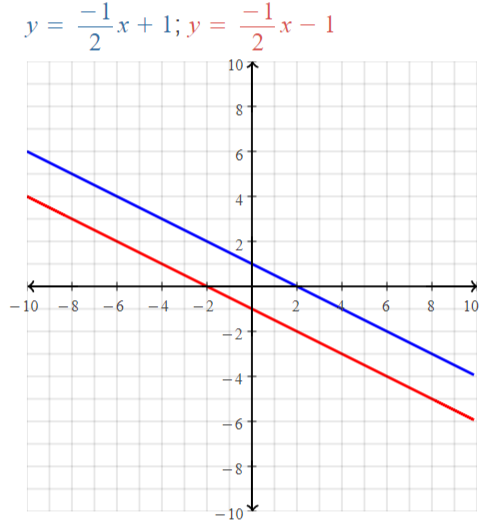
We know that,
For a pair of lines to be parallel, the pair of lines have the same slope but different y-intercepts
So,
By comparing the given pair of lines with
y = mx + b
We get
m1 = –\(\frac{1}{2}\), b1 = 1
m2 = \(\frac{1}{2}\), b2 = -1
Hence, from the above,
We can conclude that the given pair of lines are parallel lines
Question 4.
x – 2y = 2
x – y = – 4
Answer:
The given pair of lines are:
x – 2y = 2
x – y = -4
Hence,
The representation of the given pair of lines in the coordinate plane is:
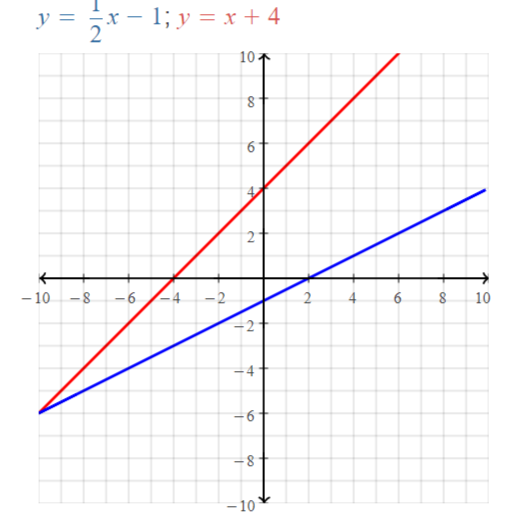
We know that,
For a pair of lines to be non-perpendicular, the product of the slopes i.e., the product of the slope of the first line and the slope of the second line will not be equal to -1
So,
By comparing the given pair of lines with
y = mx + b
We get
The slope of first line (m1) = \(\frac{1}{2}\)
The slope of second line (m2) = 1
So,
m1 ×m2 = \(\frac{1}{2}\)
Hence, from the above,
We can conclude that the given pair of lines are non-perpendicular lines
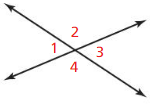
3.1 Pairs of Lines and Angles
Exploration 1
Points of intersection
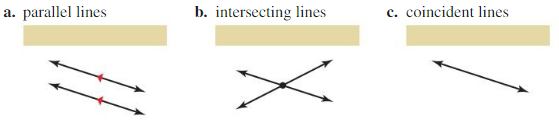
work with a partner: Write the number of points of intersection of each pair of coplanar lines.
Answer:
The given coplanar lines are:

a. The points of intersection of parallel lines:
We know that,
The “Parallel lines” have the same slope but have different y-intercepts
So,
We can say that any parallel line do not intersect at any point
Hence, from the above,
We can conclude that the number of points of intersection of parallel lines is: 0
a. The points of intersection of intersecting lines:
We know that,
The “Intersecting lines” have a common point to intersect
So,
We can say that any intersecting line do intersect at 1 point
Hence, from the above,
We can conclude that the number of points of intersection of intersecting lines is: 1
c. The points of intersection of coincident lines:
We know that,
The “Coincident lines” may be intersecting or parallel
So,
We can say that any coincident line do not intersect at any point or intersect at 1 point
Hence, from the above,
We can conclude that the number of points of intersection of coincident lines is: 0 or 1
Exploration 2
Classifying Pairs of Lines
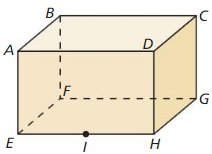
Work with a partner: The figure shows a right rectangular prism. All its angles are right angles. Classify each of the following pairs of lines as parallel, intersecting, coincident, or skew. Justify your answers. (Two lines are skew lines when they do not intersect and are not coplanar.)

Answer:
The given rectangular prism is:

We know that,
The “Parallel lines” are the lines that do not intersect with each other and present in the same plane
The “Intersecting lines” are the lines that intersect with each other and in the same plane
The “Coincident lines” are the lines that lie on one another and in the same plane
The “Skew lines” are the lines that do not present in the same plane and do not intersect
Hence,
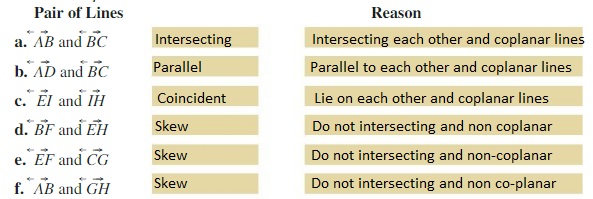
The completed table of the nature of the given pair of lines is:
Exploration 3
Identifying Pairs of Angles
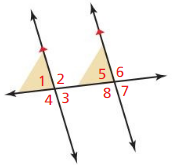
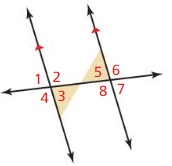
Work with a partner: In the figure, two parallel lines are intersected by a third line called a transversal.
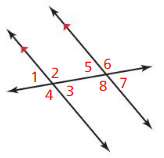
a. Identify all the pairs of vertical angles. Explain your reasoning.
CONSTRUCTING VIABLE ARGUMENTS
To be proficient in math, you need to understand and use stated assumptions, definitions, and previously established results.
Answer:
We know that,
The angles that are opposite to each other when two lines cross are called “Vertical angles”
Hence, from the given figure,
We can conclude that the vertical angles are:
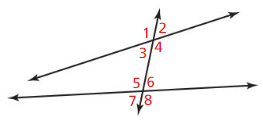
∠1 and ∠3; ∠2 and ∠4; ∠5 and ∠7; ∠6 and ∠8
b. Identify all the linear pairs of angles. Explain your reasoning.
Answer:
We know that,
A “Linear pair” is a pair of adjacent angles formed when two lines intersect
Hence, from the given figure,
We can conclude that the linear pair of angles is:
∠1 and ∠2; ∠4 and ∠3; ∠5 and ∠6; ∠8 and ∠7
Communicate Your Answer
Question 4.
What does it mean when two lines are parallel, intersecting, coincident, or skew?
Answer:
The two lines are “Parallel” when they do not intersect each other and are coplanar
The two lines are “Intersecting” when they intersect each other and are coplanar
The two lines are “Coincident” when they lie on each other and are coplanar
The two lines are “Skew” when they do not intersect each other and are not coplanar
Question 5.
In Exploration 2. find more pairs of lines that are different from those given. Classify the pairs of lines as parallel, intersecting, coincident, or skew. Justify your answers.
Answer:
The given rectangular prism of Exploration 2 is:

The pair of lines that are different from the given pair of lines in Exploration 2 are:
a. \(\overline{C D}\) and \(\overline{A E}\)
b. \(\overline{D H}\) and \(\overline{F G}\)
Hence, from the above,
We can conclude that
a. \(\overline{C D}\) and \(\overline{A E}\) are “Skew lines” because they are not intersecting and are non coplanar
b. \(\overline{D H}\) and \(\overline{F G}\) are “Skew lines” because they are not intersecting and are non coplanar
Lesson 3.1 Pairs of Lines and Angles
Monitoring Progress
Question 1.
Look at the diagram in Example 1. Name the line(s) through point F that appear skew to ![]() .
.
Answer:
From Example 1,
We can observe that
The line that passes through point F that appear skew to \(\overline{E H}\) is: \(\overline{F C}\)
Question 2.
In Example 2, can you use the Perpendicular Postulate to show that ![]() is not perpendicular to
is not perpendicular to ![]() ? Explain why or why not.
? Explain why or why not.
Answer:
Perpendicular Postulate:
According to this Postulate,
If there is a line and a point not on the line, then there is exactly one line through the point perpendicular to the given line
Now,
In Example 2,
We can observe that \(\overline{A C}\) is not perpendicular to \(\overline{B F}\) because according to the perpendicular Postulate, \(\overline{A C}\) will be a straight line but it is not a straight line when we observe Example 2
Hence, from the above,
We can conclude that we can use “Perpendicular Postulate” to show that \(\overline{A C}\) is not perpendicular to \(\overline{B F}\)
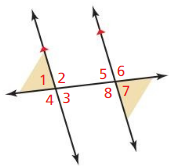
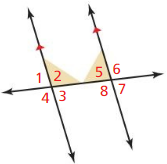
Classify the pair of numbered angles.
Question 3.
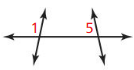
Answer:
The given figure is:

We know that,
The angles that have the same corner are called “Adjacent angles”
Hence, from the above,
We can conclude that ∠1 and ∠5 are the adjacent angles
Question 4.
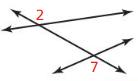
Answer:
The given figure is:

We know that,
The angles that have the opposite corners are called “Vertical angles”
Hence, from the above,
We can conclude that ∠2 and ∠7 are the “Vertical angles”
Question 5.
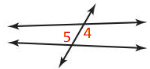
Answer:
The given figure is:

We know that,
The angles that have the opposite corners are called “Vertical angles”
Hence, from the above,
We can conclude that ∠4 and ∠5 are the “Vertical angles”
Exercise 3.1 Pairs of Lines and Angles
Vocabulary and Core Concept Check
Question 1.
COMPLETE THE SENTENCE
Two lines that do not intersect and are also not parallel are ________ lines.
Answer:
Question 2.
WHICH ONE did DOESN’T BELONG?
Which angle pair does not belong with the other three? Explain our reasoning.
∠2 and ∠3
∠4 and ∠5
∠1 and ∠8
∠2 and∠7
Answer:
The given figure is:

We know that,
The angles that have the common side are called “Adjacent angles”
The angles that are opposite to each other when 2 lines cross are called “Vertical angles”
So,
∠2 and ∠3 are vertical angles
∠4 and ∠5 are adjacent angles
∠1 and ∠8 are vertical angles
∠2 and ∠7 are vertical angles
Hence, from the above,
We can conclude that ∠4 and ∠5 angle-pair do not belong with the other three
Monitoring Progress and Modeling with Mathematics
In Exercises 3 – 6, think of each segment in the diagram as part of a line. All the angles are right angles. Which line(s) or plane(s) contain point B and appear to fit the description?

Question 3.
line(s) parallel to ![]() .
.
Answer:
![]()
Question 4.
line(s) PerPendicular to ![]() .
.
Answer:
We know that,
The lines that are a straight angle with the given line and are coplanar is called “Perpendicular lines”
So,
From the given figure,
We can conclude that the line that is perpendicular to \(\overline{C D}\) is: \(\overline{A D}\) and \(\overline{C B}\)
Question 5.
line(s) skew to ![]()
Answer:
![]()
Question 6.
plane(s) parallel to plane CDH
Answer:
From the given figure,
We can observe that the plane parallel to plane CDH is: Plane BAE
In Exercises 7-10, Use the diagram.
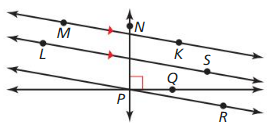
Question 7.
Name a pair of parallel lines.
Answer:
![]()
Question 8.
Name a pair of perpendicular lines.
Answer:
We know that,
The lines that have an angle of 90° with each other are called “Perpendicular lines”
Hence,
From the figure,
We can conclude that \(\overline{N P}\) and \(\overline{P O}\) are perpendicular lines
Question 9.
![]()
Answer:
![]()
Question 10.
![]()
Answer:
We know that,
The lines that have an angle of 90° with each other are called “Perpendicular lines”
Hence,
From the figure,
We can conclude that \(\overline{P R}\) and \(\overline{P O}\) are not perpendicular lines
In Exercises 11-14, identify all pairs of angles of the given type.
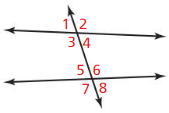
Question 11.
corresponding
Answer:
![]()
Question 12.
alternate interior
Answer:
We know that,
“Alternate Interior angles” are a pair of angles on the inner side of each of those two lines but on opposite sides of the transversal.
Hence,
From the given figure,
We can conclude that the alternate interior angles are: ∠4 and ∠5; ∠3 and ∠6
Question 13.
alternate exterior
Answer:
![]()
Question 14.
consecutive interior
Answer:
We know that,
The pair of angles on one side of the transversal and inside the two lines are called the “Consecutive interior angles”.
Hence,
From the given figure,
We can conclude that the consecutive interior angles are: ∠3 and ∠5; ∠4 and ∠6
USING STRUCTURE
In Exercises 15-18, classify the angle pair as corresponding. alternate interior, alternate exterior, or consecutive interior angles.
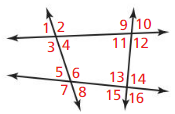
Question 15.
∠5 and ∠1
Answer:
![]()
Question 16.
∠11 and ∠13
Answer:
We know that,
The pair of angles on one side of the transversal and inside the two lines are called the “Consecutive interior angles”.
Hence,
From the given figure,
We can conclude that ∠11 and ∠13 are the “Consecutive interior angles”
Question 17.
∠6 and ∠13
Answer:
![]()
Question 18.
∠2 and ∠11
Answer:
We know that,
“Vertical Angles” are the angles opposite each other when two lines cross
Hence, from the above figure,
We can conclude that ∠2 and ∠11 are the “Vertical angles”
ERROR ANALYSIS
In Exercises 19 and 20. describe and correct the error in the conditional statement about lines.
Question 19.

Answer:

Question 20.

Answer:
We know that,
The “Perpendicular Postulate” states that if there is a line and a point not on the line, then there is exactly one line through the point perpendicular to the given line.
Hence, from the above,
We can conclude that the given statement is not correct
Question 21.
MODELING WITH MATHEMATICS
Use the photo to decide whether the statement is true or false. Explain Your reasoning.

a. The plane containing the floor of the treehouse is parallel to the ground.
b. The lines containing the railings of the staircase, such as ![]() , are skew to all lines in the plane containing the ground.
, are skew to all lines in the plane containing the ground.
c. All the lines containing the balusters. such as ![]() , are perpendicular to the plane containing the floor of the treehouse.
, are perpendicular to the plane containing the floor of the treehouse.
Answer:

Question 22.
THOUGHT-PROVOKING
If two lines are intersected by a third line, is the third line necessarily a transversal? Justify your answer with a diagram.
Answer:
No, the third line does not necessarily be a transversal
Explanation:
We know that,
“Parallel lines” do not intersect each other
“Perpendicular lines” intersect at each other at right angles
The third intersecting line can intersect at the same point that the two lines have intersected as shown below:
Hence, from the above,
We can conclude that the third line does not need to be a transversal
Question 23.
MATHEMATICAL CONNECTIONS
Two lines are cut by a transversal. Is it possible for all eight angles formed to have the same measure? Explain your reasoning.
Answer:

Question 24.
HOW DO YOU SEE IT?
Think of each segment in the figure as part of a line.
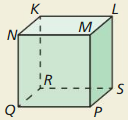
a. Which lines are parallel to ![]() ?
?
Answer:
We know that,
The lines that do not intersect to each other and are coplanar are called “Parallel lines”
Hence, from the above figure,
We can conclude that the line parallel to \(\overline{N Q}\) is: \(\overline{M P}\)
b. Which lines intersect ![]() ?
?
Answer:
We know that,
The lines that are coplanar and any two lines that have a common point are called “Intersecting lines”
Hence, from the above figure,
We can conclude that the lines that intersect \(\overline{N Q}\) are: \(\overline{N K}\), \(\overline{N M}\), and \(\overline{Q P}\)
c. Which lines are skew to ![]() ?
?
Answer:
We know that,
The lines that do not intersect or not parallel and non-coplanar are called “Skew lines”
Hence, from the above figure,
We can conclude that \(\overline{K L}\), \(\overline{L M}\), and \(\overline{L S}\)
d. Should you have named all the lines on the cube in parts (a)-(c) except \(\overline{N Q}\)? Explain.
Answer:
No, we did not name all the lines on the cube in parts (a) – (c) except \(\overline{N Q}\)
In exercises 25-28. copy and complete the statement. List all possible correct answers.
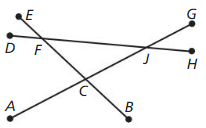
Question 25.
∠BCG and __________ are corresponding angles.
Answer:
![]()
Question 26.
∠BCG and __________ are consecutive interior angles.
Answer:
We know that,
When two lines are cut by a transversal, the pair of angles on one side of the transversal and inside the two lines are called the “Consecutive interior angles”
Hence,
From the given figure,
We can conclude that the consecutive interior angles of ∠BCG are: ∠FCA and ∠BCA
Question 27.
∠FCJ and __________ are alternate interior angles.
Answer:
![]()
Question 28.
∠FCA and __________ are alternate exterior angles.
Answer:
“Alternate exterior angles” are the pair of angles that lie on the outer side of the two parallel lines but on either side of the transversal line
Hence,
From the above figure,
We can conclude that ∠FCA and ∠JCB are alternate exterior angles
Question 29.
MAKING AN ARGUMENT
Your friend claims the uneven parallel bars in gymnastics are not really Parallel. She says one is higher than the other. so they cannot be on the same plane. Is she correct? Explain.

Answer:
Maintaining Mathematical Proficiency
Use the diagram to find the measure of all the angles.
Question 30.
m∠1 = 76°
Answer:
We know that,
The sum of the adjacent angles is: 180°
The vertical angles are congruent i.e., the angle measures of the vertical angles are equal
Now,
From the figure,
The adjacent angles are: ∠1 and ∠2; ∠2 and ∠3; ∠3 and ∠4; and ∠4 and ∠1
The vertical angles are: ∠1 and ∠3; ∠2 and ∠4
Now,
∠1 + ∠2 = 180°
∠2 = 180° – ∠1
= 180° – 76°
= 104°
So,
∠3 = 76° and ∠4 = 104°
Hence, from the above,
We can conclude that,
∠1 = 76°, ∠2 = 104°, ∠3 = 76°, and ∠4 = 104°
Question 31.
m∠2 = 159°
Answer:

3.2 Parallel Lines and Transversals
Exploration 1
Exploring parallel Lines
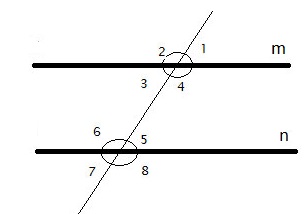
Work with a partner: Use dynamic geometry software to draw two parallel lines. Draw a third line that intersects both parallel lines. Find the measures of the eight angles that are formed. What can you conclude?
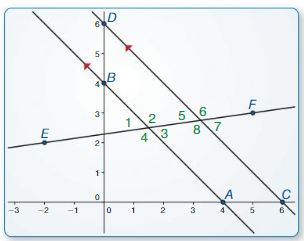
Answer:
By using the dynamic geometry,
The representation of the given coordinate plane along with parallel lines is:
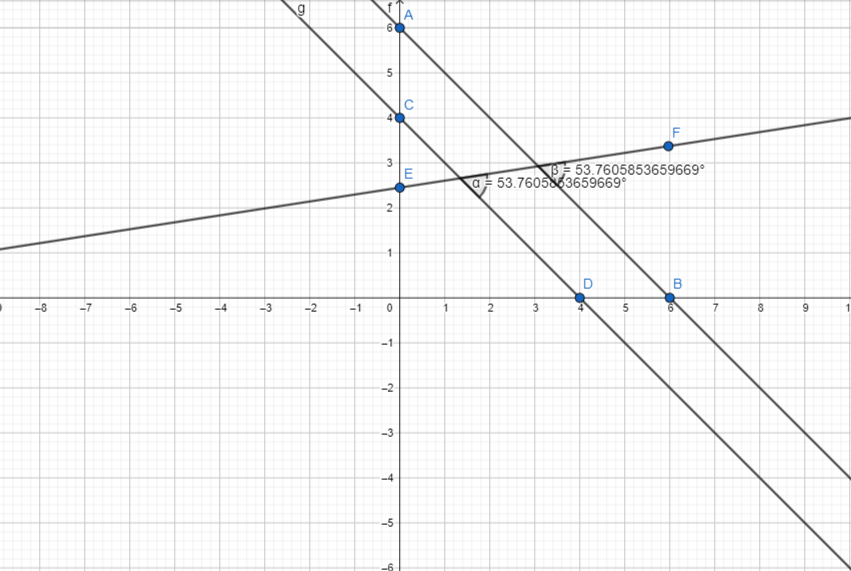
Hence, from the coordinate plane,
We can observe that,
∠3 = 53.7° and ∠4 = 53.7°
We know that,
The angle measures of the vertical angles are congruent
So,
∠1 = 53.7° and ∠5 = 53.7°
We know that,
In the parallel lines,
All the angle measures are equal
Hence, from the above,
We can conclude that
∠1 = ∠2 = ∠3 = ∠4 = ∠5 = ∠6 = ∠7 = 53.7°
Exploration 2
Writing conjectures
Work with a partner. Use the results of Exploration 1 to write conjectures about the following pairs of angles formed by two parallel lines and a transversal.
ATTENDING TO PRECISION
To be proficient in math, you need to communicate precisely with others.
a. corresponding angles
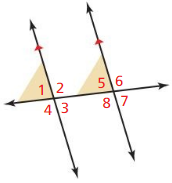
Answer:
We know that,
When two lines are crossed by another line (which is called the Transversal), the angles in matching corners are called “Corresponding angles”
Hence, from the given figure,
We can conclude that
The corresponding angles are: ∠ and ∠5; ∠4 and ∠8
b. alternate interior angles
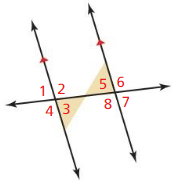
Answer:
We know that,
“Alternate Interior Angles” are a pair of angles on the inner side of each of those two lines but on opposite sides of the transversal.
Hence, from the above figure,
We can conclude that
The alternate interior angles are: ∠3 and ∠5; ∠2 and ∠8
c. alternate exterior angles
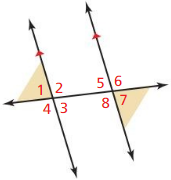
Answer:
We know that,
“Alternate exterior angles” are the pair of angles that lie on the outer side of the two parallel lines but on either side of the transversal line.
Hence, from the above figure,
We can conclude that
The alternate exterior angles are: ∠1 and ∠7; ∠6 and ∠4
d. consecutive interior angles
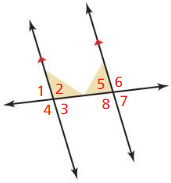
Answer:
We know that,
When two lines are cut by a transversal, the pair of angles on one side of the transversal and inside the two lines are called the “Consecutive interior angles”
Hence, from the above figure,
We can conclude that
The consecutive interior angles are: ∠2 and ∠5; ∠3 and ∠8
Communicate Your Answer
Question 3.
When two parallel lines are cut by a transversal, which of the resulting pairs of angles are congruent?
Answer:
If two parallel lines are cut by a transversal, then the pairs of “Corresponding angles” are congruent.
If two parallel lines are cut by a transversal, then the pairs of “Alternate interior angles” are congruent.
If two parallel lines are cut by a transversal, then the pairs of “Alternate exterior angles” are congruent.
Question 4.
In Exploration 2. m∠1 = 80°. Find the other angle measures.
Answer:
It is given that
In Exploration 2,
∠1 = 80°
We know that,
Exploration 2 comes from Exploration 1
From Exploration 1,
We can say that all the angle measures are equal in Exploration 1
Hence, from the above,
We can conclude that
∠1 = ∠2 = ∠3 = ∠4 = ∠5 = ∠6 = ∠7 = ∠8 = 80°
Lesson 3.2 Parallel Lines and Transversals
Monitoring Progress
Use the diagram
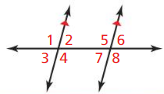
Question 1.
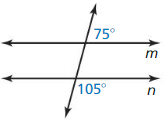
Given m∠1 = 105°, find m∠4, m∠5, and m∠8. Tell which theorem you use in each case.
Answer:
It is given that ∠1 = 105°
Now,
We have to find ∠4, ∠5, and ∠8
Now,
To find ∠4:
Verticle angle theorem:
Vertical Angles Theorem states that vertical angles, angles that are opposite each other and formed by two intersecting straight lines, are congruent
So,
∠1 = ∠4
Hence,
∠4 = 105°
To find ∠5:
Alternate Interior angles theorem:
The Alternate Interior Angles Theorem states that, when two parallel lines are cut by a transversal, the resulting alternate interior angles are congruent
So,
∠4 = ∠5
Hence,
∠5 = 105°
To find ∠8:
Verticle angle theorem:
Vertical Angles Theorem states that vertical angles, angles that are opposite each other and formed by two intersecting straight lines, are congruent
So,
∠5 = ∠8
Hence,
∠8 = 105°
Question 2.
Given m∠3 = 68° and m∠8 = (2x + 4)°, what is the value of x? Show your steps.
Answer:
It is given that
∠3 = 68° and ∠8 = (2x + 4)°
From the given figure,
We can observe that ∠3 and ∠8 are consecutive exterior angles.
So,
According to the consecutive exterior angles theorem,
If parallel lines are cut by a transversal line, then consecutive exterior angles are supplementary.
So,
∠3 + ∠8 = 180°
68° + (2x + 4)° = 180°
2x + 72° = 180°
2x° = 180° – 72°
2x° = 108°
x = \(\frac{108}{2}\)
x = 54°
Hence, from the above,
We can conclude that the value of x is: 54°
Question 3.
In the proof in Example 4, if you use the third statement before the second statement. could you still prove the theorem? Explain.
Answer:
In Example 4, the given theorem is “Alternate interior angle theorem”
If you even interchange the second and third statements, you could still prove the theorem as the second line before interchange is not necessary
Hence, from the above,
We can conclude that if you use the third statement before the second statement, you could still prove the theorem
Question 4.
WHAT IF?
In Example 5. yellow light leaves a drop at an angle of m∠2 = 41°. What is m∠1? How do you know?
Answer:
In Example 5,
If we observe ∠1 and ∠2, then they are alternate interior angles
Now,
According to Alternate interior angle theorem,
∠1 = ∠2
It is given that,
∠2 = 41°
So,
∠1 = 41°
Hence, from the above,
We can conclude that
∠1 = 41°
Exercise 3.2 Parallel Lines and Transversals
Vocabulary and Core Concept Check
Question 1.
WRITING
How are the Alternate Interior Angles Theorem (Theorem 3.2) and the Alternate Exterior
Angles Theorem (Theorem 3.3) alike? How are they different?
Answer:

Question 2.
WHICH ONE did DOESN’T BELONG?
Which pair of angle measures does not belong with the other three? Explain.
m∠1 and m∠3
m∠2 and m∠4
m∠2 and m∠3
m∠1 and m∠5
Answer:
The given figure is:

From the given figure,
∠1 and ∠3 are the vertical angles
∠2 and ∠4 are the alternate interior angles
∠2 and ∠3 are the consecutive interior angles
∠1 and ∠5 are the alternate exterior angles
So,
From the above,
We can observe that all the angles except ∠1 and ∠3 are the interior and exterior angles
Hence, from the above,
We can conclude that ∠1 and ∠3 pair does not belong with the other three
Monitoring Progress and Modeling with Mathematics
In Exercises 3-6, find m∠1 and m∠2. Tell which theorem you use in each case.
Question 3.
Answer:
Question 4.
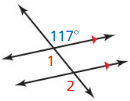
Answer:
The given figure is:

From the given figure,
We can observe that,
∠1 = ∠2 (By using the Vertical Angles theorem)
∠2 = 150° (By using the Alternate exterior angles theorem)
Hence, from the above,
We can conclude that
∠1 = ∠2 = 150°
Question 5.
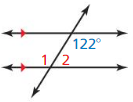
Answer:

Question 6.
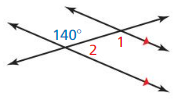
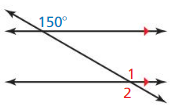
Answer:
The given figure is:

From the given figure,
We can observe that,
∠1 + ∠2 = 180° (By using the consecutive interior angles theorem)
∠2 = 140° (By using the Vertical angles theorem)
So,
∠1 = 180° – 140°
∠1 = 40°
Hence, from the above,
We can conclude that
∠1 = 40° and ∠2 = 140°
In Exercises 7-10. find the value of x. Show your steps.
Question 7.
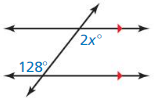
Answer:

Question 8.
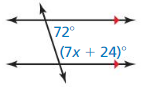
Answer:
The given figure is:

From the given figure,
We can observe that
72° + (7x + 24)° = 180° (By using the Consecutive interior angles theory)
(7x + 24)° = 180° – 72°
(7x + 24)° = 108°
7x° = 108° – 24°
7x° = 84°
x° = \(\frac{84}{7}\)
x° = 12°
Hence, from the above,
We can conclude that the value of x is: 12°
Question 9.
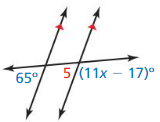
Answer:
Question 10.
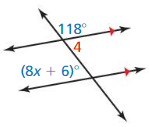
Answer:
The given figure is:

From the given figure,
We can observe that
(8x + 6)° = 118° (By using the Vertical Angles theorem)
8x° = 118° – 6°
8x° = 112°
x° = \(\frac{112}{8}\)
x° = 14°
Hence, from the above,
We can conclude that the value of x is: 14°
In Exercises 11 and 12. find m∠1, m∠2, and m∠3. Explain our reasoning.
Question 11.
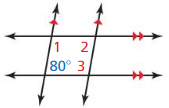
Answer:

Question 12.
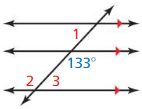
Answer:
The given figure is:

From the given figure,
We can observe that
∠3 + 133° = 180° (By using the Consecutive Interior angles theorem)
∠3 = 180° – 133°
∠3 = 47°
Now,
We can observe that
∠2 + ∠3 = 180°
∠2 = 180° – ∠3
∠2 = 180° – 47°
∠2 = 133°
Now,
We can observe that
∠1 = ∠2
Hence, from the above,
We can conclude that
∠1 = ∠2 = 133° and ∠3 = 47°
Question 13.
ERROR ANALYSIS
Describe and correct the error in the students reasoning
Answer:

Question 14.
HOW DO YOU SEE IT?
Use the diagram
a. Name two pairs of congruent angles when \(\overline{A D}\) and \(\overline{B C}\) are parallel? Explain your reasoning?
Answer:
Let the congruent angle be ∠P
So,
From the figure,
We can observe that the pair of angle when \(\overline{A D}\) and \(\overline{B C}\) are parallel is: ∠APB and ∠DPB
b. Name two pairs of supplementary angles when \(\overline{A B}\) and \(\overline{D C}\) are parallel. Explain your reasoning.
Answer:
From the given figure,
The two pairs of supplementary angles when \(\overline{A B}\) and \(\overline{D C}\) are parallel is: ∠ACD and ∠BDC
PROVING A THEOREM
In Exercises 15 and 16, prove the theorem.
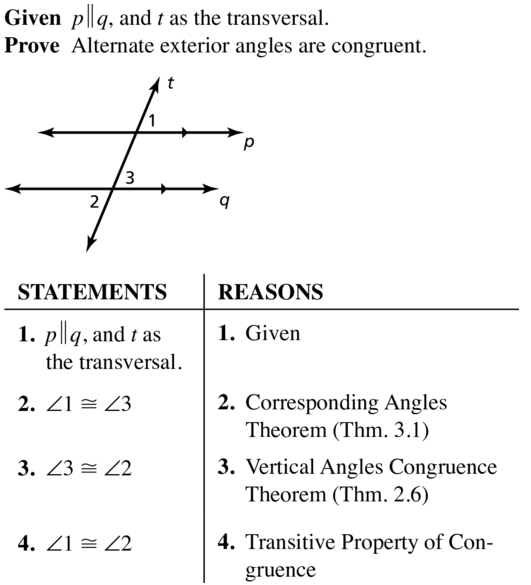
Question 15.
Alternate Exterior Angles Theorem (Thm. 3.3)
Answer:
Question 16.
Consecutive Interior Angles Theorem (Thm. 3.4)
Answer:
Statement of consecutive Interior angles theorem:
If two parallel lines are cut by a transversal, then the pairs of consecutive interior angles formed are supplementary
Proof:
Question 17.
PROBLEM-SOLVING
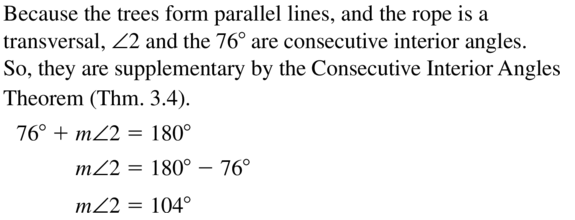
A group of campers ties up their food between two parallel trees, as shown. The rope is pulled taut. forming a straight line. Find m∠2. Explain our reasoning.
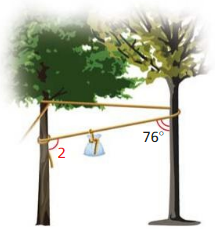
Answer:
Question 18.
DRAWING CONCLUSIONS
You are designing a box like the one shown.

a. The measure of ∠1 is 70°. Find m∠2 and m∠3.
b. Explain why ∠ABC is a straight angle.
c. If m∠1 is 60°, will ∠ABC still he a straight angle? Will the opening of the box be more steep or less steep? Explain.
Answer:
Question 19.
CRITICAL THINKING
Is it possible for consecutive interior angles to be congruent? Explain.
Answer:

Question 20.
THOUGHT-PROVOKING
The postulates and theorems in this book represent Euclidean geometry. In spherical geometry, all points are points on the surface of a sphere. A line is a circle on the sphere whose diameter is equal to the diameter of the sphere. In spherical geometry, is it possible that a transversal intersects two parallel lines? Explain your reasoning.
Answer:
We know that,
According to Euclidean geometry,
For a parallel line, there will be no intersecting point
But, In spherical geometry, even though there is some resemblance between circles and lines, there is no possibility to form parallel lines as the lines will intersect at least at 1 point on the circle which is called a tangent
Hence, from the above,
We can conclude that it is not possible that a transversal intersects two parallel lines
MATHEMATICAL CONNECTIONS
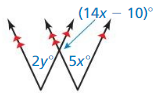
In Exercises 21 and 22, write and solve a system of linear equations to find the values of x and y.
Question 21.
Answer:
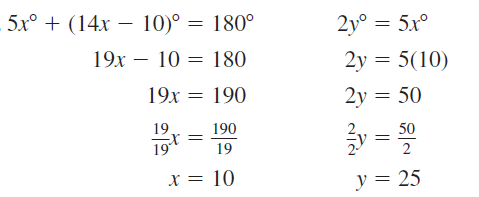
Question 22.
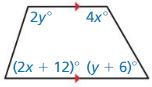
Answer:
The given figure is:

From the given figure,
We can observe that the given pairs of angles are consecutive interior angles
So,
2y° + 4x° = 180°
(2x + 12)° + (y + 6)° = 180°
2x° + y° + 18° = 180°
2x° + y° = 180° – 18°
2x° + y° = 162°———(1)
4x° + 2y° = 180°——–(2)
Solve eq. (1) and eq. (2) to get the values of x and y
2x° = 18°
x° = \(\frac{18}{2}\)
x° = 9°
Now,
y° = 162° – 2 (9°)
y° = 162° – 18°
y° = 144°
Hence, from the above,
We can conclude that the values of x and y are: 9° and 14° respectively
Question 23.
MAKING AN ARGUMENT
During a game of pool. your friend claims to be able to make the shot Shown in the diagram by hitting the cue ball so that m∠1 = 25°. Is your friend correct? Explain your reasoning.
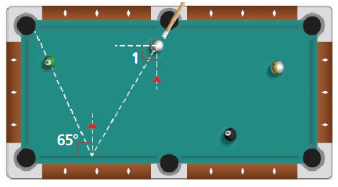
Answer:

Question 24.
REASONING
In diagram. ∠4 ≅∠5 and \(\overline{S E}\) bisects ∠RSF. Find m∠1. Explain your reasoning.
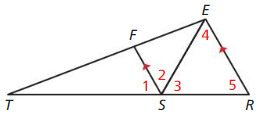
Answer:
It is given that ∠4 ≅∠5 and \(\overline{S E}\) bisects ∠RSF
So,
∠FSE = ∠ESR
From ΔESR,
We know that,
The sum of the angle measures of a triangle is: 180°
So,
∠3 + ∠4 + ∠5 = 180°
So,
∠3 = 60° (Since ∠4 ≅ ∠5 and the triangle is not a right triangle)
From the given figure,
We can observe that,
∠1 = ∠3 (By using the Corresponding angles theorem)
So,
∠1 = 60°
Hence, from the above,
We can conclude that ∠1 = 60°
Maintaining Mathematical Proficiency
Write the converse of the conditional statement. Decide whether it is true or false.
Question 25.
If two angles are vertical angles. then they are congruent.
Answer:
Question 26.
If you go to the zoo, then you will see a tiger.
Answer:
The given statement is:
If you go to the zoo, then you will see a tiger
The converse of the given statement is:
If you will see a tiger, then you go to the zoo———-> False
Question 27.
If two angles form a linear pair. then they are supplementary.
Answer:
Question 28.
If it is warm outside, then we will go to the park.
Answer:
The given statement is:
If it is warm outside, then we will go to the park
The converse of the given statement is:
If you will go to the park, then it is warm outside —————-> False
3.3 Proofs with Parallel Lines
Exploration 1
Exploring Converses
Work with a partner: Write the converse of each conditional statement. Draw a diagram to represent the converse. Determine whether the converse is true. Justify your conclusion.
CONSTRUCTING VIABLE ARGUMENTS
To be proficient in math, you need to make conjectures and build a logical progression of statements to explore the truth of your conjectures.
a. Corresponding Angles Theorem (Theorem 3.1): If two parallel lines are cut by a transversal, then the pairs of corresponding angles are congruent.
Converse:
If the pairs of corresponding angles are
congruent, then the two parallel lines are
cut by a transversal.
Answer:
The Converse of the Corresponding Angles Theorem:
The Converse of the Corresponding Angles Theorem says that if two lines and a transversal form congruent corresponding angles, then the lines are parallel.
So,
When we compare the converses we obtained from the given statement and the actual converse,
We can conclude that both converses are the same
Hence,
We can conclude that the converse we obtained from the given statement is true
Now,
Proof of Converse of Corresponding Angles Theorem:
Consider the 2 lines L1 and L2 intersected by a transversal line L3 creating 2 corresponding angles 1 and 2 which are congruent
We want to prove L1 and L2 are parallel and we will prove this by using “Proof of Contradiction”
Now,
According to Contradiction,
Assume L1 is not parallel to L2
Then, according to the parallel line axiom, there is a different line than L2 that passes through the intersection point of L2 and L3 (point A in the drawing), which is parallel to L1.
Let’s draw that line, and call it P. Let’s also call the angle formed by the traversal line and this new line angle 3, and we see that if we add some other angle, call it angle 4, to it, it will be the same as angle 2.
Now,
P || L1
So,
∠1 ≅ ∠3,
as corresponding angles formed by a transversal of parallel lines, and so,
m∠1=m∠3
This contradicts what was given, that angles 1 and 2 are congruent.
This contradiction means our assumption (“L1 is not parallel to L2”) is false, and so L1 must be parallel to L2.
The representation of the Converse of Corresponding Angles Theorem is:
b. Alternate Interior Angles Theorem (Theorem 3.2): If two parallel lines are cut by a transversal, then the pairs of alternate interior angles are congruent.
Converse:
If the pairs of alternate interior angles are
congruent, then the two parallel lines are
cut by a transversal.
Answer:
The converse of the Alternate Interior angles Theorem:
The “Converse of the Alternate Interior Angles Theorem” states that if two lines are cut by a transversal and the alternate interior angles are congruent, then the lines are parallel
So,
When we compare the actual converse and the converse according to the given statement,
we can conclude that the converse we obtained from the given statement is false
c. Alternate Exterior Angles Theorem (Theorem 3.3): If two parallel lines are cut by a transversal, then the pairs of alternate exterior angles are congruent.
Converse:
If the pairs of alternate exterior angles
are congruent, then the two parallel
lines are cut by a transversal.
Answer:
The Converse of the alternate exterior angles Theorem:
The “Converse of the Alternate Exterior Angles Theorem” states that if alternate exterior angles of two lines crossed by a transversal are congruent, then the two lines are parallel.
So,
When we compare the converses we obtained from the given statement and the actual converse,
We can conclude that both converses are the same
Hence,
We can conclude that the converse we obtained from the given statement is true
Now,
Proof of Alternate exterior angles Theorem:
Given: ∠1 ≅ ∠2
Prove: l || m
The flow proof for the Converse of Alternate exterior angles Theorem is:
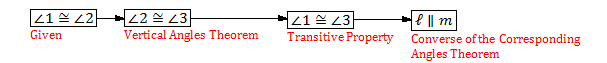
The representation of the Converse of the Exterior angles Theorem is:
d. Consecutive Interior Angles Theorem (Theorem 3.4): If two parallel lines are cut by a transversal. then the pairs of consecutive interior angles are supplementary.
Converse:
If the pairs of consecutive interior angles
are supplementary, then the two parallel lines
are cut by a transversal
Answer:
The Converse of the Consecutive Interior angles Theorem:
The “Converse of the consecutive Interior angles Theorem” states that if the consecutive interior angles on the same side of a transversal line intersecting two lines are supplementary, then the two lines are parallel.
So,
When we compare the converses we obtained from the given statement and the actual converse,
We can conclude that both converses are the same
Hence,
We can conclude that the converse we obtained from the given statement is true
Now,
Proof of the Converse of the Consecutive Interior angles Theorem:
a. m∠5 + m∠4 = 180° //From the given statement
b. m∠1 + m∠4 = 180° // Linear pair of angles are supplementary
c. m∠5=m∠1 // (1), (2), transitive property of equality
d. AB||CD // Converse of the Corresponding Angles Theorem
The representation of the Converse of the Consecutive Interior angles Theorem is:
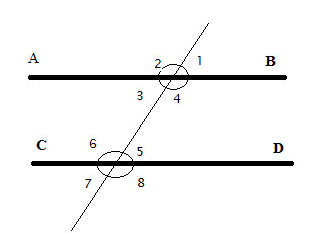
Communicate Your Answer
Question 2.
For which of the theorems involving parallel lines and transversals is the converse true?
Answer:
From Exploration 1,
The theorems involving parallel lines and transversals that the converse is true are:
a. Corresponding Angles Theorem
b. Alternate Exterior angles Theorem
c. Consecutive Interior angles Theorem
Question 3.
In Exploration 1, explain how you would prove any of the theorems that you found to be true.
Answer:
For the proofs of the theorems that you found to be true, refer to Exploration 1
Lesson 3.3 Proofs with Parallel Lines
Monitoring Progress
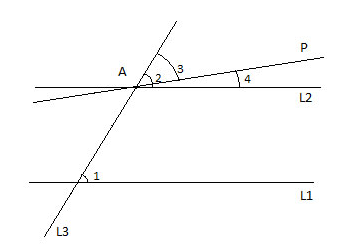
Question 1.
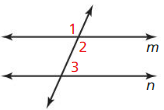
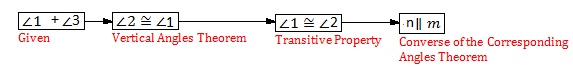
Is there enough information in the diagram to conclude that m || n? Explain.
Answer:
Yes, there is enough information in the diagram to conclude m || n.
Explanation:
The given figure is:

From the given figure,
We can observe that the given angles are the consecutive exterior angles
Now,
We have to prove that m || n
So,
We will use “Converse of Consecutive Exterior angles Theorem” to prove m || n
Proof of the Converse of the Consecutive Exterior angles Theorem:
a. m∠1 + m∠8 = 180° //From the given statement
b. m∠1 + m∠4 = 180° // Linear pair of angles are supplementary
c. m∠5=m∠1 // (1), (2), transitive property of equality
d. AB||CD // Converse of the Corresponding Angles Theorem
The representation of the Converse of the Consecutive Interior angles Theorem is:
Question 2.
Explain why the Corresponding Angles Converse is the converse of the Corresponding Angles Theorem (Theorem 3.1).
Answer:
Corresponding Angles Theorem:
The “Corresponding Angles Postulate” states that, when two parallel lines are cut by a transversal, the resulting corresponding angles are congruent
Converse:
When the corresponding angles are congruent, the two parallel lines are cut by a transversal
Now,
The Converse of Corresponding Angles Theorem:
If the corresponding angles formed are congruent, then two lines l and m are cut by a transversal.
So,
When we observe the Converse of the Corresponding Angles Theorem we obtained and the actual definition, both are the same
Hence, from the above,
We can conclude that the Corresponding Angles Converse is the converse of the Corresponding Angles Theorem
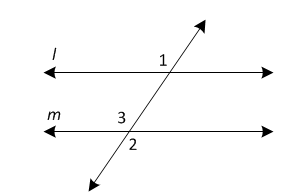
Question 3.
If you use the diagram below to prove the Alternate Exterior Angles Converse. what Given and Prove statements would you use?
Answer:
The given figure is:

It is given that the given angles are the alternate exterior angles
Now,
Alternate Exterior angle Theorem:
If two parallel lines are cut by a transversal, then the pairs of alternate exterior angles are congruent
The Converse of the Alternate Exterior Angles Theorem:
The “Converse of the Alternate Exterior Angles Theorem” states that if alternate exterior angles of two lines crossed by a transversal are congruent, then the two lines are parallel.
Hence, from the above,
We can conclude that
For the Converse of the alternate exterior angles Theorem,
The given statement is: ∠1 ≅ 8
To prove: l || k
Question 4.
Copy and complete the following paragraph proof of the Alternate Interior Angles Converse using the diagram in Example 2.
It is given that ∠4 ≅∠5. By the _______ . ∠1 ≅ ∠4. Then by the Transitive Property of Congruence (Theorem 2.2), _______ . So, by the _______ , g || h.
Answer:
The completed proof of the Alternate Interior Angles Converse using the diagram in Example 2 is:
It is given that ∠4 ≅∠5.
By the Vertical Angles Congruence Theorem (Theorem 2.6). ∠1 ≅ ∠4.
Then by the Transitive Property of Congruence (Theorem 2.2), ∠1 ≅∠5.
So, by the Corresponding Angles Converse, g || h.
Question 5.
Each step is parallel to the step immediately above it. The bottom step is parallel to the ground. Explain why the top step is parallel t0 the ground.

Answer:
From the given figure,
We can observe that not any step is intersecting at each other
In the same way, when we observe the floor from any step,
We can say that they are also parallel
Hence, from the above,
We can conclude that the top step is also parallel to the ground since they do not intersect each other at any point
Question 6.
In the diagram below. p || q and q || r. Find m∠8. Explain your reasoning.
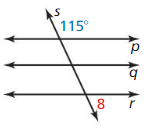
Answer:
The given figure is:

From the figure,
We can observe that the given angles are the consecutive exterior angles
We know that,
According to the Consecutive Exterior angles Theorem,
∠8 + 115° = 180°
∠8 = 180° – 115°
∠8 = 65°
Hence, from the above,
We can conclude that
∠8 = 65°
Exercise 3.3 Proofs with Parallel Lines
Vocabulary and Core Concept Check
Question 1.
VOCABULARY
Two lines are cut by a transversal. Which angle pairs must be congruent for the lines to be parallel?
Answer:

Question 2.
WRITING
Use the theorems from Section 3.2 and the converses of those theorems in this section to write three biconditional statements about parallel lines and transversals.
Answer:
Corresponding Angles theorem states that if two lines are cut by a transversal, then the pairs of corresponding angles are congruent.
Corresponding Angles converse states that if two lines are cut by a transversal so the corresponding angles are congruent, then the lines are parallel
Biconditional statement is Two lines, which are cut by a transversal, are parallel if and only if the corresponding angles are congruent.
Alternate interior angles theorem states that if two parallel lines are cut by a transversal, then the pairs of alternate interior angles are congruent.
Alternate interior angles converse states that if two lines are cut by a transversal so the alternate interior angles are congruent, then the lines are parallel
Biconditional statement is Two lines, which are cut by a transversal, are parallel if and only if the alternate interior angle are congruent.
Alternate exterior angles theorem states that if two parallel lines are cut by a transversal, then the pairs of alternate exterior angles are congruent.
Alternate exterior angles converse states that if two lines are cut by a transversal so the alternate exterior angles are congruent, then the lines are parallel
A Biconditional statement is Two lines, which are cut by a transversal, are parallel if and only if the alternate exterior angle are congruent.
Monitoring Progress and Modeling with Mathematics
In Exercises 3-8. find the value of x that makes m || n. Explain your reasoning.
Question 3.
Answer:

Question 4.
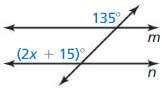
Answer:
The given figure is:

From the given figure,
We can observe that the given angles are the corresponding angles
Now,
According to Corresponding Angles Theorem,
(2x + 15)° = 135°
2x° = 135° – 15°
2x° = 120°
x° = \(\frac{120}{2}\)
x° = 60°
Hence, from the above,
We can conclude that the value of x is: 60°
Question 5.
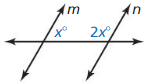
Answer:

Question 6.
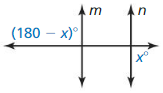
Answer:
The given figure is:

From the given figure,
We can observe that the given angles are the corresponding angles
Now,
According to Corresponding Angles Theorem,
(180 – x)° = x°
180° = x° + x°
2x° = 180°
x° = \(\frac{180}{2}\)
x° = 90°
Hence, from the above,
We can conclude that the value of x is: 90°
Question 7.
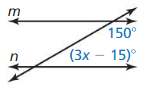
Answer:

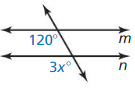
Question 8.
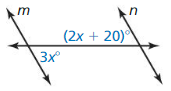
Answer:
The given figure is:

From the given figure,
We can observe that the given angles are the corresponding angles
Now,
According to Corresponding Angles Theorem,
(2x + 20)° = 3x°
20° = 3x° – 2x°
x° = 20°
Hence, from the above,
We can conclude that the value of x is: 20°
In Exercises 9 and 10, use a compass and straightedge to construct a line through point P that is parallel to line m.
Question 9.
Answer:
Question 10.
Answer:
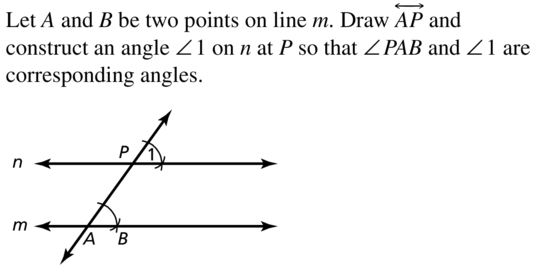
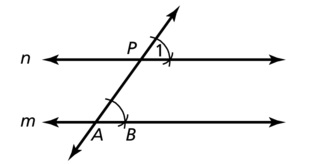
Let A and B be two points on line m.
Draw \(\overline{A P}\) and construct an angle ∠1 on n at P so that ∠PAB and ∠1 are corresponding angles
Hence,
The representation of the complete figure is:
PROVING A THEOREM
In Exercises 11 and 12. prove the theorem.
Question 11.
Alternate Exterior Angles Converse (Theorem 3.7)
Answer:
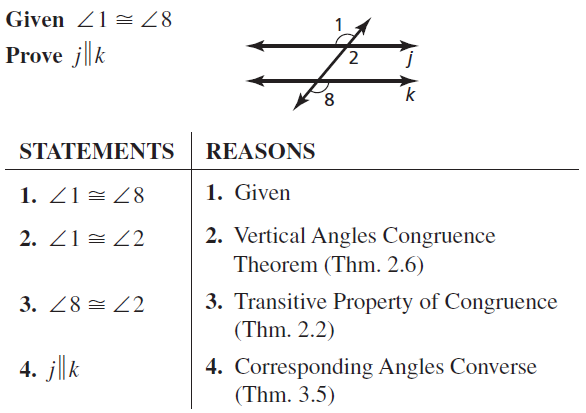
Question 12.
Consecutive Interior Angles Converse (Theorem 3.8)
Answer:
Proof of the Converse of the Consecutive Interior angles Theorem:
Given: m∠5 + m∠4 = 180°
Prove: AB || CD

Now,
a. m∠5 + m∠4 = 180° //From the given statement
b. m∠1 + m∠4 = 180° // Linear pair of angles are supplementary
c. m∠5=m∠1 // (1), (2), transitive property of equality
d. AB||CD // Converse of the Corresponding Angles Theorem
In Exercises 13-18. decide whether there is enough information to prove that m || n. If so, state the theorem you would use.
Question 13.
Answer:
![]()
Question 14.
Answer:
Yes, there is enough information to prove m || n
The theorem we can use to prove that m || n is: Alternate Exterior angles Converse theorem
Question 15.
Answer:
![]()
Question 16.
Answer:
No, there is no enough information to prove m || n
Question 17.
Answer:
![]()
Question 18.
Answer:
Yes, there is enough information to prove m || n
The theorem we can use to prove that m || n is: Alternate Exterior angles Converse theorem
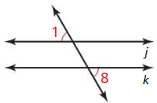
ERROR ANALYSIS
In Exercises 19 and 20, describe and correct the error in the reasoning.
Question 19.
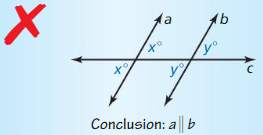
Answer:

Question 20.
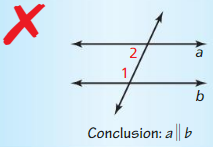
Answer:
The given figure shows that angles 1 and 2 are Consecutive Interior angles
It also shows that a and b are cut by a transversal and they have the same length
So,
From the converse of the Consecutive Interior angles Theorem,
We can conclude that a || b
In Exercises 21-24. are ![]() and
and ![]() parallel? Explain your reasoning.
parallel? Explain your reasoning.
Question 21.
Answer:

Question 22.
Answer:
The given figure is:

From the given figure,
We can observe that
The sum of the given angle measures is: 180°
From the given figure,
We can observe that the given angles are consecutive exterior angles
So,
From the Consecutive Exterior angles Converse,
We can conclude that AC || DF
Question 23.
Answer:

Question 24.
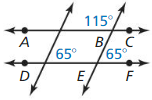
Answer:
The given figure is:

From the given figure,
We can observe that the sum of the angle measures of all the pairs i.e., (115 + 65)°, (115 + 65)°, and (65 + 65)° is not 180°
Since,
The sum of the angle measures are not supplementary, according to the Consecutive Exterior Angles Converse,
AC is not parallel to DF
Question 25.
ANALYZING RELATIONSHIPS
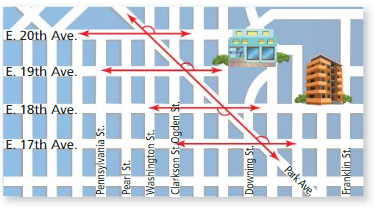
The map shows part of Denser, Colorado, Use the markings on the map. Are the numbered streets parallel to one another? Explain your reasoning.
Answer:

Question 26.
ANALYZING RELATIONSHIPS
Each rung of the ladder is parallel to the rung directly above it. Explain why the top rung is parallel to the bottom rung.

Answer:
When we observe the ladder,
The rungs are not intersecting at any point i.e., they have different points
We know that,
The parallel lines do not have any intersecting points
Hence, from the above,
We can conclude that the top rung is parallel to the bottom rung
Question 27.
MODELING WITH MATHEMATICS
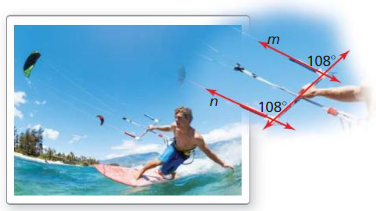
The diagram of the control bar of the kite shows the angles formed between the Control bar and the kite lines. How do you know that n is parallel to m?
Answer:

Question 28.
REASONING
Use the diagram. Which rays are parallel? Which rays are not parallel? Explain your reasoning.
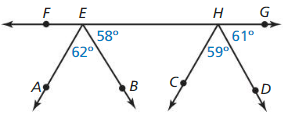
Answer:
m∠1 + m∠2
61 + 59 = 120 degrees
m∠3 = 180 – (m∠1 + m∠2)
m∠3 = 180 – 120
m∠3 = 60
m∠4 + m∠5
58 + 62 = 120
m∠6 = 180 – (m∠4 + m∠5)
m∠6 = 180 – 120
m∠6 = 60
m∠3 = m∠6, this shows the corresponding angle between the lines AE and CH.
EB is not parallel to line HD.
Question 29.
ATTENDING TO PRECISION
Use the diagram. Which theorems allow you to conclude that m || n? Select all that apply. Explain your reasoning.
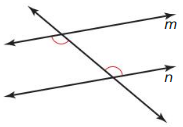
(A) Corresponding Angles Converse (Thm 3.5)
(B) Alternate Interior Angles Converse (Thm 3.6)
(C) Alternate Exterior Angles Converse (Thm 3.7)
(D) Consecutive Interior Angles Converse (Thm 3.8)
Answer:
Question 30.
MODELING WITH MATHEMATICS
One way to build stairs is to attach triangular blocks to angled support, as shown. The sides of the angled support are parallel. If the support makes a 32° angle with the floor, what must m∠1 so the top of the step will be parallel to the floor? Explain your reasoning.
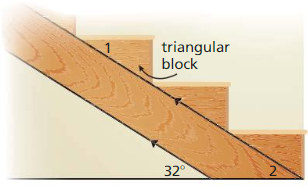
Answer:
It is given that the sides of the angled support are parallel and the support makes a 32° angle with the floor
So,
To make the top of the step where ∠1 is present to be parallel to the floor, the angles must be “Alternate Interior angles”
We know that,
The “Alternate Interior angles” are congruent
So,
∠1 = 32°
Hence, from the above,
We can conclude that
∠1 = 32°
Question 31.
ABSTRACT REASONING
In the diagram, how many angles must be given to determine whether j || k? Give four examples that would allow you to conclude that j || k using the theorems from this lesson.
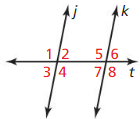
Answer:

Question 32.
THOUGHT-PROVOKING
Draw a diagram of at least two lines cut by at least one transversal. Mark your diagram so that it cannot be proven that any lines are parallel. Then explain how your diagram would need to change in order to prove that lines are parallel.
Answer:
The diagram that represents the figure that it can not be proven that any lines are parallel is:
From the above,
The diagram can be changed by the transformation of transversals into parallel lines and a parallel line into transversal
Hence,
The diagram that represents the figure that it can be proven that the lines are parallel is:
PROOF
In Exercises 33-36, write a proof.
Question 33.
Given m∠1 = 115°, m∠2 = 65°
Prove m||n
Answer:
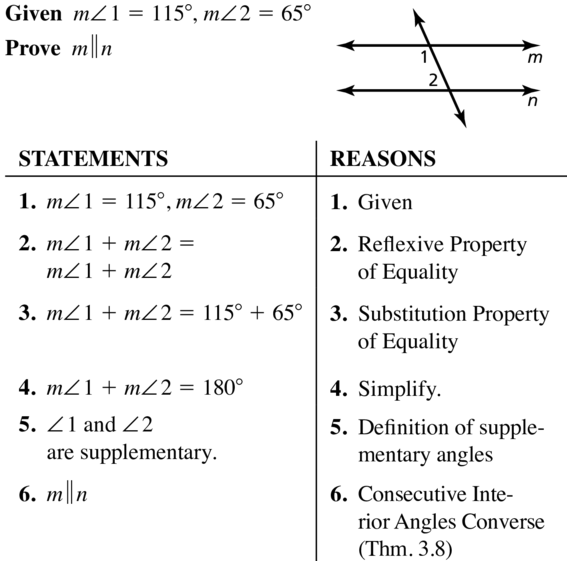
Question 34.
Given ∠1 and ∠3 are supplementary.
Prove m||n
Answer:
Given: ∠1 and ∠3 are supplementary
Prove: m || n
Hence,
Question 35.
Given ∠1 ≅ ∠2, ∠3 ≅ ∠4
Prove \(\overline{A B} \| \overline{C D}\)
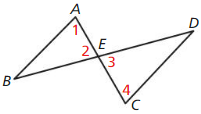
Answer:
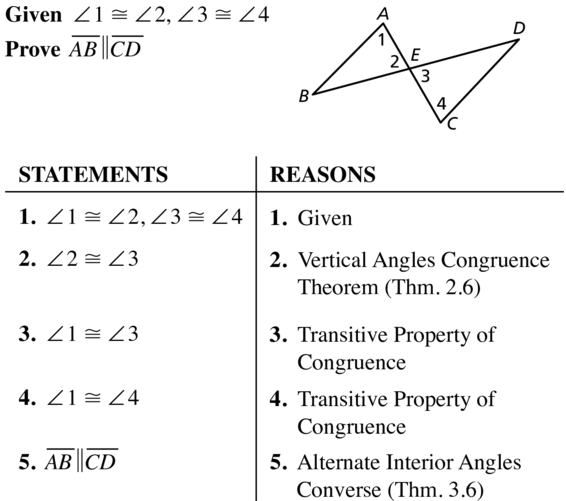
Question 36.
Given a||b, ∠2 ≅ ∠3
Prove c||d
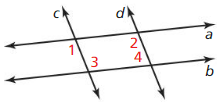
Answer:
Given: a || b, ∠2 ≅ ∠3
Prove: c || d
Hence,
Question 37.
MAKING AN ARGUMENT
Your classmate decided that ![]() based on the diagram. Is your classmate correct? Explain your reasoning.
based on the diagram. Is your classmate correct? Explain your reasoning.
Answer:
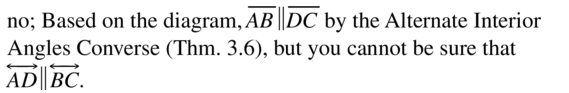
Question 38.
HOW DO YOU SEE IT?
Are the markings on the diagram enough to conclude that any lines are parallel? If so. which ones? If not, what other information is needed?
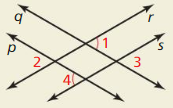
Answer:
The given diagram is:

From the given diagram,
We can observe that
∠1 and ∠4; ∠2 and ∠3 are the pairs of corresponding angles
We know that,
According to the Converse of the Corresponding angles Theorem,
If the corresponding angles are congruent, then the two lines that cut by a transversal are parallel lines
Hence,
We can conclude that p and q; r and s are the pairs of parallel lines
Question 39.
PROVING A THEOREM
Use these steps to prove the Transitive Property of Parallel Lines Theorem
a. Cops the diagram with the Transitive Property of Parallel Lines Theorem on page 141.
b. Write the Given and Prove statements.
c. Use the properties of angles formed by parallel lines cut by a transversal to prove the theorem.
Answer:
Question 40.
MATHEMATICAL CONNECTIONS
Use the diagram
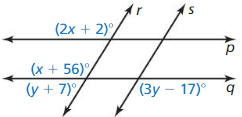
a. Find the value of x that makes p || q.
Answer:
From the given figure,
We can observe that when p || q,
The angles are: (2x + 2)° and (x + 56)°
We can observe that the given angles are corresponding angles
Hence,
(2x + 2)° = (x + 56)°
2x – x = 56° – 2°
x° = 54°
Hence, from the above,
We can conclude that the value of x when p || q is: 54°
b. Find the value of y that makes r || s.
Answer:
We can observe that when r || s,
The angles are (y + 7)° and (3y – 17)°
We can observe that the given angles are corresponding angles
Hence,
(y + 7)° = (3y – 17)°
y – 3y = -17° – 7°
-2y° = -24°
y = \(\frac{24}{2}\)
y = 12°
Hence, from the above,
We can conclude that the value of y when r || s is: 12°
c. Can r be parallel to s and can p, be parallel to q at the same time? Explain your reasoning.
Answer:
No, p ||q and r ||s will not be possible at the same time because when p || q, r, and s can act as transversal and when r || s, p, and q can act as transversal
Maintaining Mathematical Proficiency
Use the Distance Formula to find the distance between the two points.
Question 41.
(1, 3) and (- 2, 9)
Answer:
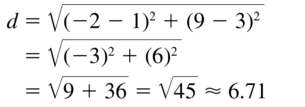
Question 42.
(- 3, 7) and (8, – 6)
Answer:
The given points are:
(-3, 7), and (8, -6)
Compare the given points with (x1, y1), and (x2, y2)
We know that,
d = \(\sqrt{(x2 – x1)² + (y2 – y1)²}\)
d = \(\sqrt{(8 + 3)² + (7 + 6)²}\)
d = \(\sqrt{(11)² + (13)²}\)
d = \(\sqrt{290}\)
d = 17.02
Hence, from the above,
We can conclude that the distance between the given 2 points is: 17.02
Question 43.
(5, – 4) and (0, 8)
Answer:

Question 44.
(13, 1) and (9, – 4)
Answer:
The given points are:
(13, 1), and (9, -4)
Compare the given points with (x1, y1), and (x2, y2)
We know that,
d = \(\sqrt{(x2 – x1)² + (y2 – y1)²}\)
d = \(\sqrt{(13 – 9)² + (1 + 4)²}\)
d = \(\sqrt{(4)² + (5)²}\)
d = \(\sqrt{41}\)
d = 6.40
Hence, from the above,
We can conclude that the distance between the given 2 points is: 6.40
3.1 – 3.3 Study Skills: Analyzing Your Errors
Mathematical Practices
Question 1.
Draw the portion of the diagram that you used to answer Exercise 26 on page 130.
Answer:
The portion of the diagram that you used to answer Exercise 26 on page 130 is:

Question 2.
In Exercise 40 on page 144. explain how you started solving the problem and why you started that way.
Answer:
In Exercise 40 on page 144,
You started solving the problem by considering the 2 lines parallel and two lines as transversals
So,
If p and q are the parallel lines, then r and s are the transversals
If r and s are the parallel lines, then p and q are the transversals
3.1 – 3.3 Quiz
Think of each segment in the diagram as part of a line. Which lines(s) or plane(s) contain point G and appear to fit the description?
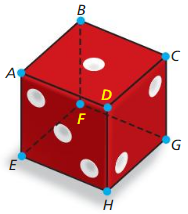
Question 1.
line(s) parallel to ![]() .
.
Answer:
The line parallel to \(\overline{E F}\) is: \(\overline{D H}\)
Question 2.
line(s) perpendicular to ![]() .
.
Answer:
The lines perpendicular to \(\overline{E F}\) are: \(\overline{F B}\) and \(\overline{F G}\)
Question 3.
line(s) skew to ![]() .
.
Answer:
The lines skew to \(\overline{E F}\) are: \(\overline{C D}\), \(\overline{C G}\), and \(\overline{A E}\)
Question 4.
plane(s) parallel to plane ADE
Answer:
The plane parallel to plane ADE is: Plane GCB
Identify all pairs of angles of the given type.
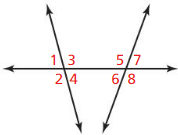
Question 5.
consecutive interior
Answer:
We know that,
When two lines are cut by a transversal, the pair of angles on one side of the transversal and inside the two lines are called the consecutive interior angles.
Hence, from the given figure,
We can conclude that the consecutive interior angles are: 3 and 5; 4 and 6
Question 6.
alternate interior
Answer:
We know that,
Alternate Interior Angles are a pair of angles on the inner side of each of those two lines but on opposite sides of the transversal.
Hence, from the given figure,
We can conclude that the alternate interior angles are: 3 and 6; 4 and 5
Question 7.
corresponding
Answer:
We know that,
When two lines are crossed by another line (which is called the Transversal), the angles in matching corners are called corresponding angles.
Hence, from the given figure,
We can conclude that the corresponding angles are: 1 and 5; 3 and 7; 2 and 4; 6 and 8
Question 8.
alternate exterior
Answer:
Alternate exterior angles are the pair of angles that lie on the outer side of the two parallel lines but on either side of the transversal line
Hence, from the above figure,
We can conclude that the alternate exterior angles are: 1 and 8; 7 and 2
Find m∠1 and m∠2. Tell which theorem you use in each case.
Question 9.
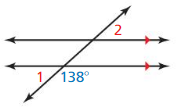
Answer:
The given figure is:

From the given figure,
By using the linear pair theorem,
∠1 + 138° = 180°
∠1 = 180° – 138°
∠1 = 42°
Now,
By using the Alternate Exterior Angles Theorem,
∠1 = ∠2
Hence, from the above,
We can conclude that
∠1 = ∠2 = 42°
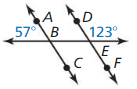
Question 10.
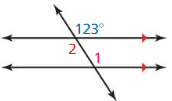
Answer:
The given figure is:

From the given figure,
We can observe that
By using the Vertical Angles Theorem,
∠2 = 123°
Now,
By using the vertical Angles Theorem,
∠1 = ∠2
Hence, from the above,
We can conclude that
∠1 = ∠2 = 123°
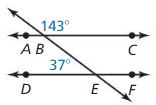
Question 11.
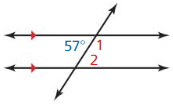
Answer:
The given figure is:

From the given figure,
By using the linear pair theorem,
∠1 + 57° = 180°
∠1 = 180° – 57°
∠1 = 123°
Now,
By using the consecutive interior angles theorem,
∠1 + ∠2 = 180°
∠2 = 180° – 123°
∠2 = 57°
Hence, from the above,
We can conclude that
∠1 = 123° and ∠2 = 57°
Decide whether there is enough information to prove that m || n. If so, state the theorem you would use.
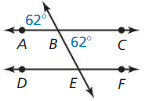
Question 12.
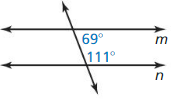
Answer:
The given figure is:

We know that,
By using the “Consecutive Interior angles Converse”,
If the angle measure of the angles is a supplementary angle, then the lines cut by a transversal are parallel
Now,
69° + 111° = 180°
Hence, from the above,
We can conclude that m || n by using the Consecutive Interior angles Theorem
Question 13.
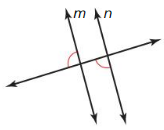
Answer:
The given figure is:

We know that,
By using the Corresponding Angles Theorem,
If the corresponding angles are congruent, then the lines cut by a transversal are parallel
Hence, from the above,
We can conclude that m || n by using the Corresponding Angles Theorem
Question 14.
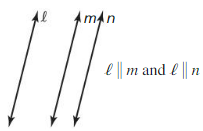
Answer:
The given figure is:

From the given figure,
It is given that l || m and l || n,
So,
We know that,
By using the parallel lines property,
If a || b and b || c, then a || c
Hence, from the above,
We can conclude that m || n
Question 15.
Cellular phones use bars like the ones shown to indicate how much signal strength a phone receives from the nearest service tower. Each bar is parallel to the bar directly next to it.
a. Explain why the tallest bar is parallel to the shortest bar.
Answer:
From the given bars,
We can observe that there is no intersection between any bars
If we represent the bars in the coordinate plane, we can observe that the number of intersection points between any bar is: 0
We know that,
The number of intersection points for parallel lines is: 0
Hence, from the above,
We can conclude that the tallest bar is parallel to the shortest bar
b. Imagine that the left side of each bar extends infinitely as a line.
If m∠1 = 58°, then what is m∠2?
Answer:
From the given figure,
We can observe that ∠1 and ∠2 are the consecutive interior angles
We know that,
The sum of the angle measure between 2 consecutive interior angles is: 180°
So,
∠1 + ∠ 2 = 180°
It is given that ∠1 = 58°
So,
∠2 = 180° – 58°
∠2 = 122°
Hence, from the above,
We can conclude that
∠2 = 122°
Question 16.
The diagram shows lines formed on a tennis court.
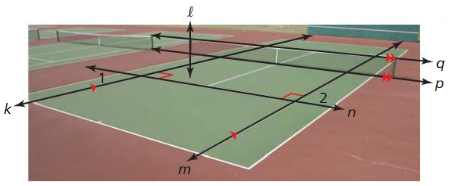
a. Identify two pairs of parallel lines so that each pair is in a different plane.
Answer:
From the given figure,
We can observe that there are a total of 5 lines.
Hence,
The two pairs of parallel lines so that each pair is in a different plane are: q and p; k and m
b. Identify two pairs of perpendicular lines.
Answer:
Fro the given figure,
We can observe that there are 2 perpendicular lines
Hence, from the above,
The two pairs of perpendicular lines are l and n
c. Identify two pairs of skew line
Answer:
From the given figure,
We can observe that there are 2 pairs of skew lines
Hence,
The 2 pair of skew lines are: q and p; l and m
d. Prove that ∠1 ≅ ∠2.
Answer:
From the given figure,
We can observe that ∠1 and ∠2 are the alternate exterior angles
We know that,
According to the Alternate Exterior angles Theorem,
If the line cut by a transversal is parallel, then the corresponding angles are congruent
Hence,
According to the above theorem,
We can conclude that ∠1 ≅ ∠2
3.4 Proofs with Perpendicular Lines
Exploration 1
Writing Conjectures
Work with a partner: Fold a piece of pair in half twice. Label points on the two creases. as shown.
a. Write a conjecture about \(\overline{A B}\) and \(\overline{C D}\). Justify your conjecture.
Answer:
The conjecture about \(\overline{A B}\) and \(\overline{c D}\) is:
If two lines intersect to form a linear pair of congruent angles, then the lines are perpendicular.
b. Write a conjecture about \(\overline{A O}\) and \(\overline{O B}\) Justify your conjecture.
Answer:
The conjecture about \(\overline{A O}\) and \(\overline{O B}\) is:
In a plane, if two lines are perpendicular to the same line, then they are parallel to each other.
Exploration 2
Exploring a segment Bisector
Work with a partner: Fold and crease a piece of paper. as shown. Label the ends of the crease as A and B.

a. Fold the paper again so that point A coincides with point B. Crease the paper on that fold.
Answer:

b. Unfold the paper and examine the four angles formed by the two creases. What can you conclude about the four angles?
Answer:
When we unfold the paper and examine the four angles formed by the two creases, we can conclude that the four angles formed are the right angles i.e., 90°
Exploration 3
Writing a conjecture
Work with a partner.
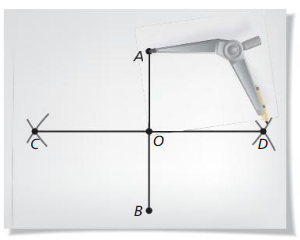
a. Draw \(\overline{A B}\), as shown.
b. Draw an arc with center A on each side of AB. Using the same compass selling, draw an arc with center B on each side \(\overline{A B}\). Label the intersections of arcs C and D.
c. Draw \(\overline{C D}\). Label its intersection with \(\overline{A B}\) as O. Write a conjecture about the resulting diagram. Justify your conjecture.
CONSTRUCTING VIABLE ARGUMENTS
To be proficient in math, you need to make conjectures and build a logical progression of statements to explore the truth of your conjectures.
Answer:
The resultant diagram is:
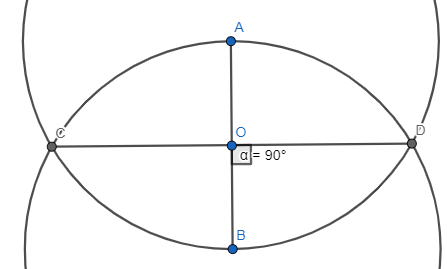
From the above diagram,
We can conclude that
The angles formed at all the intersection points are: 90°
The lengths of the line segments are equal i.e., AO = OB and CO = OD
Communicate Your Answer
Question 4.
What conjectures can you make about perpendicular lines?
Answer:
The conjectures about perpendicular lines are:
a. If two lines intersect to form a linear pair of congruent angles, then the lines are perpendicular.
b. In a plane, if a transversal is perpendicular to one of two parallel lines, then it is perpendicular to the other line. c. In a plane, if two lines are perpendicular to the same line, then they are parallel to each other.
Question 5.
In Exploration 3. find AO and OB when AB = 4 units.
Answer:
From Exploration 2,
We can observe that,
AB = AO + OB
AO = OB
It is given that
AB = 4 units
Hence,
The values of AO and OB are: 2 units
Lesson 3.4 Proofs with Perpendicular Lines
Monitoring Progress
Question 1.
Find the distance from point E to ![]()
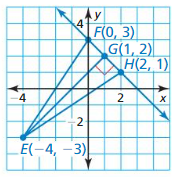
Answer:
The given figure is:

It is given that E is ⊥ to \(\overline{F H}\)
So,
To find the distance between E and \(\overline{F H}\), we need to find the distance between E and G i.e., EG
Now,
From the coordinate plane,
E (-4, -3), G (1, 2)
Compare the given points with
E (x1, y1), G (x2, y2)
So,
EG = \(\sqrt{(x2 – x1)² + (y2 – y1)²}\)
EG = \(\sqrt{(1 + 4)² + (2 + 3)²}\)
EG = \(\sqrt{(5)² + (5)²}\)
EG = \(\sqrt{50}\)
EG = 7.07
Hence, from the above,
We can conclude that the distance from point E to \(\overline{F H}\) is: 7.07
Question 2.
Prove the Perpendicular Transversal Theorem using the diagram in Example 2 and the Alternate Exterior Angles Theorem (Theorem 3.3).
Answer:
Perpendicular transversal theorem:
In a plane, if a line is perpendicular to one of the two parallel lines, then it is perpendicular to the other line also
Proof:
Given: k || l, t ⊥ k
Prove: t ⊥ l
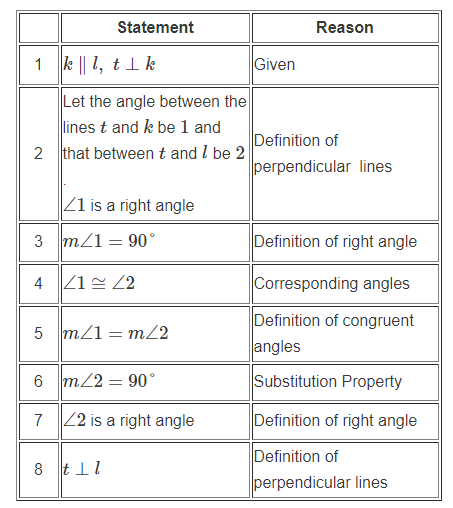
Alternate Exterior Angles Theorem:
The Alternate Exterior Angles Theorem states that, when two parallel lines are cut by a transversal, the resulting alternate exterior angles are congruent
Proof:
Given: k || l
Prove: ∠1 ≅ ∠7 and ∠4 ≅ ∠6
Since k || l, by the Corresponding Angles Postulate,
∠1 ≅ ∠5
Also, by the Vertical Angles Theorem,
∠5 ≅ ∠7
Then, by the Transitive Property of Congruence,
∠1 ≅ ∠7
You can prove that ∠4 and ∠6 are congruent using the same method.
Use the lines marked in the photo.

Question 3.
Is b || a? Explain your reasoning.
Answer:
From the given figure,
There is not any intersection between a and b
Hence, from the above,
We can conclude that b || a
Question 4.
Is b ⊥ c? Explain your reasoning.
Answer:
From the given figure,
We can observe that the angle between b and c is 90°
Hence, from the above,
We can conclude that b is perpendicular to c
Exercise 3.4 Proofs with Perpendicular Lines
Vocabulary and core Concept Check
Question 1.
COMPLETE THE SENTENCE
The perpendicular bisector of a segment is the line that passes through the _______________ of the segment at a _______________ angle.
Answer:
Question 2.
DIFFERENT WORDS, SAME QUESTION
Which is different? Find “both” answers.
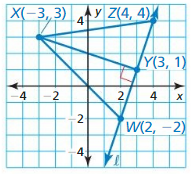
Find the distance from point X to ![]()
Answer:
The given figure is:

To find the distance from point X to \(\overline{W Z}\),
We have to find the distance between X and Y i.e., XY
Now,
The given points are:
X (-3, 3), Y (3, 1)
Compare the given points with
(x1, y1), (x2, y2)
Now,
XY = \(\sqrt{(x2 – x1)² + (y2 – y1)²}\)
XY = \(\sqrt{(3 + 3)² + (3 – 1)²}\)
XY = \(\sqrt{(6)² + (2)²}\)
XY = 6.32
Hence, from the above,
We can conclude that the distance from point X to \(\overline{W Z}\) is: 6.32
Find XZ
Answer:
The given points are:
X (-3, 3), Z (4, 4)
Compare the given points with
(x1, y1), (x2, y2)
Now,
XZ = \(\sqrt{(x2 – x1)² + (y2 – y1)²}\)
XZ = \(\sqrt{(4 + 3)² + (3 – 4)²}\)
XZ = \(\sqrt{(7)² + (1)²}\)
XZ = 7.07
Hence, from the above,
We can conclude that the value of XZ is: 7.07
Find the length of \(\overline{X Y}\)
Answer:
The given points are:
X (-3, 3), Y (3, 1)
Compare the given points with
(x1, y1), (x2, y2)
Now,
XY = \(\sqrt{(x2 – x1)² + (y2 – y1)²}\)
XY = \(\sqrt{(3 + 3)² + (3 – 1)²}\)
XY = \(\sqrt{(6)² + (2)²}\)
XY = 6.32
Hence, from the above,
We can conclude that the value of XY is: 6.32
Find the distance from line l to point X.
Answer:
To find the distance from line l to point X,
We have to find the distance between X and Y i.e., XY
Now,
The given points are:
X (-3, 3), Y (3, 1)
Compare the given points with
(x1, y1), (x2, y2)
Now,
XY = \(\sqrt{(x2 – x1)² + (y2 – y1)²}\)
XY = \(\sqrt{(3 + 3)² + (3 – 1)²}\)
XY = \(\sqrt{(6)² + (2)²}\)
XY = 6.32
Hence, from the above,
We can conclude that the distance from line l to point X is: 6.32
Monitoring Progress and Modeling with Mathematics
In Exercises 3 and 4. find the distance from point A to ![]() .
.
Question 3.
Answer:
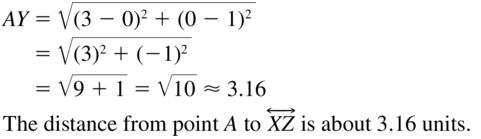
Question 4.
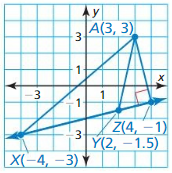
Answer:
To find the distance from point A to \(\overline{X Z}\),
We have to find the distance between A and Y i.e., AY
Now,
The given points are:
X (3, 3), Y (2, -1.5)
Compare the given points with
(x1, y1), (x2, y2)
Now,
XY = \(\sqrt{(x2 – x1)² + (y2 – y1)²}\)
XY = \(\sqrt{(3 + 1.5)² + (3 – 2)²}\)
XY = \(\sqrt{(4.5)² + (1)²}\)
XY = 4.60
Hence, from the above,
We can conclude that the distance from point A to \(\overline{X Z}\) is: 4.60
CONSTRUCTION
In Exercises 5-8, trace line m and point P. Then use a compass and straightedge to construct a line perpendicular to line m through point P.
Question 5.
Answer:
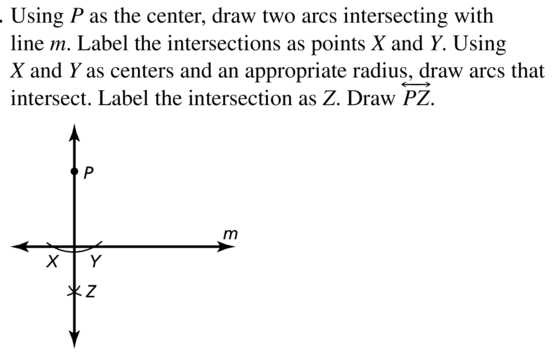
Question 6.
Answer:
The given figure is:

Now,
Using P as the center, draw two arcs intersecting with line m.
Label the intersections as points X and Y.
Using X and Y as centers and an appropriate radius, draw arcs that intersect.
Label the intersection as Z. Draw \(\overline{P Z}\)
Question 7.
Answer:
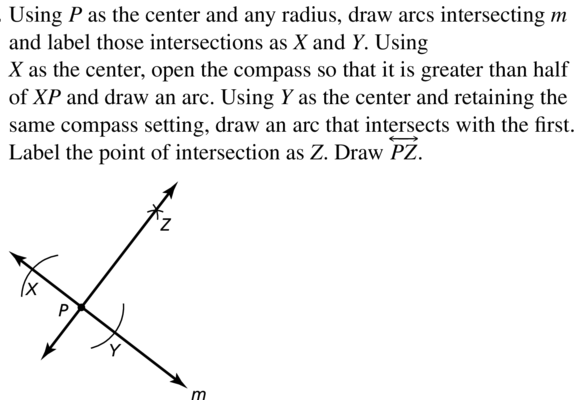
Question 8.
![]()
Answer:
The given figure is:
![]()
Now,
Using P as the center and any radius, draw arcs intersecting m and label those intersections as X and Y.
Using X as the center, open the compass so that it is greater than half of XP and draw an arc.
Using Y as the center and retaining the same compass setting, draw an arc that intersects with the first
Label the point of intersection as Z. Draw \(\overline{P Z}\)
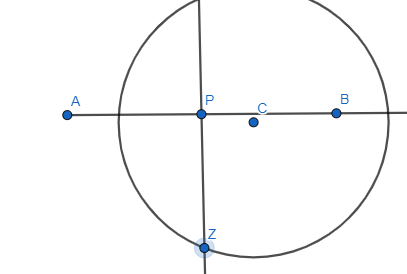
CONSTRUCTION
In Exercises 9 and 10, trace \(\overline{A B}\). Then use a compass and straightedge to construct the perpendicular bisector of \(\overline{A B}\)
Question 9.
Answer:

Question 10.

Answer:
The given figure is:

Now,
Using a compass setting greater than half of AB, draw two arcs using A and B as centers
Connect the points of intersection of the arcs with a straight line
ERROR ANALYSIS
In Exercises 11 and 12, describe and correct the error in the statement about the diagram.
Question 11.
Answer:

Question 12.
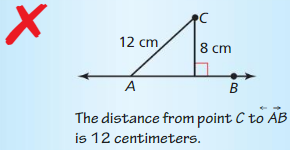
Answer:
We know that,
According to the Perpendicular Transversal theorem,
The distance from the perpendicular to the line is given as the distance between the point and the non-perpendicular line
So,
From the given figure,
The distance from point C to AB is the distance between point C and A i.e., AC
Hence, from the above,
We can conclude that the distance from point C to AB is: 12 cm
PROVING A THEOREM
In Exercises 13 and 14, prove the theorem.
Question 13.
Linear Pair Perpendicular Theorem (Thm. 3. 10)
Answer:
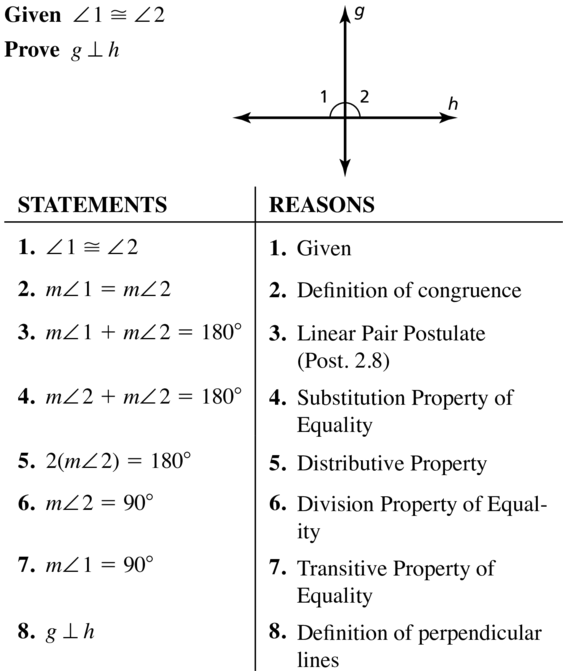
Question 14.
Lines Perpendicular to a Transversal Theorem (Thm. 3.12)
Answer:
In a plane, if a line is perpendicular to one of the two parallel lines, then it is perpendicular to the other line also

Proof:
Given: k || l, t ⊥ k
Prove: t ⊥ l

PROOF
In Exercises 15 and 16, use the diagram to write a proof of the statement.
Question 15.
If two intersecting lines are perpendicular. then they intersect to form four right angles.
Given a ⊥ b
Prove ∠1, ∠2, ∠3, and ∠4 are right angles.
Answer:
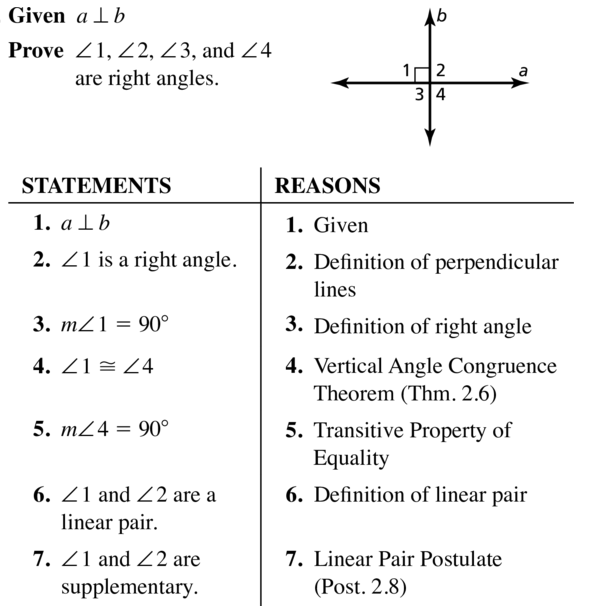
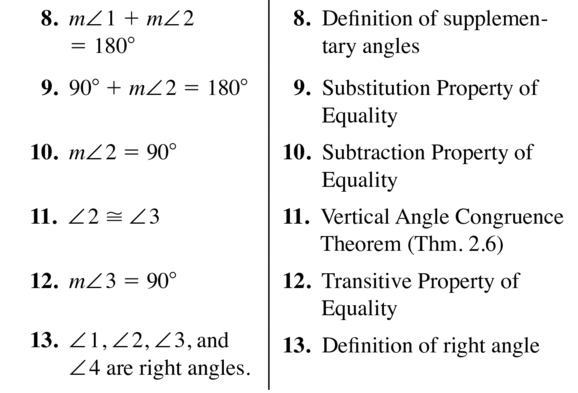
Question 16.
If two sides of two adjacent acute angles are perpendicular, then the angles are complementary.
Given \(\overrightarrow{B A}\) ⊥\(\vec{B}\)C
Prove ∠1 and ∠2 are complementary
Answer:
We have to find the theorem that satisfies the given statement.
We know that the given angles∠4, ∠5 are complementary thus, the theorem 3.10
justifies the statement which states that-If two sides of two adjacent acute angles are perpendicular, then the angles are complementary.
In Exercises 17-22, determine which lines, if any, must be parallel. Explain your reasoning.
Question 17.
Answer:

Question 18.
Answer:
The given figure is:

From the given figure,
We can observe that a is perpendicular to both the lines b and c
Hence, from the above,
We can conclude that
By using the Perpendicular transversal theorem,
a is both perpendicular to b and c and b is parallel to c
Question 19.
Answer:

Question 20.
Answer:
The given figure is;

From the given figure,
We can observe that
a is perpendicular to d and b is perpendicular to c
For parallel lines, we can’t say anything
Hence, from the above,
By using the Perpendicular transversal theorem,
a is perpendicular to d and b isperpendicular to c
Question 21.
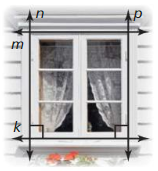
Answer:
Question 22.
Answer:
The given figure is:

From the given figure,
We can observe that
w ⊥ v and w⊥ y
So,
We can say that w and v are parallel lines by “Perpendicular Transversal Theorem”
We can observe that
z ⊥ x and w ⊥ z
So,
We can say that w and x are parallel lines by “Perpendicular Transversal theorem”
Question 23.
USING STRUCTURE
Find all the unknown angle measures in the diagram. Justify your answer for cacti angle measure.
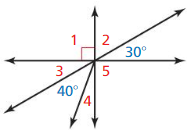
Answer:
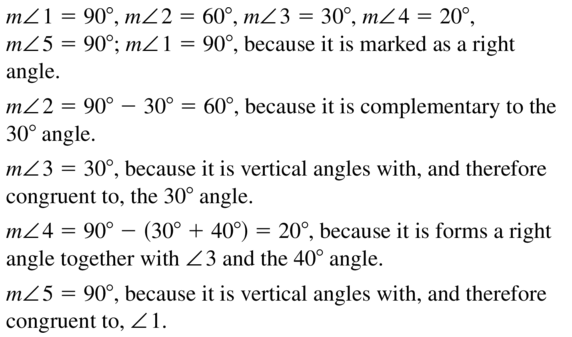
Question 24.
MAKING AN ARGUMENT
Your friend claims that because you can find the distance from a point to a line, you should be able to find the distance between any two lines. Is your friend correct? Explain your reasoning.
Answer:
No, your friend is not correct
Explanation:
It is given that your friend claims that because you can find the distance from a point to a line, you should be able to find the distance between any two lines
Now,
This can be proven by following the below steps:
Hence, from the above,
We can conclude that your friend is not correct
Question 25.
MATHEMATICAL CONNECTIONS
Find the value of x when a ⊥ b and b || c.
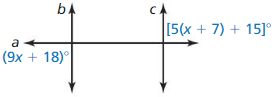
Answer:
Question 26.
HOW DO YOU SEE IT?
You are trying to cross a stream from point A. Which point should you jump to in order to jump the shortest distance? Explain your reasoning.
Answer:
From the given figure,
We can observe that
Point A is perpendicular to Point C
We know that,
According to Perpendicular Transversal Theorem,
The distance between the perpendicular points is the shortest
Hence, from the above,
We can conclude that in order to jump the shortest distance, you have to jump to point C from point A
Question 27.
ATTENDING TO PRECISION
In which of the following diagrams is \(\overline{A C}\) || \(\overline{B D}\) and \(\overline{A C}\) ⊥ \(\overline{C D}\)? Select all that apply.
(A) 
(B) 
(C) 
(D) 
(E) 
Answer:
![]()
Question 28.
THOUGHT-PROVOKING
The postulates and theorems in this book represent Euclidean geometry. In spherical geometry, all points are points on the surface of a sphere. A line is a circle on the sphere whose diameter is equal to the diameter of the sphere. In spherical geometry. how many right angles are formed by two perpendicular lines? Justify your answer.
Answer:
It is given that in spherical geometry, all points are points on the surface of a sphere. A line is a circle on the sphere whose diameter is equal to the diameter of the sphere.
We know that,
In Euclidean geometry, the two perpendicular lines form 4 right angles whereas, In spherical geometry, the two perpendicular lines form 8 right angles according to the “Parallel lines Postulate” in spherical geometry.
Hence, from the above,
We can conclude that 8 right angles are formed by two perpendicular lines in spherical geometry
Question 29.
CONSTRUCTION
Construct a square of side length AB
![]()
Answer:
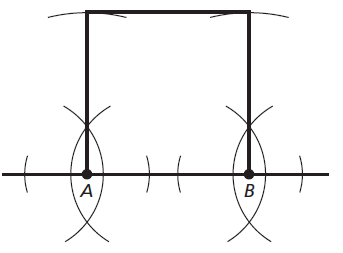
Question 30.
ANALYZING RELATIONSHIPS
The painted line segments that brain the path of a crosswalk are usually perpendicular to the crosswalk. Sketch what the segments in the photo would look like if they were perpendicular to the crosswalk. Which type of line segment requires less paint? Explain your reasoning.
Answer:
Question 31.
ABSTRACT REASONING
Two lines, a and b, are perpendicular to line c. Line d is parallel to line c. The distance between lines a and b is x meters. The distance between lines c and d is y meters. What shape is formed by the intersections of the four lines?
Answer:

Question 32.
MATHEMATICAL CONNECTIONS
Find the distance between the lines with the equations y = \(\frac{3}{2}\) + 4 and – 3x + 2y = – 1.
Answer:
The given equations are:
y = \(\frac{3}{2}\) + 4 and -3x + 2y = -1
y = \(\frac{3}{2}\) + 4 and y = \(\frac{3}{2}\)x – \(\frac{1}{2}\)
Compare the given equations with
y = mx + c
So,
m1 = m2 = \(\frac{3}{2}\)
c1 = 4
c2= –\(\frac{1}{2}\)
We know that,
The distance between the two parallel lines is:
d = | c1 – c2 |
So,
The distance between the given 2 parallel lines = | c1 – c2 |
= | 4 + \(\frac{1}{2}\) |
= \(\frac{9}{2}\)
Hence, from the above,
We can conclude that the distance between the given lines is: \(\frac{7}{2}\)
Question 33.
WRITING
Describe how you would find the distance from a point to a plane. Can you find the distance from a line to a plane? Explain your reasoning.
Answer:

Maintaining Mathematical Proficiency
Simplify the ratio.
Question 34.
\(\frac{6-(-4)}{8-3}\)
Answer:
The given expression is:
\(\frac{6 – (-4)}{8 – 3}\)
= \(\frac{6 + 4}{8 – 3}\)
= \(\frac{10}{5}\)
= 2
Hence,f rom the above,
We can conclude that the value of the given expression is: 2
Question 35.
\(\frac{3-5}{4-1}\)
Answer:
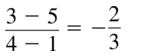
Question 36.
\(\frac{8-(-3)}{7-(-2)}\)
Answer:
The given expression is:
\(\frac{8 – (-3)}{7 – (-2)}\)
= \(\frac{8 + 3}{7 + 2}\)
= \(\frac{11}{9}\)
Hence,f rom the above,
We can conclude that the value of the given expression is: \(\frac{11}{9}\)
Question 37.
\(\frac{13-4}{2-(-1)}\)
Answer:
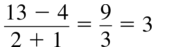
Identify the slope and the y-intercept of the line.
Question 38.
y = 3x + 9
Answer:
The given equation is:
y = 3x + 9 ——-(1)
Compare the above equation with
y = mx + c
Where,
m is the slope
c is the y-intercept
By comparing eq. (1) with the y = mx + c,
m = 3 and c = 9
Hence, from the above,
We can conclude that
The slope is: 3
The y-intercept is: 9
Question 39.
y = –\(\frac{1}{2}\)x + 7
Answer:
![]()
Question 40.
y = \(\frac{1}{6}\)x – 8
Answer:
The given equation is:
y = \(\frac{1}{6}\)x – 8
Compare the above equation with
y = mx + c
So,
m = \(\frac{1}{6}\) and c = -8
Hence, from the above,
We can conclude that
The slope is: \(\frac{1}{6}\)
The y-intercept is: -8
Question 41.
y = – 8x – 6
Answer:
![]()
3.5 Equations of Parallel and Perpendicular Lines
Exploration 1
Writing Equations of Parallel and Perpendicular Lines
Work with a partner: Write an equation of the line that is parallel or perpendicular to the given line and passes through the given point. Use a graphing calculator to verify your answer. What is the relationship between the slopes?
a.
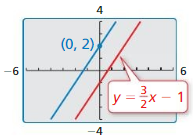
Answer:
The given figure is:

We know that,
From the given figure,
We can observe that the given lines are parallel lines
Now,
The equation for another line is:
y = \(\frac{3}{2}\)x + c
Substitute (0, 2) in the above equation
So,
2 = 0 + c
c = 2
So,
The equation for another parallel line is:
y = \(\frac{3}{2}\)x + 2
When we compare the given equation with the obtained equation,
We can observe that the slopes are the same and the y-intercepts are different
We know that,
The lines that have the same slope and different y-intercepts are “Parallel lines”
Hence, from the above,
We can conclude that the parallel lines are:
y = \(\frac{3}{2}\)x – 1
y = \(\frac{3}{2}\)x + 2
b.
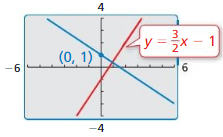
Answer:
The given figure is:

We know that,
From the given figure,
We can observe that the given lines are perpendicular lines
The equation for another line is:
y = \(\frac{3}{2}\)x + c
We know that,
The slope of perpendicular lines is: -1
So,
m1m2 = -1
\(\frac{3}{2}\) . m2 = -1
So,
m2 = –\(\frac{2}{3}\)
So,
y = –\(\frac{2}{3}\)x + c
Substitute (0, 1) in the above equation
So,
1 = 0 + c
c = 1
So,
The equation for another perpendicular line is:
y = –\(\frac{2}{3}\)x + 1
When we compare the given equation with the obtained equation,
We can observe that the product of the slopes are -1 and the y-intercepts are different
We know that,
The lines that have the slopes product -1 and different y-intercepts are “Perpendicular lines”
Hence, from the above,
We can conclude that the perpendicular lines are:
y = \(\frac{3}{2}\)x – 1
y = –\(\frac{2}{3}\)x + 1
c.
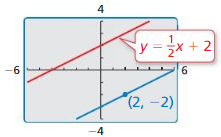
Answer:
The given figure is:

We know that,
From the given figure,
We can observe that the given lines are parallel lines
Now,
The equation for another line is:
y = \(\frac{1}{2}\)x + c
Substitute (2, -2) in the above equation
So,
-2 = \(\frac{1}{2}\) (2) + c
-2 = 1 + c
c = 2 – 1
c = -3
So,
The equation for another parallel line is:
y = \(\frac{1}{2}\)x – 3
When we compare the given equation with the obtained equation,
We can observe that the slopes are the same and the y-intercepts are different
We know that,
The lines that have the same slope and different y-intercepts are “Parallel lines”
Hence, from the above,
We can conclude that the parallel lines are:
y = \(\frac{1}{2}\)x + 2
y = \(\frac{1}{2}\)x – 3
d.
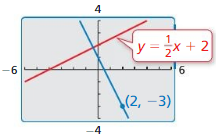
Answer:
The given figure is:

We know that,
From the given figure,
We can observe that the given lines are perpendicular lines
The equation for another line is:
y = \(\frac{1}{2}\)x + c
We know that,
The slope of perpendicular lines is: -1
So,
m1m2 = -1
\(\frac{1}{2}\) . m2 = -1
So,
m2 = -2
So,
y = -2x + c
Substitute (2, -3) in the above equation
So,
-3 = -2 (2) + c
-3 = -4 + c
c = 4 – 3
c = 1
So,
The equation for another perpendicular line is:
y = -2x + 1
When we compare the given equation with the obtained equation,
We can observe that the product of the slopes are -1 and the y-intercepts are different
We know that,
The lines that have the slopes product -1 and different y-intercepts are “Perpendicular lines”
Hence, from the above,
We can conclude that the perpendicular lines are:
y = \(\frac{1}{2}\)x + 2
y = -2x + 1
e.
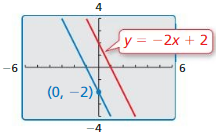
Answer:
The given figure is:

We know that,
From the given figure,
We can observe that the given lines are parallel lines
Now,
The equation for another line is:
y = -2x + c
Substitute (0, -2) in the above equation
So,
-2 = 0 + c
c = -2
So,
The equation for another parallel line is:
y = -2x – 2
When we compare the given equation with the obtained equation,
We can observe that the slopes are the same and the y-intercepts are different
We know that,
The lines that have the same slope and different y-intercepts are “Parallel lines”
Hence, from the above,
We can conclude that the parallel lines are:
y = -2x + 2
y = -2x – 2
f.
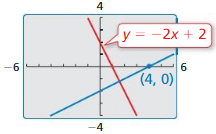
Answer:
The given figure is:

We know that,
From the given figure,
We can observe that the given lines are perpendicular lines
The equation for another line is:
y = -2x + c
We know that,
The slope of perpendicular lines is: -1
So,
m1m2 = -1
-2 . m2 = -1
So,
m2 = \(\frac{1}{2}\)
So,
y = \(\frac{1}{2}\)x + c
Substitute (4, 0) in the above equation
So,
0 = \(\frac{1}{2}\) (4) + c
0 = 2 + c
c = 0 – 2
c = -2
So,
The equation for another perpendicular line is:
y = \(\frac{1}{2}\)x – 2
When we compare the given equation with the obtained equation,
We can observe that the product of the slopes are -1 and the y-intercepts are different
We know that,
The lines that have the slopes product -1 and different y-intercepts are “Perpendicular lines”
Hence, from the above,
We can conclude that the perpendicular lines are:
y = \(\frac{1}{2}\)x – 2
y = -2x + 2
Exploration 2
Writing Equations of Parallel and Perpendicular Lines
Work with a partner: Write the equations of the parallel or perpendicular lines. Use a graphing calculator to verify your answers.
a.
Answer:
The given figure is:

From the given graph,
We can observe that
The given lines are the parallel lines
Now,
The coordinates of the line of the first equation are: (-1.5, 0), and (0, 3)
The coordinates of the line of the second equation are: (1, 0), and (0, -2)
Compare the given coordinates with
A (x1, y1), and B (x2, y2)
We know that,
Slope (m) = \(\frac{y2 – y1}{x2 – x1}\)
Now,
The slope of the line of the first equation is:
m = \(\frac{3 – 0}{0 + 1.5}\)
m = \(\frac{3}{1.5}\)
m = 2
Now,
We know that,
The standard linear equation is:
y = mx + c
So,
y = 2x + c
We know that,
For parallel lines,
The slopes are the same but the y-intercepts are different
Hence,
The given parallel line equations are:
y = 2x + c1
y = 2x + c2
b.
Answer:
The given figure is:

From the given figure,
We can observe that
The given lines are perpendicular lines
So,
The coordinates of the line of the first equation are: (0, -3), and (-1.5, 0)
The coordinates of the line of the second equation are: (-4, 0), and (0, 2)
Compare the given coordinates with
A (x1, y1), and B (x2, y2)
We know that,
Slope (m) = \(\frac{y2 – y1}{x2 – x1}\)
Now,
The slope of the line of the first equation is:
m = \(\frac{0 + 3}{0 – 1.5}\)
m = \(\frac{3}{-1.5}\)
m = \(\frac{-30}{15}\)
m = -2
Now,
We know that,
The standard linear equation is:
y = mx + c
So,
y = -2x + c
We know that,
For perpediclar lines,
The product of the slopes is -1 and the y-intercepts are different
So,
m1 × m2 = -1
-2 × m2 = -1
m2 = \(\frac{1}{2}\)
Hence,
The given perpendicular line equations are:
y = -2x + c1
y = \(\frac{1}{2}\)x + c2
Communicate Your Answer
Question 3.
How can you write an equation of a line that is parallel or perpendicular to a given line and passes through a given point?
MODELING WITH MATHEMATICS
To be proficient in math, you need to analyze relationships mathematically to draw conclusions.
Answer:
We know that,
The standard form of a linear equation is:
y = mx + c
Now,
For parallel lines,
We know that,
The slopes are the same and the y-intercepts are different
So,
To find the y-intercept of the equation that is parallel to the given equation, substitute the given point and find the value of c
Now,
For perpendicular lines,
we know that,
The product of the slopes is -1
So,
The slope of the equation that is perpendicular to the given equation is: –\(\frac{1}{m}\)
To find the y-intercept of the equation that is perpendicular to the given equation, substitute the given point and find the value of c
Question 4.
Write an equation of the line that is (a) parallel and (b) perpendicular to the line y = 3x + 2 and passes through the point (1, -2).
Answer:
The given equation is:
y = 3x + 2
The given point is: (1, -2)
a) Parallel line equation:
We know that,
The slope of the parallel equations are the same
So,
The slope of the equation that is parallel t the given equation is: 3
Now,
The equation that is parallel to the given equation is:
y = 3x + c
Substitute (1, -2) in the above equation
So,
-2 = 3 (1) + c
-2 – 3 = c
c = -5
Hence,
The equation of the line that is parallel to the given equation is:
y = 3x – 5
b) Perpendicular line equation:
We know that,
The product of the slope of the perpendicular equations is: -1
So,
m1 m2 = -1
3m2 = -1
So,
m2 = –\(\frac{1}{3}\)
So,
The slope of the equation that is parallel t the given equation is: –\(\frac{1}{3}\)
Now,
The equation that is perpendicular to the given equation is:
y = –\(\frac{1}{3}\)x + c
Substitute (1, -2) in the above equation
So,
-2 = –\(\frac{1}{3}\) (-2) + c
-2 – \(\frac{2}{3}\) = c
c = –\(\frac{8}{3}\)
Hence,
The equation of the line that is perpendicular to the given equation is:
y = –\(\frac{1}{3}\)x –\(\frac{8}{3}\)
Lesson 3.5 Equations of Parallel and Perpendicular Lines
Monitoring Progress
Find the coordinates of point P along the directed line segment AB so that AP to PB is the given ratio.
Question 1.
A(1, 3), B(8, 4); 4 to 1
Answer:
The given coordinates are: A (1, 3), and B (8, 4)
Compare the given points with
A (x1, y1), and B (x2, y2)
It is given that
AP : PB = 4 : 1
So,
We have to divide AB into 5 parts
Now,
We know that,
Slope (m) = \(\frac{y2 – y1}{x2 – x1}\)
So,
Slope of AB = \(\frac{4 – 3}{8 – 1}\)
Slope of AB = \(\frac{1}{7}\)
Now,
To find the coordinates of P, add slope to AP and PB
So,
P = (4 + (4 / 5) × 7, 1 + (4 / 5) × 1)
P = (22.4, 1.8)
Hence, from he above,
We can conclude that
The coordinates of P are (22.4, 1.8)
Question 2.
A(- 2, 1), B(4, 5); 3 to 7
Answer:
The given coordinates are: A (-2, 1), and B (4, 5)
Compare the given points with
A (x1, y1), and B (x2, y2)
It is given that
AP : PB = 3 : 7
So,
We have to divide AB into 10 parts
Now,
We know that,
Slope (m) = \(\frac{y2 – y1}{x2 – x1}\)
So,
Slope of AB = \(\frac{5 – 1}{4 + 2}\)
Slope of AB = \(\frac{4}{6}\)
Slope of AB = \(\frac{2}{3}\)
Now,
To find the coordinates of P, add slope to AP and PB
So,
P = (3 + (\(\frac{3}{10}\) × 3), 7 + (\(\frac{3}{10}\) × 2))
P = (3.9, 7.6)
Hence, from the above,
We can conclude that
The coordinates of P are (3.9, 7.6)
Question 3.
Determine which of the lines are parallel and which of the lines are perpendicular.
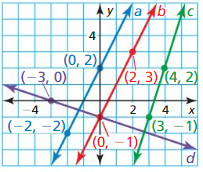
Answer:
The given figure is:

From the given figure,
The coordinates of line a are: (0, 2), and (-2, -2)
The coordinates of line b are: (2, 3), and (0, -1)
The coordinates of line c are: (4, 2), and (3, -1)
The coordinates of line d are: (-3, 0), and (0, -1)
Now,
Compare the given coordinates with (x1, y1), and (x2, y2)
So,
The slope of line a (m) = \(\frac{y2 – y1}{x2 – x1}\)
= \(\frac{-2 – 2}{-2 – 0}\)
= \(\frac{-4}{-2}\)
= 2
The slope of line b (m) = \(\frac{y2 – y1}{x2 – x1}\)
= \(\frac{-1 – 3}{0 – 2}\)
= \(\frac{-4}{-2}\)
= 2
The slope of line c (m) = \(\frac{y2 – y1}{x2 – x1}\)
= \(\frac{-1 – 2}{3 – 4}\)
= \(\frac{-3}{-1}\)
= 3
The slope of line d (m) = \(\frac{y2 – y1}{x2 – x1}\)
= \(\frac{-1 – 0}{0 + 3}\)
= \(\frac{-1}{3}\)
= –\(\frac{1}{3}\)
We know that,
The parallel lines have the same slopes
The perpendicular lines have the product of slopes equal to -1
Hence, from the above,
We can conclude that
Linea and Line b are parallel lines
Line c and Line d are perpendicular lines
Question 4.
Write an equation of the line that passes through the point (1, 5) and is
(a) parallel to the line y = 3x – 5 and
Answer:
The given equation is:
y = 3x – 5
The given point is: (1, 5)
We know that,
The parallel lines have the same slope
Compare the given equation with
y = mx + c
So,
The slope of the parallel line that passes through (1, 5) is: 3
So,
The equation of the parallel line that passes through (1, 5) is
y = 3x + c
To find the value of c, substitute (1, 5) in the above equation
So,
5 = 3 (1) + c
c = 5 – 3
c = 2
Hence,
The equation of the parallel line that passes through (1, 5) is:
y = 3x + 2
(b) perpendicular to the line y = 3x – 5.
Answer:
The given equation is:
y = 3x – 5
The given point is: (1, 5)
We know that,
The product of the slopes of the perpendicular lines is equal to -1
Now,
Compare the given equation with
y = mx + c
So,
m = 3
Now,
The slope of the perpendicular line that passes through (1, 5) is:
m1m2 = -1
3m2 = -1
m2 = –\(\frac{1}{3}\)
Now,
The equation of the perpendicular line that passes through (1, 5) is:
y = –\(\frac{1}{3}\)x + c
To find the value of c, substitute (1, 5) in the above equation
So,
5 = –\(\frac{1}{3}\) + c
c = 5 + \(\frac{1}{3}\)
c = \(\frac{16}{3}\)
Hence,
The equation of the perpendicular line that passes through (1, 5) is:
y = –\(\frac{1}{3}\)x + \(\frac{16}{3}\)
Question 5.
How do you know that the lines x = 4 and y = 2 are perpendiculars?
Answer:
The given lines are:
x = 4 and y = 2
We know that,
The line x = 4 is a vertical line that has the right angle i.e., 90°
The line y = 4 is a horizontal line that have the straight angle i.e., 0°
So,
The angle at the intersection of the 2 lines = 90° – 0° = 90°
Hence, from the above,
We can conclude that the lines x = 4 and y = 2 are perpendicular lines
Question 6.
Find the distance from the point (6, 4) to the line y = x + 4.
Answer:
The given equation is:
y = x + 4
Compare the given equation with
ax + by + c = 0
So,
x – y + 4 = 0
So,
a = 1, and b = -1
The given point is: (6, 4)
We know that,
The distance from the point (x, y) to the line ax + by + c = 0 is:
d = | ax + by + c| /\(\sqrt{a² + b²}\)
d = | x – y + 4 | / \(\sqrt{1² + (-1)²}\)
d = | x – y + 4 | / \(\sqrt{2}\)}
Substitute (6, 4) in the above equation
So,
d = | 6 – 4 + 4 |/ \(\sqrt{2}\)}
d = 3√2
Hence, from the above,
We can conclude that the distance from the given point to the given line is: 3√2
Question 7.
Find the distance from the point (- 1, 6) to the line y = – 2x.
Answer:
The given equation is:
y = -2x
Compare the given equation with
ax + by + c = 0
So,
2x + y = 0
So,
a = 2, and b = 1
The given point is: (-1, 6)
We know that,
The distance from the point (x, y) to the line ax + by + c = 0 is:
d = | ax + by + c| /\(\sqrt{a² + b²}\)
d = | 2x + y | / \(\sqrt{2² + (1)²}\)
d = | 2x + y | / \(\sqrt{5}\)}
Substitute (-1, 6) in the above equation
So,
d = | -2 + 6 |/ \(\sqrt{5}\)
d = \(\frac{4}{√5}\)
Hence, from the above,
We can conclude that the distance from the given point to the given line is: \(\frac{4}{√5}\)
Exercise 3.5 Equations of Parallel and Perpendicular Lines
Vocabulary and Core Concept Check
Question 1.
COMPLETE THE SENTENCE
A _________ line segment AB is a segment that represents moving from point A to point B.
Answer:
Question 2.
WRITING
How are the slopes of perpendicular lines related?
Answer:
We know that,
The “Perpendicular lines” are lines that intersect at right angles.
If you multiply the slopes of two perpendicular lines in the plane, you get −1 i.e., the slopes of perpendicular lines are opposite reciprocals
Monitoring Progress and Modeling with Mathematics
In Exercises 3 – 6. find the coordinates of point P along the directed line segment AB so that AP to PB is the given ratio.
Question 3.
A(8, 0), B(3, – 2); 1 to 4
Answer:
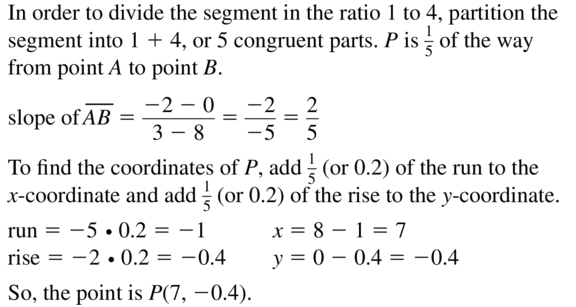
Question 4.
A(- 2, – 4), B(6, 1); 3 to 2
Answer:
The given coordinates are: A (-2, -4), and B (6, 1)
Compare the given points with
A (x1, y1), and B (x2, y2)
It is given that
AP : PB = 3 : 2
So,
We have to divide AB into 5 parts
Now,
We know that,
Slope (m) = \(\frac{y2 – y1}{x2 – x1}\)
So,
Slope of AB = \(\frac{1 + 4}{6 + 2}\)
Slope of AB = \(\frac{5}{8}\)
Now,
To find the coordinates of P, add slope to AP and PB
So,
P = (3 + (3 / 5) × 8, 2 + (3 / 5) × 5)
P = (7.8, 5)
Hence, from he above,
We can conclude that
The coordinates of P are (7.8, 5)
Question 5.
A(1, 6), B(- 2, – 3); 5 to 1
Answer:
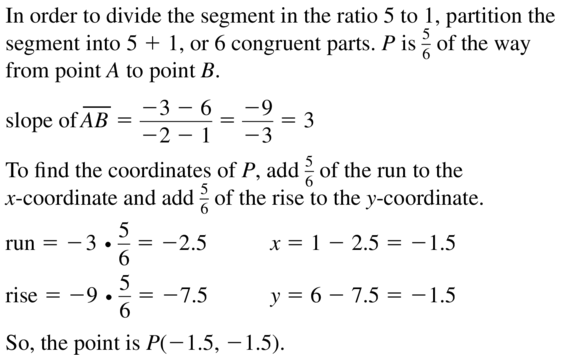
Question 6.
A(- 3, 2), B(5, – 4); 2 to 6
Answer:
The given coordinates are: A (-3, 2), and B (5, -4)
Compare the given points with
A (x1, y1), and B (x2, y2)
It is given that
AP : PB = 2 : 6
So,
We have to divide AB into 8 parts
Now,
We know that,
Slope (m) = \(\frac{y2 – y1}{x2 – x1}\)
So,
Slope of AB = \(\frac{-4 – 2}{5 + 3}\)
Slope of AB = \(\frac{-6}{8}\)
Now,
To find the coordinates of P, add slope to AP and PB
So,
P = (2 + (2 / 8) × 8, 6 + (2 / 8) × (-6))
P = (4, 4.5)
Hence, from the above,
We can conclude that
The coordinates of P are (4, 4.5)
In Exercises 7 and 8, determine which of the lines are parallel and which of the lines are perpendicular.
Question 7.
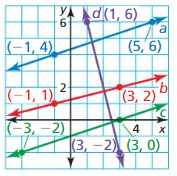
Answer:
Question 8.
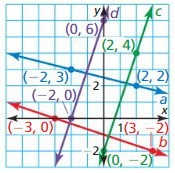
Answer:
The given figure is:

From the given figure,
The coordinates of line a are: (2, 2), and (-2, 3)
The coordinates of line b are: (3, -2), and (-3, 0)
The coordinates of line c are: (2, 4), and (0, -2)
The coordinates of line d are: (0, 6), and (-2, 0)
Now,
Compare the given coordinates with (x1, y1), and (x2, y2)
So,
The slope of line a (m) = \(\frac{y2 – y1}{x2 – x1}\)
= \(\frac{3 – 2}{-2 – 2}\)
= \(\frac{1}{-4}\)
= –\(\frac{1}{4}\)
The slope of line b (m) = \(\frac{y2 – y1}{x2 – x1}\)
= \(\frac{0 + 2}{-3 – 3}\)
= \(\frac{2}{-6}\)
= –\(\frac{1}{3}\)
The slope of line c (m) = \(\frac{y2 – y1}{x2 – x1}\)
= \(\frac{-4 – 2}{0 – 2}\)
= \(\frac{-6}{-2}\)
= 3
The slope of line d (m) = \(\frac{y2 – y1}{x2 – x1}\)
= \(\frac{6 – 0}{0 + 2}\)
= \(\frac{6}{2}\)
= 3
We know that,
The parallel lines have the same slopes
The perpendicular lines have the product of slopes equal to -1
Hence, from the above,
We can conclude that
Line c and Line d are parallel lines
Line b and Line c are perpendicular lines
In Exercises 9 – 12, tell whether the lines through the given points are parallel, perpendicular, or neither. justify your answer.
Question 9.
Line 1: (1, 0), (7, 4)
Line 2: (7, 0), (3, 6)
Answer:
Question 10.
Line 1: (- 3, 1), (- 7, – 2)
Line 2: (2, – 1), (8, 4)
Answer:
The coordinates of line 1 are: (-3, 1), (-7, -2)
The coordinates of line 2 are: (2, -1), (8, 4)
Compare the given coordinates with
(x1, y1), (x2, y2)
We know that,
Slope (m) = \(\frac{y2 – y1}{x2 – x1}\)
So,
Slope of line 1 = \(\frac{-2 – 1}{-7 + 3}\)
= \(\frac{-3}{-4}\)
= \(\frac{3}{4}\)
Slope of line 2 = \(\frac{4 + 1}{8 – 2}\)
= \(\frac{5}{6}\)
Hence,
By comparing the slopes,
We can conclude that the given lines are neither parallel nor perpendicular
Question 11.
Line 1: (- 9, 3), (- 5, 7)
Line 2: (- 11, 6), (- 7, 2)
Answer:
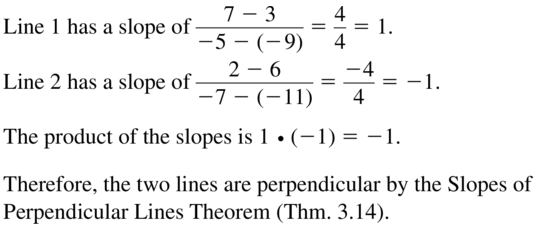
Question 12.
Line 1: (10, 5), (- 8, 9)
Line 2: (2, – 4), (11, – 6)
Answer:
The coordinates of line 1 are: (10, 5), (-8, 9)
The coordinates of line 2 are: (2, -4), (11, -6)
Compare the given coordinates with
(x1, y1), (x2, y2)
We know that,
Slope (m) = \(\frac{y2 – y1}{x2 – x1}\)
So,
Slope of line 1 = \(\frac{9 – 5}{-8 – 10}\)
= \(\frac{4}{-18}\)
= –\(\frac{2}{9}\)
Slope of line 2 = \(\frac{4 – 6}{11 – 2}\)
= \(\frac{-2}{9}\)
= –\(\frac{2}{9}\)
Hence,
By comparing the slopes,
We can conclude that the given lines are parallel
In Exercises 13 – 16. write an equation of the line passing through point P that ¡s parallel to the given line. Graph the equations of the lines to check that they are parallel.
Question 13.
P(0, – 1), y = – 2x + 3
Answer:
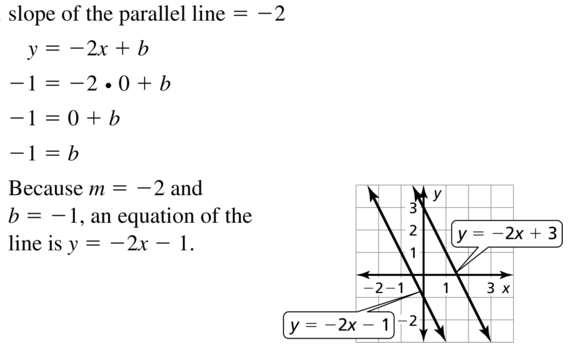
Question 14.
P(3, 8), y = \(\frac{1}{5}\)(x + 4)
Answer:
The given equation is:
y = \(\frac{1}{5}\) (x + 4)
The given point is: P (3, 8)
So,
y = \(\frac{1}{5}\)x + \(\frac{4}{5}\)
Now,
We know that,
The slopes are equal fot the parallel lines
So,
The equation that is parallel to the given equation is:
y = \(\frac{1}{5}\)x + c
Substitute P (3, 8) in the above equation to find the value of c
So,
8 = \(\frac{1}{5}\) (3) + c
c = 8 – \(\frac{3}{5}\)
c = \(\frac{37}{5}\)
Hence,
The parallel line equation that is parallel to the given equation is:
y = \(\frac{1}{5}\)x + \(\frac{37}{5}\)
The representation of the parallel lines in the coordinate plane is:
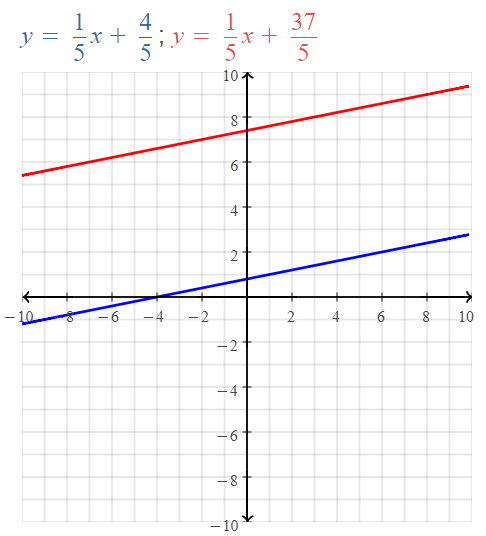
Question 15.
P(- 2, 6), x = – 5
Answer:
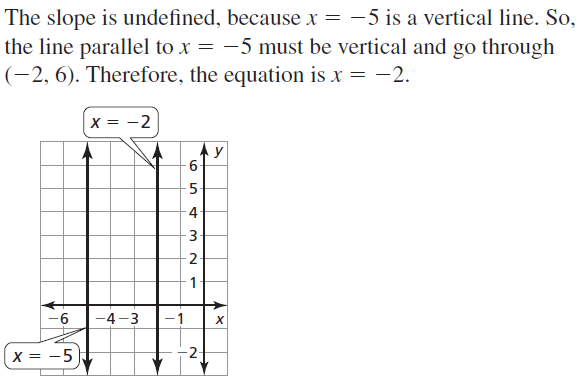
Question 16.
P(4, 0), – x + 2y = 12
Answer:
The given equation is:
-x + 2y = 12
The given point is: P (4, 0)
So,
y = \(\frac{1}{2}\)x + 6
Now,
We know that,
The slopes are equal fot the parallel lines
So,
The equation that is parallel to the given equation is:
y = \(\frac{1}{2}\)x + c
Substitute P (4, 0) in the above equation to find the value of c
So,
0 = \(\frac{1}{2}\) (4) + c
c = 2 – 0
c = 2
Hence,
The parallel line equation that is parallel to the given equation is:
y = \(\frac{1}{2}\)x + 2
The representation of the parallel lines in the coordinate plane is:
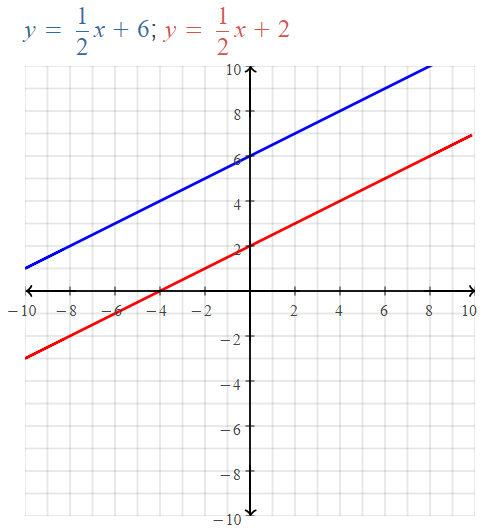
In Exercises 17 – 20. write an equation of the line passing through point P that is perpendicular to the given line. Graph the equations of the lines to check that they are perpendicular.
Question 17.
P(0, 0), y = – 9x – 1
Answer:
Question 18.
P(4, – 6)y = – 3
Answer:
The given equation is:
y = -3
The given point is: P (4, -6)
We know that,
The line that is perpendicular to y=n is:
x = n
So,
The line that is perpendicular to the given equation is:
x = n
Substitute P (4, -6) in the above equation
So,
x = 4
Hence, from the above,
We can conclude that
The equation that is perpendicular to y = -3 is:
x = 4
The representation of the perpendicular lines in the coordinate plane is:
Question 19.
P(2, 3), y – 4 = – 2(x + 3)
Answer:
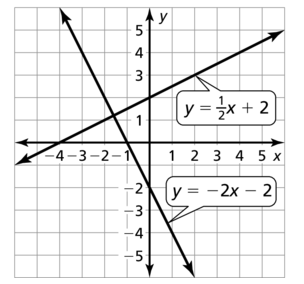
Question 20.
P(- 8, 0), 3x – 5y = 6
Answer:
The given equation is:
3x – 5y = 6
The given point is: P (-8, 0)
Now,
5y = 3x – 6
y = \(\frac{3}{5}\)x – \(\frac{6}{5}\)
We know that,
The product of the slopes of perpendicular lines is equal to -1
So,
m = –\(\frac{5}{3}\)
So,
The equation that is perpendicular to the given line equation is:
y = –\(\frac{5}{3}\)x + c
Substitute P(-8, 0) in the above equation
0 = –\(\frac{5}{3}\) ( -8) + c
c = \(\frac{40}{3}\)
Hence,
The equation that is perpendicular to the given equation is:
y = –\(\frac{5}{3}\)x + \(\frac{40}{3}\)
The representation of the perpendicular lines in the coordinate plane is:
In Exercises 21 – 24, find the distance from point A to the given line.
Question 21.
A(- 1, 7), y = 3x
Answer:

Question 22.
A(- 9, – 3), y = x – 6
Answer:
The given equation is:
y = x – 6 ——-(1)
The given point is: A (-9, -3)
Compare the given equation with
y = mx + c
We know that,
The product of the slopes of the perpendicular lines is equal to -1
So,
m1m2 = -1
1 (m2) = -3
m2 = -1
So,
The equation that is perpendicular to the given line equation is:
y = -x + c
Substitute A (-9, -3) in the above equation to find the value of c
So,
-3 = 9 + c
c = -9 – 3
c = -12
Hence,
The equation that is perpendicular to the given line equation is:
y = -x – 12 ——(2)
Now,
We have to find the point of intersection
So,
Eq. (1) = Eq. (2)
x – 6 = -x – 12
x + x = -12 + 6
2x = -6
x = \(\frac{-6}{2}\)
x = -3
So,
y = x – 6
y = -3 – 6
y = -9
So,
The point of intersection = (-3, -9)
Now,
The points are: (-9, -3), (-3, -9)
So,
d = \(\sqrt{(x2 – x1)² + (y2 – y1)²}\)
= \(\sqrt{(9 – 3)² + (9 – 3)²}\)
= \(\sqrt{(6)² + (6)²}\)
= 8.48
Hence, from the above,
We can conclude that the distance from point A to the given line is: 8.48
Question 23.
A(15, – 21), 5x + 2y = 4
Answer:
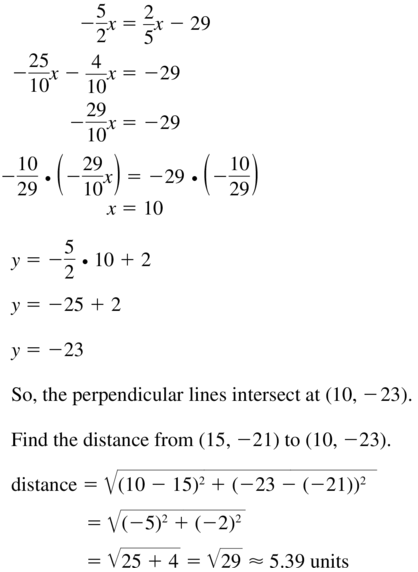
Question 24.
A(- \(\frac{1}{4}\), 5), – x + 2y = 14
Answer:
The given equation is:
-x + 2y = 14
y = \(\frac{1}{2}\)x + 7 ——-(1)
The given point is: A (-\(\frac{1}{4}\), 5)
Compare the given equation with
y = mx + c
We know that,
The product of the slopes of the perpendicular lines is equal to -1
So,
m1m2 = -1
\(\frac{1}{2}\) (m2) = -1
m2 = -2
So,
The equation that is perpendicular to the given line equation is:
y = -2x + c
Substitute A (-\(\frac{1}{4}\), 5) in the above equation to find the value of c
So,
5 = -2 (-\(\frac{1}{4}\)) + c
c = 5 – \(\frac{1}{2}\)
c = \(\frac{9}{2}\)
Hence,
The equation that is perpendicular to the given line equation is:
y = -2x + \(\frac{9}{2}\) ——(2)
Now,
We have to find the point of intersection
So,
Eq. (1) = Eq. (2)
\(\frac{1}{2}\)x + 7 = -2x + \(\frac{9}{2}\)
\(\frac{1}{2}\)x + 2x = -7 + 9/2
\(\frac{5}{2}\)x = –\(\frac{5}{2}\)
x = -1
So,
y = -2x + 3
y = -2 (-1) + \(\frac{9}{2}\)
y = \(\frac{13}{2}\)
So,
The point of intersection = (-1, \(\frac{13}{2}\))
Now,
The points are: (-\(\frac{1}{4}\), 5), (-1, \(\frac{13}{2}\))
So,
d = \(\sqrt{(x2 – x1)² + (y2 – y1)²}\)
= \(\sqrt{(3 / 2)² + (3 / 4)²}\)
= 1.67
Hence, from the above,
We can conclude that the distance from point A to the given line is: 1.67
Question 25.
ERROR ANALYSIS
Describe and correct the error in determining whether the lines are parallel. perpendicular, or neither.

Answer:

Question 26.
ERROR ANALYSIS
Describe and correct the error in writing an equation of the line that passes through the point (3, 4) and is parallel to the line y = 2x + 1.
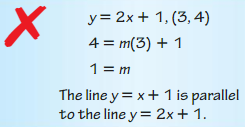
Answer:
The given equation of the line is:
y = 2x + 1
The given point is: (3, 4)
We know that,
The slopes of the parallel lines are the same
Now,
Compare the given equation with
y = mx + c
So,
m = 2
So,
The slope of the line that is aprallle to the given line equation is:
m = 2
So,
The equation of the line that is parallel to the given equation is:
y = 2x + c
To find the value of c,
Substitute (3, 4) in the above equation
So,
4 = 2 (3) + c
4 – 6 = c
c = -2
Hence, from the above,
We can conclude that the equation of the line that is parallel to the given line is:
y = 2x – 2
In Exercises 27-30. find the midpoint of \(\overline{P Q}\). Then write
an equation of the line that passes through the midpoint and is perpendicular to \(\overline{P Q}\). This line is called the perpendicular bisector.
Question 27.
P( – 4, 3), Q(4, – 1)
Answer:
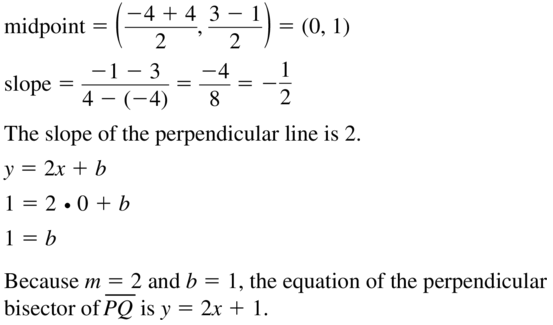
Question 28.
P(- 5, – 5), Q(3, 3)
Answer:
The given points are: P (-5, -5), Q (3, 3)
Compare the given points with (x1, y1), (x2, y2)
We know that,
The midpoint of PQ = (\(\frac{x1 + x2}{2}\), \(\frac{y1 + y2}{2}\))
= (\(\frac{-5 + 3}{2}\), \(\frac{-5 + 3}{2}\))
= (\(\frac{-2}{2}\), \(\frac{-2}{2}\))
= (-1, -1)
Now,
The slope of PQ = \(\frac{y2 – y1}{x2 – x1}\)
= \(\frac{3 + 5}{3 + 5}\)
= \(\frac{8}{8}\)
= 1
Now,
We know that,
The product of the slopes of the perpendicular lines is equal to -1
So,
m1m2 = -1
So,
m2 = -1
We know that,
The equation of the perpendicular line that passes through the midpoint of PQ is:
y = -x + c
To find the value of c,
Substitute (-1, -1) in the above equation
So,
-1 = -1 + c
c = 0
Hence, from the above,
We can conclude that the equation of the line that is perpendicular bisector is:
y = -x
Question 29.
P(0, 2), Q(6, – 2)
Answer:
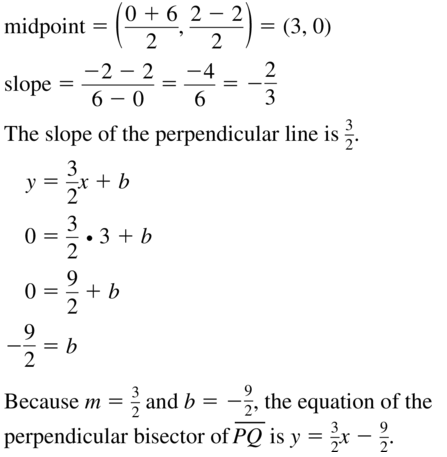
Question 30.
P(- 7, 0), Q(1, 8)
Answer:
The given points are: P (-7, 0), Q (1, 8)
Compare the given points with (x1, y1), (x2, y2)
We know that,
The midpoint of PQ = (\(\frac{x1 + x2}{2}\), \(\frac{y1 + y2}{2}\))
= (\(\frac{8 + 0}{2}\), \(\frac{-7 + 1}{2}\))
= (\(\frac{8}{2}\), \(\frac{-6}{2}\))
= (4, -3)
Now,
The slope of PQ = \(\frac{y2 – y1}{x2 – x1}\)
= \(\frac{8 – 0}{1 + 7}\)
= \(\frac{8}{8}\)
= 1
Now,
We know that,
The product of the slopes of the perpendicular lines is equal to -1
So,
m1m2 = -1
So,
m2 = -1
We know that,
The equation of the perpendicular line that passes through the midpoint of PQ is:
y = -x + c
To find the value of c,
Substitute (4, -3) in the above equation
So,
-3 = -4 + c
c = -3 + 4
c = 1
Hence, from the above,
We can conclude that the equation of the line that is perpendicular bisector is:
y = -x + 1
Question 31.
MODELING WITH MATHEMATICS
Your school lies directly between your house and the movie theater. The distance from your house to the school is one-fourth of the distance from the school to the movie theater. What point on the graph represents your school?

Answer:
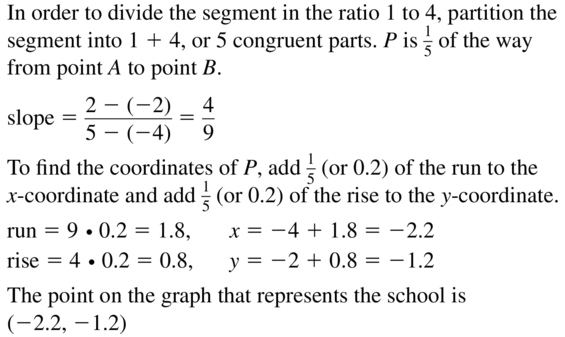
Question 32.
REASONING
Is quadrilateral QRST a parallelogram? Explain your reasoning.
Answer:
The given figure is:

From the above figure,
The coordinates of the quadrilateral QRST is:
Q (2, 6), R (6, 4), S (5, 1), and T (1, 3)
Compare the given points with (x1, y1), and (x2, y2)
Now,
We know that,
If both pairs of opposite sides of a quadrilateral are parallel, then it is a parallelogram
So,
If the slopes of the opposite sides of the quadrilateral are equal, then it is called as “Parallelogram”
We know that,
Slope (m) = \(\frac{y2 – y1}{x2 – x1}\)
So,
Slope of QR = \(\frac{4 – 6}{6 – 2}\)
Slope of QR = \(\frac{-2}{4}\)
Slope of QR = –\(\frac{1}{2}\)
Slope of RS = \(\frac{1 – 4}{5 – 6}\)
Slope of RS = \(\frac{-3}{-1}\)
Slope of RS = 3
Slope of ST = \(\frac{3 – 1}{1 – 5}\)
Slope of ST = \(\frac{2}{-4}\)
Slope of ST = –\(\frac{1}{2}\)
Slope of TQ = \(\frac{3 – 6}{1 – 2}\)
Slope of TQ = \(\frac{-3}{-1}\)
Slope of TQ = 3
Now,
From the slopes,
We can observe that the slopes of the opposite sides are equal i.e., the opposite sides are parallel
Hence, from the above,
We can conclude that the quadrilateral QRST is a parallelogram
Question 33.
REASONING
A triangle has vertices L(0, 6), M(5, 8). and N(4, – 1), Is the triangle a right triangle? Explain ‘your reasoning.
Answer:
Question 34.
MODELING WITH MATHEMATICS
A new road is being constructed parallel to the train tracks through points V. An equation of the line representing the train tracks is y = 2x. Find an equation of the line representing the new road.
Answer:
The given figure is:

It is given that a new road is being constructed parallel to the train tracks through points V. An equation of the line representing the train tracks is y = 2x.
So,
From the figure,
V = (-2, 3)
We know that,
The slopes of the parallel lines are the same
So,
By comparing the given equation with
y = mx + c
We get,
m = 2
So,
The equation of the line that is parallel to the line that represents the train tracks is:
y = 2x + c
Now,
To find the value of c,
Substitute (-2, 3) in the above equation
So,
3 = 2 (-2) + x
3 + 4 = c
c = 7
Hence, from the above,
We can conclude that the equation of the line that is parallel to the line representing railway tracks is:
y = 2x + 7
Question 35.
MODELING WITH MATHEMATICS
A bike path is being constructed perpendicular to Washington Boulevard through point P(2, 2). An equation of the line representing Washington Boulevard is y = –\(\frac{2}{3}\)x. Find an equation of the line representing the bike path.

Answer:

Question 36.
PROBLEM-SOLVING
A gazebo is being built near a nature trail. An equation of the line representing the nature trail is y = \(\frac{1}{3}\)x – 4. Each unit in the coordinate plane corresponds to 10 feet. Approximately how far is the gazebo from the nature trail?
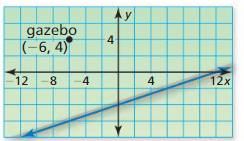
Answer:
The given figure is:

It is given that a gazebo is being built near a nature trail. An equation of the line representing the nature trail is y = \(\frac{1}{3}\)x – 4. Each unit in the coordinate plane corresponds to 10 feet
So,
It can be observed that
1 unit either in the x-plane or y-plane = 10 feet
So,
y = \(\frac{1}{3}\)x – 4
y = \(\frac{1}{3}\) (10) – 4
y = \(\frac{10 – 12}{3}\)
y = –\(\frac{2}{3}\)
We know that,
The distance won’t be in negative value,
y = \(\frac{2}{3}\)
y = 0.66 feet
Hence, from the above,
We can conclude that the distance of the gazebo from the nature trail is: 0.66 feet
Question 37.
CRITICAL THINKING
The slope of line l is greater than 0 and less than 1. Write an inequality for the slope of a line perpendicular to l. Explain your reasoning.
Answer:

Question 38.
HOW DO YOU SEE IT?
Determine whether quadrilateral JKLM is a square. Explain your reasoning.
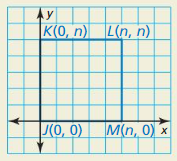
Answer:
The given figure is:

From the given figure,
The coordinates of a quadrilateral are:
J (0 0), K (0, n), L (n, n), M (n, 0)
Compare the given points with (x1, y1), and (x2, y2)
We know that,
Slope (m) = \(\frac{y2 – y1}{x2 – x1}\)
So,
Slope of JK = \(\frac{n – 0}{0 – 0}\)
= Undefined
Slope of KL = \(\frac{n – n}{n – 0}\)
= 0
Slope of LM = \(\frac{0 – n}{n – n}\)
= Undefined
Slope of MJ = \(\frac{0 – 0}{n – 0}\)
= 0
We know that,
For a square,
The slopes of perpendicular lines are undefined and 0 respectively
Hence, from the above,
We can conclude that quadrilateral JKLM is a square
Question 39.
CRITICAL THINKING
Suppose point P divides the directed line segment XY So that the ratio 0f XP to PY is 3 to 5. Describe the point that divides the directed line segment YX so that the ratio of YP Lo PX is 5 to 3.
Answer:

Question 40.
MAKING AN ARGUMENT
Your classmate claims that no two nonvertical parallel lines can have the same y-intercept. Is your classmate correct? Explain.
Answer:
Yes, your classmate is correct
Explanation:
It is given that your classmate claims that no two nonvertical parallel lines can have the same y-intercept
We know that,
The parallel lines have the same slope but have different y-intercepts and do not intersect
The intersecting lines intersect each other and have different slopes and have the same y-intercept
Hence, from the above,
We can conclude that the claim of your classmate is correct
Question 41.
MATHEMATICAL CONNECTIONS
Solve each system of equations algebraically. Make a conjecture about what the solution(s) can tell you about whether the lines intersect. are parallel, or are the same line.
a. y = 4x + 9
4x – y = 1
b. 3y + 4x = 16
2x – y = 18
c. y = – 5x + 6
10x + 2y = 12
Answer:
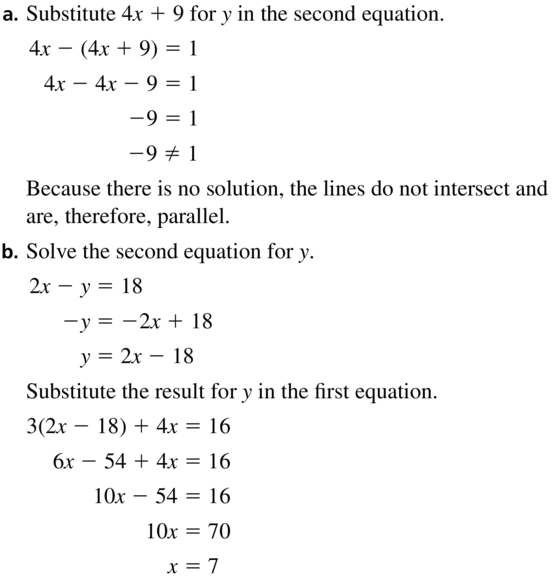

Question 42.
THOUGHT-PROVOKING
Find a formula for the distance from the point (x0, Y0) to the line ax + by = 0. Verify your formula using a point and a line.
Answer:

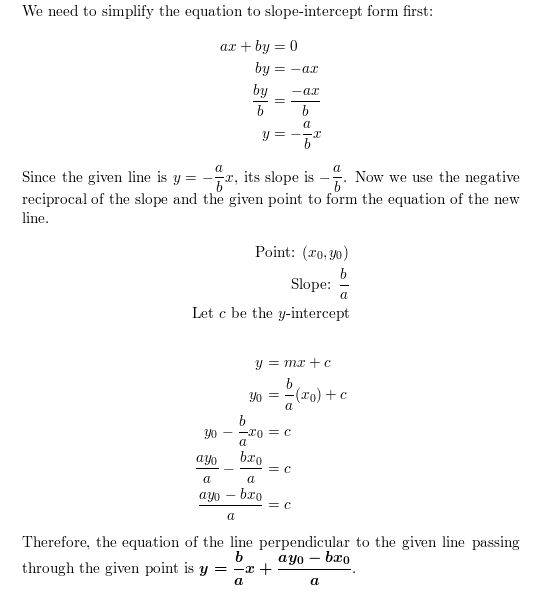
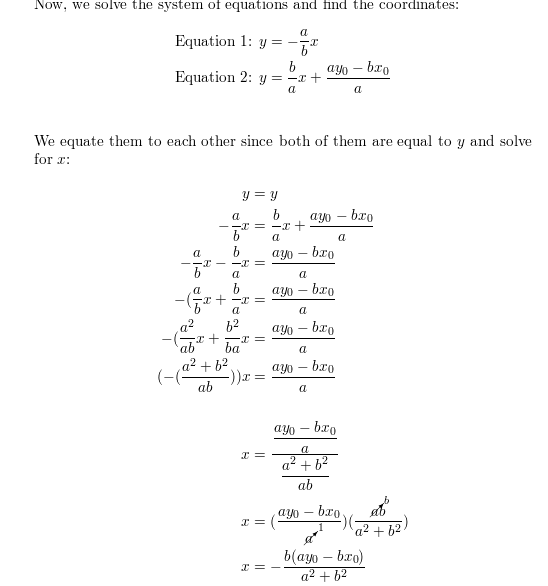
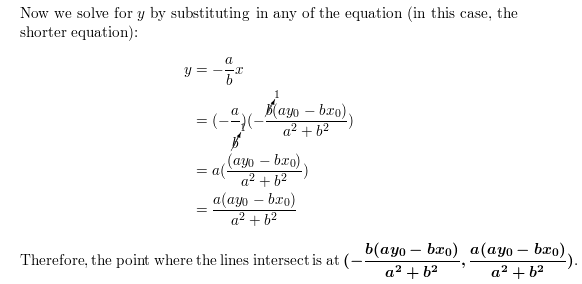

MATHEMATICAL CONNECTIONS
In Exercises 43 and 44, find a value for k based on the given description.
Question 43.
The line through (- 1, k) and (- 7, – 2) is parallel to the line y = x + 1.
Answer:
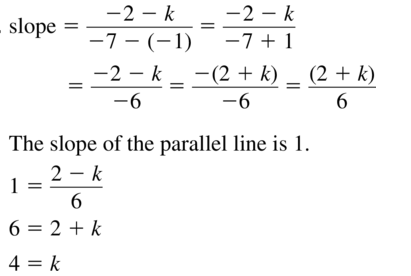
Question 44.
The line through (k, 2) and (7, 0) is perpendicular to the line y = x – \(\frac{28}{5}\).
Answer:
The given points are: (k, 2), and (7, 0)
The given line that is perpendicular to the given points is:
y = x – \(\frac{28}{5}\)
Now,
Compare the given points with (x1, y1), and (x2, y2)
So,
Slope (m) = \(\frac{y2 – y1}{x2 – x1}\)
m = \(\frac{0 – 2}{7 – k}\)
m = \(\frac{-2}{7 – k}\)
Now,
The slope that is perpendicular to the given line is:
m = -1 [ Since we know that m1m2 = -1]
So,
-1 = \(\frac{-2}{7 – k}\)
k – 7 = -2
k = -2 + 7
k = 5
Hence, from the above,
We can conclude that the value of k is: 5
Question 45.
ABSTRACT REASONING
Make a conjecture about how to find the coordinates of a point that lies beyond point B along \(\vec{A}\)B. Use an example to support your conjecture.
Answer:

Question 46.
PROBLEM-SOLVING
What is the distance between the lines y = 2x and y = 2x + 5? Verify your answer.
Answer:
The given lines are:
y = 2x and y = 2x + 5
Compare the given equations with
y = mx + c
Now,
To find the distance between the two lines, we have to find the intersection point of the line
So,
The intersection of the line is the y-intercept
So,
From y = 2x + 5,
The intersection point is: (0, 5)
Now,
For the intersection point of y = 2x,
Find the perpendicular line of y = 2x and find the intersection point of the two lines
We know that,
The product of the slopes of the perpendicular lines is equal to -1
So,
2m2 = -1
m2 = –\(\frac{1}{2}\)
So,
The perpendicular equation of y = 2x is:
y = –\(\frac{1}{2}\)x + c
To find the value of c in the above equation, substitue (0, 5) in the above equation
So,
5 = c
c = 5
So,
The perpendicular line equation of y = 2x is:
y = –\(\frac{1}{2}\)x + 5
Now,
For the intersection point,
2x = –\(\frac{1}{2}\)x + 5
2x + \(\frac{1}{2}\)x = 5
\(\frac{5}{2}\)x = 5
x = 2
So,
y = 2x
= 2 (2)
= 4
So,
The intersection point of y = 2x is: (2, 4)
Now,
The points are: (0, 5), and (2, 4)
Compare the given points with (x1, y1), and (x2, y2)
So,
d = \(\sqrt{(x2 – x1)² + (y2 – y1)²}\)
= \(\sqrt{(4 – 5)² + (2 – 0)²}\)
= \(\sqrt{1 + 4}\)
= 2.23
Hence, from the above,
We can conclude that the distance between the lines y = 2x and y = 2x + 5 is: 2.23
PROVING A THEOREM
In Exercises 47 and 48, use the slopes of lines to write a paragraph proof of the theorem.
Question 47.
Lines Perpendicular to a Transversal Theorem (Theorem 3.12): In a plane. if two lines are perpendicular to the same line. then they are parallel to each other.
Answer:

Question 48.
Transitive Property of Parallel Lines Theorem (Theorem 3.9),/+: If two lines are parallel to the same line, then they are parallel to each other.
Answer:
The given statement is:
If two lines are parallel to the same line, then they are parallel to each other
Proof:
Let the two parallel lines be E and F and the plane they lie be plane x
Let the two parallel lines that are parallel to the same line be G
Hence,
According to the Transitive Property of parallel lines,
If line E is parallel to line F and line F is parallel to line G, then line E is parallel to line G.
Question 49.
PROOF
Prove the statement: If two lines are vertical. then they are parallel.
Answer:

Question 50.
PROOF
Prove the statement: If two lines are horizontal, then they are parallel.
Answer:
The given statement is:
If two lines are horizontal, then they are parallel
Proof:
If two lines x and y are horizontal lines and they are cut by a vertical transversal z, then
x ⊥ z and y ⊥ z
Hence,
x || y is proved by the Lines parallel to Transversal Theorem
Question 51.
PROOF
Prove that horizontal lines are perpendicular to vertical lines.
Answer:
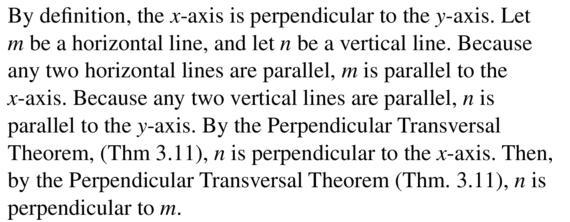
Maintaining Mathematical Proficiency
Plot the point in a coordinate plane.
Question 52.
A(3, 6)
Answer:
The given point is: A(3, 6)
The representation of the given point in the coordinate plane is:
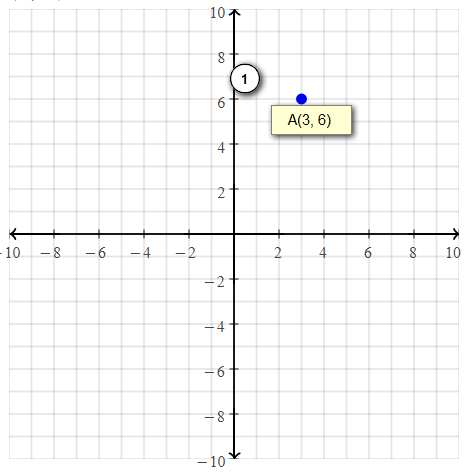
Question 53.
B(0, – 4)
Answer:
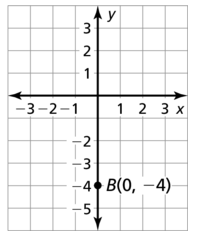
Question 54.
C(5, 0)
Answer:
The given point is: C (5, 0)
The representation of the given point in the coordinate plane is:
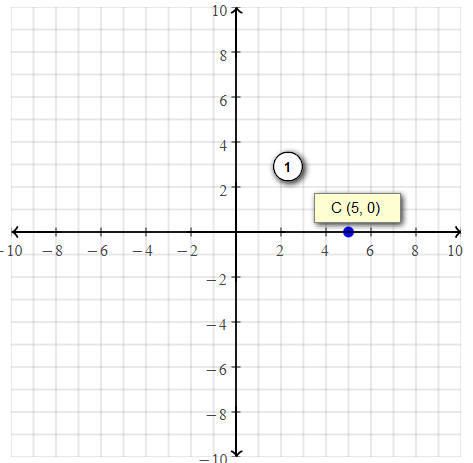
Question 55.
D( – 1, – 2)
Answer:
Copy and complete the table.
Question 56.
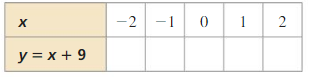
Answer:
The given table is:

From the above table,
The given equation is:
y = x + 9
Hence,
The completed table is:
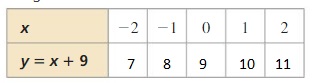
Question 57.
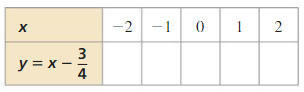
Answer:
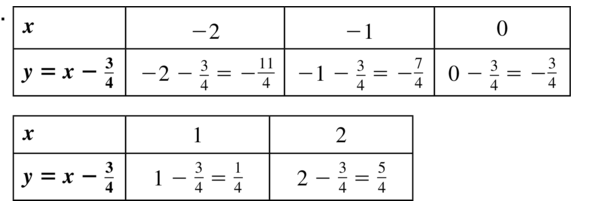
3.4 – 3.5 Performance Task: Navajo Rugs
Mathematical Practices
Question 1.
Compare the effectiveness of the argument in Exercise 24 on page 153 with the argument “You can find the distance between any two parallel lines” What flaw(s) exist in the argument(s)? Does either argument use correct reasoning? Explain.
Answer:
From the argument in Exercise 24 on page 153,
We can say that
The claim of your friend is not correct
We know that,
If we want to find the distance from the point to a given line, we need the perpendicular distance of a point and a line
Hence, from the above,
We can conclude that we can not find the distance between any two parallel lines if a point and a line is given to find the distance
Question 2.
Look back at your construction of a square in Exercise 29 on page 154. How would your
construction change if you were to construct a rectangle?
Answer:
From the construction of a square in Exercise 29 on page 154,
We can observe that the length of all the line segments are equal
Now,
If you were to construct a rectangle,
We have to keep the lengths of the length of the rectangles the same and the widths of the rectangle also the same
Question 3.
In Exercise 31 on page 161, a classmate tells you that our answer is incorrect because you should have divided the segment into four congruent pieces. Respond to your classmates’ argument by justifying your original answer.
Answer:
In Exercise 31 on page 161, from the coordinate plane,
We can observe that we divided the total distance into the four congruent segments or pieces
Hence, from the above,
We can conclude that the argument of your friend that the answer is incorrect is not correct
Parallel and Perpendicular Lines Chapter Review
3.1 Pairs of Lines and Angles
Think of each segment in the figure as part of a line. Which line(s) or plane(s) appear to fit the description?
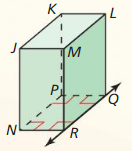
Question 1.
line(s) perpendicular to ![]()
Answer:
We know that,
The lines that are at 90° are “Perpendicular lines”
Hence,
From the above figure,
The lines perpendicular to \(\overline{Q R}\) are: \(\overline{R M}\) and \(\overline{Q L}\)
Question 2.
line(s) parallel to ![]()
Answer:
We know that,
The lines that do not have any intersection points are called “Parallel lines”
Hence,
From the above figure,
The line parallel to \(\overline{Q R}\) is: \(\overline {L M}\)
Question 3.
line(s) skew to ![]()
Answer:
We know that,
The lines that do not intersect and are not parallel and are not coplanar are “Skew lines”
Hence,
From the above figure,
The lines skew to \(\overline{Q R}\) are: \(\overline{J N}\), \(\overline{J K}\), \(\overline{K L}\), and \(\overline{L M}\)
Question 4.
plane(s) parallel to plane LMQ
Answer:
From the given figure,
We can conclude that the plane parallel to plane LMQ is: Plane JKL
3.2 Parallel Lines and Transversals
Find the values of x and y.
Question 5.
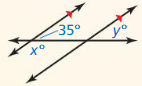
Answer:
The given figure is:

From the given figure,
We can observe that x and 35° are the corresponding angles
We know that,
By using the Corresponding Angles Theorem,
x = 35°
Now,
We can observe that 35° and y are the consecutive interior angles
So,
35° + y = 180°
y = 180° – 35°
y = 145°
Hence, from the above,
We can conclude that
x° = 35° and y° = 145°
Question 6.
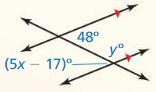
Answer:
The given figure is:

From the given figure,
We can observe that 48° and y are the consecutive interior angles and y and (5x – 17)° are the corresponding angles
So,
By using the Consecutive Interior Angles Theorem,
48° + y° = 180°
y° = 180° – 48°
y° = 132°
Now,
By using the corresponding angles theorem,
y° = (5x – 17)°
132° = (5x – 17)°
5x = 132° + 17°
5x = 149°
x = \(\frac{149}{5}\)
x° = 29.8°
Hence, from the above,
We can conclude that
x° = 29.8° and y° = 132°
Question 7.
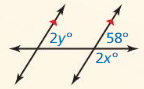
Answer:
The given figure is:

From the above figure,
We can observe that
2x° and 2y° are the alternate exterior angles
2y° and 58° are the alternate interior angles
So,
2x° = 2y° = 58°
So,
x° = y° =29°
Hence, from the above,
We can conclude that
x° = y° = 29°
Question 8.
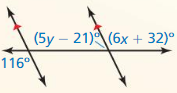
Answer:
The given figure is:

From the given figure,
We can observe that
(5y – 21)° and 116° are the corresponding angles
So,
(5y – 21)° = 116°
5y° = 116° + 21°
5y° = 137°
y° = \(\frac{137}{5}\)
y° = 27.4°
Now,
We can observe that
(5y – 21)° ad (6x + 32)° are the alternate interior angles
So,
(5y – 21)° = (6x + 32)°
5 (28)° – 21° = (6x + 32)°
140 – 21 – 32 = 6x°
6x° = 140° – 53°
6x = 87°
x = \(\frac{87}{6}\)
x° = 14.5°
Hence, from the above,
We can conclude that
x° = 14.5° and y° = 27.4°
3.3 Proofs with Parallel Lines
Find the value of x that makes m || n.
Question 9.
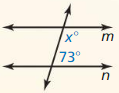
Answer:
The given figure is:

We know that,
m || n is true only when x and 73° are the consecutive interior angles according to the “Converse of Consecutive Interior angles Theorem”
Now,
It is given that m || n
So,
x + 73° = 180°
x = 180° – 73°
x = 107°
Hence, from the above,
We can conclude that the value of x is: 107°
Question 10.
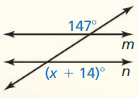
Answer:
The given figure is:

We know that,
m || n is true only when 147° and (x + 14)° are the corresponding angles by using the “Converse of the Alternate Exterior Angles Theorem”
Now,
It is given that m || n
So,
(x + 14)°= 147°
x° = 147° – 14°
x° = 133°
Hence, from the above,
We can conclude that the value of x is: 133°
Question 11.
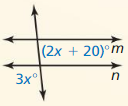
Answer:
The given figure is:

m || n is true only when 3x° and (2x + 20)° are the corresponding angles by using the “Converse of the Corresponding Angles Theorem”
Now,
It is given that m || n
So,
(2x + 20)°= 3x°
3x° – 2x° = 20°
x° = 20°
Hence, from the above,
We can conclude that the value of x is: 20°
Question 12.
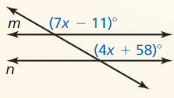
Answer:
The given figure is:

We know that,
m || n is true only when (7x – 11)° and (4x + 58)° are the alternate interior angles by the “Convesre of the Consecutive Interior Angles Theorem”
Now,
It is given that m || n
So,
(7x – 11)° = (4x + 58)°
7x° – 4x° = 58° + 11°
3x° = 69°
x° = \(\frac{69}{3}\)
x° = 23°
Hence, from the above,
We can conclude that the value of x is: 23°
3.4 Proofs with Perpendicular Lines
Determine which lines, if any, must be parallel. Explain your reasoning.
Question 13.
Answer:
The given figure is:

From the given figure,
We can observe that
x ⊥ z and y ⊥ z
We know that,
According to the Perpendicular Transversal Theorem,
In a plane, if a line is perpendicular to one of two parallel lines, then it is perpendicular to the other line also.
Hence, from the above,
We can conclude that x and y are parallel lines
Question 14.
Answer:
The given figure is:

From the given figure,
We can observe that
w ⊥ y and z ⊥ x
We can also observe that w and z is not both ⊥ to x and y
We know that,
According to the Perpendicular Transversal Theorem,
In a plane, if a line is perpendicular to one of two parallel lines, then it is perpendicular to the other line also.
Hence, from the above,
We can conclude that there are not any parallel lines in the given figure
Question 15.
Answer:
The given figure is:

From the given figure,
We can observe that
m ⊥ a, n ⊥ a, l ⊥ b, and n ⊥ b
We know that,
According to the Perpendicular Transversal Theorem,
In a plane, if a line is perpendicular to one of two parallel lines, then it is perpendicular to the other line also.
Hence, from the above,
We can conclude that m and n are parallel lines
Question 16.
Answer:
The given figure is:

From the given figure,
We can observe that
a ⊥ n, b ⊥ n, and c ⊥ m
We know that,
According to the Perpendicular Transversal Theorem,
In a plane, if a line is perpendicular to one of two parallel lines, then it is perpendicular to the other line also.
Hence, from the above,
We can conclude that there are not any parallel lines in the given figure
3.5 Equations of Parallel and Perpendicular Lines
Write an equation of the line passing through the given point that is parallel to the given line.
Question 17.
A(3, – 4),y = – x + 8
Answer:
The given equation is:
y = -x + 8
The given point is: A (3, -4)
So,
y = -x + 8
Now,
We know that,
The slopes are equal fot the parallel lines
So,
The equation that is parallel to the given equation is:
y = -x + c
Substitute A (3, -4) in the above equation to find the value of c
So,
-4 = -3 + c
c = -4 + 3
c = -1
Hence,
The parallel line equation that is parallel to the given equation is:
y = -x – 1
Question 18.
A(- 6, 5), y = \(\frac{1}{2}\)x – 7
Answer:
The given equation is:
y = \(\frac{1}{2}\)x – 7
The given point is: A (-6, 5)
So,
y = \(\frac{1}{2}\)x – 7
Now,
We know that,
The slopes are equal fot the parallel lines
So,
The equation that is parallel to the given equation is:
y = \(\frac{1}{2}\)x + c
Substitute A (-6, 5) in the above equation to find the value of c
So,
5 = \(\frac{1}{2}\) (-6) + c
c = 5 + 3
c = 8
Hence,
The parallel line equation that is parallel to the given equation is:
y = \(\frac{1}{2}\)x + 8
Question 19.
A(2, 0), y = 3x – 5
Answer:
The given equation is:
y = 3x – 5
The given point is: A (2, 0)
So,
y = 3x – 5
Now,
We know that,
The slopes are equal for the parallel lines
So,
The equation that is parallel to the given equation is:
y = 3x + c
Substitute A (2, 0) in the above equation to find the value of c
So,
0 = 3 (2) + c
c = 6 – 0
c = 6
Hence,
The parallel line equation that is parallel to the given equation is:
y = 3x – 6
Question 20.
A(3, – 1), y = \(\frac{1}{3}\)x + 10
Answer:
The given point is: A (3, -1)
The given equation is:,
y = \(\frac{1}{3}\)x + 10
Now,
We know that,
The slopes are equal fot the parallel lines
So,
The equation that is parallel to the given equation is:
y = \(\frac{1}{3}\)x + c
Substitute A (3, -1) in the above equation to find the value of c
So,
-1 = \(\frac{1}{3}\) (3) + c
c = -1 – 1
c = -2
Hence,
The parallel line equation that is parallel to the given equation is:
y = \(\frac{1}{3}\)x – 2
Write an equation of the line passing through the given point that is perpendicular to the given line.
Question 21.
A(6, – 1), y = – 2x + 8
Answer:
The given equation is:
y = -2x + 8
The given point is:A (6, -1)
Now,
y = -2x + 8
We know that,
The product of the slopes of perpendicular lines is equal to -1
So,
m = \(\frac{1}{2}\)
So,
The equation that is perpendicular to the given line equation is:
y = \(\frac{1}{2}\)x + c
Substitute A (6, -1) in the above equation
-1 = \(\frac{1}{2}\) ( 6) + c
c = -1 – 3
c = -4
Hence,
The equation that is perpendicular to the given equation is:
y = \(\frac{1}{2}\)x – 4
Question 22.
A(0, 3), y = – \(\frac{1}{2}\)x – 6
Answer:
The given equation is:
y = –\(\frac{1}{2}\)x – 6
The given point is: A (0, 3)
Now,
y = \(\frac{1}{2}\)x – 6
We know that,
The product of the slopes of perpendicular lines is equal to -1
So,
m = 2
So,
The equation that is perpendicular to the given line equation is:
y = 2x + c
Substitute A (0, 3) in the above equation
3 = 2 ( 0) + c
c = 3
Hence,
The equation that is perpendicular to the given equation is:
y = 2x + 3
Question 23.
A(8, 2),y = 4x – 7
Answer:
The given equation is:
y = 4x – 7
The given point is: A (8, 2)
Now,
y = 4x – 7
We know that,
The product of the slopes of perpendicular lines is equal to -1
So,
m = –\(\frac{1}{4}\)
So,
The equation that is perpendicular to the given line equation is:
y = –\(\frac{1}{4}\)x + c
Substitute A (8, 2) in the above equation
2 = –\(\frac{1}{4}\) (8) + c
c = 2 + 2
c = 4
Hence,
The equation that is perpendicular to the given equation is:
y = –\(\frac{1}{4}\)x + 4
Question 24.
A(-1, 5), y = \(\frac{1}{7}\)x + 4
Answer:
The given equation is:
y = \(\frac{1}{7}\)x + 4
The given point is: A (-1, 5)
Now,
y = \(\frac{1}{7}\)x + 4
We know that,
The product of the slopes of perpendicular lines is equal to -1
So,
m = -7
So,
the equation that is perpendicular to the given line equation is:
y = -7x + c
Substitute A (-1, 5) in the above equation
5 = -7 ( -1) + c
c = 5 – 7
c = -2
Hence,
The equation that is perpendicular to the given equation is:
y = -7x – 2
Find the distance front point A to the given line.
Question 25.
A(2, – 1), y = – x + 4
Answer:
The given equation is:
y = -x + 4 ——-(1)
The given point is: A (2, -1)
Compare the given equation with
y = mx + c
We know that,
The product of the slopes of the perpendicular lines is equal to -1
So,
m1m2 = -1
(-1) (m2) = -1
m2 = 1
So,
The equation that is perpendicular to the given line equation is:
y = x + c
Substitute A (2, -1) in the above equation to find the value of c
So,
-1 = 2 + c
c = -1 – 2
c = -3
Hence,
The equation that is perpendicular to the given line equation is:
y = x – 3 ——(2)
Now,
We have to find the point of intersection
So,
Eq. (1) = Eq. (2)
-x + 4 = x – 3
-x – x = -3 – 4
2x = 7
x = \(\frac{7}{2}\)
So,
y = x – 3
y = \(\frac{7}{2}\) – 3
y = \(\frac{1}{2}\)
So,
The point of intersection = (\(\frac{7}{2}\), \(\frac{1}{2}\))
Now,
The points are: (2, -1), (\(\frac{7}{2}\), \(\frac{1}{2}\))
So,
d = \(\sqrt{(x2 – x1)² + (y2 – y1)²}\)
= \(\sqrt{(3 / 2)² + (3 / 2)²}\)
= 2.12
Hence, from the above,
We can conclude that the distance from point A to the given line is: 2.12
Question 26.
A(- 2, 3), y = \(\frac{1}{2}\)x + 1
Answer:
The given equation is:
y = \(\frac{1}{2}\)x + 1 ——-(1)
The given point is: A (-2, 3)
Compare the given equation with
y = mx + c
We know that,
The product of the slopes of the perpendicular lines is equal to -1
So,
m1m2 = -1
(\(\frac{1}{2}\)) (m2) = -1
m2 = -2
So,
The equation that is perpendicular to the given line equation is:
y = -2x + c
Substitute A (-2, 3) in the above equation to find the value of c
So,
3 = -2 (-2) + c
c = 3 – 4
c = -1
Hence,
The equation that is perpendicular to the given line equation is:
y = -2x – 1 ——(2)
Now,
We have to find the point of intersection
So,
Eq. (1) = Eq. (2)
\(\frac{1}{2}\)x + 1 = -2x – 1
\(\frac{5}{2}\)x = 2
x = \(\frac{4}{5}\)
So,
y = -2x – 1
y = –\(\frac{8}{5}\) – 1
y = –\(\frac{13}{5}\)
So,
The point of intersection = (\(\frac{4}{5}\), –\(\frac{13}{5}\))
Now,
The points are: (-2, 3), (\(\frac{4}{5}\), –\(\frac{13}{5}\))
So,
d = \(\sqrt{(x2 – x1)² + (y2 – y1)²}\)
= \(\sqrt{31.36 + 7.84}\)
= 6.26
Hence, from the above,
We can conclude that the distance from point A to the given line is: 6.26
Parallel and Perpendicular Lines Test
Find the values of x and y. State which theorem(s) you used.
Question 1.
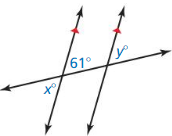
Answer:
The given figure is:

From the given figure,
We can observe that
x and 61° are the vertical angles
61° and y° are the alternate interior angles
We know that,
According to the “Alternate Interior Angles theorem”, the alternate interior angles are congruent
According to the “Vertical Angles Theorem”, the vertical angles are congruent
Hence,
x° = y° = 61°
Question 2.
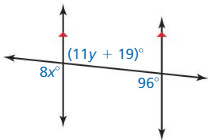
Answer:
The given figure is:

From the given figure,
We can observe that
8x° and 96° are the alternate interior angles
(11y + 19)° and 96° are the corresponding angles
We know that,
According to the “Alternate Interior Angles Theorem”, the alternate interior angles are congruent
According to the “Corresponding Angles Theorem”, the corresponding angles are congruent
So,
8x° = 96°
x° = \(\frac{96}{8}\)
x° = 12°
Now,
(11y + 19)° = 96°
11y° = 96° – 19°
11y° = 77°
y° = \(\frac{77}{11}\)
y° = 7°
Hence, from the above,
We can conclude that
x° = 12° and y° = 7°
Question 3.
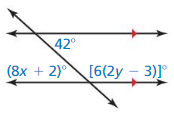
Answer:
The given figure is:

From the given figure,
We can observe that
42° and 6(2y – 3)° are the consecutive interior angles
42° and (8x + 2)° are the vertical angles
We know that,
According to the “Consecutive Interior Angles Theorem”, the sum of the consecutive interior angles is 180°
According to the “Vertical Angles Theorem”, the vertical angles are congruent
So,
42° + 6 (2y – 3)° = 180°
6 (2y°) – 6(3)° = 180° – 42°
12y° – 18° = 138°
12y° = 138° + 18°
12y° = 156°
y° = \(\frac{156}{12}\)
y° = 13°
Now,
42° = (8x + 2)°
8x° = 42° – 2°
x° = 40°
x° = \(\frac{40}{8}\)
x° = 5°
Hence, from the above,
We can conclude that
x° = 5° and y° = 13°
Find the distance from point A to the given line.
Question 4.
A(3, 4), y = – x
Answer:
The given equation is:
y = -x ——-(1)
The given point is: A (3, 4)
Compare the given equation with
y = mx + c
We know that,
The product of the slopes of the perpendicular lines is equal to -1
So,
m1m2 = -1
(-1) (m2) = -1
m2 = 1
So,
The equation that is perpendicular to the given line equation is:
x = c
Substitute A (3, 4) in the above equation to find the value of c
So,
c = 3
Hence,
The equation that is perpendicular to the given line equation is:
x = 3 ——(2)
Now,
We have to find the point of intersection
So,
Eq. (1) = Eq. (2)
-x = x – 3
-x – x = -3
2x = 3
x = \(\frac{3}{2}\)
So,
y = -x
y = –\(\frac{3}{2}\)
So,
The point of intersection = (\(\frac{3}{2}\), –\(\frac{3}{2}\))
Now,
The points are: (3, 4), (\(\frac{3}{2}\), –\(\frac{3}{2}\))
So,
d = \(\sqrt{(x2 – x1)² + (y2 – y1)²}\)
= \(\sqrt{30.25 + 2.25}\)
= 5.70
Hence, from the above,
We can conclude that the distance from point A to the given line is: 5.70
Question 5.
A(- 3, 7), y = \(\frac{1}{3}\)x – 2
Answer:
The given equation is:
y = \(\frac{1}{3}\)x – 2 ——-(1)
The given point is: A (-3, 7)
Compare the given equation with
y = mx + c
We know that,
The product of the slopes of the perpendicular lines is equal to -1
So,
m1m2 = -1
(\(\frac{1}{3}\)) (m2) = -1
m2 = -3
So,
The equation that is perpendicular to the given line equation is:
y = -3x + c
Substitute A (-3, 7) in the above equation to find the value of c
So,
7 = -3 (-3) + c
c = 7 – 9
c = -2
Hence,
The equation that is perpendicular to the given line equation is:
y = -3x – 2 ——(2)
Now,
We have to find the point of intersection
So,
Eq. (1) = Eq. (2)
\(\frac{1}{3}\)x – 2 = -3x – 2
\(\frac{1}{3}\)x + 3x = -2 + 2
x = 0
So,
y = -3x – 2
y = -3 (0) – 2
y = -2
So,
The point of intersection = (0, -2)
Now,
The points are: (-3, 7), (0, -2)
So,
d = \(\sqrt{(x2 – x1)² + (y2 – y1)²}\)
= \(\sqrt{(-2 – 7)² + (0 + 3)²}\)
= 9.48
Hence, from the above,
We can conclude that the distance from point A to the given line is: 9.48
Find the value of x that makes m || n.
Question 6.
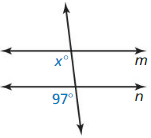
Answer:
The given figure is:

From the given figure,
We can observe that
x° and 97° are the corresponding angles
We know that,
According to the “Converse of the Corresponding Angles Theorem”, m || n is true only when the corresponding angles are congruent
It is given that m || n
So,
x° = 97°
Hence, from the above,
We can conclude that
x° = 97°
Question 7.
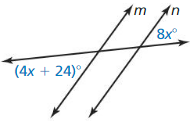
Answer:
The given figure is:

From the given figure,
We can observe that
8x° and (4x + 24)° are the alternate exterior angles
We know that,
According to the “Converse of the Alternate Exterior Angles Theorem”, m || n is true only when the alternate exterior angles are congruent
It is given that m || n
So,
8x° = (4x + 24)°
8x° – 4x° = 24°
4x° = 24°
x° = \(\frac{24}{4}\)
x° = 6°
Hence, from the above,
We can conclude that
x° = 6°
Question 8.
Answer:
The given figure is:

From the given figure,
We can observe that
(11x + 33)° and (6x – 6)° are the interior angles
We know that,
According to the “Converse of the Interior Angles Theory”, m || n is true only when the sum of the interior angles are supplementary
It is given that m || n
So,
(11x + 33)°+(6x – 6)° = 180°
17x° + 27° = 180°
17x° = 180° – 27°
x° = –\(\frac{153}{17}\)
x° = 9°
Hence, from the above,
We can conclude that
x° = 9°
Write an equation of the line that passes through the given point and is
(a) parallel to and
(b) perpendicular to the given line.
Question 9.
(- 5, 2), y = 2x – 3
Answer:
The given equation is:
y= 2x – 3
The given point is: (-5, 2)
a) Parallel to the given line:
We know that,
The slopes of the parallel lines are the same
Compare the given equation with
y = mx + c
So,
The equation of the line that is parallel to the given line is:
y = 2x + c
To find the value of c,
Substitute (-5, 2) in the above equation
So,
2 = 2 (-5) + c
2 + 10 = c
c = 12
Hence,
The equation of the line that is parallel to the given line equation is:
y = 2x + 12
b) Perpendicular to the given line:
We know that,
The product of the slopes of the perpendicular lines is equal to -1
So,
m1 m2 = -1
2m2 = -1
m2 = –\(\frac{1}{2}\)
So,
The equation of the line that is perpendicular to the given line equation is:
y = –\(\frac{1}{2}\)x + c
To find the value of c,
Substitute (-5, 2) in the given equation
2 = –\(\frac{1}{2}\) (-5) + c
c = –\(\frac{1}{2}\)
Hence,
The equation of the line that is perpendicular to the given line equation is:
y = –\(\frac{1}{2}\)x – \(\frac{1}{2}\)
Question 10.
(- 1, – 9), y = – \(\frac{1}{3}\)x + 4
Answer:
The given equation is:
y= –\(\frac{1}{3}\)x + 4
The given point is: (-1, -9)
a) Parallel to the given line:
We know that,
The slopes of the parallel lines are the same
Compare the given equation with
y = mx + c
So,
The equation of the line that is parallel to the given line is:
y = –\(\frac{1}{3}\)x + c
To find the value of c,
Substitute (-1, -9) in the above equation
So,
-9 = –\(\frac{1}{3}\) (-1) + c
c = \(\frac{26}{3}\)
Hence,
The equation of the line that is parallel to the given line equation is:
y = –\(\frac{1}{3}\)x + \(\frac{26}{3}\)
b) Perpendicular to the given line:
We know that,
The product of the slopes of the perpendicular lines is equal to -1
So,
m1 m2 = -1
–\(\frac{1}{3}\)m2 = -1
m2 = 3
So,
The equation of the line that is perpendicular to the given line equation is:
y = 3x + c
To find the value of c,
Substitute (-1, -9) in the given equation
-9 = 3 (-1) + c
c = -6
Hence,
The equation of the line that is perpendicular to the given line equation is:
y = 3x – 6
Question 11.
A student says. “Because j ⊥ K, j ⊥ l’ What missing information is the student assuming from the diagram? Which theorem is the student trying to use?
Answer:
The given figure is:

It is given that a student claimed that j ⊥ K, j ⊥ l
We know that,
According to the “Perpendicular Transversal Theorem”,
In a plane, if a line is perpendicular to one of two parallel lines, then it is perpendicular to the other line also.
So,
From the above definition,
The missing information the student assuming from the diagram is:
The line l is also perpendicular to the line j
Hence, from the above,
We can conclude that the theorem student trying to use is the “Perpendicular Transversal Theorem”
Question 12.
You and your family are visiting some attractions while on vacation. You and your mom visit the shopping mall while your dad and your sister visit the aquarium. You decide to meet at the intersection of lines q and p. Each unit in the coordinate plane corresponds to 50 yards.
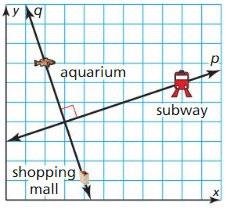
Answer:
You and your family are visiting some attractions while on vacation. You and your mom visit the shopping mall while your dad and your sister visit the aquarium. You decide to meet at the intersection of lines q and p. Each unit in the coordinate plane corresponds to 50 yards.
a. Find an equation of line q.
Answer:
From the figure,
The coordinates of line q are:
(50, 500), (200, 50)
Compare the given points with
(x1, y1), (x2, y2)
We know that,
Slope (m) = \(\frac{y2 – y1}{x2 – x1}\)
= \(\frac{50 – 500}{200 – 50}\)
= \(\frac{-450}{150}\)
= –\(\frac{45}{15}\)
= -3
Now,
We know that,
The standard form of the equation is:
y – y1 = m (x – x1)
So,
y – 500 = -3 (x -50)
y – 500 = -3x + 150
y = -3x + 150 + 500
y = -3x + 650
Hence, from the above,
We can conclude that
The equation of line q is:
y = -3x + 650
b. Find an equation of line p.
Answer:
From the figure,
The coordinates of line p are:
(50, 175), (500, 325)
Compare the given points with
(x1, y1), (x2, y2)
We know that,
Slope (m) = \(\frac{y2 – y1}{x2 – x1}\)
= \(\frac{325 – 175}{500 – 50}\)
= \(\frac{15}{45}\)
= \(\frac{1}{3}\)
Now,
We know that,
The standard form of the equation is:
y – y1 = m (x – x1)
So,
y – 175 = \(\frac{1}{3}\) (x -50)
3 (y – 175) = x – 50
3y – 525 = x – 50
3y = x – 50 + 525
3y = x + 475
y = \(\frac{1}{3}\)x + \(\frac{475}{3}\)
Hence, from the above,
We can conclude that
The equation of line p is:
y = \(\frac{1}{3}\)x + \(\frac{475}{3}\)
c. What are the coordinates of the meeting point?
Answer:
From the given figure,
We can conclue that
The coordinates of the meeting point are: (150. 200)
d. What is the distance from the meeting point to the subway?
Answer:
From the given figure,
The coordinates of the meeting point are: (150, 200)
The coordinates of the subway are: (500, 300)
Now,
The distance between the meeting point and the subway is:
d = \(\sqrt{(x2 – x1)² + (y2 – y1)²}\)
d = \(\sqrt{(300 – 200)² + (500 – 150)²}\)
d = 364.5 yards
Hence, from the above,
We can conclude that the distance between the meeting point and the subway is: 364.5 yards
Question 13.
Identify an example on the puzzle cube of each description. Explain your reasoning.
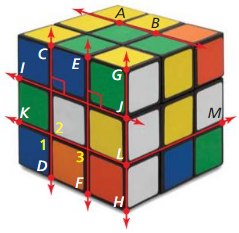
a. a pair of skew lines
Answer:
We know that,
The “Skew lines” are the lines that are non-intersecting, non-parallel and non-coplanar
Hence,
From the given figure,
We can conclude that the pair of skew lines are:
\(\overline{A B}\) and \(\overline{G H}\)
b. a pair of perpendicular lines
Answer:
We know that,
The “Perpendicular lines” are the lines that are intersected at the right angles
Hence,
From the given figure,
We can conclude that the pair of perpendicular lines are:
\(\overline{I J}\) and \(\overline{C D}\)
c. a pair of paralIeI lines
Answer:
We know that,
The “parallel lines” are the lines that do not have any intersection point
Hence,
From the given figure,
We can conclude that the pair of parallel lines are:
\(\overline{C D}\) and \(\overline{E F}\)
d. a pair of congruent corresponding angles
Answer:
From the given figure,
We can conclude that
∠1 and ∠3 are the corresponding angles
e. a pair of congruent alternate interior angles
Answer:
From the given figure,
We can conclude that
∠2 and ∠3 are the congruent alternate interior angles
Parallel and Perpendicular Lines Cumulative Assessment
Question 1.
Use the steps in the construction to explain how you know that\(\overline{C D}\) is the perpendicular bisector of \(\overline{A B}\).
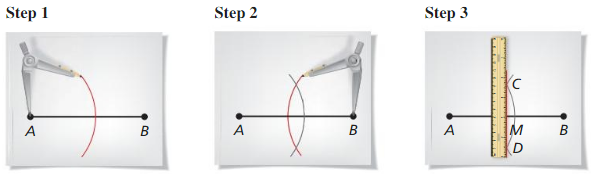
Answer:
Step 1:
Draw a line segment of any length and name that line segment as AB
Step 2:
Draw an arc by using a compass with above half of the length of AB by taking the center at A above AB
Step 3:
Draw another arc by using a compass with above half of the length of AB by taking the center at B above AB
Step 4:
Repeat steps 3 and 4 below AB
Step 5:
Draw a line segment CD by joining the arcs above and below AB
Step 6:
Measure the lengths of the midpoint of AB i.e., AD and DB.
By measuring their lengths, we can prove that CD is the perpendicular bisector of AB
Question 2.
The equation of a line is x + 2y = 10.
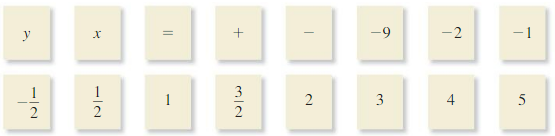
a. Use the numbers and symbols to create the equation of a line in slope-intercept form
that passes through the point (4, – 5) and is parallel to the given line.
Answer:
The given line equation is:
x + 2y = 10
The given point is: (4, -5)
Now,
The given equation in the slope-intercept form is:
y = –\(\frac{1}{2}\)x + 5
We know that,
The slopes of the parallel lines are the same
So,
The equation of the line that is parallel to the given line equation is:
y = –\(\frac{1}{2}\)x + c
To find the value of c,
Substitute (4, -5) in the above equation
So,
-5 = –\(\frac{1}{2}\) (4) + c
c = -5 + 2
c = -3
Hence, from the above,
We can conclude that the line that is parallel to the given line equation is:
y = –\(\frac{1}{2}\)x – 3
b. Use the numbers and symbols to create the equation of a line in slope-intercept form
that passes through the point (2, – 1) and is perpendicular to the given line.
Answer:
The given line equation is:
x + 2y = 10
The given point is: (4, -5)
Now,
The given equation in the slope-intercept form is:
y = –\(\frac{1}{2}\)x + 5
We know that,
The product of the slopes of the perpendicular lines is equal to -1
So,
m1m2 = -1
–\(\frac{1}{2}\) (m2) = -1
m2 = 2
So,
The equation of the line that is perpendicular to the given line equation is:
y = 2x + c
To find the value of c,
Substitute (4, -5) in the above equation
So,
-5 = 2 (4) + c
-5 – 8 = c
c = -13
Hence, from the above,
We can conclude that a line equation that is perpendicular to the given line equation is:
y = 2x – 13
Question 3.
Classify each pair of angles whose measurements are given.
a.
Answer:
The given figure is:

From the given figure,
We can conclude that 44° and 136° are the adjacent angles
b.
Answer:
The given figure is:

From the given figure,
We can conclude that 18° and 23° are the adjacent angles
c.
Answer:
The given figure is:

From the given figure,
We can conclude that 75° and 75° are alternate interior angles
d.
Answer:
The given figure is:

From the given figure,
We can conclude that 42° and 48° are the vertical angles
Question 4.
Your school is installing new turf on the football held. A coordinate plane has been superimposed on a diagram of the football field where 1 unit = 20 feet.
a. What is the length of the field?
Answer:
It is given that a coordinate plane has been superimposed on a diagram of the football field where 1 unit is 20 feet.
So,
From the given figure,
The length of the field = | 20 – 340 |
= 320 feet
Hence, from the above,
We can conclude that the length of the field is: 320 feet
b. What is the perimeter of the field?
Answer:
From the figure,
The width of the field is: 140 feet
From the figure,
We can observe that the figure is in the form of a rectangle
We know that,
The perimeter of the field = 2 ( Length + Width)
= 2 (320 + 140)
= 2 (460)
= 920 feet
Hence, from the above,
We can conclude that the perimeter of the field is: 920 feet
c. Turf costs $2.69 per square foot. Your school has a $1,50,000 budget. Does the school have enough money to purchase new turf for the entire field?
Answer:
We know that,
The area of the field = Length × Width
So,
The area of the field = 320 × 140
= 44,800 square feet
it is given that the turf costs $2.69 per square foot
So,
The total cost of the turf = 44,800 × 2.69
= $1,20,512
It is given that your school has a budget of $1,50,000 but we only need $1,20,512
Hence, from the above,
We can conclude that the school have enough money to purchase new turf for the entire field
Question 5.
Enter a statement or reason in each blank to complete the two-column proof.
Given ∠1 ≅∠3
Prove ∠2 ≅∠4

Answer:
The given table is:

Hence,
The completed table is:

Question 6.
Your friend claims that lines m and n are parallel. Do you support your friend’s claim? Explain your reasoning.
Answer:
Yes, I support my friend’s claim
Explanation:

From the given figure,
We can observe that 141° and 39° are the consecutive interior angles
We know that,
According to the consecutive Interior Angles Theorem,
If the sum of the angles of the consecutive interior angles is 180°, then the two lines that are cut by a transversal are parallel
Hence, from the above,
We can conclude that the claim of your friend can be supported
Question 7.
Which of the following is true when ![]() are skew?
are skew?
(A) ![]() are parallel.
are parallel.
(B) ![]() intersect
intersect
(C) ![]() are perpendicular
are perpendicular
(D) A, B, and C are noncollinear.
Answer:
We know that,
The “Skew lines” are the lines that are not parallel, non-intersect, and non-coplanar
Hene, from the given options,
We can conclude that option D) is correct because parallel and perpendicular lines have to be lie in the same plane
Question 8.
Select the angle that makes the statement true.
∠1 ∠2 ∠3 ∠4 ∠5 ∠6 ∠7 ∠8
a. ∠4 ≅ ________ b the Alternate Interior Angles Theorem (Thm. 3.2).
Answer:
From the given figure,
We can conclude that
By using the Alternate interior angles Theorem,
∠4 ≅ ∠5
b. ∠2 ≅ ________ by the Corresponding Angles Theorem (Thm. 3. 1)
Answer:
From the given figure,
We can conclude that
By using the Corresponding angles Theorem,
∠2 ≅ ∠6
c. ∠1 ≅ ________ by the Alternate Exterior Angles Theorem (Thm. 3.3).
Answer:
From the given figure,
We can conclude that
By using the Alternate exterior angles Theorem,
∠1 ≅ ∠8
d. m∠6 + m ________ = 180° by the Consecutive Interior Angles Theorem (Thm. 3.4).
Answer:
From the given figure,
We can conclude that
By using the Consecutive interior angles Theorem,
∠6 + ∠4 = 180°
Question 9.
You and your friend walk to school together every day. You meet at the halfway point between your houses first and then walk to school. Each unit in the coordinate plane corresponds to 50 yards.
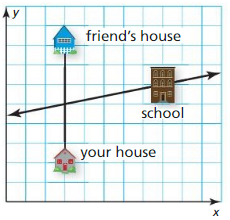
Answer:
It is given that you and your friend walk to school together every day. You meet at the halfway point between your houses first and then walk to school. Each unit in the coordinate plane corresponds to 50 yards.
a. What are the coordinates of the midpoint of the line segment joining the two houses?
Answer:
From the given figure,
We can conclude that the midpoint of the line segment joining the two houses is:
M = (150, 250)
b. What is the distance that the two of you walk together?
Answer:
From the given figure,
We can observe that
The coordinates of the school = (400, 300)
The coordinates of the midpoint of the line segment joining the two houses = (150, 250)
It is given that the two friends walk together from the midpoint of the houses to the school
Now,
We know that,
The distance that the two of you walk together is:
d = \(\sqrt{(x2 – x1)² + (y2 – y1)²}\)
= \(\sqrt{(250 – 300)² + (150 – 400)²}\)
= \(\sqrt{2500 + 62,500}\)
= 255 yards
Hence, from the above,
We can conclude that the distance that the two of the friends walk together is: 255 yards